Question

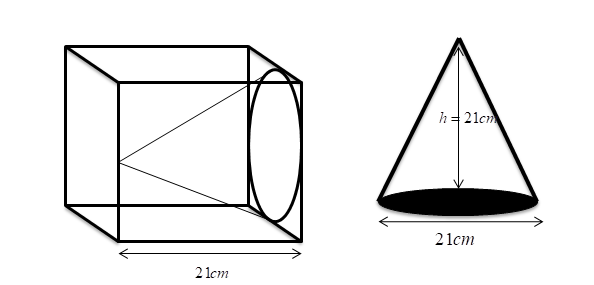
The largest cone is curved out from one face of a solid cube of side 21cm. Find the volume of the remaining solid.

Answer 1

Hint: To solve this question, we will first find the area of the cube and then we will find the area of the cone. We will subtract the area to find the area of the remaining solid. Here when we will go for curving out the largest cone, the maximum diameter that can be curved out will be equal to the side of the cube.

Complete step-by-step answer:
Formula used- volume of cone = $ \dfrac{1}{3}\pi {r^2}h $
Where
r is the radius
h is the height of the cone
Volume of the cube = $ {\left( {side} \right)^3} $
Given
Side of cube s =21cm
For the largest cone
 Side of the cube = diameter of the cone = 21cm
Radius of the cone = $ \dfrac{{21}}{2}cm $
And height of the cone = $ 21cm $

The volume of the cone is given by
 $
  {V_{cone}} = \dfrac{1}{3}\pi {r^2}h \\
  {V_{cone}} = \dfrac{1}{3}\pi \times {\left( {\dfrac{{21}}{2}} \right)^2} \times 21 \\
  {V_{cone}} = 2424.52c{m^3} \\
 $
The volume of the cube is given by
 $
  {V_{cube}} = {\left( {side} \right)^3} \\
  {V_{cube}} = {\left( {21} \right)^3} \\
  {V_{cube}} = 9261c{m^3} \\
 $
The volume of the remaining solid is given as
 $
  {V_{remaining\,\,solid}} = {V_{cube}} - {V_{cone}} \\
  {V_{remaining\,\,solid}} = 9261 - 2424.52 \\
  {V_{remaining\,\,solid}} = 6836.48c{m^3} \\
  $
Hence, the volume of the remaining solid is $ 6836.48c{m^3} $

Note: In order to solve these types of questions, remember the formula to find the area, volume of the triangle, cube, cone, square and many more figures. Read the question carefully and extract all the data given in the question required to find the solution. Also remember, when you need to frame an equation and find the solution of the equations; to find the solutions the number of equations framed must be equal to the number of variables.