
The length, breadth and height of a cuboid are in the ratio 5 : 4 : 2 and the total surface area is 1216
A.2460
B.2560
C.2660
D.2700
Answer
520.8k+ views
Hint: In the above question assume the length, breadth and height as 5x, 4x and 2x of the given cuboid and find the value of x by equating the given total surface area. Now use the formula of the volume of the cuboid and find its volume. The formulae of the total surface area and the volume of the cuboid are as follows:
Complete step-by-step answer:
Let us consider the length, breadth and height of a cuboid to be l, b and h. Then,
Now, we have been given that the length, breadth and height of a cuboid are in the ratio 5 : 4 : 2 and total surface area is 1216
Let us assume the length, breadth and height of a cuboid are 5x, 4x and 2x respectively. So, we have the figure as
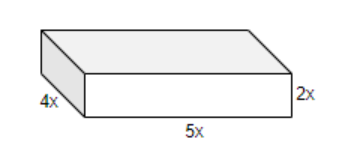
Then, we can write the total surface area =
So, the length, breadth and height of the cuboid after substituting the value of x, are as follows:
Length = 20 cm
Breadth = 16 cm
Height = 8 cm
Now, the volume of the cuboid =
Therefore, the correct option of the above question is option B.
Note: Just be careful while doing calculation as there is a chance that you might make a mistake and you will get the incorrect answer. You should read the question carefully, by mistake if you read it as volume is given as 1216, then you will formulate the wrong equation and get a different value of x.
Complete step-by-step answer:
Let us consider the length, breadth and height of a cuboid to be l, b and h. Then,
Now, we have been given that the length, breadth and height of a cuboid are in the ratio 5 : 4 : 2 and total surface area is 1216
Let us assume the length, breadth and height of a cuboid are 5x, 4x and 2x respectively. So, we have the figure as

Then, we can write the total surface area =
So, the length, breadth and height of the cuboid after substituting the value of x, are as follows:
Length = 20 cm
Breadth = 16 cm
Height = 8 cm
Now, the volume of the cuboid =
Therefore, the correct option of the above question is option B.
Note: Just be careful while doing calculation as there is a chance that you might make a mistake and you will get the incorrect answer. You should read the question carefully, by mistake if you read it as volume is given as 1216, then you will formulate the wrong equation and get a different value of x.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




