
The length of one side of a rhombus is 3.5 cm and its altitude is 10 cm. If the length of one of its diagonals is 26 cm, what will be the length of the other diagonal?
a) 5 cm
b) 10 cm
c) 8 cm
d) 6cm
Answer
526.5k+ views
1 likes
Hint: Area of the rhombus in terms of base and height is given as;
Complete step by step answer:
Here, it is given that the length of an altitude on the side of rhombus of length 6.5 cm, is 10 cm. And we need to determine the length of a diagonal if the length of another diagonal is 26 cm. so, we can draw an sketch as
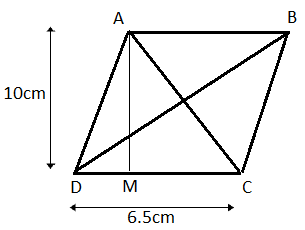
So, let us suppose a Rhombus ABCD with side DC = 6.5 cm and altitude AD is of length 10 cm on it. And we need to determine length BD if AC = 26 cm. We know the area of rhombus can be given two ways. One in form of base and corresponding height and another in terms of both the diagonals. So, both can be given as
So, area with side DC and AM as a corresponding altitude from the first relation of the area can be given as
Area of rhombus ABCD
Similarly, area of rhombus with the help of diagonals BD and AC can be given from the second relation as
Area of rhombus ABCD
So, equating both the areas calculated above, as both are representing the area of rhombus ABCD. Hence we get
Hence, the length of the other diagonal is 5 cm.
So option (a) is correct.
Note: Area of rhombus can be given in terms of diagonals and side corresponding altitude as well. We need to know both the identities to solve this kind of question. One may confuse with the properties of diagonals of the rhombus and may give length another diagonal as 26 cm as well. As he or she may think that rhombus has equal diagonals. But it’s not true, Diagonals of rhombus are not equal to each other. Rectangle and square have equal diagonals only. So be careful with the properties of the rhombus as well.
Complete step by step answer:
Here, it is given that the length of an altitude on the side of rhombus of length 6.5 cm, is 10 cm. And we need to determine the length of a diagonal if the length of another diagonal is 26 cm. so, we can draw an sketch as

So, let us suppose a Rhombus ABCD with side DC = 6.5 cm and altitude AD is of length 10 cm on it. And we need to determine length BD if AC = 26 cm. We know the area of rhombus can be given two ways. One in form of base and corresponding height and another in terms of both the diagonals. So, both can be given as
So, area with side DC and AM as a corresponding altitude from the first relation of the area can be given as
Area of rhombus ABCD
Similarly, area of rhombus with the help of diagonals BD and AC can be given from the second relation as
Area of rhombus ABCD
So, equating both the areas calculated above, as both are representing the area of rhombus ABCD. Hence we get
Hence, the length of the other diagonal is 5 cm.
So option (a) is correct.
Note: Area of rhombus can be given in terms of diagonals and side corresponding altitude as well. We need to know both the identities to solve this kind of question. One may confuse with the properties of diagonals of the rhombus and may give length another diagonal as 26 cm as well. As he or she may think that rhombus has equal diagonals. But it’s not true, Diagonals of rhombus are not equal to each other. Rectangle and square have equal diagonals only. So be careful with the properties of the rhombus as well.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




