
The perimeter of a school volleyball court is 177ft and the length is twice the width. What are the dimensions of the volleyball court?
Answer
504.3k+ views
Hint: We know the perimeter of a rectangular school volleyball court is
Complete step-by-step answer:
According to the question the perimeter of a school volleyball court is 177ft.
Let us assume that the width of the volleyball field is
From the question we know that length is twice the width. So the length is
Now let us imagine the volleyball field layout by the diagram below.
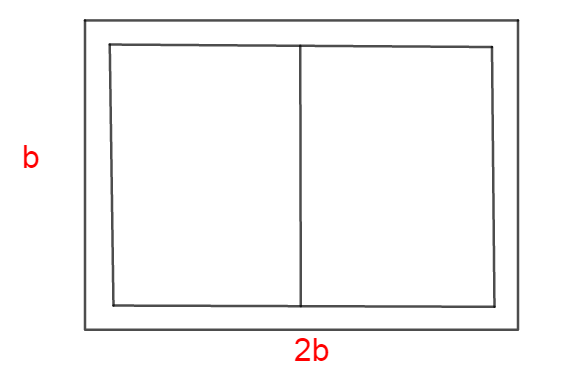
We know the formula of perimeter of a rectangular field is
From the question we know the perimeter of a school volleyball court is 177ft.
Now replacing the value of “P” with the value 177 we get,
Now put the assumed value of length and width of school volleyball court we get,
So the width of the school volleyball court is 29.5ft.
We know that length is twice the width.
The length of the school volleyball court is
The length and the width of the school volleyball court is 59ft and 29.5ft respectively.
Note: We can assume the value of length
Perimeter is given 177ft. putting all the values in the formulae we get
Length is 59ft. Width is
The length and the width of the school volleyball court is 59ft and 29.5ft respectively.
Complete step-by-step answer:
According to the question the perimeter of a school volleyball court is 177ft.
Let us assume that the width of the volleyball field is
From the question we know that length is twice the width. So the length is
Now let us imagine the volleyball field layout by the diagram below.

We know the formula of perimeter of a rectangular field is
From the question we know the perimeter of a school volleyball court is 177ft.
Now replacing the value of “P” with the value 177 we get,
Now put the assumed value of length and width of school volleyball court we get,
So the width of the school volleyball court is 29.5ft.
We know that length is twice the width.
The length of the school volleyball court is
The length and the width of the school volleyball court is 59ft and 29.5ft respectively.
Note: We can assume the value of length
Perimeter is given 177ft. putting all the values in the formulae we get
Length is 59ft. Width is
The length and the width of the school volleyball court is 59ft and 29.5ft respectively.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What was the capital of the king Kharavela of Kalinga class 9 social science CBSE




