Question

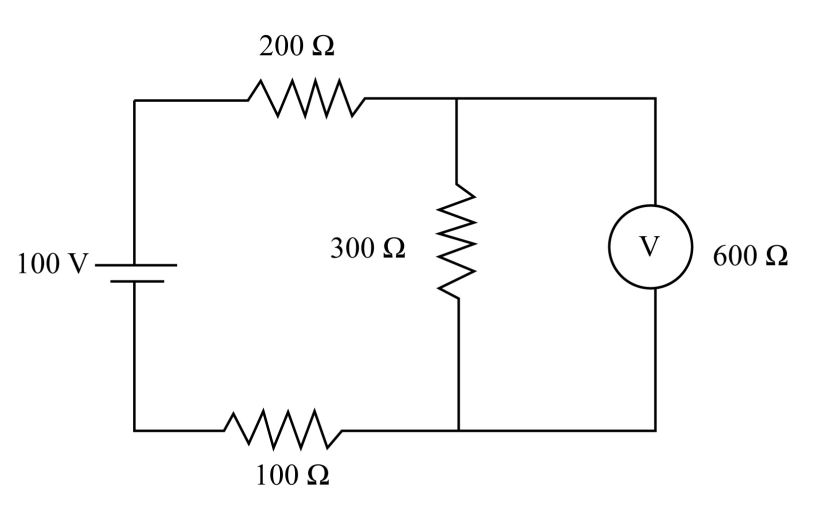
The reading of the voltmeter is

Answer 1

Hint: As we know when the resistors are connected into series, the same current flows through them, but when the resistors are connected into parallel, different values of current flow through them as the potential drop across each resistor is same when the resistors are connected in parallel.

Complete step by step answer:
We all have studied to know that if two resistors $R_1$ and $R_2$ are connected in parallel, then the net resistance $R_p$ is given by the formula:
$ \Rightarrow {R_p} = \dfrac{{{R_1}{R_2}}}{{{R_1} + {R_2}}}$
As we can see in the given problem, here 300 ohms and 600-ohm voltmeter are connected in parallel, so the net resistance can be found out by substituting $R_1=600$ ohm and $R_2=300$ ohm to find $R_p$. Therefore, it becomes,
$ \Rightarrow {R_p} = \dfrac{{600\Omega \times 300\Omega }}{{600\Omega + 300\Omega }}$
$ \Rightarrow {R_p} = \dfrac{{300 \times 6}}{9}$
As we will simplify the above equation, then it will become,
$\therefore {R_p} = 200\Omega $
We all have studied to know that if three resistors $R_1$ and $R_2$ and $R_p$ are connected in series, then the net resistance $R_s$ is given by the formula:
${R_s} = {R_1} + {R_2} + {R_p}$ …… (I)
We will now substitute${R_p} = 200\Omega $, ${R_1} = 200\Omega $ and ${R_2} = 100\Omega $ in equation (I) to find $R_s$.
$ \Rightarrow {R_s} = \left( {100 + 200 + 200} \right)\Omega $
$ \Rightarrow {R_s} = 500\Omega $
Now we can calculate the current through the whole circuit by applying ohm’s law. We know that,
$I = \dfrac{V}{{{R_s}}}$
We will now substitute ${R_s} = 500\Omega $ and $V = 100V$ to find the current I.
$I = \dfrac{{100V}}{{500\Omega }}$
$I = 0.2A$
This is the current through the whole circuit. Now the voltage reading of the voltmeter can be calculated by the formula,
${V_R} = I{R_V}$
In this equation, we can see that ${V_R}$ it is the voltmeter reading, and ${R_V}$ is the voltmeter resistance. We will now substitute ${R_V} = 200\Omega $ and $I = 0.2A$ to find the value of ${V_R}$.
$ \Rightarrow {V_R} = 0.2A \times 200\Omega $
$ \Rightarrow {V_R} = 40V$

Therefore the voltmeter reading is 40 V.

Note:
We can see that in the given problem as the resistors are connected into a series along a single path, so the equal current flows through all of the resistors. The current passing through every component is the same, so the net voltage in the whole circuit is the sum of the entire voltage drop across these components. In a parallel circuit, the total current is the sum of all the current in each of these components. Since the voltmeter has a resistance, hence it is also considered as a separate resistor.