
The semi perimeter of a triangle having the length of its sides as 20 cm, 15 cm and 9 cm is :
A.44 cm
B.21 cm
C.22 cm
D.24 cm
Answer
485.7k+ views
Hint: We recall the dentition of length of sides of a triangle, the perimeter of the triangle and then semi-perimeter. We add the given lengths of triangles to get the perimeter of the triangle and divide the perimeter by 2 to get the semi-perimeter.
Complete step by step answer:
We know that the perimeter of any geometrical shape in a plane is the length of outline of the shape. We know that the triangle is a polygon with three vertices and the lien segment which joins those vertices are called sides. The distance between two vertices is called the length of the side.
The outline of the triangle is the three sides of the triangle. The perimeter of the triangle is the length of the whole outline that is the sum of the lengths of the sides of a triangle. If
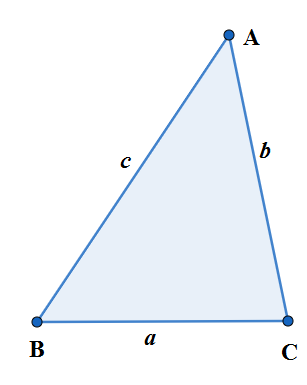
We know that the word ‘semi’ means half. That is why the word semi-perimeter means half of the perimeter. The semi-perimeter of triangle is generally denoted as
We are given in the question the length of three sides of the triangle sides as 20 cm, 15 cm and 9 cm. We can assign in our choice
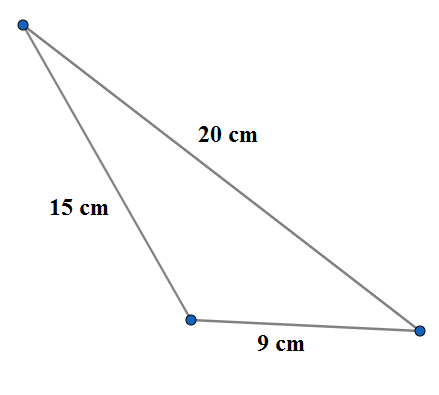
So, the correct answer is “Option C”.
Note: We can use the semi-perimeter of the triangle to find the area of the triangle using the formula
Complete step by step answer:
We know that the perimeter of any geometrical shape in a plane is the length of outline of the shape. We know that the triangle is a polygon with three vertices and the lien segment which joins those vertices are called sides. The distance between two vertices is called the length of the side.
The outline of the triangle is the three sides of the triangle. The perimeter of the triangle is the length of the whole outline that is the sum of the lengths of the sides of a triangle. If

We know that the word ‘semi’ means half. That is why the word semi-perimeter means half of the perimeter. The semi-perimeter of triangle is generally denoted as
We are given in the question the length of three sides of the triangle sides as 20 cm, 15 cm and 9 cm. We can assign in our choice

So, the correct answer is “Option C”.
Note: We can use the semi-perimeter of the triangle to find the area of the triangle using the formula
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




