Answer
113.7k+ views
Hint: If the water in the jug is at a certain height, it will cause the water to flow through the hole with a certain velocity and as a result, the height will decrease at a certain rate. The volume of water flowing out through the orifice will be proportional to the decrease in height of water in the jug. We will make use of this property to find our answer.
Formula Used: \[{{v}_{y}}=\sqrt{2gy}\] , \[V={{v}_{y}}\times A\]
Complete step by step solution:
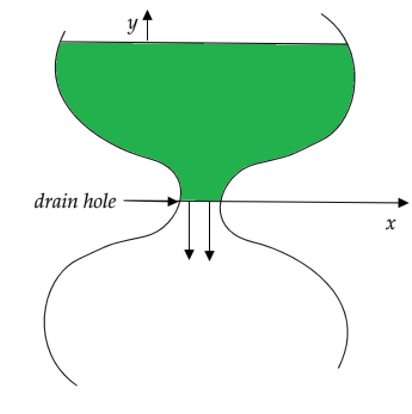
Let the height of the water column in the jug at a certain instant of time, say \[t=0\] , is \[y\]
The velocity of the water through the drain hole will be \[\sqrt{2gy}\]
Radius of the drain hole \[(r)=2mm=2\times {{10}^{-3}}m\left[ \because 1mm={{10}^{-3}}m \right]\]
Hence area of the drain hole \[(A)=\pi {{r}^{2}}\]
Substituting the values, we get
\[\begin{align}
& A=\pi \times {{(2\times {{10}^{-3}})}^{2}} \\
& \Rightarrow A=\pi \times 4\times {{10}^{-6}} \\
& \Rightarrow A=1.26\times {{10}^{-5}}{{m}^{2}} \\
\end{align}\]
The volume of the water flowing through the orifice will be equal to the product of the velocity of the water through the drain hole and the area of the drain hole, that is
Volume of water flown \[(V)=A.\sqrt{2gy}\]
Now, the corresponding decrease in the water in the mug can be given as \[\pi {{x}^{2}}\times \left( -\dfrac{dy}{dt} \right)\] where \[\dfrac{dy}{dt}\] is the infinitesimal decrease in the height of the water column and is the area of the open surface of water in the jug at that instant in time.
Rate of fall of water level (per second) \[\left( -\dfrac{dy}{dt} \right)=\dfrac{4cm}{3600s}=\dfrac{4\times {{10}^{-2}}m}{3600s}=1.11\times \times {{10}^{-5}}m/s\left[ \because 1cm={{10}^{-2}}m;1hr=3600s \right]\]
As already discussed, the water flowing out must be equal to the corresponding decrease in the water from the jug, that is
\[A.\sqrt{2gy}=\pi {{x}^{2}}\times \left( -\dfrac{dy}{dt} \right)\]
We have our variables in the above equation and the other quantities are known to us, hence substituting the values, we will get
\[\begin{align}
& 1.26\times {{10}^{-5}}\times \sqrt{2\times 9.8\times y}=\pi \times {{x}^{2}}\times 1.11\times \times {{10}^{-5}} \\
& \Rightarrow \sqrt{19.6\times y}=2.77{{x}^{2}} \\
\end{align}\]
Squaring both sides and simplifying further, we have
\[y=0.4{{x}^{2}}\]
This is the equation for the shape or the curve of the jar.
Note: We were given the rate of fall of water level for an hour, but we always consider the rate of change in terms of seconds, so we had to convert it into seconds. Some students directly put the value of the rate of fall of water level given to us in hours and reach the wrong answer even after using the correct approach. So always make sure that you’re analytically as well as logically correct. Also, note that the negative sign with the rate of change of water level only indicates that the level is falling.
Formula Used: \[{{v}_{y}}=\sqrt{2gy}\] , \[V={{v}_{y}}\times A\]
Complete step by step solution:
Let the height of the water column in the jug at a certain instant of time, say \[t=0\] , is \[y\]
The velocity of the water through the drain hole will be \[\sqrt{2gy}\]
Radius of the drain hole \[(r)=2mm=2\times {{10}^{-3}}m\left[ \because 1mm={{10}^{-3}}m \right]\]
Hence area of the drain hole \[(A)=\pi {{r}^{2}}\]
Substituting the values, we get
\[\begin{align}
& A=\pi \times {{(2\times {{10}^{-3}})}^{2}} \\
& \Rightarrow A=\pi \times 4\times {{10}^{-6}} \\
& \Rightarrow A=1.26\times {{10}^{-5}}{{m}^{2}} \\
\end{align}\]
The volume of the water flowing through the orifice will be equal to the product of the velocity of the water through the drain hole and the area of the drain hole, that is
Volume of water flown \[(V)=A.\sqrt{2gy}\]
Now, the corresponding decrease in the water in the mug can be given as \[\pi {{x}^{2}}\times \left( -\dfrac{dy}{dt} \right)\] where \[\dfrac{dy}{dt}\] is the infinitesimal decrease in the height of the water column and is the area of the open surface of water in the jug at that instant in time.
Rate of fall of water level (per second) \[\left( -\dfrac{dy}{dt} \right)=\dfrac{4cm}{3600s}=\dfrac{4\times {{10}^{-2}}m}{3600s}=1.11\times \times {{10}^{-5}}m/s\left[ \because 1cm={{10}^{-2}}m;1hr=3600s \right]\]
As already discussed, the water flowing out must be equal to the corresponding decrease in the water from the jug, that is
\[A.\sqrt{2gy}=\pi {{x}^{2}}\times \left( -\dfrac{dy}{dt} \right)\]
We have our variables in the above equation and the other quantities are known to us, hence substituting the values, we will get
\[\begin{align}
& 1.26\times {{10}^{-5}}\times \sqrt{2\times 9.8\times y}=\pi \times {{x}^{2}}\times 1.11\times \times {{10}^{-5}} \\
& \Rightarrow \sqrt{19.6\times y}=2.77{{x}^{2}} \\
\end{align}\]
Squaring both sides and simplifying further, we have
\[y=0.4{{x}^{2}}\]
This is the equation for the shape or the curve of the jar.
Note: We were given the rate of fall of water level for an hour, but we always consider the rate of change in terms of seconds, so we had to convert it into seconds. Some students directly put the value of the rate of fall of water level given to us in hours and reach the wrong answer even after using the correct approach. So always make sure that you’re analytically as well as logically correct. Also, note that the negative sign with the rate of change of water level only indicates that the level is falling.
Recently Updated Pages
JEE Main 2023 January 25 Shift 1 Question Paper with Answer Key

Geostationary Satellites and Geosynchronous Satellites for JEE

Complex Numbers - Important Concepts and Tips for JEE

JEE Main 2023 (February 1st Shift 2) Maths Question Paper with Answer Key

JEE Main 2022 (July 25th Shift 2) Physics Question Paper with Answer Key

Inertial and Non-Inertial Frame of Reference for JEE

Trending doubts
JEE Main Chemistry Question Paper with Answer Keys and Solutions

Average and RMS Value for JEE Main

Inductive Effect and Acidic Strength - Types, Relation and Applications for JEE

Charging and Discharging of Capacitor

Displacement-Time Graph and Velocity-Time Graph for JEE

Clemmenson and Wolff Kishner Reductions for JEE

Other Pages
NCERT Solutions for Class 11 Physics Chapter 4 Laws of Motion

NCERT Solutions for Class 11 Physics Chapter 3 Motion In A Plane

A solid sphere of radius r made of a soft material class 11 physics JEE_MAIN

A particle performs SHM of amplitude A along a straight class 11 physics JEE_Main

JEE Main 2023 April 6 Shift 1 Question Paper with Answer Keys & Solutions

Thermodynamics Class 11 Notes CBSE Physics Chapter 11 (Free PDF Download)