
The sides of an equilateral triangle ABC are 12 cm each , D is the foot of the perpendicular from A to BC and E is the midpoint of AD. Then BE is
A.
B.
C.
D.None of these
Answer
504.3k+ views
Hint: Since we are given an equilateral triangle ABC and AD is perpendicular to BC. By using the property the height of an equilateral triangle bisects its base we get BD=6cm and then by using Pythagoras theorem we can find AD and then considering the small triangle BDE and using Pythagoras theorem we can find BE
Complete step-by-step answer:
Given ABC is an equilateral triangle .
Hence all its sides are equal.
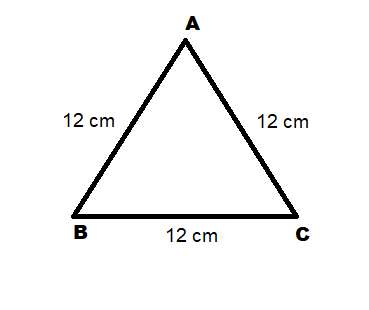
And it's also given that D is the foot of the perpendicular from A to BC
It means that AD is perpendicular to BC
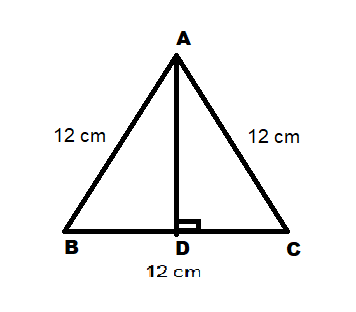
Step 2 :
Since ABC is an equilateral triangle , we know that the height or altitude of an equilateral triangle bisects its base.
Here AD is the height of the triangle .
Therefore it bisects the base and now we have BD=BC=6cm
Step 3:
Now let's find AD by using Pythagoras theorem
Consider the triangle ABD . It is a right triangle .
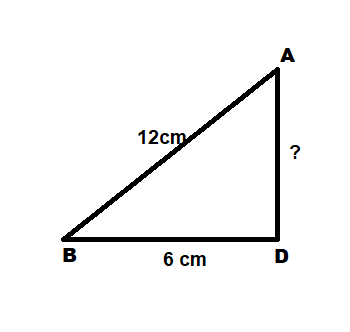
By using Pythagoras theorem
Now we have that
Step 4 :
We are given that E is the midpoint of AD.
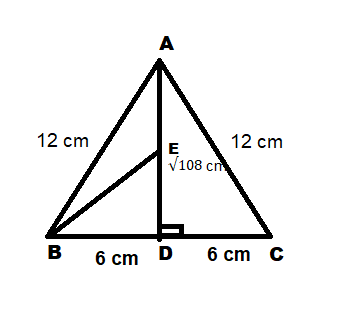
Since E is the midpoint of AD , we have AE=ED=
We need to find BE , so let's consider the right triangle BDE
Lets use Pythagoras theorem to find BE
Now by taking lcm we get
Therefore
The correct option is C
Note: The sides of an equilateral triangle are congruent.
An equilateral triangle is a special case of a triangle where all 3 sides have equal length and all 3 angles are equal to 60 degrees.
Complete step-by-step answer:
Given ABC is an equilateral triangle .
Hence all its sides are equal.

And it's also given that D is the foot of the perpendicular from A to BC
It means that AD is perpendicular to BC

Step 2 :
Since ABC is an equilateral triangle , we know that the height or altitude of an equilateral triangle bisects its base.
Here AD is the height of the triangle .
Therefore it bisects the base and now we have BD=BC=6cm
Step 3:
Now let's find AD by using Pythagoras theorem
Consider the triangle ABD . It is a right triangle .

By using Pythagoras theorem
Now we have that
Step 4 :
We are given that E is the midpoint of AD.

Since E is the midpoint of AD , we have AE=ED=
We need to find BE , so let's consider the right triangle BDE
Lets use Pythagoras theorem to find BE
Now by taking lcm we get
Therefore
The correct option is C
Note: The sides of an equilateral triangle are congruent.
An equilateral triangle is a special case of a triangle where all 3 sides have equal length and all 3 angles are equal to 60 degrees.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




