
The slope of a distance-time graph of a moving body gives its
A. Speed
B. Displacement
C. Velocity
D. Acceleration
Answer
509.1k+ views
Hint: Slope of a graph between two variables
Complete answer:
Two physical quantities are plotted on a graph with
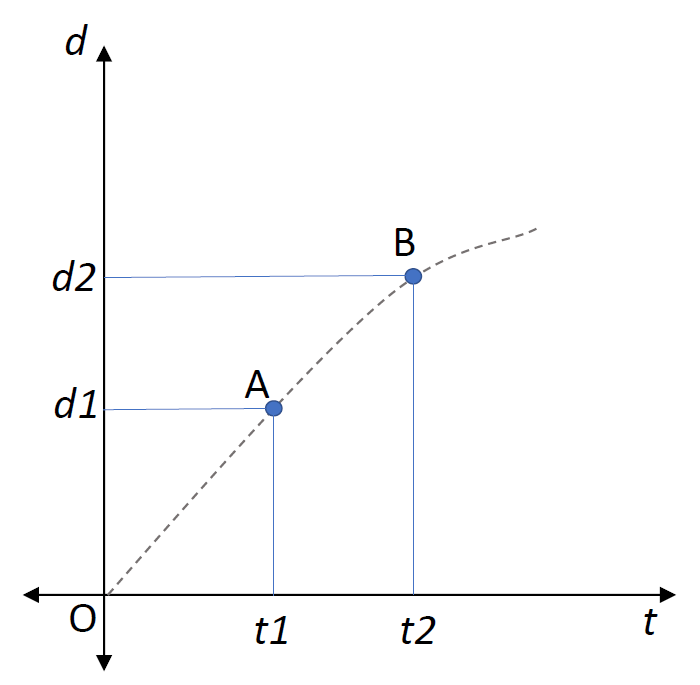
Let us consider an object started moving at
Note: We mentioned averaged speed because distance covered may be distributed through the time between A and B. Here we gave a simple example of a straight line. But actually, the line may not be straight in most of the cases.
Complete answer:
Two physical quantities are plotted on a graph with

Let us consider an object started moving at
Note: We mentioned averaged speed because distance covered may be distributed through the time between A and B. Here we gave a simple example of a straight line. But actually, the line may not be straight in most of the cases.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




