
The square root of 1764 is:
(a) 32
(b) 42
(c) 48
(d) 38
Answer
505.5k+ views
Hint: First of all, divide the digits into pairs of two starting from the rightmost digit. Then select the largest number as the divisor whose square is less than or equal to the pair on the extreme left. Repeat these steps after doubling the divisor each time to get the desired result.
Complete step-by-step answer:
Here, we have to find the square root of 1764.
1. First of all, we have to group the digits of numbers in pairs, starting with the digits in units. For this, we will place a bar over the pair of digits starting from the unit’s digit.
\[\overline{17}\text{ }\overline{64}\]
2. Now, we will take the largest number as the divisor whose square is less than or equal to the number on the extreme left that is 17. So, 17 is our dividend. Divide and write the quotient. Here, we will get the quotient 4 and remainder as 1. We will do it as follows:
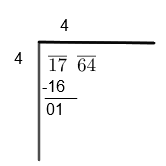
3. We then bring down the digits which are under the next bar to the right side of the remainder as follows:
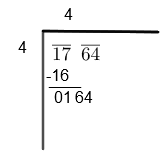
4. Now double the value of the quotient and write it with a blank on the right side. We will do it as follows:
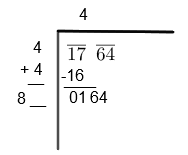
5. Now, we have to select the largest digit for the unit’s place of the divisor (8_) such that the new number when multiplied by the new unit’s digit is equal to or less than 164. In this case, \[82\times 2=164\]. So our digit is 2. We will do it as follows:
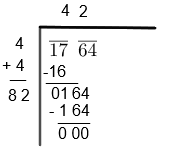
6. Now our remainder is 0 and we have no number left for multiplication. Therefore, we get,
\[\sqrt{1764}=42\]
Therefore, option (b) is the right answer.
Note: Students can also solve the question by prime factorization method as follows:

So we can write,
\[1764=2\times 2\times 3\times 3\times 7\times 7={{2}^{2}}{{.3}^{2}}{{.7}^{2}}\]
Complete step-by-step answer:
Here, we have to find the square root of 1764.
1. First of all, we have to group the digits of numbers in pairs, starting with the digits in units. For this, we will place a bar over the pair of digits starting from the unit’s digit.
\[\overline{17}\text{ }\overline{64}\]
2. Now, we will take the largest number as the divisor whose square is less than or equal to the number on the extreme left that is 17. So, 17 is our dividend. Divide and write the quotient. Here, we will get the quotient 4 and remainder as 1. We will do it as follows:

3. We then bring down the digits which are under the next bar to the right side of the remainder as follows:
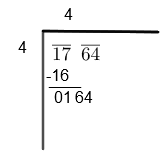
4. Now double the value of the quotient and write it with a blank on the right side. We will do it as follows:

5. Now, we have to select the largest digit for the unit’s place of the divisor (8_) such that the new number when multiplied by the new unit’s digit is equal to or less than 164. In this case, \[82\times 2=164\]. So our digit is 2. We will do it as follows:
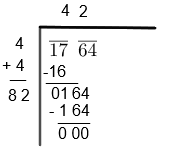
6. Now our remainder is 0 and we have no number left for multiplication. Therefore, we get,
\[\sqrt{1764}=42\]
Therefore, option (b) is the right answer.
Note: Students can also solve the question by prime factorization method as follows:

So we can write,
\[1764=2\times 2\times 3\times 3\times 7\times 7={{2}^{2}}{{.3}^{2}}{{.7}^{2}}\]
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

How do you graph the function fx 4x class 9 maths CBSE

Difference Between Plant Cell and Animal Cell

What is pollution? How many types of pollution? Define it

What is the color of ferrous sulphate crystals? How does this color change after heating? Name the products formed on strongly heating ferrous sulphate crystals. What type of chemical reaction occurs in this type of change.

Which places in India experience sunrise first and class 9 social science CBSE




