Question

The surface of a lake is at $2^\circ C$ and the depth of the lake is 20 m. Find the temperatures of the bottom of the lake?
A. $552^\circ C$
B. $42^\circ C$
C. $4^\circ C$
D. $825^\circ C$

Answer 1

Hint: The geothermal gradient is the term used when the earth gets hotter as one travels towards the core. But in water, the condition is different. Water is a very poor conductor of heat and it has a greater capacity to hold energy. Thus after 2m depth, the water temperature remains the same. With this, we can find a solution to the above question.

Complete step by step answer:
In the lake, 2 meters deep, $98\% $ of the energy has been absorbed and transformed into heat.
The water near the surface warmed by the sun is less dense than water near the bottom because the density of water changes as the temperature of the water changes.
In the lower temperature of the water, the higher the density of that water – until around $4^\circ C$. At $4^\circ C$, water reaches its maximum density. Water below $4^\circ C$ is lighter than water at$4^\circ C$ the water molecules begin to form into ice crystals.

 

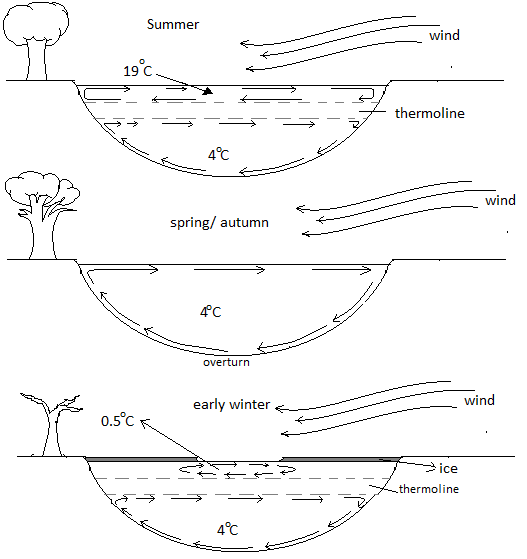
The temperature of the bottom of the lake depends on several factors like the depth of the lake, how long has the temperature been at $2^\circ C$, is any movement in the water like any streams entering or leaving the lake. The deep is denser and no heat sources or no movement caused in the deep, thus the bottom of the lake is twice the above surface temperature.
Thus the bottom of the lake temperature is $4^\circ C$ (3.98 to be more accurate).

So, the correct answer is Option C.

Note: The geothermal gradient that the increase in temperature with an increase in depth in the earth is not equal all over the world. On average, the temperature increases $2^\circ C - 3^\circ C$ per 100 m in depth. But on the water surface, the temperature remains constant.