Question

The tamer of wild animals has to bring one by one 5 lions and 4 tigers to the circle arena. The number of ways this can be done if no two tigers immediately follow each other is ...

Answer 1

Hint: In this question, we have to arrange 5 lions and 4 tigers in such a way that two tigers do not follow each other. For this, we will combine to specify position for lions and tigers and then calculate the number of ways it can be done. We will draw a small diagram to understand the concept. Formula of combination that will be used when we have to pick r position n is given by ${}^{n}{{C}_{r}}=\dfrac{n!}{r!\left( n-r \right)!}$.

Complete step-by-step answer:
Here we have to arrange 5 lions and 4 tigers in such a way that no two tigers follow immediately. Hence, there is always a lion between two tigers. Let us draw a small diagram to understand the concept.

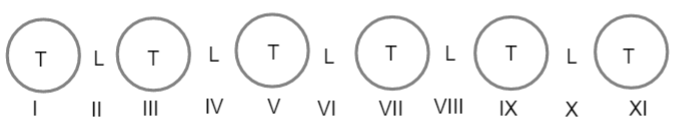
As there are four tigers and for them to not follow each other, we have six positions that are I, III, V, VII, IX and XI. But we can apply for 4 positions only. Hence, the number of ways 4 positions can be selected out of 6 positions becomes equal to ${}^{6}{{C}_{4}}$.
Since, all tigers are different. So, for four positions, the number of ways one tiger can occupy any of the four positions is 4. For the second tiger, the number of positions left is 3 and hence, the number of ways is 3. For the third tiger, the number of ways becomes 2 and for the last number of ways are 1. Hence, the number of ways tigers can be given four positions is $4\times 3\times 2\times 1=4!$.
Similarly, lions have five position and number of ways lions can occupy position are $5\times 4\times 3\times 2\times 1=5!$
So, we have estimated all possibilities and concluded that there are three possibilities included which are:
Ways in which tiger can occupy 4 positions out of 6 = ${}^{6}{{C}_{4}}$.
Ways in which 4 tigers occupy position = 4!
Ways in which 5 lions occupy position = 5!
Hence, total number of ways in which lions and tigers are to bring one by one to circle so that no two tiger follow each other becomes equal to
\[\begin{align}
  & {}^{6}{{C}_{4}}\times 4!\times 5! \\
 & \Rightarrow \dfrac{6!}{4!\times \left( 6-4 \right)!}\times 4!\times 5! \\
 & \Rightarrow \dfrac{6\times 5\times 4!}{4!\times 2!}\times 4!\times 5! \\
 & \Rightarrow 43200 \\
\end{align}\]
Hence, our required answer is 43200.

Note: Students should be careful while considering every possibility. Make sure that all possibilities are covered. After that we have to multiply all the ways and not add to get our final answer. Students should try to draw small diagrams for understanding all possibilities. Students should try to remember factorial values of 4!, 5! to make calculation easier.