
The two legs of a right triangle are equal and the square of its hypotenuse is 50. Find the length of each leg.
Answer
505.8k+ views
Hint: We will first construct the figure of the right-angled triangle. We are given that two sides of the right triangle are equal and the value of hypotenuse is given. So, we will let the sides of the triangle be
Complete step by step answer:
We will first start by considering the given data that two sides are equal to the right triangle and the square of the hypotenuse is given as 50.
We will draw the figure as follows,
So, we will let the two sides of the right triangle that is base and height as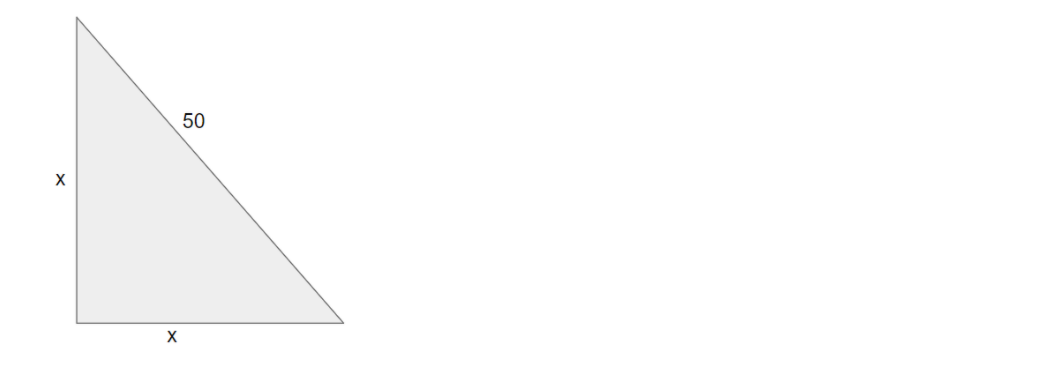
Now, we will apply the Pythagoras theorem,
We know that Pythagoras theorem is given by
Thus, we will substitute the values and find the value of each leg.
Now, we will find the square root of the obtained value. Thus, we get,
As the sides can not be negative so, we will consider the positive value.
Hence, we can conclude that the length of each leg is 5.
Note: We have to use the concept of Pythagoras theorem in this question so, we have to remember the formula for it that is
Complete step by step answer:
We will first start by considering the given data that two sides are equal to the right triangle and the square of the hypotenuse is given as 50.
We will draw the figure as follows,
So, we will let the two sides of the right triangle that is base and height as
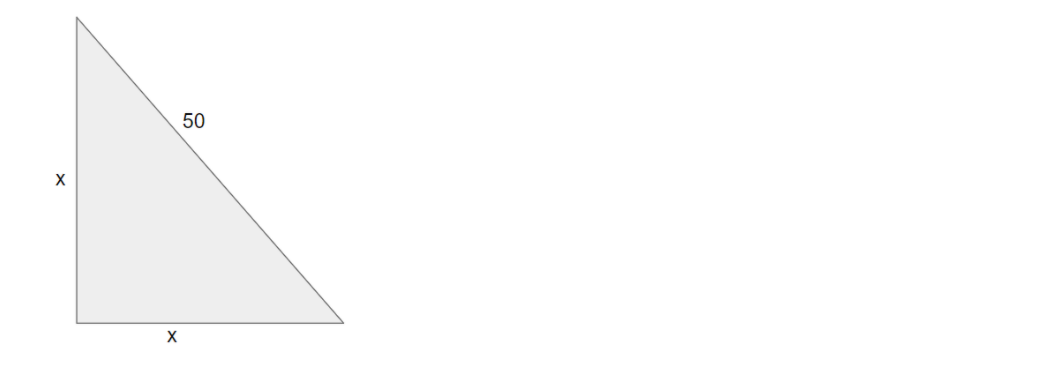
Now, we will apply the Pythagoras theorem,
We know that Pythagoras theorem is given by
Thus, we will substitute the values and find the value of each leg.
Now, we will find the square root of the obtained value. Thus, we get,
As the sides can not be negative so, we will consider the positive value.
Hence, we can conclude that the length of each leg is 5.
Note: We have to use the concept of Pythagoras theorem in this question so, we have to remember the formula for it that is
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE





