
Three of six vertices of a regular hexagon are chosen at random. The probability that triangle with three vertices is equilateral, is equal to
Answer
518.4k+ views
Hint: The formula for selecting r different things out of n different things is given by
The formula for evaluating probability of any event is
P
Equilateral triangle is a type of triangle in which all three sides are of equal length.
Complete Step-by-step answer:
As mentioned in the question, we need to know how many equilateral triangles are there if we join 3 vertices of a regular hexagon.
Another fact about equilateral triangles is that all the angles of an equilateral triangle is equal to
Using this fact, we can calculate that there can be only two triangles that are equilateral when we join three vertices of a regular hexagon.
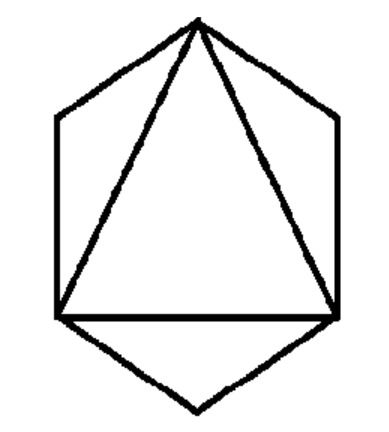
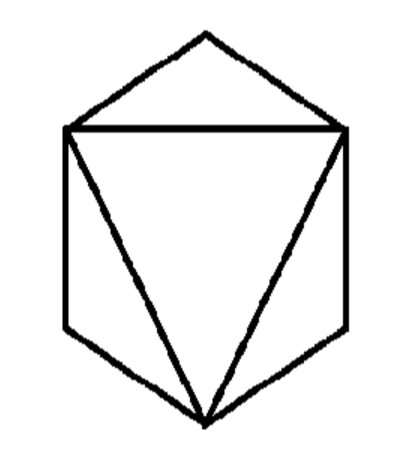
In the figure, the two possible equilateral triangles are marked.
Hence, the total outcomes for the event is given by
Hence, the total outcomes are 20.
Therefore, the probability of the event to take place is
Hence, the probability is
Note: The students can make an error in evaluating the number of equilateral triangles that are formed when three vertices of the regular hexagon are selected at random.
Without knowing the property of equilateral triangles that equilateral triangles are those with all the angles of an equilateral triangle is equal to
The formula for evaluating probability of any event is
P
Equilateral triangle is a type of triangle in which all three sides are of equal length.
Complete Step-by-step answer:
As mentioned in the question, we need to know how many equilateral triangles are there if we join 3 vertices of a regular hexagon.
Another fact about equilateral triangles is that all the angles of an equilateral triangle is equal to
Using this fact, we can calculate that there can be only two triangles that are equilateral when we join three vertices of a regular hexagon.


In the figure, the two possible equilateral triangles are marked.
Hence, the total outcomes for the event is given by
Hence, the total outcomes are 20.
Therefore, the probability of the event to take place is
Hence, the probability is
Note: The students can make an error in evaluating the number of equilateral triangles that are formed when three vertices of the regular hexagon are selected at random.
Without knowing the property of equilateral triangles that equilateral triangles are those with all the angles of an equilateral triangle is equal to
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What was the capital of the king Kharavela of Kalinga class 9 social science CBSE




