Question

Two charges of value $2\mu C$ and $-50\mu C$are placed $80cm$ apart. Calculate the distance of the point from the smaller charge where the intensity is zero.

Answer 1

Hint: Electric field intensity at a given point is given by
$E=\dfrac{Kq}{{{r}^{2}}}$
Where \[q\] be given as charges given at the question and r is the distance between them. This sum of electric field should be zero to get the distance between the smaller charge and the point which intensity is zero.

Complete step-by-step answer:
First of all let us take a look at what electric field intensity is. Each and every particle with an electric charge makes a space around it in which electric force is experienced. This space is called the electric field. If a unit test charge is kept in this electric field, then it will experience the force emitted by the source. The quantity of force felt by charged particles with unit charge if it is placed in the electric field is called Electric field intensity.

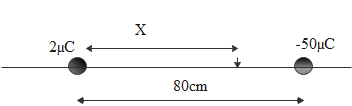
In this question, ${{q}_{1}}$ = $2\mu C$ and \[{{q}_{2}}\] = $-50\mu C$ are the charges and let x be the distance between smallest charge and point at which intensity is zero. It is mentioned that both charges are opposite in charge. In this case the point at which electric field intensity is zero lies outside. Therefore electric field due to smaller charge + electric field due to higher charge will be zero. Substituting the values,
$k\left( \dfrac{2}{{{x}^{2}}} \right)+k\left( \dfrac{-50}{{{\left( 80+x \right)}^{2}}} \right)=0$
Hence
$k\left( \dfrac{2}{{{x}^{2}}} \right)=k\left( \dfrac{50}{{{\left( 80+x \right)}^{2}}} \right)$
k and 2 are common so let’s cancel it
$\left( \dfrac{1}{{{x}^{2}}} \right)=\left( \dfrac{25}{{{\left( 80+x \right)}^{2}}} \right)$
$5x=\left( 80+x \right)$
Therefore
$x=\dfrac{80}{5-1}=20cm$
The distance between the smaller charge and the point at which intensity is zero is 20 cm.

Note: As the electric field is a vector quantity, the direction should be taken into consideration when we calculate the total sum of the electric field intensity. The magnitude of the electric field intensity will not be negative, but the direction can be negative. This should be kept in mind while solving the question.