Question

Two symmetrical double convex lenses A and B have same focal length, but the radii of curvature differ so that ${{R}_{B}}=0.9{{R}_{A}}$. If ${{\mu }_{A}}=1.63$, Find $\mu_B$.

Answer 1

Hint: Relation of radius of curvature, refractive index and focal length of a lens is given by Lens maker’s formula. We will obtain the lens maker formula for symmetric biconvex lenses and then use it to relate focal length of both lenses with their respective radius of curvatures and refractive indices. Then, these equations can be solved to obtain a refractive index of double convex lens B.

Formula used:
Lens maker’s formula, $\dfrac{1}{f}=\left( \mu -1 \right)\left[ \dfrac{1}{{{R}_{1}}}-\dfrac{1}{{{R}_{2}}} \right]$

Complete step-by-step answer:
Relation of radius of curvature, refractive index and focal length of a lens is given by Lens maker’s formula which is given as
$\dfrac{1}{f}=\left( \mu -1 \right)\left[ \dfrac{1}{{{R}_{1}}}-\dfrac{1}{{{R}_{2}}} \right]$

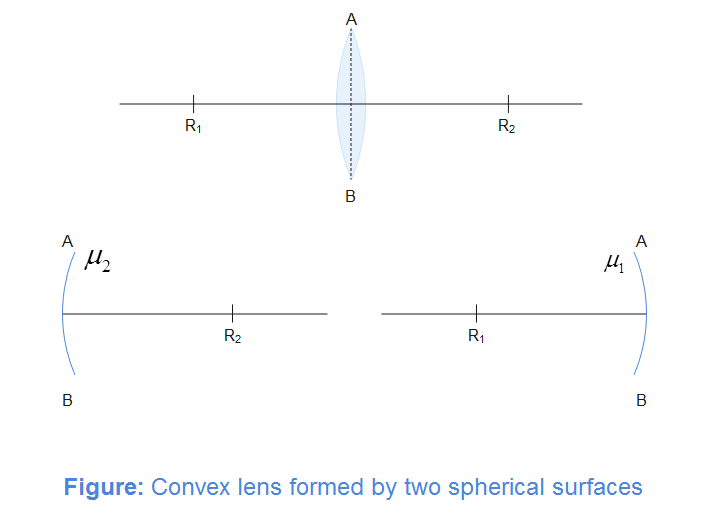
Where ${{R}_{1}}$ and ${{R}_{2}}$ are the radius of left and right spherical curvature respectively and $\mu$ is the refractive index of the material of the lens.
For symmetrical double convex lens, $\left| {{R}_{1}} \right|=\left| {{R}_{2}} \right|=R(say)$
The lens maker formula for symmetric double convex lens becomes,
$\dfrac{1}{f}=(\mu -1)\left[ \dfrac{1}{R}-\left( \dfrac{1}{-R} \right) \right]=\left( \mu -1 \right)\dfrac{2}{R}$
For double convex lens A, we have
$\dfrac{1}{{{f}_{A}}}=\left( {{\mu }_{A}}-1 \right)\dfrac{2}{{{R}_{A}}}$
And for double convex lens B, lens maker formula is
$\dfrac{1}{{{f}_{B}}}=\left( {{\mu }_{B}}-1 \right)\dfrac{2}{{{R}_{B}}}$
We are given that both symmetrical double convex lenses A and B have the same focal length. That is, ${{f}_{A}}={{f}_{B}}$. This implies that, $\dfrac{1}{{{f}_{A}}}=\dfrac{1}{{{f}_{B}}}$. From above equations we can write,
$\left( {{\mu }_{A}}-1 \right)\dfrac{2}{{{R}_{A}}}=\left( {{\mu }_{B}}-1 \right)\dfrac{2}{{{R}_{B}}}$
It is also given that, ${{R}_{B}}=0.9{{R}_{A}}$. For ${{\mu }_{A}}=1.63$, we have
$\left( 1.63-1 \right)\dfrac{2}{{{R}_{A}}}=\left( {{\mu }_{B}}-1 \right)\dfrac{2}{0.9{{R}_{A}}}$
On rearranging the terms and simplifying we get
$0.63\times 0.9={{\mu }_{B}}-1$
This implies that
$\mu_B=1+0.567=1.567$
The refractive index of the convex lens B is 1.567

Note: Lens maker formula relates focal length of a lens with the refractive index of the material used for making lens and radius of curvature of the spherical surface. While substituting values of the quantities in optics, sign convention must be followed. If sign convention is not followed, the result obtained may be wrong.