
What type of quadrilateral has four congruent sides and two obtuse angles?
Answer
440.4k+ views
Hint: Here, we have to determine which quadrilateral has four congruent sides and two obtuse angles. Out of all quadrilaterals, only 2 of them have four congruent sides: Square and rhombus. Now, square has all angles right angles and rhombus has one opposite pair of obtuse angles. Hence, rhombus is our answer.
Complete step-by-step answer:
A quadrilateral is a polygon with four sides.
There are many types of quadrilaterals and each quadrilateral have its own special properties.
In this question, we are supposed to find which quadrilateral has four congruent sides and two obtuse angles.
Let us discuss some quadrilaterals and their properties to find out which one has four congruent sides and two obtuse angles.
Parallelogram: A parallelogram is a quadrilateral with four sides in which two pairs are parallel.
A parallelogram has the following properties.
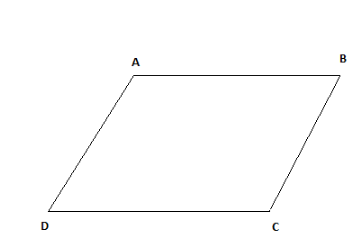
So, parallelogram is not the answer we are looking for.
Square: A square is a quadrilateral with all four sides congruent and all four angles congruent.
A square has the following properties.
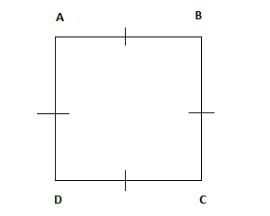
Here, square has all four sides congruent but it does not have obtuse angles. So, square is also not our answer.
Rhombus: A rhombus is a quadrilateral with all four sides congruent. A rhombus differs from a square as the angles of the rhombus do not need to be right angles.
A rhombus has the following properties.
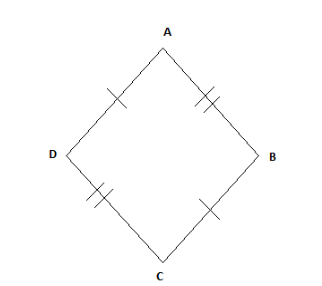
Hence, rhombus is our answer.
So, the correct answer is “rhombus”.
Note: Do not confuse a rhombus with a square. As a rhombus does not have all the properties of a square, a rhombus cannot be a square. But as a square has all the properties of a rhombus, a square can be a rhombus. The area and the perimeter of a rhombus are given by the formula below:
Area of rhombus
Perimeter of rhombus
Complete step-by-step answer:
A quadrilateral is a polygon with four sides.
There are many types of quadrilaterals and each quadrilateral have its own special properties.
In this question, we are supposed to find which quadrilateral has four congruent sides and two obtuse angles.
Let us discuss some quadrilaterals and their properties to find out which one has four congruent sides and two obtuse angles.
Parallelogram: A parallelogram is a quadrilateral with four sides in which two pairs are parallel.
A parallelogram has the following properties.

So, parallelogram is not the answer we are looking for.
Square: A square is a quadrilateral with all four sides congruent and all four angles congruent.
A square has the following properties.

Here, square has all four sides congruent but it does not have obtuse angles. So, square is also not our answer.
Rhombus: A rhombus is a quadrilateral with all four sides congruent. A rhombus differs from a square as the angles of the rhombus do not need to be right angles.
A rhombus has the following properties.

Hence, rhombus is our answer.
So, the correct answer is “rhombus”.
Note: Do not confuse a rhombus with a square. As a rhombus does not have all the properties of a square, a rhombus cannot be a square. But as a square has all the properties of a rhombus, a square can be a rhombus. The area and the perimeter of a rhombus are given by the formula below:
Area of rhombus
Perimeter of rhombus
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




