
What is the value of $\theta $ in the following ray diagram?
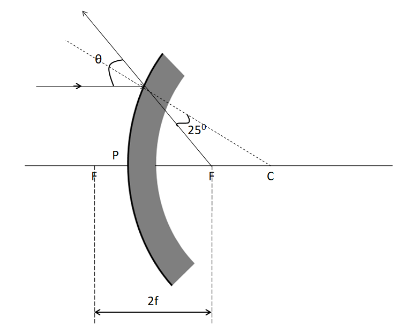
(A) ${25^ \circ }$
(B) ${35^ \circ }$
(C) ${50^ \circ }$
(D) None of these

Answer
459.6k+ views
Hint: To solve this question, we need to use one of the basic properties of reflection which is, the angle of incidence is equal to the angle of refraction. Then, with the application of geometry of the given rays, we can find out the value of the angle.
Complete step by step solution:
The optical instrument given in this question is a mirror. This is because the incident ray after falling on the instrument bounced back. So, it is being reflected back. And since it is polished on the concave side, so its convex side is the reflecting surface. Therefore, it is a convex mirror. Now, considering the diagram given in the question with proper labelling
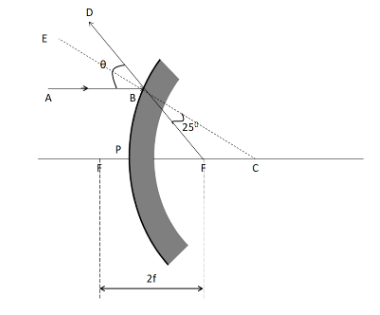
From the above figure, as the dashed line is passing undeflected through the mirror, so it is the normal at the point of incidence B. Now, we know that the angle of incidence is the angle which the incident ray makes with the normal. So the angle of incidence from the above figure is
$\Rightarrow i = \angle ABE$ ……………….(1)
Similarly, the angle of reflection is
$\Rightarrow r = \angle DBE$ ……………..(2)
We know from the properties of reflection that the angle of incidence is equal to the angle of reflection. So, we have
$\Rightarrow i = r$
From (1) and (2) we get
$\Rightarrow \angle ABE = \angle DBE$ ………….(3)
Also, from above figure we can see that
$\Rightarrow \angle ABE + \angle DBE = \theta $ ……………...(4)
Solving (3) and (4) we get
$\Rightarrow \angle ABE = \angle DBE = \dfrac{\theta }{2}$ ……………...(5)
Now, considering the extended lines EC and DF in the above figure, we have
$\Rightarrow \angle DBE = \angle FBC$ (Vertically opposite angles)
According to the question we have
$\Rightarrow \angle FBC = {25^ \circ }$
So we get
$\Rightarrow \angle DBE = {25^ \circ }$ ……………….(6)
From (5) and (6)
$\Rightarrow \dfrac{\theta }{2} = {25^ \circ }$
Multiplying by $2$ both sides, we finally get
$\Rightarrow \theta = {50^ \circ }$
Hence, the correct answer is option C.
Note:
It must be noted that all the information given in the question regarding the distances from the mirror, the type of mirror, are useless. This fact can be appreciated by going through the above solution. We should not try to think from the point of view of the mirror equation. We just needed what type of optical instrument we are given, reflecting or refracting.
Complete step by step solution:
The optical instrument given in this question is a mirror. This is because the incident ray after falling on the instrument bounced back. So, it is being reflected back. And since it is polished on the concave side, so its convex side is the reflecting surface. Therefore, it is a convex mirror. Now, considering the diagram given in the question with proper labelling

From the above figure, as the dashed line is passing undeflected through the mirror, so it is the normal at the point of incidence B. Now, we know that the angle of incidence is the angle which the incident ray makes with the normal. So the angle of incidence from the above figure is
$\Rightarrow i = \angle ABE$ ……………….(1)
Similarly, the angle of reflection is
$\Rightarrow r = \angle DBE$ ……………..(2)
We know from the properties of reflection that the angle of incidence is equal to the angle of reflection. So, we have
$\Rightarrow i = r$
From (1) and (2) we get
$\Rightarrow \angle ABE = \angle DBE$ ………….(3)
Also, from above figure we can see that
$\Rightarrow \angle ABE + \angle DBE = \theta $ ……………...(4)
Solving (3) and (4) we get
$\Rightarrow \angle ABE = \angle DBE = \dfrac{\theta }{2}$ ……………...(5)
Now, considering the extended lines EC and DF in the above figure, we have
$\Rightarrow \angle DBE = \angle FBC$ (Vertically opposite angles)
According to the question we have
$\Rightarrow \angle FBC = {25^ \circ }$
So we get
$\Rightarrow \angle DBE = {25^ \circ }$ ……………….(6)
From (5) and (6)
$\Rightarrow \dfrac{\theta }{2} = {25^ \circ }$
Multiplying by $2$ both sides, we finally get
$\Rightarrow \theta = {50^ \circ }$
Hence, the correct answer is option C.
Note:
It must be noted that all the information given in the question regarding the distances from the mirror, the type of mirror, are useless. This fact can be appreciated by going through the above solution. We should not try to think from the point of view of the mirror equation. We just needed what type of optical instrument we are given, reflecting or refracting.
Recently Updated Pages
Using the following information to help you answer class 12 chemistry CBSE

Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

What is a transformer Explain the principle construction class 12 physics CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?




