
Verify LMVT (Lagrange’s mean value theorem) for the function
Answer
521.4k+ views
Hint: Use the concept that log x is both differentiable as well as continuous in the interval [1, e], so according to Lagrange’s mean value theorem there exists a point c such that
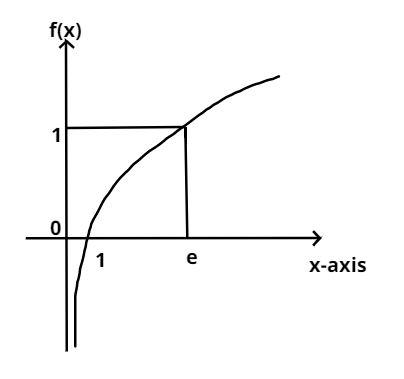
Complete Step-by-Step solution:
Lagrange’s mean value theorem (LMVT) states that if a function f(x) is continuous on a closed interval [a, b] and differentiable on the open interval (a, b), then there is at least one point x = c on this interval, such that
Now given function is
The graph of log x is shown above which is true for
Now as we know that log x is differentiable as well as continuous in the interval [1, e] so there exists a point x = c such that
Now differentiate f(x) we have,
Now in place of x substitute (c) we have,
Now from equation (1) we have,
Now as we know the value of log e is 1 and the value of log 1 is zero so we have,
Hence c is belongs between (1, e)
Hence LMVT is verified.
Note: If a function is continuous at some points then it may or may not be differentiable at those points, but if a function is differentiable at some points that we can say with certainty that it has to be continuous. That is differentiability is a sure condition for continuity however converse is not true. These tricks help commenting upon continuity and differentiability while solving problems of such kind.
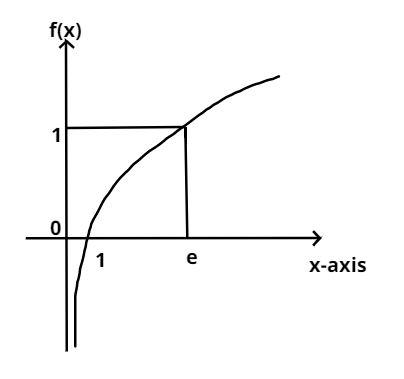
Complete Step-by-Step solution:
Lagrange’s mean value theorem (LMVT) states that if a function f(x) is continuous on a closed interval [a, b] and differentiable on the open interval (a, b), then there is at least one point x = c on this interval, such that
Now given function is
The graph of log x is shown above which is true for
Now as we know that log x is differentiable as well as continuous in the interval [1, e] so there exists a point x = c such that
Now differentiate f(x) we have,
Now in place of x substitute (c) we have,
Now from equation (1) we have,
Now as we know the value of log e is 1 and the value of log 1 is zero so we have,
Hence c is belongs between (1, e)
Hence LMVT is verified.
Note: If a function is continuous at some points then it may or may not be differentiable at those points, but if a function is differentiable at some points that we can say with certainty that it has to be continuous. That is differentiability is a sure condition for continuity however converse is not true. These tricks help commenting upon continuity and differentiability while solving problems of such kind.
Latest Vedantu courses for you
Grade 6 | CBSE | SCHOOL | English
Vedantu 6 Pro Course (2025-26)
School Full course for CBSE students
₹42,500 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility

Difference between mass and weight class 10 physics CBSE

SI unit of electrical energy is A Joule B Kilowatt class 10 physics CBSE




