
Write the formula for calculating wattless current.
Answer
432.3k+ views
Hint: In order to answer the above question, we will refresh our knowledge about a simple LCR circuit which is the most basic circuit. We will be deriving the equation to find out the power consumed by such a circuit. Then we will derive the equation for wattless current.
Complete step by step solution:
First of all we will have a look at an LCR circuit:
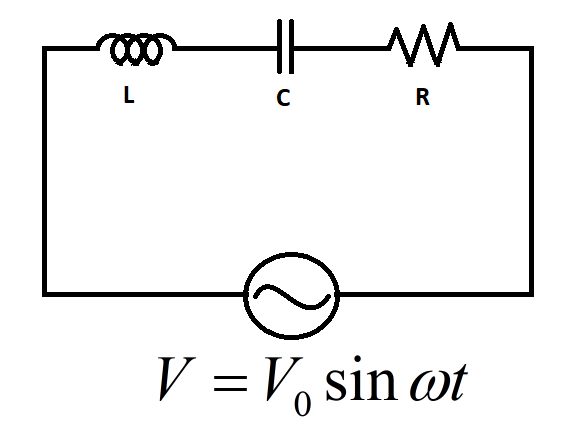
As we can see from the above figure, we have an LCR circuit which comprises an inductor
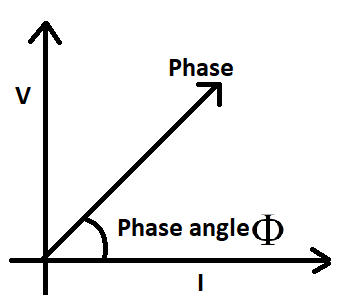
To understand the phase difference properly, we will draw a phase diagram
Now, the work done by the AC source during time interval of
The total work done in one complete cycle is given by taking integral of the above equation over
Now, to calculate the average power delivered by the source, we divide the above equation by time period
Simplifying a little
Now, we know that
The above formula is the formula for power dissipated in an AC circuit.
Now, we want to find the formula for wattless current. When the power dissipated or consumed is zero, the current is said to be wattless current or an idle current.
From the above derived formula of power, we can say that the power can only be zero if the value of
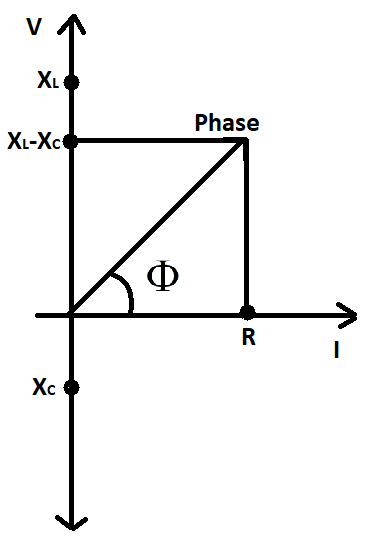
Now as we know that the current in the circuit is given by
Note:
It is very important to note that the power consumed can be made zero only when a resistance is connected with either a capacitor or an inductor and not with both of them together. The phase angle will become
Complete step by step solution:
First of all we will have a look at an LCR circuit:

As we can see from the above figure, we have an LCR circuit which comprises an inductor
To understand the phase difference properly, we will draw a phase diagram

Now, the work done by the AC source during time interval of
The total work done in one complete cycle is given by taking integral of the above equation over
Now, to calculate the average power delivered by the source, we divide the above equation by time period
Simplifying a little
Now, we know that
The above formula is the formula for power dissipated in an AC circuit.
Now, we want to find the formula for wattless current. When the power dissipated or consumed is zero, the current is said to be wattless current or an idle current.
From the above derived formula of power, we can say that the power can only be zero if the value of

Now as we know that the current in the circuit is given by
Note:
It is very important to note that the power consumed can be made zero only when a resistance is connected with either a capacitor or an inductor and not with both of them together. The phase angle will become
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Draw a diagram of a flower and name the parts class 12 biology ICSE




