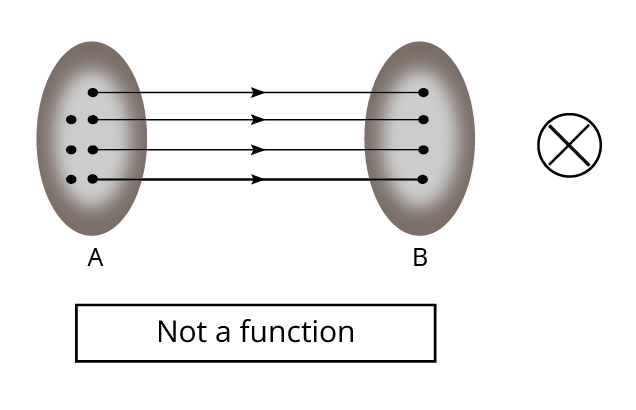
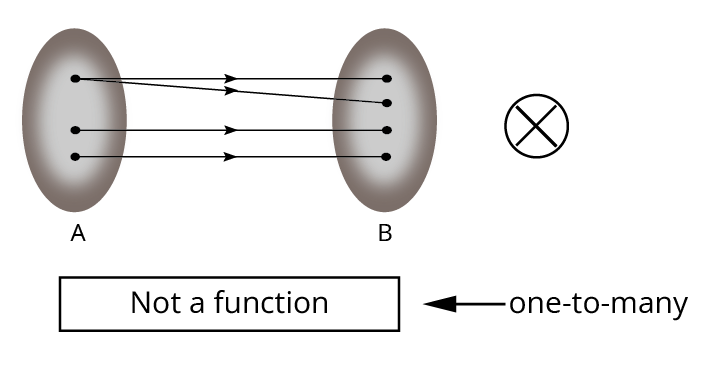
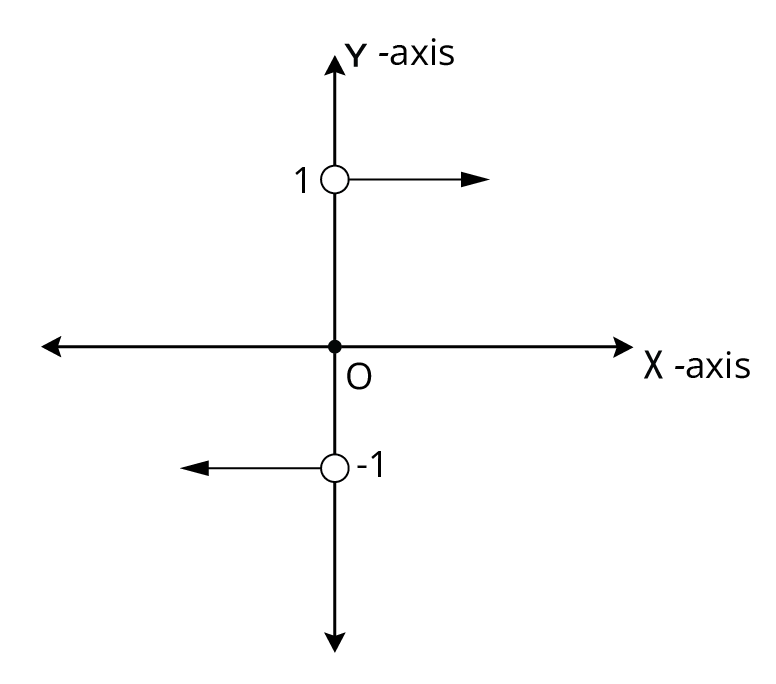
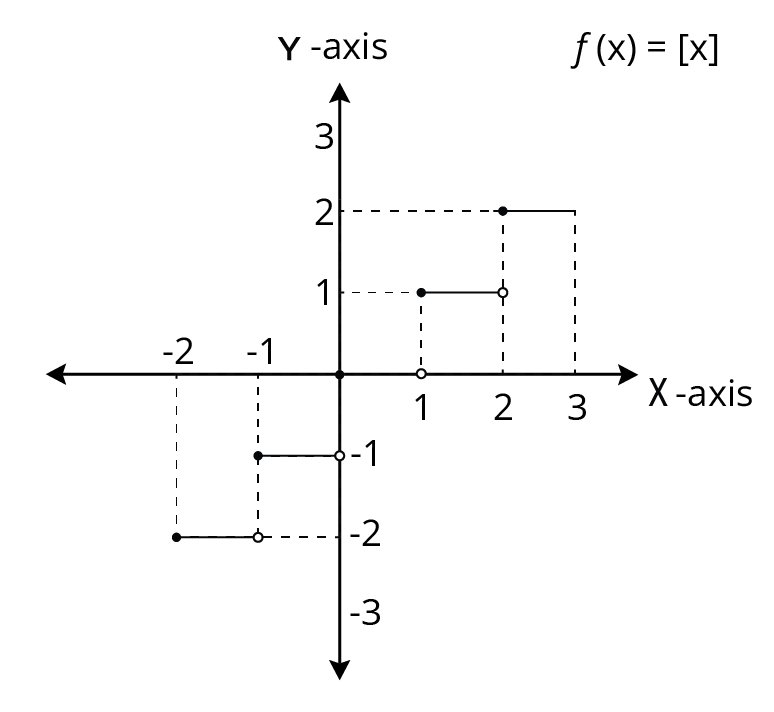
Class 11 Maths Chapter 2 Relations and Functions Notes FREE PDF Download
FAQs on Relations and Functions Class 11 Notes: CBSE Maths Chapter 2
1. What topics are covered in the Class 11 Relations and Functions notes?
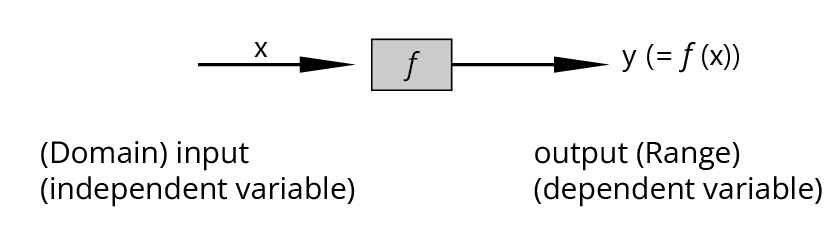
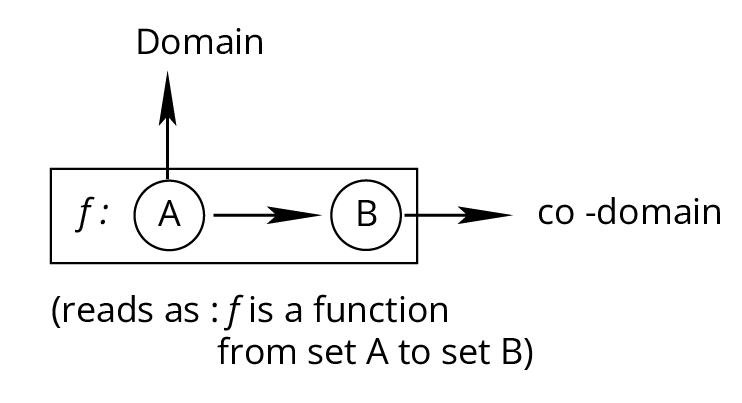
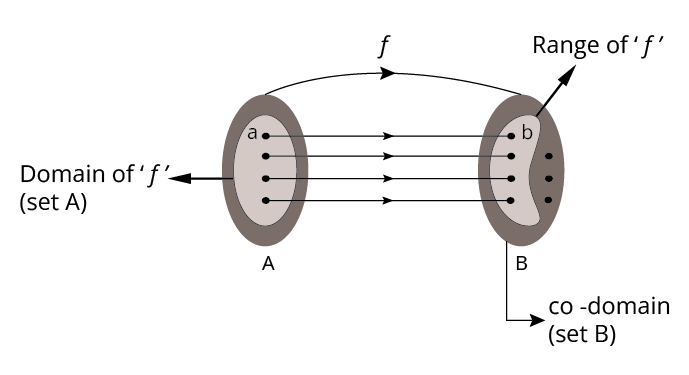
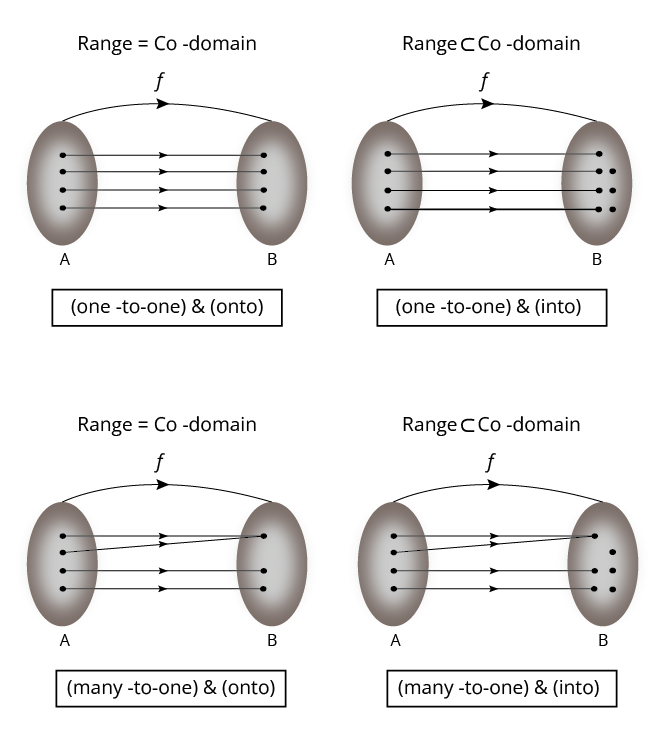
The Class 11 Relations and Functions notes cover fundamental concepts, including types of relations, functions, domain, range, function composition, and inverse functions.
2. How can I download the Class 11 Relations and Functions notes in PDF format?
The Class 11 Relations and Functions notes can be downloaded as a PDF from Vedantu that offering free or paid study resources.
3. What is included in the Class 11 Relations and Functions notes?
The notes typically include detailed explanations of concepts, solved examples, practice questions, and key formulas related to relations and functions.
4. How do Class 11 Relations and Functions notes help in studying?
These notes provide structured and comprehensive coverage of the chapter, aiding in better understanding, efficient revision, and effective exam preparation.
5. Are there any free resources for Class 11 Relations and Functions notes?
Yes, many educational websites offer free PDF downloads of Class 11 Relations and Functions notes for easy access and study.
6. Can the Class 11 Relations and Functions notes be used for self-study?
The notes are designed to support self-study by providing clear explanations, examples, and exercises to help students learn independently.
7. How frequently should I review the Class 11 Relations and Functions notes?
Regular review is recommended to reinforce understanding and keep the concepts fresh. Frequent revision helps improve retention and exam readiness.
8. Are solved examples included in the Class 11 Relations and Functions notes?
Yes, the notes often include solved examples to demonstrate how to approach and solve various types of problems related to relations and functions.
9. How can I use Class 11 Relations and Functions notes to prepare for exams?
Use the notes to review key concepts, practice problems, and understand the application of formulas. They are valuable for structured study and focused exam preparation.