Trigonometric Functions Class 11 Notes: CBSE Maths Chapter 3

1. The Meaning of Trigonometry

${{\text{Tri }}}{{\text{ Gon }}}{{\text{ Metron }}}$

$\downarrow\quad\;\;\;\;\downarrow\quad\;\;\;\;\downarrow$

$3\quad{{\text{ sides }}}{{\text{ Measure }}}$

As a result, this area of mathematics was established in the ancient past to measure a triangle's three sides, three angles, and six components. Time-trigonometric functions are utilised in a variety of ways nowadays. The sine and cosine of an angle in a right-angled triangle are the two fundamental functions, and there are four more derivative functions.

2. Basic Trigonometric Identities

(a) ${\sin ^2}\theta  + {\cos ^2}\theta  = 1: - 1 \leqslant \sin \theta  \leqslant 1; - 1 \leqslant \cos \theta  \leqslant 1\forall \theta  \in {\text{R}}$

(b) ${\sec ^2}\theta  - {\tan ^2}\theta  = 1:|\sec \theta | \geqslant 1\forall \theta  \in {\text{R}}$

(c) ${\operatorname{cosec} ^2}\theta  - {\cot ^2}\theta  = 1:|\operatorname{cosec} \theta | \geqslant 1\forall \theta  \in {\text{R}}$

Trigonometric Ratios of Standard Angles:

The relation between these trigonometric identities with the sides of the triangles can be given as follows:

• Sine θ $=$ Opposite/Hypotenuse

• Cos θ  $=$ Adjacent/Hypotenuse

• Tan θ  $=$ Opposite/Adjacent

• Cot θ $=$ Adjacent/Opposite

• Cosec θ  = Hypotenuse/Opposite

• Sec θ  = Hypotenuse/Adjacent

The following are the signs of trigonometric ratios in different quadrants:

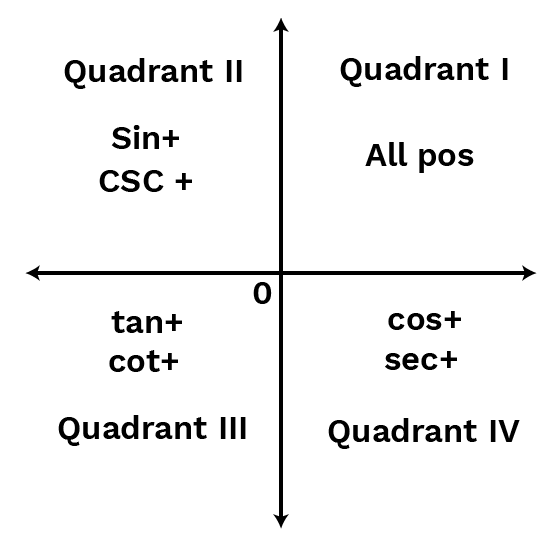
3. Trigonometric Ratios of Allied Angles

We might calculate the trigonometric ratios of angles of any value using the trigonometric ratio of allied angles.

1. Sin(–θ)=–Sinθ

2. Cos(–θ)=Cosθ

3. Tan(–θ)=–Tanθ

4. Sin(90o–θ)=Cosθ

5. Cos(90o–θ)=Sinθ

6. Tan(90o–θ)=Cotθ

7. Sin(180o–θ)=Sinθ

8. Cos(180o–θ)=–Cosθ

9. Tan(180o–θ)=–Tanθ

10. Sin(270o–θ)=–Cosθ

11. Cos(270o–θ)=–Sinθ

12. Tan(270o–θ)=Cotθ

13. Sin(90o+θ)=Cosθ

14. Cos(90o+θ)=–Sinθ

15. Tan(90o+θ)=–Cotθ

16. Sin(180o+θ)=–Sinθ

17. Cos(180o+θ)=–Cosθ

18. Tan(180o+θ)=Tanθ

19. Sin(270o+θ)=–Cosθ

20. Cos(270o+θ)=Sinθ

21. Tan(270o+θ)=–Cotθ

4. Trigonometric Functions of Sum or Difference of Two Angles

(a) $\sin ({\text{A}} + {\text{B}}) = \sin {\text{A}}\cos {\text{B}} + \cos {\text{A}}\sin {\text{B}}$

(b) $\sin ({\text{A}} - {\text{B}}) = \sin {\text{A}}\cos {\text{B}} - \cos {\text{A}}\sin {\text{B}}$

(c) $\cos (A + B) = \cos A\cos B - \sin A\sin B$

(d) $\cos ({\text{A}} - {\text{B}}) = \cos {\text{A}}\cos {\text{B}} + \sin {\text{A}}\sin {\text{B}}$

(e) $\tan (A + B) = \dfrac{{\tan A + \tan B}}{{1 - \tan A\tan B}}$

(f) $\tan ({\text{A}} - {\text{B}}) = \dfrac{{\tan {\text{A}} - \tan {\text{B}}}}{{1 + \tan {\text{A}}\tan {\text{B}}}}$

(g) $\cot (A + B) = \dfrac{{\cot A\cot B - 1}}{{\cot B + \cot A}}$

(f) $\cot ({\text{A}} - {\text{B}}) = \dfrac{{\cot {\text{A}}\cot {\text{B}} + 1}}{{\cot {\text{B}} - \cot {\text{A}}}}$

(h) ${\sin ^2}\;{\text{A}} - {\sin ^2}\;{\text{B}} = {\cos ^2}\;{\text{B}} - {\cos ^2}\;{\text{A}} = \sin ({\text{A}} + {\text{B}}) \cdot \sin ({\text{A}} - {\text{B}})$

(i) ${\cos ^2}\;{\text{A}} - {\sin ^2}\;{\text{B}} = {\cos ^2}\;{\text{B}} - {\sin ^2}\;{\text{A}} = \cos ({\text{A}} + {\text{B}}) \cdot \cos ({\text{A}} - {\text{B}})$

(j) $\tan (A + B + C) = \dfrac{{\tan A + \tan B + \tan C - \tan A\tan B\tan C}}{{1 - \tan A\tan B - \tan B\tan C - \tan C\tan A}}$

5. Multiple Angles and Half Angles

(a) $\sin 2\;{\text{A}} = 2\sin {\text{A}}\cos {\text{A}};\quad \sin \theta  = 2\sin \dfrac{\theta }{2}\cos \dfrac{\theta }{2}$

(b) $\cos 2\;{\text{A}} = {\cos ^2}\;{\text{A}} - {\sin ^2}\;{\text{A}} = 2{\cos ^2}\;{\text{A}} - 1 = 1 - 2{\sin ^2}\;{\text{A}}$ 

$2{\cos ^2}\dfrac{\theta }{2} = 1 + \cos \theta ,2{\sin ^2}\dfrac{\theta }{2} = 1 - \cos \theta $

(c) $\tan 2\;{\text{A}} = \dfrac{{2\tan {\text{A}}}}{{1 - {{\tan }^2}\;{\text{A}}}};\tan \theta  = \dfrac{{2\tan \dfrac{\theta }{2}}}{{1 - {{\tan }^2}\dfrac{\theta }{2}}}$

(d) $\sin 2\;{\text{A}} = \dfrac{{2\tan {\text{A}}}}{{1 - {{\tan }^2}\;{\text{A}}}};\cos 2\;{\text{A}} = \dfrac{{1 - {{\tan }^2}\;{\text{A}}}}{{1 + {{\tan }^2}\;{\text{A}}}}$

(e) $\sin 3\;{\text{A}} = 3\sin {\text{A}} - 4{\sin ^3}\;{\text{A}}$

(f) $\cos 3\;{\text{A}} = 4{\cos ^3}\;{\text{A}} - 3\cos {\text{A}}$

(g) $\tan 3\;{\text{A}} = \dfrac{{3\tan {\text{A}} - {{\tan }^3}\;{\text{A}}}}{{1 - 3{{\tan }^2}\;{\text{A}}}}$

6. Transformation of Products into Sum or Difference of Sines & Cosines

(a) $2\sin {\text{A}}\cos {\text{B}} = \sin ({\text{A}} + {\text{B}}) + \sin ({\text{A}} - {\text{B}})$

(b) $2\cos {\text{A}}\sin {\text{B}} = \sin ({\text{A}} + {\text{B}}) - \sin ({\text{A}} - {\text{B}})$

(c) $2\cos {\text{A}}\cos {\text{B}} = \cos ({\text{A}} + {\text{B}}) + \cos ({\text{A}} - {\text{B}})$

(d) $2\sin {\text{A}}\sin {\text{B}} = \cos ({\text{A}} - {\text{B}}) - \cos ({\text{A}} + {\text{B}})$

7. Factorisation of the Sum or Difference of Two Sines or Cosines

(a) $\sin {\text{C}} + \sin {\text{D}} = 2\sin \dfrac{{{\text{C}} + {\text{D}}}}{2}\cos \dfrac{{{\text{C}} - {\text{D}}}}{2}$

(b) $\sin {\text{C}} - \sin {\text{D}} = 2\cos \dfrac{{{\text{C}} + {\text{D}}}}{2}\sin \dfrac{{{\text{C}} - {\text{D}}}}{2}$

(c) $\cos C + \cos D = 2\cos \dfrac{{C + D}}{2}\cos \dfrac{{C - D}}{2}$

(d) $\cos {\text{C}} - \cos {\text{D}} =  - 2\sin \dfrac{{{\text{C}} + {\text{D}}}}{2}\sin \dfrac{{{\text{C}} - {\text{D}}}}{2}$

8. Important Trigonometric Ratios

(a) $\sin {\text{n}}\pi  = 0;\cos {\text{n}}\pi  = {( - 1)^{\text{n}}};\tan {\text{n}}\pi  = 0$ where ${\text{n}} \in {\text{Z}}$

(b) $\sin {15^\circ }$ or $\sin \dfrac{\pi }{{12}} = \dfrac{{\sqrt 3  - 1}}{{2\sqrt 2 }} = \cos {75^\circ }$ or $\cos \dfrac{{5\pi }}{{12}}$; 

$\cos {15^\circ }$ or $\cos \dfrac{\pi }{{12}} = \dfrac{{\sqrt 3  + 1}}{{2\sqrt 2 }} = \sin {75^\circ }$ or $\sin \dfrac{{5\pi }}{{12}}$ 

$\tan {15^\circ } = \dfrac{{\sqrt 3  - 1}}{{\sqrt 3  + 1}} = 2 - \sqrt 3  = \cot {75^\circ }$ 

$\tan {75^\circ } = \dfrac{{\sqrt 3  + 1}}{{\sqrt 3  - 1}} = 2 + \sqrt 3  = \cot {15^\circ }$

(c) $\sin \dfrac{\pi }{{10}}$ or $\sin {18^\circ } = \dfrac{{\sqrt 5  - 1}}{4}$ & $\cos {36^\circ }$ or $\cos \dfrac{\pi }{5} = \dfrac{{\sqrt 5  + 1}}{4}$

9. Conditional Identities

If ${\text{A}} + {\text{B}} + {\text{C}} = \pi $ then :

(i) $\sin 2\;{\text{A}} + \sin 2\;{\text{B}} + \sin 2{\text{C}} = 4\sin {\text{A}}\sin {\text{B}}\sin {\text{C}}$

(ii) $\sin {\text{A}} + \sin {\text{B}} + \sin {\text{C}} = 4\cos \dfrac{{\text{A}}}{2}\cos \dfrac{{\text{B}}}{2}\cos \dfrac{{\text{C}}}{2}$

(iii) $\cos 2\;{\text{A}} + \cos 2\;{\text{B}} + \cos 2{\text{C}} =  - 1 - 4\cos {\text{A}}\cos {\text{B}}\cos {\text{C}}$

(iv) $\cos {\text{A}} + \cos {\text{B}} + \cos {\text{C}} = 1 + 4\sin \dfrac{{\text{A}}}{2}\sin \dfrac{{\text{B}}}{2}\sin \dfrac{{\text{C}}}{2}$

(v) $\tan {\text{A}} + \tan {\text{B}} + \tan {\text{C}} = \tan {\text{A}}\tan {\text{B}}\tan {\text{C}}$

(vi) $\tan \dfrac{{\text{A}}}{2}\tan \dfrac{{\text{B}}}{2} + \tan \dfrac{{\text{B}}}{2}\tan \dfrac{{\text{C}}}{2} + \tan \dfrac{{\text{C}}}{2}\tan \dfrac{{\text{A}}}{2} = 1$

(vii) $\cot \dfrac{{\text{A}}}{2} + \cot \dfrac{{\text{B}}}{2} + \cot \dfrac{{\text{C}}}{2} = \cot \dfrac{{\text{A}}}{2} \cdot \cot \dfrac{{\text{B}}}{2} \cdot \cot \dfrac{{\text{C}}}{2}$

(viii) $\cot A\cot B + \cot B\cot C + \cot C\cot A = 1$

10. Range of Trigonometric Expression

${{\text{E}} = {\text{a}}\sin \theta  + {\text{b}}\cos \theta }$

${{\text{E}} = \sqrt {{{\text{a}}^2} + {{\text{b}}^2}} \sin (\theta  + \alpha ),\left( {{\text{ where }}\tan \alpha  = \dfrac{{\text{b}}}{{\text{a}}}} \right)}$ 

${{\text{E}} = \sqrt {{{\text{a}}^2} + {{\text{b}}^2}} \cos (\theta  - \beta ),\left( {{\text{ where }}\tan \beta  = \dfrac{{\text{a}}}{{\text{b}}}} \right)}$

Hence for any real value of $\theta , - \sqrt {{a^2} + {b^2}}  \leqslant E \leqslant \sqrt {{a^2} + {b^2}} $

The trigonometric functions are very important for studying triangles, light, sound or waves. The values of these trigonometric functions in different domains and ranges can be used from the following table:

Trigonometric Functions in Different Domains and Ranges

11. Sine and Cosine Series

(a) $\quad \sin \alpha  + \sin (\alpha  + \beta ) + \sin (\alpha  + 2\beta ) +  \ldots . + \sin (\alpha  + \overline {n - 1} \beta )$

$ = \dfrac{{\sin \dfrac{{{\text{n}}\beta }}{2}}}{{\sin \dfrac{\beta }{2}}}\sin \left( {\alpha  + \dfrac{{{\text{n}} - 1}}{2}\beta } \right)$

(b) 

${\cos \alpha  + \cos (\alpha  + \beta ) + \cos (\alpha  + 2\beta ) +  \ldots  + \cos (\alpha  + \overline {n - 1} \beta )}$

${ = \dfrac{{\sin \dfrac{{n\beta }}{2}}}{{\sin \dfrac{\beta }{2}}}\cos \left( {\alpha  + \dfrac{{n - 1}}{2}\beta } \right)}$ 

12. Graphs of Trigonometric Functions 

(a). ${y = \sin x,}$

${x \in R;y \in [ - 1,1]}$ 

(b). $y = \cos x$

$x \in R;y \in [ - 1,1]$ 

(c) ${y = \tan x}$

${x \in R - \left\{ {(2n + 1)\dfrac{\pi }{2};n \in Z} \right\};y \in R}$ 

(d) ${y = \cot x}$

${x \in R - \{ n\pi ;n \in z\} ;y \in R}$ 

(e) ${y = \operatorname{cosec} x}$ 

${x \in R - \{ n\pi ;n \in Z\} ;y \in ( - \infty , - 1] \cup [1,\infty )}$

(f) $y = \sec x\quad $

$x \in R - \left\{ {(2n + 1)\dfrac{\pi }{2};n \in Z} \right\};y \in ( - \infty , - 1] \cup [1,\infty )$ 

Trigonometric Equations

13. Trigonometric Equations

Trigonometric equations are equations using trigonometric functions with unknown angles. 

e.g.,  $\cos \theta  = 0,{\cos ^2}\theta  - 4\cos \theta  = 1$.

The value of the unknown angle that satisfies a trigonometric equation is called a solution.

e.g., $\quad \sin \theta  = \dfrac{1}{{\sqrt 2 }} \Rightarrow \theta  = \dfrac{\pi }{4}$ or $\theta  = \dfrac{\pi }{4},\dfrac{{3\pi }}{4},\dfrac{{9\pi }}{4},\dfrac{{11\pi }}{4}, \ldots $

As a result, the trigonometric equation can have an unlimited number of solutions and is categorised as follows:

(i). Principal Solution

As we know, the values of $\sin x$ and $\cos x$ will get repeated after an interval of $2 \pi$. In the same way, the values of $\tan x$ will get repeated after an interval of $\pi$. 

So, if the equation has a variable $0 \leq \mathrm{x}<2 \pi$, then the solutions will be termed as principal solutions. 

Example:

Find the principal solutions of the equation $\sin x=\dfrac{\sqrt{3}}{2}$.

Solution: We know that, $\sin \dfrac{\pi}{3}=\dfrac{\sqrt{3}}{2}$

Also, $\sin \dfrac{2 \pi}{3}=\sin \left(\pi-\dfrac{\pi}{3}\right)$

Now, we know that $\sin (\pi-x)=\sin x$. 

Hence, $\sin \dfrac{2 \pi}{3}=\sin \dfrac{\pi}{3}=\dfrac{\sqrt{3}}{2}$

Therefore, the principal solutions of $\sin x=\dfrac{\sqrt{3}}{2}$ are $\mathrm{x}=\dfrac{\pi}{3}$ and $\dfrac{2 \pi}{3}$.

(ii). General solution

A general solution is one that involves the integer 'n' and yields all trigonometric equation solutions. Also, the character ' $\mathrm{Z}$ ' is used to denote the set of integers.

Find the solution of $\sin x=-\dfrac{\sqrt{3}}{2}$.

Solution: We know that $\sin \dfrac{\pi}{3}=\dfrac{\sqrt{3}}{2}$. Therefore, $\sin x=-\dfrac{\sqrt{3}}{2}=-\sin \dfrac{\pi}{3}$

Using the unit circle properties, we get $\sin x=-\sin \dfrac{\pi}{3}=\sin \left(\pi+\dfrac{\pi}{3}\right)=\sin \dfrac{4 \pi}{3}$ Hence, $\sin x=\sin \dfrac{4 \pi}{3}$

Since, we know that for any real numbers $x$ and $y, \sin x=\sin y$ implies $x=n \pi+(-1)^{n} y$, where $n \in Z$.

So, we get, $x=n \pi+(-1)^{\mathrm{n}}\left(\dfrac{4 \pi}{3}\right)$

14. Results

1. $\quad \sin \theta  = 0 \Leftrightarrow \theta  = \operatorname{n} \pi $

2. $\cos \theta  = 0 \Leftrightarrow \theta (2{\text{n}} + 1)\dfrac{\pi }{2}$

3. $\tan \theta  = 0 \Leftrightarrow \theta  = {\text{n}}\pi $

4. $\sin \theta  = \sin \alpha  \Leftrightarrow \theta  = {\text{n}}\pi  + {( - 1)^{\text{n}}}\alpha $, where $\alpha  \in \left[ { - \dfrac{\pi }{2},\dfrac{\pi }{2}} \right]$

5. $\cos \theta  = \cos \alpha  \Leftrightarrow \theta  = 2{\text{n}}\pi  \pm \alpha $, where $\alpha  \in [0,\pi ]$

6. $\tan \theta  = \tan \alpha  \Leftrightarrow \theta  = {\text{n}}\pi  + \alpha $, where $\alpha  \in \left( { - \dfrac{\pi }{2},\dfrac{\pi }{2}} \right)$

7. ${\sin ^2}\theta  = {\sin ^2}\alpha  \Leftrightarrow \theta  = {\text{n}}\pi  \pm \alpha $.

8. ${\cos ^2}\theta  = {\cos ^2}\alpha  \Leftrightarrow \theta  = $ n $\pi  \pm \alpha $.

9. ${\tan ^2}\theta  = {\tan ^2}\alpha  \Leftrightarrow \theta  = {\text{n}}\pi  \pm \alpha $.

10. $\sin \theta  = 1 \Leftrightarrow \theta  = (4{\text{n}} + 1)\dfrac{\pi }{2}$

11. $\cos \theta  = 1 \Leftrightarrow \theta  = 2{\text{n}}\pi $

12. $\cos \theta  =  - 1 \Leftrightarrow \theta  = (2{\text{n}} + 1)\pi $.

13. $\sin \theta  = \sin \alpha $ and $\cos \theta  = \cos \alpha  \Leftrightarrow \theta  = 2{\text{n}}\pi  + \alpha $

Steps to Solve Trigonometric Functions:

The following are the stages of solving trigonometric equations:

Step 1: Decompose the trigonometric equation into a single trigonometric ratio, preferably the sine or cos function.

Step 2: Factor the trigonometric polynomial given in terms of the ratio.

Step 3: Write down the general solution after solving for each factor.

Note:

1. Unless otherwise stated, is treated as an integer throughout this chapter.

2. Unless the answer is required in a specific interval or range, the general solution should be supplied.

3. The angle's main value is regarded as $\alpha $. (The main value is the angle with the least numerical value.)

Trigonometric Functions Class 11 Notes Maths - Basic Subjective Questions

Section–A (1 Mark Questions)

1. If $tan\Theta =\frac{-4}{3}$ , then find $sin\Theta$.

Ans. Since, $\tan \theta=-\frac{4}{3}$ is negative, $\theta$ lies either in second quadrant or in fourth quadrant.

$$ \begin{aligned} & \because 1+\cot ^2 \theta=\operatorname{cosec}^2 \theta \\ & \Rightarrow 1+\frac{1}{\left(-\frac{4}{3}\right)^2}=\frac{1}{\sin ^2 \theta} \\ & \Rightarrow \sin ^2 \theta=\left(\frac{4}{5}\right)^2 \end{aligned} $$

Thus $\sin \theta=\frac{4}{5}$ if $\theta$ lies in the second quadrant or $\sin \theta=-\frac{4}{5}$, if $\theta$ lies in the fourth quadrant.

2. Find the greatest value of sin x cos x.

Ans. $\sin x \cos x=\frac{1}{2}(2 \sin x \cos x)=\frac{1}{2} \sin 2 x$ Greatest value of $\sin 2 x=1$

$\therefore$ Greatest value of $\dfrac{1}{2} \sin 2 x=\dfrac{1}{2} \times 1=\dfrac{1}{2}$

3. Find the degree measure of angle $\left ( \frac{\pi }{8} \right )^{c}$  radian.

Ans. We know that, $\pi$ radians $=180^{\circ}$

$$ \begin{aligned} & \Rightarrow \frac{\pi}{8} \text { radians }=22.5^{\circ} \\ & =22^{\circ}+0.5^{\circ}=22^{\circ}+30^{\prime}=22^{\circ} 30^{\prime} \end{aligned} $$

4. Evaluate: $sin \left ( \frac{-15\pi }{4} \right )$ .

Ans.

$$ \begin{aligned} & \text { 4. } \sin \left(\frac{-15 \pi}{4}\right)=\sin \left(\frac{-16 \pi+\pi}{4}\right) \\ & =\sin \left(-4 \pi+\frac{\pi}{4}\right) \\ & =\sin \left(\frac{\pi}{4}\right)=\frac{1}{\sqrt{2}} \end{aligned} $$

5. The minute hand of a watch is 1.5 cm long. How far does it tip move in 40 minutes? (Use $\pi$ = 3.14).

Ans. Angle rotated by minute hand in 60 minutes = $2\pi$    radians 

Therefore, angle rotated by minute hand in 40 minutes = $\frac{40}{60}\times2\pi =\frac{4\pi }{3}$  radians.

Hence, the required distance travelled is given by

$l=r\Theta =1.5\times\frac{4\pi }{3}cm=2\pi\;cm$

$=2\times3.14\;cm=6.28\;cm$

Section–B (2 Marks Questions)

6. Prove that cot2x cotx - cot3x cot2x - cot3x cotx = 1

Ans. We have, cot 3x=cot (2x+x)

$\Rightarrow cot\;3x=\dfrac{cot\;2x\;cot\;x -1}{cot\;2x+cot\;x}$

$\Rightarrow$ Cot 3x cot 2x + cot 3x cot x = cot 2x cot x-1

$\Rightarrow$ cot 2x cot x - cot 3x cot 2x - cot 3x xot x=1

7. Evaluate: $\frac{1-\tan^2{15^\circ}}{1+\tan^2{15^\circ}}$ 

Ans. Given that: $\frac{1-\tan^2{15^\circ}}{1+\tan^2{15^\circ}}$

Let $\Theta =15^{\circ}$

$\therefore 2\Theta =30^{\circ}$

We know that, $\therefore cos\;2\Theta =\frac{1-\tan^2{15^\circ}}{1+\tan^2{15^\circ}}$

$\Rightarrow  cos\;30 =\frac{1-\tan^2{15^\circ}}{1+\tan^2{15^\circ}}$

$\frac{1-\tan^2{15^\circ}}{1+\tan^2{15^\circ}}=\frac{\sqrt{3}}{3}$

8. If for real value of x, $cos\Theta =x+\frac{1}{x}$ then what can you say about $\Theta$   ?

Ans. Given that: $cos\Theta =x+\frac{1}{x}$ 

$\Rightarrow cos\Theta =x+\frac{1}{x}$

$$ \begin{aligned} & \Rightarrow x^2+1=x \cos \theta \\ & \Rightarrow x^2-x \cos \theta+1=0 \end{aligned} $$

For real value of $x, b^2-4 a c \geq 0$

$$ \begin{aligned} & \Rightarrow(-\cos \theta)^2-4 \times 1 \times 1 \geq 0 \\ & \Rightarrow \cos ^2 \theta-4 \geq 0 \\ & \Rightarrow \cos ^2 \theta \geq 4 \\ & \Rightarrow \cos \theta \geq \pm 2 \quad[\because-1 \leq \cos \theta \leq 1] \end{aligned} $$

So, the value of $\theta$ is not possible.

9. Find the value of $sin\left ( \frac{\pi }{4}+\Theta  \right )-cos \left ( \frac{\pi }{4}-\Theta  \right )$ .

Ans. Given expression :

$$ \begin{aligned} & \sin \left(\frac{\pi}{4}+\theta\right)-\cos \left(\frac{\pi}{4}-\theta\right) \\ & \sin \left(\frac{\pi}{4}+\theta\right)=\sin \left(\frac{\pi}{4}\right) \cos \theta+\cos \left(\frac{\pi}{4}\right) \sin \theta \\ & =\frac{1}{\sqrt{2}} \cos \theta+\frac{1}{\sqrt{2}} \sin \theta \\ & \cos \left(\frac{\pi}{4}-\theta\right)=\cos \left(\frac{\pi}{4}\right) \cos \theta+\sin \left(\frac{\pi}{4}\right) \sin \theta \\ & =\frac{1}{\sqrt{2}} \cos \theta+\frac{1}{\sqrt{2}} \sin \theta \\ & \therefore \sin \left(\frac{\pi}{4}+\theta\right)-\cos \left(\frac{\pi}{4}-\theta\right) \\ & =\frac{1}{\sqrt{2}} \cos \theta+\frac{1}{\sqrt{2}} \sin \theta-\frac{1}{\sqrt{2}} \cos \theta-\frac{1}{\sqrt{2}} \sin \theta \\ & =0 \end{aligned} $$

10. Find the value of $tan\;\frac{\pi }{12}$ .

Ans. $\tan \frac{\pi}{12}=\tan \left(\frac{\pi}{4}-\frac{\pi}{6}\right)$

$$ \begin{aligned} & =\frac{\tan \frac{\pi}{4}-\tan \frac{\pi}{6}}{1+\tan \frac{\pi}{4} \cdot \tan \frac{\pi}{6}}=\frac{1-\frac{1}{\sqrt{3}}}{1+1 \cdot \frac{1}{\sqrt{3}}}=\frac{\sqrt{3}-1}{\sqrt{3}+1} \\ & =\frac{(\sqrt{3}-1)(\sqrt{3}-1)}{(\sqrt{3}+1)(\sqrt{3}-1)}=\frac{4-2 \sqrt{3}}{2}=2-\sqrt{3} \end{aligned} $$

11. Prove that : $tan\;225^{\circ}\;cot\;405^{\circ}+tan\;765^{\circ}\;cot\;675^{\circ}=0$ 

Ans.

$$ \begin{aligned} & \text { 11. LHS }= \\ & \tan 225^{\circ} \cot 405^{\circ}+\tan 765^{\circ} \cot 675^{\circ} \\ & =\tan \left(180^{\circ}+45^{\circ}\right) \cot \left(360^{\circ}+45^{\circ}\right) \\ & \quad \quad \quad \quad \tan \left(360^{\circ} \times 2+45^{\circ}\right) \cot \left(360^{\circ} \times 2-45^{\circ}\right) \\ & =\tan 45^{\circ} \cot 45^{\circ}+\tan 45^{\circ}\left[-\cot 45^{\circ}\right] \\ & =1 \times 1-1 \times 1 \\ & =0=\text { RHS } \end{aligned} $$

Hence proved.

12. For $0<x<\frac{\pi }{2}$ , show that $\sqrt{\frac{(1-cos2x)}{1+cos2x}}=tan\;x$ 

Ans.

$$ \begin{aligned} & \text { LHS }=\sqrt{\frac{(1-\cos 2 x)}{(1+\cos 2 x)}} \\ & =\sqrt{\frac{1-\left(1-2 \sin ^2 x\right)}{1+\left(2 \cos ^2 x-1\right)}}=\sqrt{\frac{2 \sin ^2 x}{2 \cos ^2 x}} \\ & =|\tan x|=\tan x \quad\left\{\because 0<x<\frac{\pi}{2}\right\} \\ & =\text { RHS } \end{aligned} $$

13. Prove that: $\frac{cos(2\pi +x)cosec(2\pi +x)tan\left ( \frac{\pi }{2}+x \right )}{sec\left ( \frac{\pi }{2}+x \right )cos\;x\;cot(\pi +x)}=1$ 

Ans. LHS $=$

$$\cos (2 \pi+x) \operatorname{cosec}(2 \pi+x) \tan \left(\frac{\pi}{2}+x\right)$$

$$ \begin{aligned} & \sec \left(\frac{\pi}{2}+x\right) \cos x \cot (\pi+x) \\ \Rightarrow & \frac{-\cos x \operatorname{cosec} x \cot x}{-\operatorname{cosec} x \cos x \cot x}=1 \end{aligned} $$

Hence proved.

Important Formulas of Class 11 Chapter 3 you shouldn’t Miss!

1. Basic Trigonometric Identities:

• $\sin^2 \theta + \cos^2 \theta = 1$

• $1 + \tan^2 \theta = \sec^2 \theta$

• $1 + \cot^2 \theta = \csc^2 \theta$

2. Angle Sum and Difference Formulas:

• $\sin(A \pm B) = \sin A \cos B \pm \cos A \sin B$

• $\cos(A \pm B) = \cos A \cos B \mp \sin A \sin B$

• $\tan(A \pm B) = \frac{\tan A \pm \tan B}{1 \mp \tan A \tan B}$

3. Double Angle Formulas:

• $\sin 2\theta = 2 \sin \theta \cos \theta$

• $\cos 2\theta = \cos^2 \theta - \sin^2 \theta$

• $\tan 2\theta = \frac{2 \tan \theta}{1 - \tan^2 \theta}$

4. Product-to-Sum Formulas:

• $\sin A \sin B = \frac{1}{2} [\cos(A - B) - \cos(A + B)]$

• $\cos A \cos B = \frac{1}{2} [\cos(A - B) + \cos(A + B)]$

• $\sin A \cos B = \frac{1}{2} [\sin(A + B) + \sin(A - B)]$

5. Inverse Trigonometric Functions:

• $\sin^{-1} (\sin \theta) = \theta, \quad \text{for} \ -\frac{\pi}{2} \leq \theta \leq \frac{\pi}{2}

• $\cos^{-1} (\cos \theta) = \theta, \quad \text{for} \ 0 \leq \theta \leq \pi

• $\tan^{-1} (\tan \theta) = \theta, \quad \text{for} \ -\frac{\pi}{2} < \theta < \frac{\pi}{2}$

Importance of Trigonometric Functions Class 11 Notes

The importance of Trigonometric Function Class 11 notes lies in their role in helping students build a strong foundation in trigonometry, which is essential for advanced mathematical studies. Here’s why these notes are crucial:

1. Clear Conceptual Understanding: The notes simplify complex trigonometric concepts, making it easier for students to grasp fundamental ideas like identities, functions, and equations.

2. Exam Preparation: Well-structured notes provide a comprehensive review of key formulas, theorems, and problem-solving techniques, which are vital for scoring well in exams.

3. Application in Higher Studies: Trigonometry forms the basis for various topics in Class 12 and competitive exams like JEE. Mastery of this chapter ensures a smoother transition to advanced topics.

4. Problem-solving Skills: These notes offer step-by-step solutions to typical problems, helping students develop strong analytical and problem-solving abilities.

5. Quick Revision: Concise and organised, these notes are perfect for quick revision before exams, ensuring students can recall essential concepts and formulas easily.

Tips for Learning the Class 11 Maths Chapter 3 Trigonometric Functions

Here are some helpful tips for learning the Class 11 Maths Chapter 3 Trigonometric Functions:

1. Master the Basics: Ensure you have a strong understanding of basic trigonometric ratios and identities from earlier classes. This foundation is crucial for tackling more complex concepts in this chapter.

2. Memorise Key Formulas: Focus on memorising important trigonometric identities, angle sum and difference formulas, and double angle formulas. These are frequently used in solving problems and are essential for success.

3. Practice Regularly: Trigonometry requires consistent practice. Work through a variety of problems from your textbook and additional reference materials to become comfortable with different types of questions.

4. Visualise with Unit Circle: Use the unit circle to visualise the relationships between different trigonometric functions. This can help in understanding how angles and functions are related.

5. Work on Inverse Functions: Spend extra time understanding inverse trigonometric functions, as they can be tricky. Practice converting between trigonometric functions and their inverses.

Conclusion

Mastering the concepts in Class 11 Maths Chapter 3, Trigonometric Functions, is essential for building a solid foundation in mathematics. By focusing on understanding key formulas, practising regularly, and applying the tips provided, students can confidently tackle trigonometric problems and excel in their exams. Regular revision and consistent practice are key to success in this crucial chapter.

Related Study Materials for Class 11 Maths Chapter 3 Trigonometric Functions

Students can also download additional study materials provided by Vedantu for 

Class 11 Maths Trigonometric Functions.

Revision Notes Links for Class 11 Maths

Important Study Materials for Class 11 Maths