Revision Notes for CBSE Class 11 Physics Chapter 7 (Gravitation) - Free PDF Download
FAQs on Gravitation Class 11 Notes CBSE Physics Chapter 7 (Free PDF Download)
1. What are the Benefits of Studying with Vedantu?
The benefits of studying with Vedantu lies hereunder.
Step-wise guidance from our faculties to score well in your exams.
Free PDF of CBSE Physics Class 11 revision notes.
Summary of the NCERT books of all chapters of Physics class 11.
On the spot solutions to your queries from our doubt experts.
Concise study materials, free tutorials, and solutions to all PYQP.
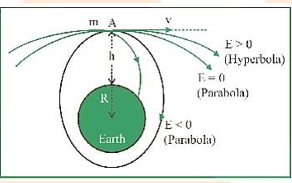
2. What do you Mean by Gravitational Potential?
The gravitational potential at a given point is the amount of work done in bringing a unit mass from infinity to that point without acceleration.
3. Write the Difference Between Mass and Weight.
Mass of an object is the amount of matter it contains, whereas the weight of an object is the measure of the force exerted on it by the gravity or the measure of how much gravitational attraction an object feels.
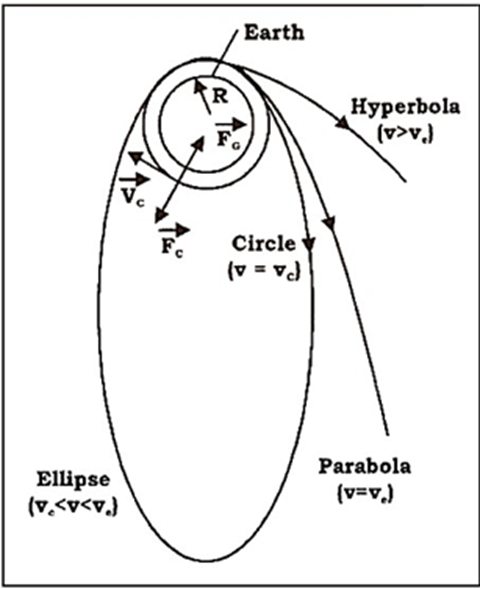
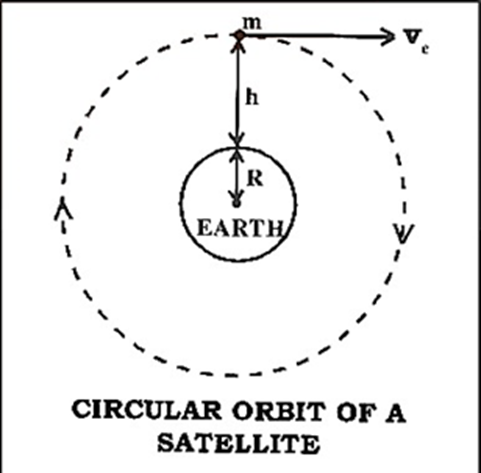
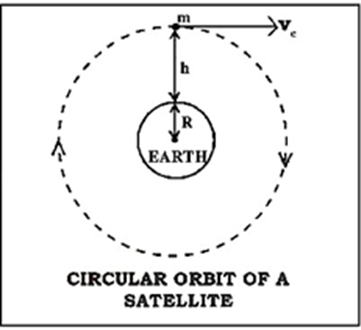
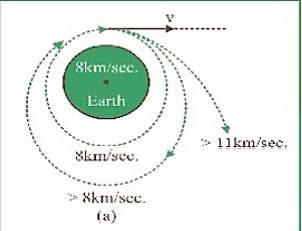
4. What is Escape Velocity?
Escape velocity is the minimum speed required by a non-propellant object to escape the Earth by counteracting its gravitational pull.
On the surface of Earth, the escape velocity (ve) is 11.2 Km/s (this speed is 33 times the speed of sound).
5. What are some important questions in Chapter 7 of Class 11 Physics?
Chapter 7 of Class 11 Physics is all about gravitation and it is one of the most important chapters in Physics for Class 11 students. For this reason, some very important questions are asked from this chapter and which form the base for higher studies as well. The topics stated below cover the areas from where these important questions are asked:
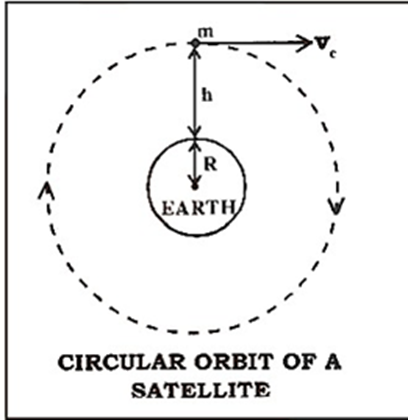
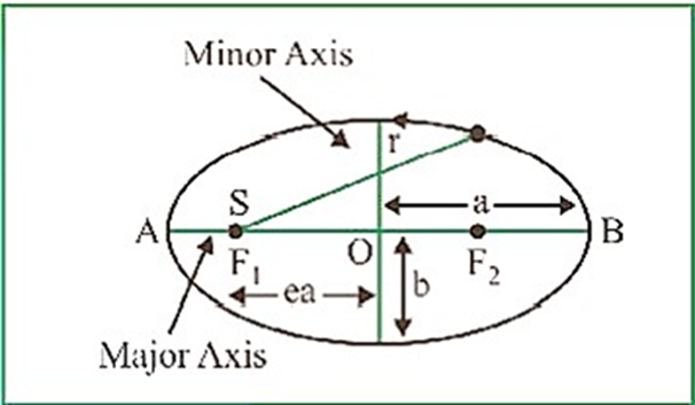
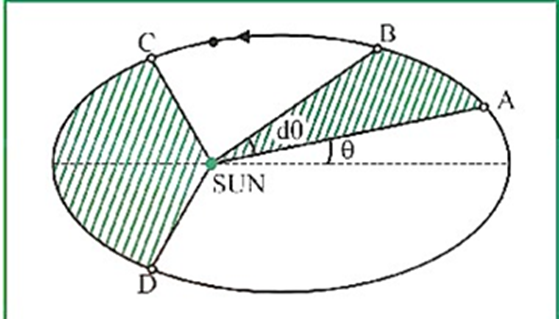
Kepler’s Laws and Universal Law of Gravitation
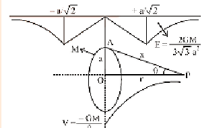
Gravitational Potential Energy
Characteristics of gravitational force
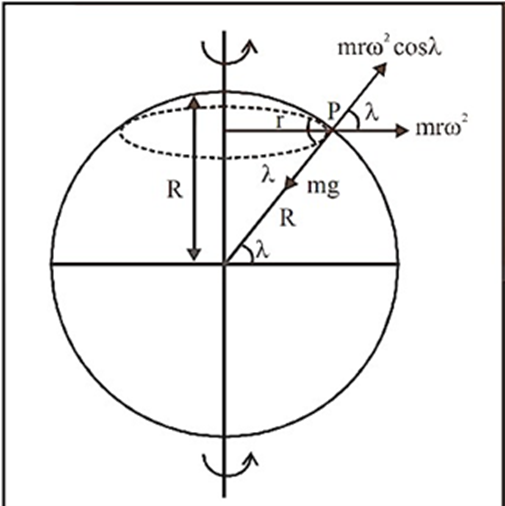
Acceleration due to gravity.
6. How can I understand Chapter 7 of Class 11 Physics?
In Class 11, students are introduced to the concept of gravitation in detail. The topic is very complicated and understanding it requires a little bit of help. The revision notes available on Vedantu are a one-stop destination that students can use to understand the chapter and topics related to gravitation. All the concepts and topics in the chapter that pass over the students’ heads are broken down into simple terms for easy comprehension and understanding. Thus, students would be able to grasp everything in the chapter. You can also access the study materials through the Vedantu App. All the resources are available free of cost.
7. What are the topics covered in Chapter 7 of Class 11 Physics?
The subject of Physics introduces students to gravitation and its various concepts in Chapter 7. Students are taught Kepler’s Laws on gravitation, Newton’s Law or the Universal Gravitational Law and its constant, characteristics of gravitational force, gravitational potential energy, acceleration due to gravity, and problems related to it. Thus, the chapter equips students with all they need to know about the basics of gravitation required for their higher studies. In short, the chapter teaches them all about gravitation.
8. What are some important equations in Chapter 7 of Class 11 Physics?
Since Chapter 7 of Class 11 Physics focuses on the concepts regarding gravitation, the important equations of the chapter would follow closely behind all these concepts. Once students understand the basics and ideas behind the formula, they can solve all problems with ease. Some important formulae are pointed out below:
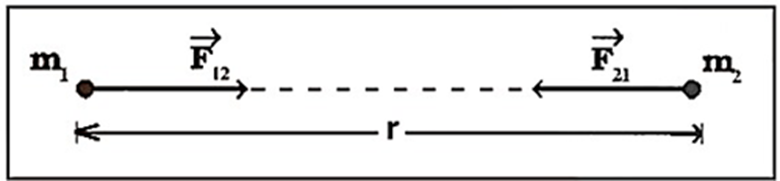
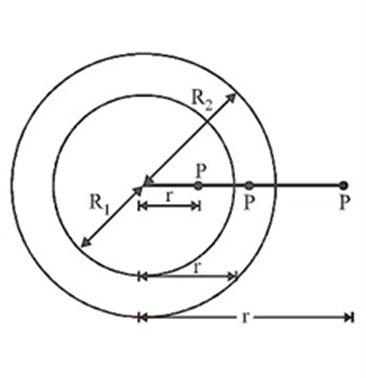
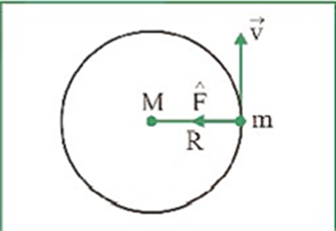
Universal Law of Gravitation: F=Gm1m2/r2
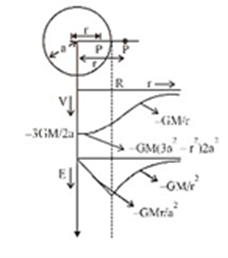
Relationship between G and g is g=Gm/R2
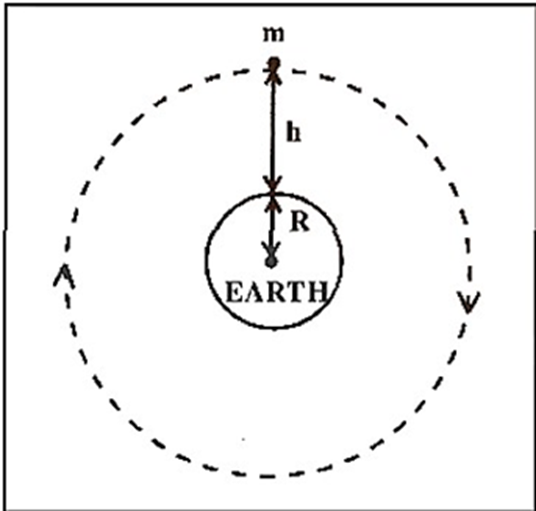
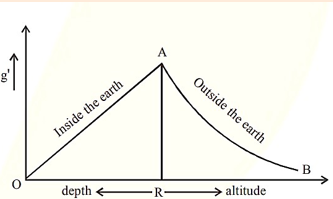
At a particular height, acceleration due to gravity: ga=g[1-(2h/R)]
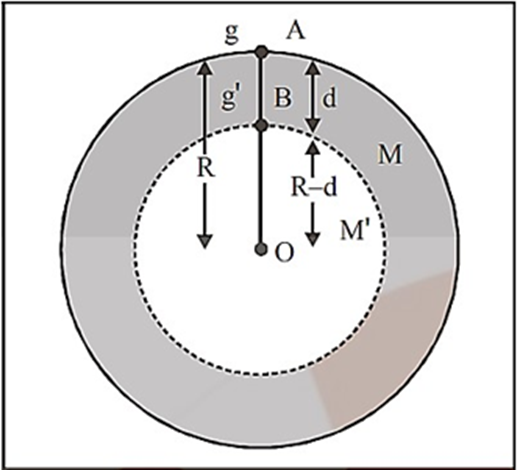
Similarly, at a certain depth: gd=g[1-(h/R)]
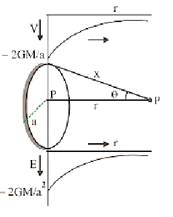
Gravitational field strength: E=F/m
9. What are the characteristics of gravitational force?
When two masses are separated by a distance, a force of attraction acts between them. This force is what is called the gravitational force. Three major characteristics of the gravitational force are discussed in Class 11:
Since the force exists between the two particles, it is long-ranged in nature as they do not depend on the medium separating them.
There is an inversely proportional relationship between them, that is, force and distance.
This force is attractive in nature.
10. What is the universal law of gravitation?
The universal law of gravitation states that every particle in the universe attracts every other particle with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them.
11. What is the significance of gravitational fields?
Gravitational fields represent the influence of a mass in the space around it. They show how the force of gravity would affect other masses placed in that space. Understanding gravitational fields helps in analysing how objects interact with gravity.
12. How can I use these notes for exam preparation?
Focus on understanding key concepts and formulas, solve practice problems, and review summaries. Use the notes to clarify any doubts and make sure you can apply the concepts to different types of questions you might encounter on the exam.
13. Where can Gravitation Notes Class 11 PDF download?
Gravitation Notes Class 11 PDF can be Downloaded by our Vedantu Website.