Class 6 Maths NCERT Exemplar Solutions Chapter 3 Integers
FAQs on NCERT Exemplar for Class 6 Maths Solutions Chapter 3 Integers
1. What are important topics in NCERT Exemplar for Class 6 Maths Solutions Chapter 3 Integers?
The NCERT Grade 6 Mathematical Model Solutions for Chapter 3 Mathematics are so comprehensively designed by the experts at Vedantu that they integrate all the parts of the Chapter simply to help you practice the difficult ones.
The questions in Chapter 3 include tests with real-life examples to help you grasp conflicting numbers.
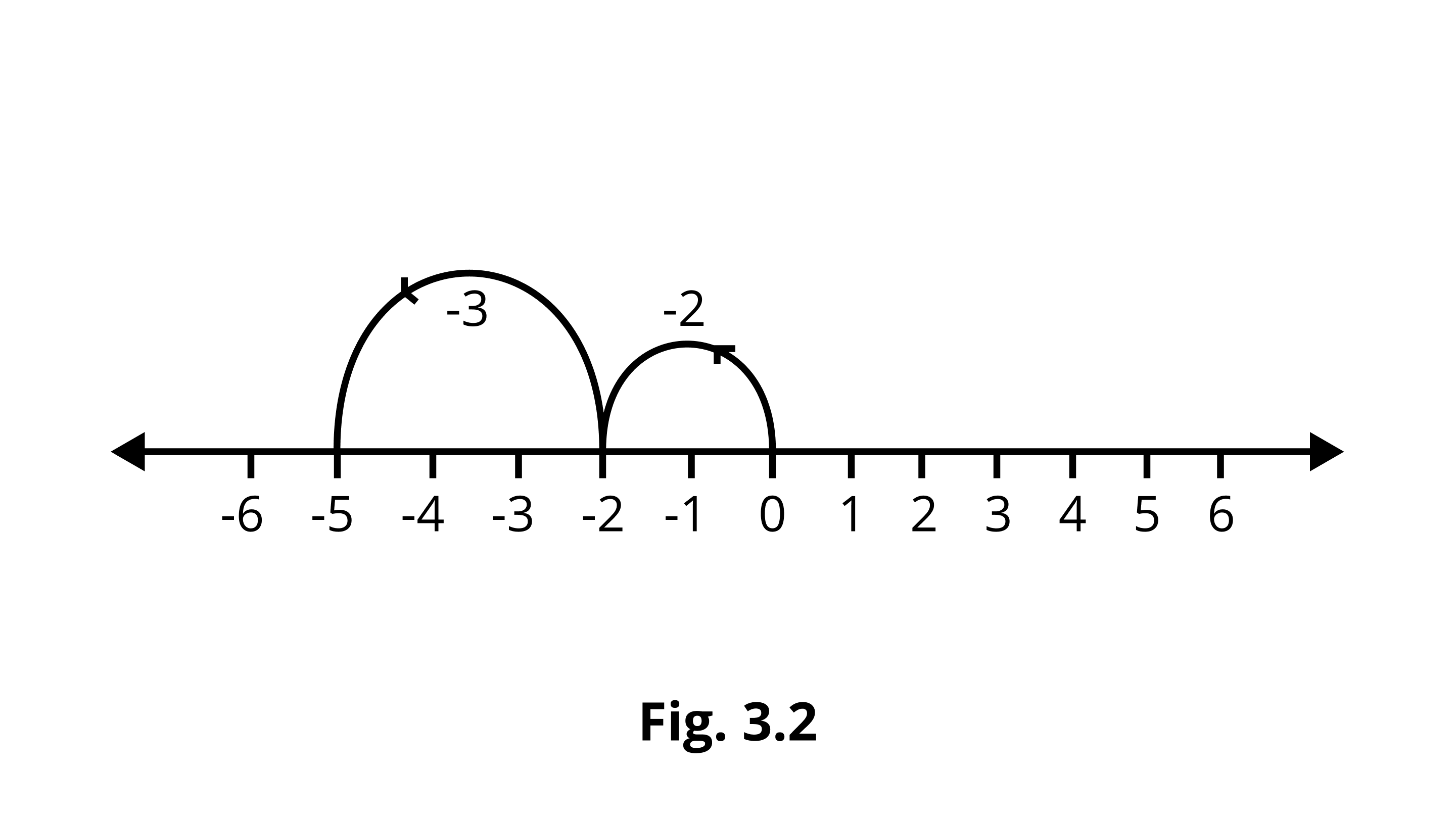
Questions also enable you to practice the correct use of positive and negative numbers. There are exercise questions based on all topics such as representation on the number line, addition and subtraction of numbers.
2. How can you get good grades in Class 6 Mathematics using NCERT Exemplar for Class 6 Maths Solutions Chapter 3 Integers?
The syllabus of the Integer’s Chapter is deeply covered, adhering to the latest trends in the CBSE test pattern with the help of practice questions.
To get good marks in the test, the students should only look at NCERT Solutions and NCERT Exemplar Class 6 Science Solutions as they are self-contained and include the entire CBSE syllabus. The NCERT Exemplar section 3 of the NCERT Mathematics is designed in easy-to-understand and systematic language to address each topic step by step.
3. Why Use NCERT Exemplar Solutions Class 6 Maths Chapter 3 by Vedantu?
NCERT Exemplar Solutions for Class 6 Maths Chapter 3 are very important from a test perspective as they are designed keeping in mind the latest CBSE test patterns.
At Vedantu, exemplary solutions are developed after extensive research on the topic and therefore, are a reliable source of your questions.
We have a specially selected team to take care of all your topic-related needs.
The goal of the Vedantu expert is to make the lesson engaging and engaging for you and therefore to provide a solid foundation for your advanced Classes.
4. Is it important to solve NCERT Exemplar for Class 6 Maths Solutions Chapter 3 Integers?
NCERT Class 6 Mathematics Chapter 3 Integers Exemplar Solutions. The exemplar questions were developed by NCERT to include practice questions of all levels and abilities of students. Questions include multiple-choice questions, short answer questions, long answer questions. The sample questions are very important and should be solved by students so that they can understand the concepts of Chapter 3 Integers Exemplar Solutions for Class 6. This will help the students to understand the Chapter correctly and identify any way to improve. The solutions provided here are prepared by experienced teachers and are free to download via PDF. You can click on the links for other Math Chapters to download some free solutions and resources.
5. Is Vedantu a reliable website for downloading NCERT Exemplar for Class 6 Maths Solutions Chapter 3 Integers?
Whenever a student starts preparing his or her mind strikes with one common question: what are the types of questions that will be appearing in the exams. The experts at Vedantu have left no stones unturned to make it simple for the students of Class 6. We have curated the best content and study material to assist the students in preparation. Every student must follow the question pattern and marking scheme in the NCERT Exemplar Class 6 Maths Solutions Chapter 3 Integers