Class 7 Maths NCERT Exemplar Solutions Chapter 9 Perimeter & Area
Free PDF download of NCERT Exemplar for Class 7 Maths Chapter - 9 Perimeter & Area solved by expert Maths teachers on Vedantu.com as per NCERT (CBSE) Book guidelines. All Chapter - 9 Perimeter & Area exercise questions with solutions will help you to revise and complete the syllabus to score more marks in your examinations.
Every NCERT Solution is provided to make the study simple and interesting on Vedantu. Subjects like Science, Maths, English will become easy to study if you have access to NCERT Solution for Class 7 Science, Maths solutions, and solutions of other subjects. You can also download NCERT Solutions for Class 7 Maths to help you to revise the complete syllabus and score more marks in your examinations.
Access NCERT Exemplar Solutions for Class 7 Mathematics Chapter 9 - Perimeter and Area
Solved Examples
In Examples 1 and 2, there are four options, out of which one is correct. Choose the correct one.
1. Following rectangle is composed of
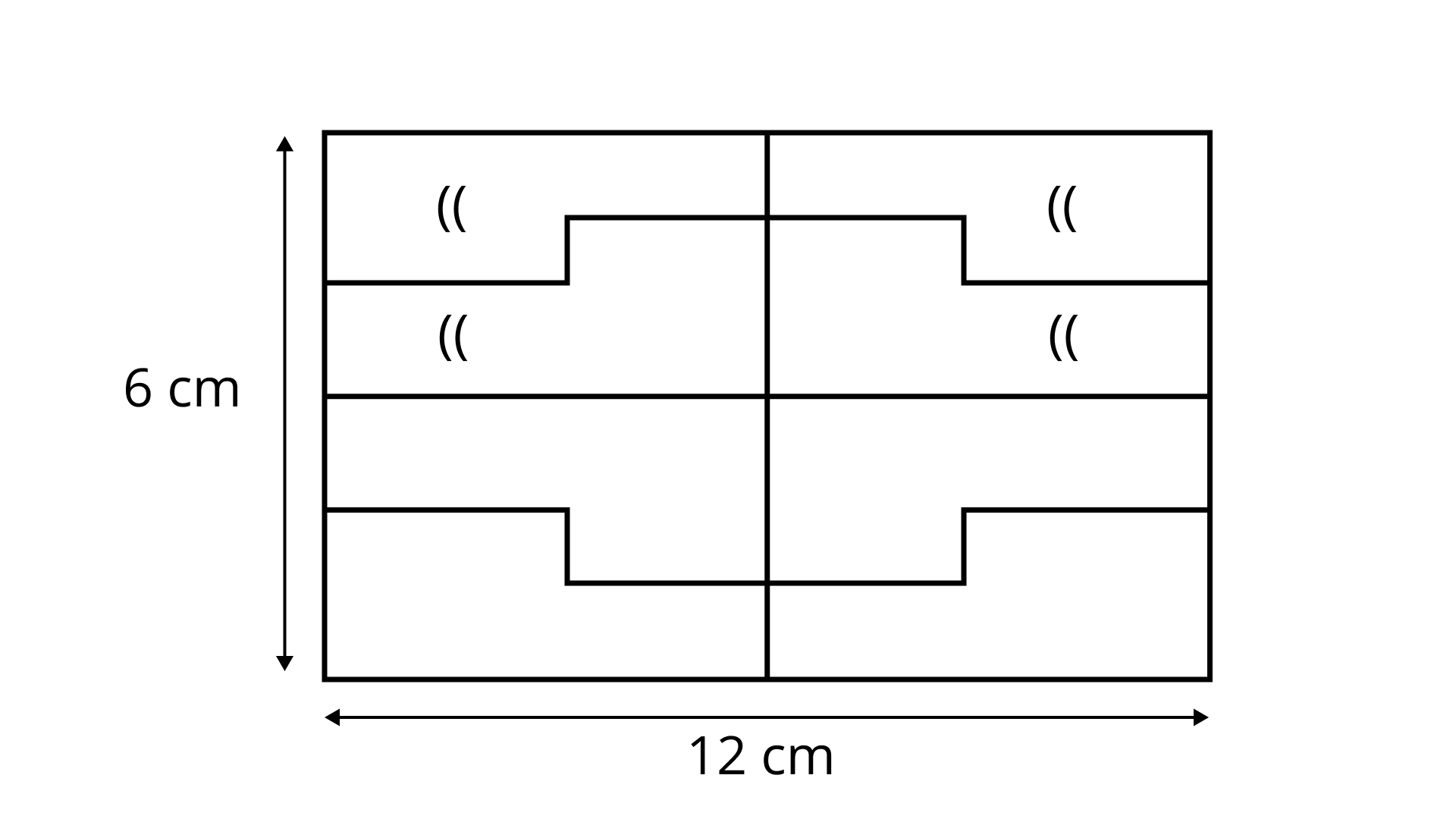
Area of each part is
(a)
(b)
(c)
(d)
Ans: Correct answer is (d).
Area of rectangle
Since, rectangle is equivalent to 8 congruent
part,
As, we know congruent figures have equal areas
So, area of rectangle
So, area of one congruent part is
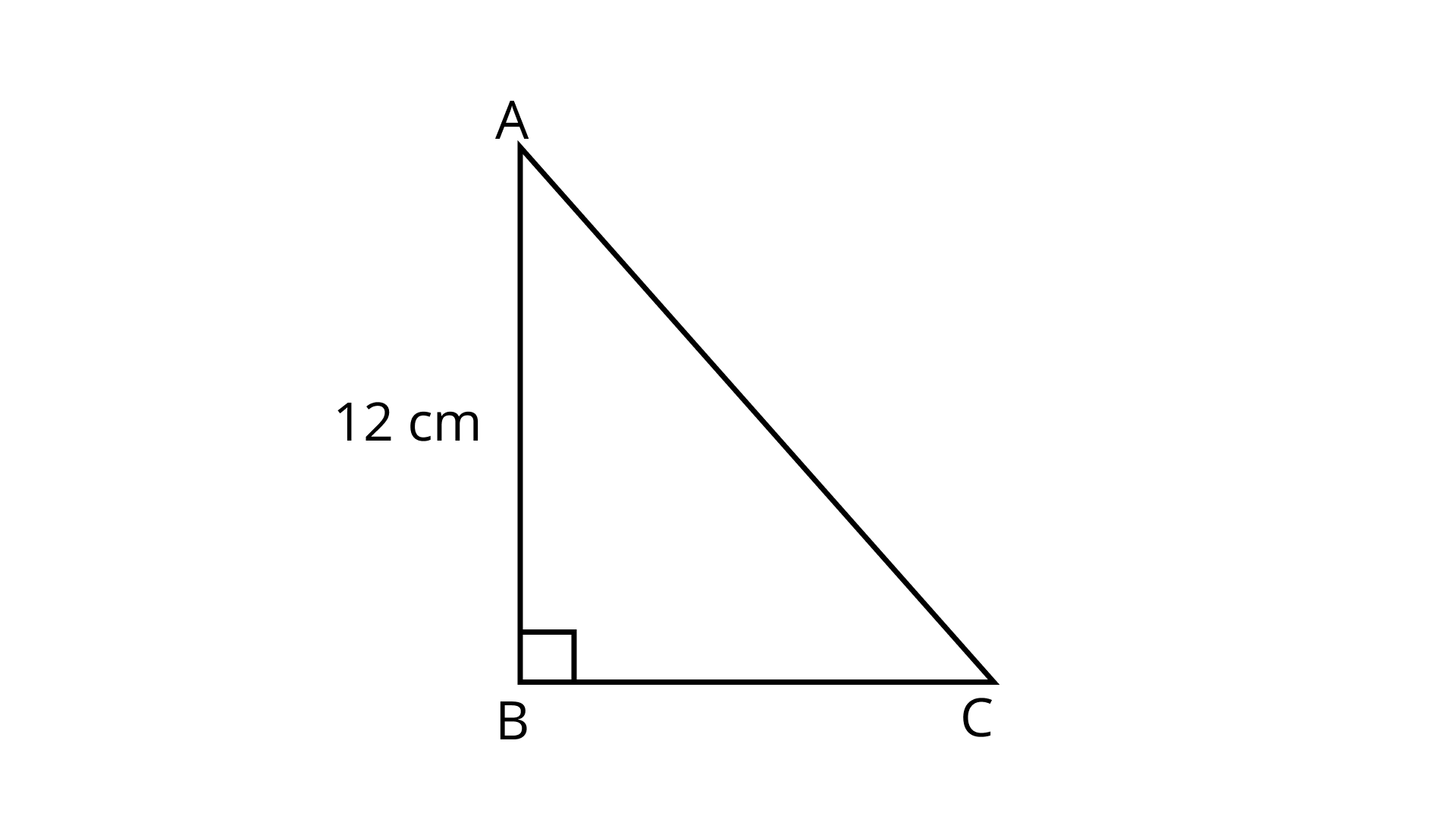
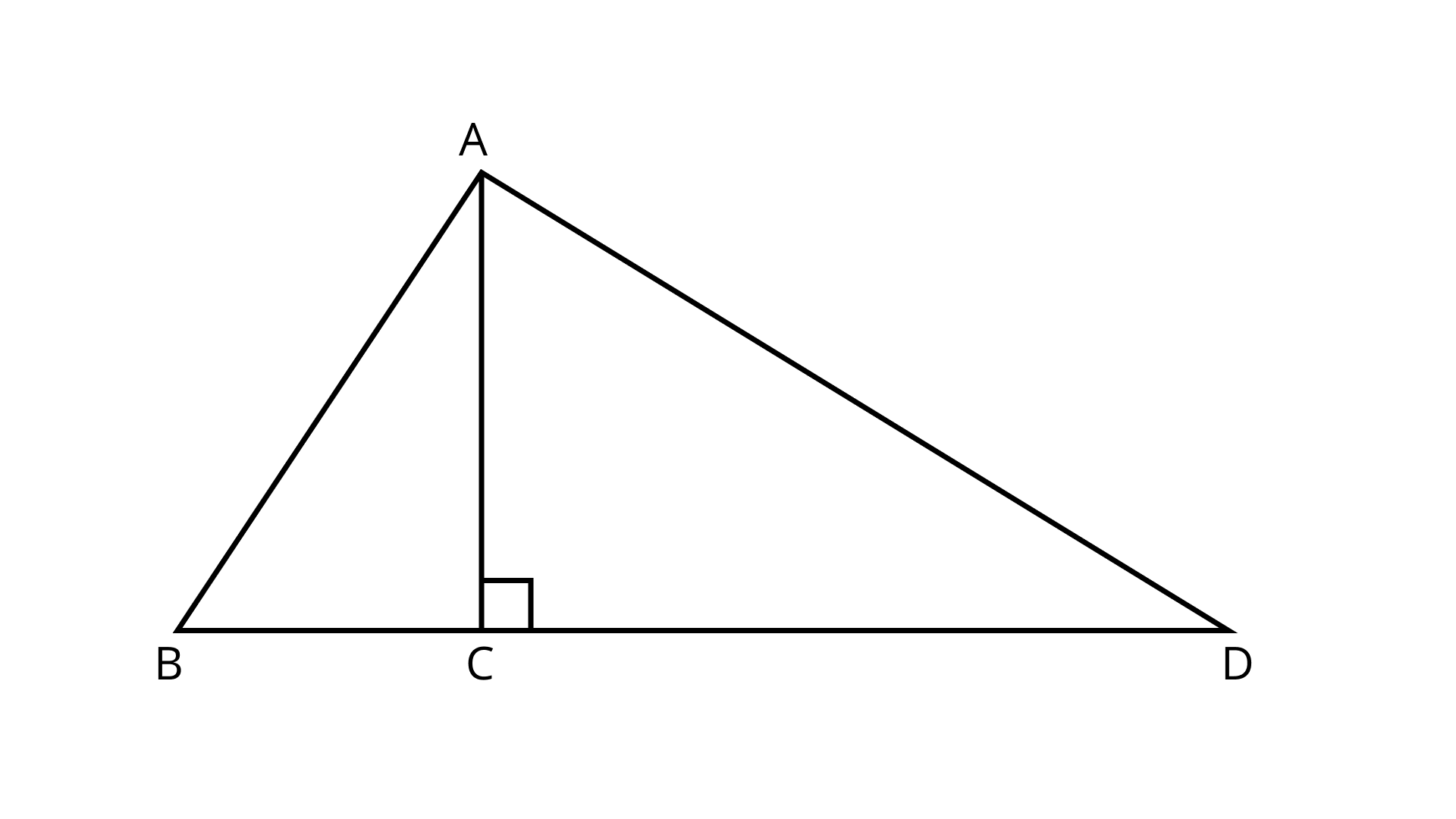
2. Area of a right triangle is
(a)
(b)
(c)
(d)
Ans: Correct answer is (c).
Given area
One of the side
Let us consider base as
Now
Area
Now for perimeter we need to find hypotenuse
Thus, by pythagoras
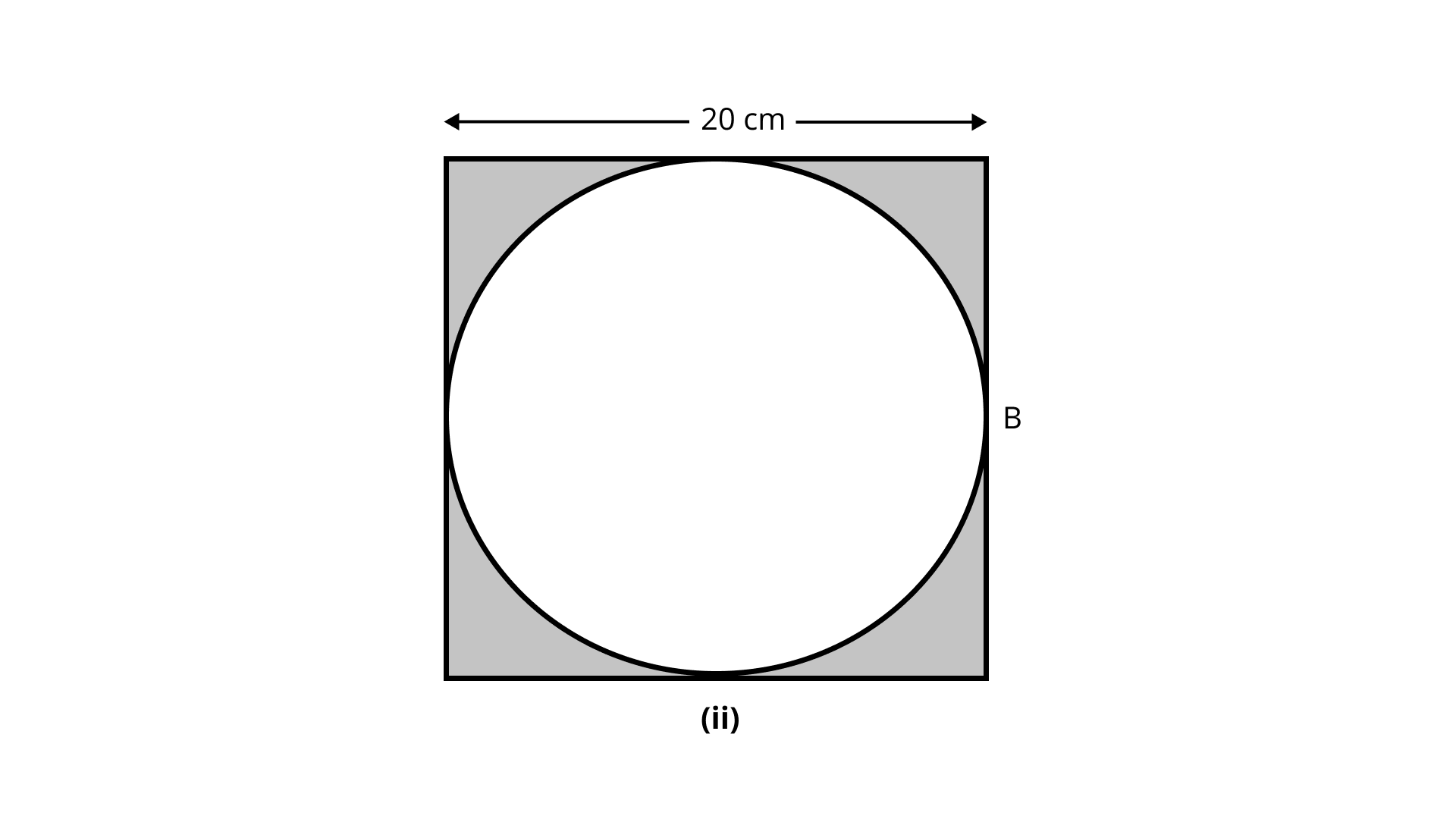
Thus,
Now perimeter
In Examples 3 to 6, fill in the blanks to make it a statement true.
3. Area of parallelogram QPON is ___
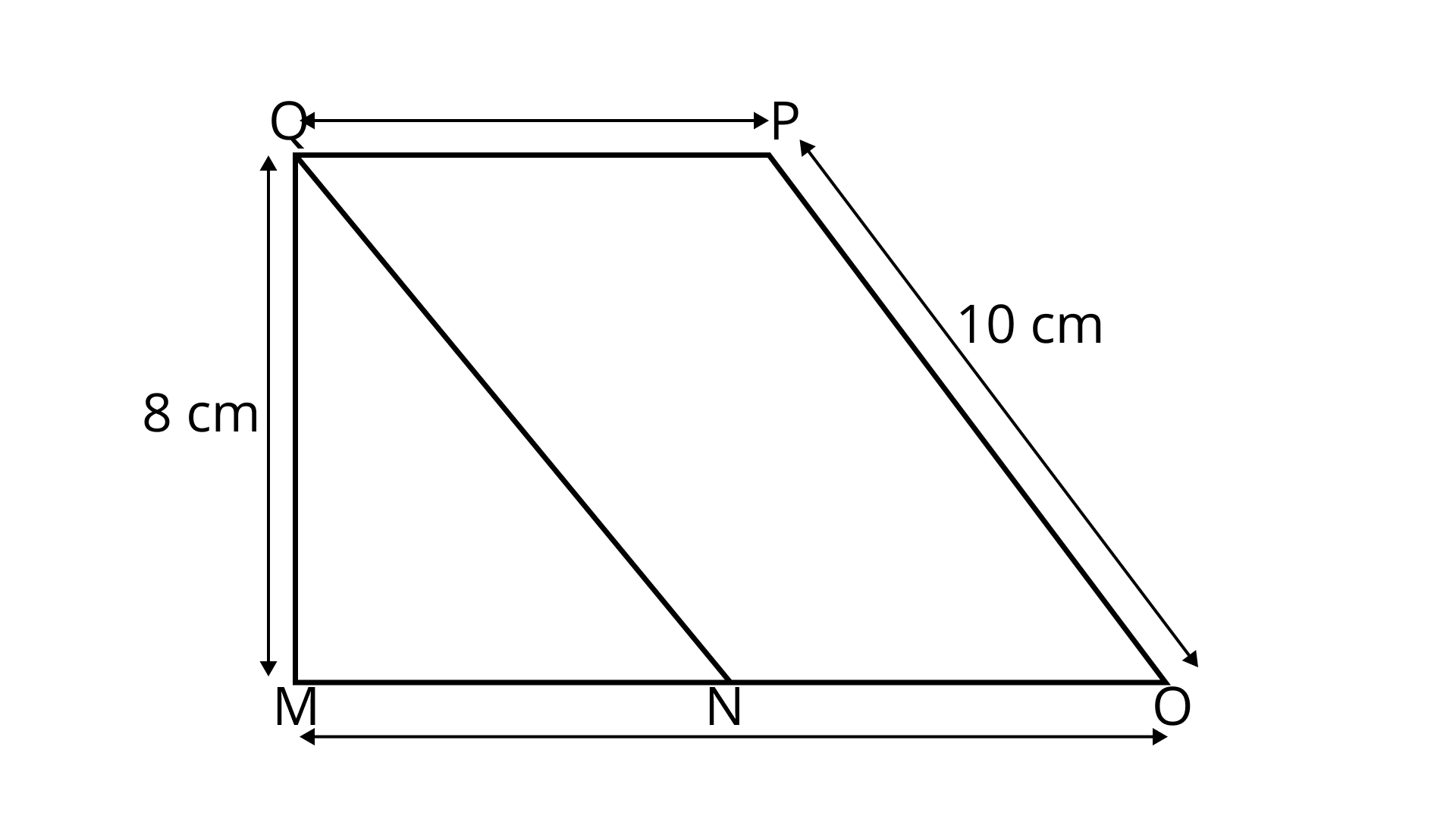
Ans: In a parallelogram, opposite sides are parallel and equal.
Distance between parallel lines
Area of parallelogram
Hence, Area of parallelogram
4.
Ans:
5. ______squares of each side
Ans: Area of bigger square
Area of smaller square
Number of squares
6. All the congruent triangles have ___area.
Ans: All the congruent triangles have equal area.
In Examples 7 to 10, state whether the statements are True or False.
7. All the triangles equal in area are congruent.
Ans: The given statement is False
8. The area of any parallelogram
Ans: The given statement is False.
9. Ratio of the circumference and the diameter of a circle is more than
Ans:
Thus the given statement is True.
10. A nursery school playground is
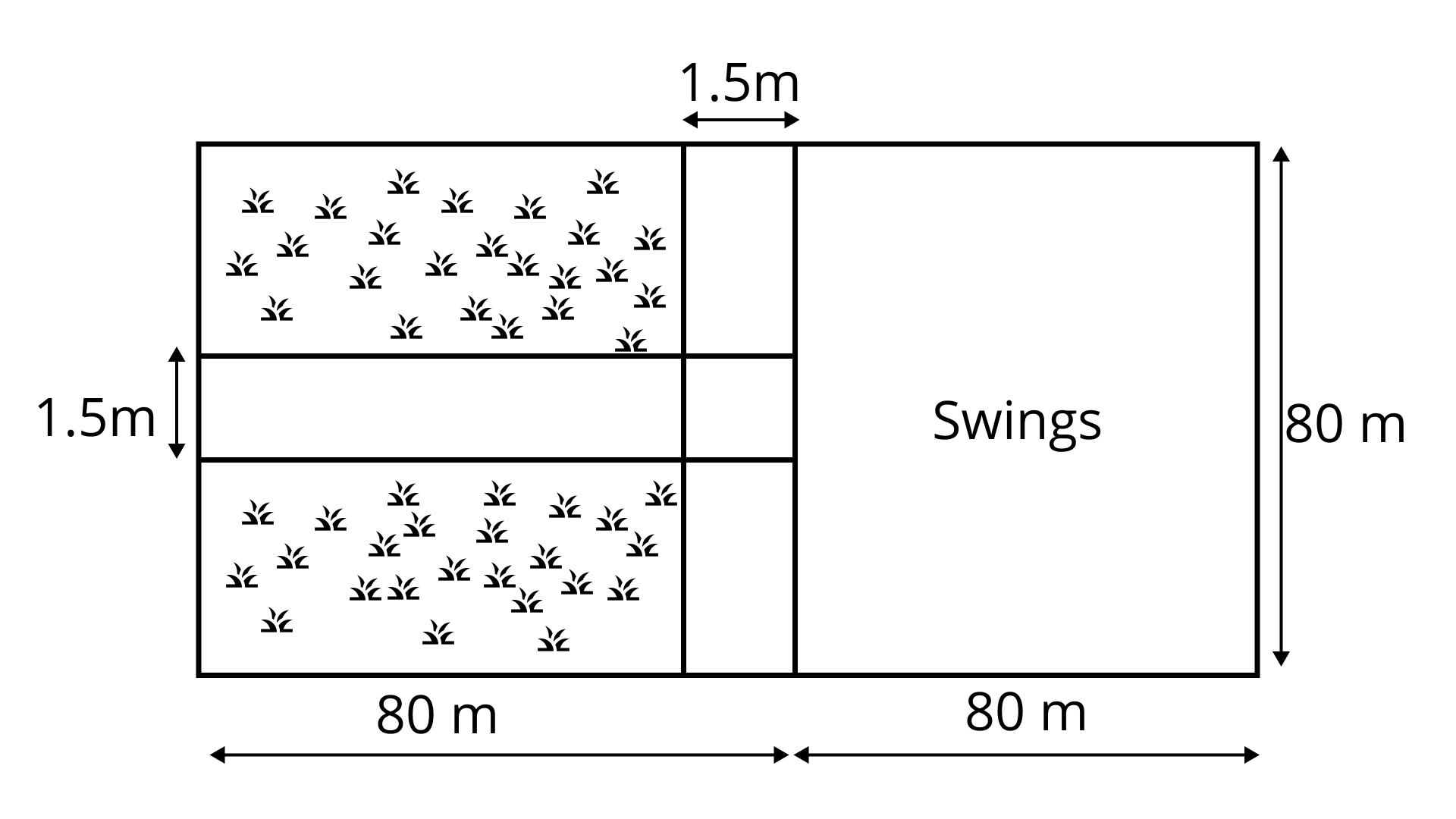
Ans: Area of school playground is
Area kept for swings
Area of path parallel to the width of playground
Area of path parallel to the remaining length of playground
Area common to both paths
Total area covered by both the paths
Area covered by grass
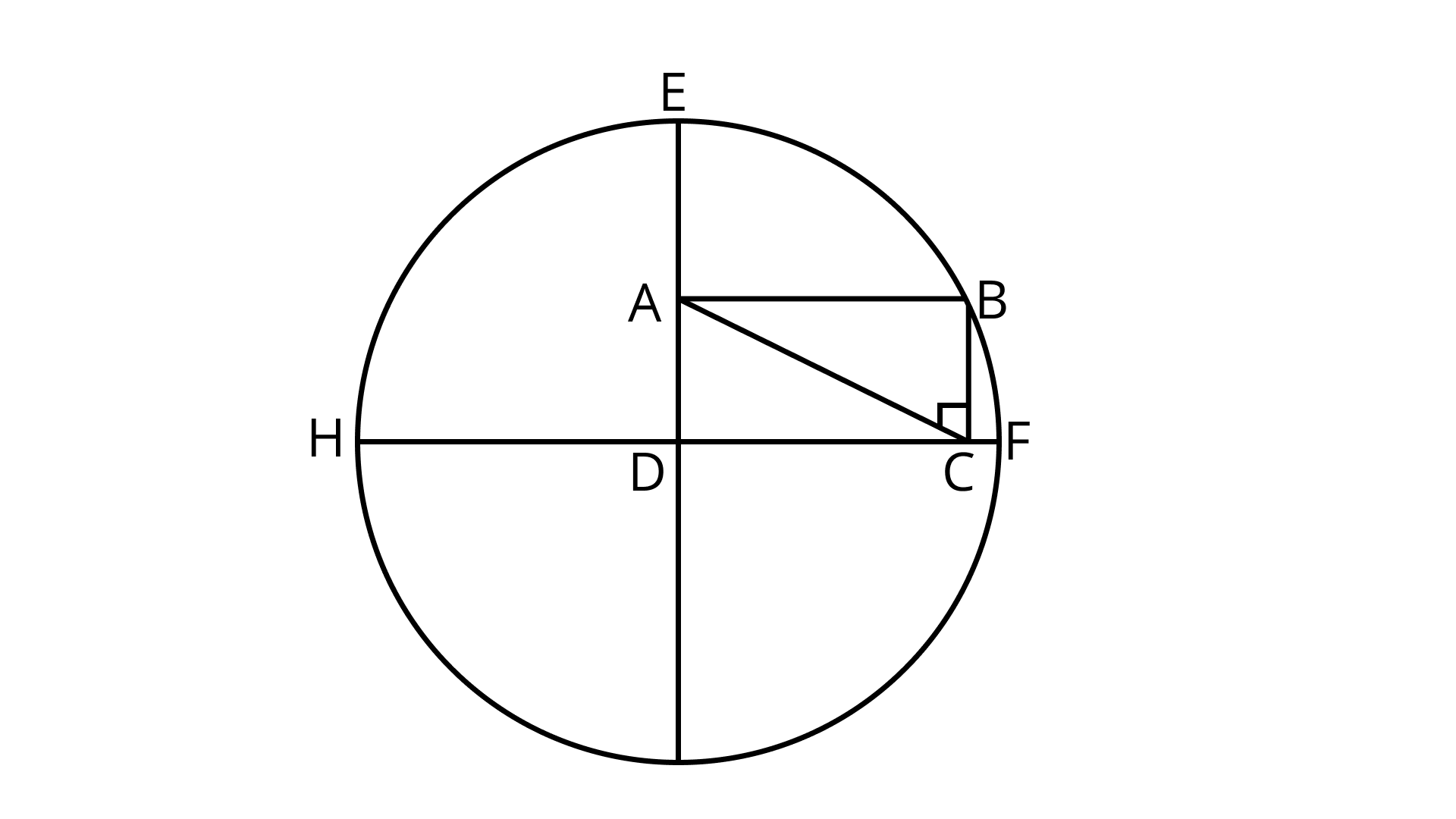
11. In Fig. 9.10,
Ans: Area of parallelogram
Let altitude corresponding to
Thus, the altitude corresponding to
12. A rectangular shaped swimming pool with dimensions

Ans: Area covered by swimming pool
Length of outer rectangle
So, the area of outer rectangle
Area of cemented path = Area of outer rectangle - Area of swimming pool =
Cost of cementing
So, total cost of cementing the path
13. Circumference of a circle is
Ans: Let the radius of the circle be
Then,
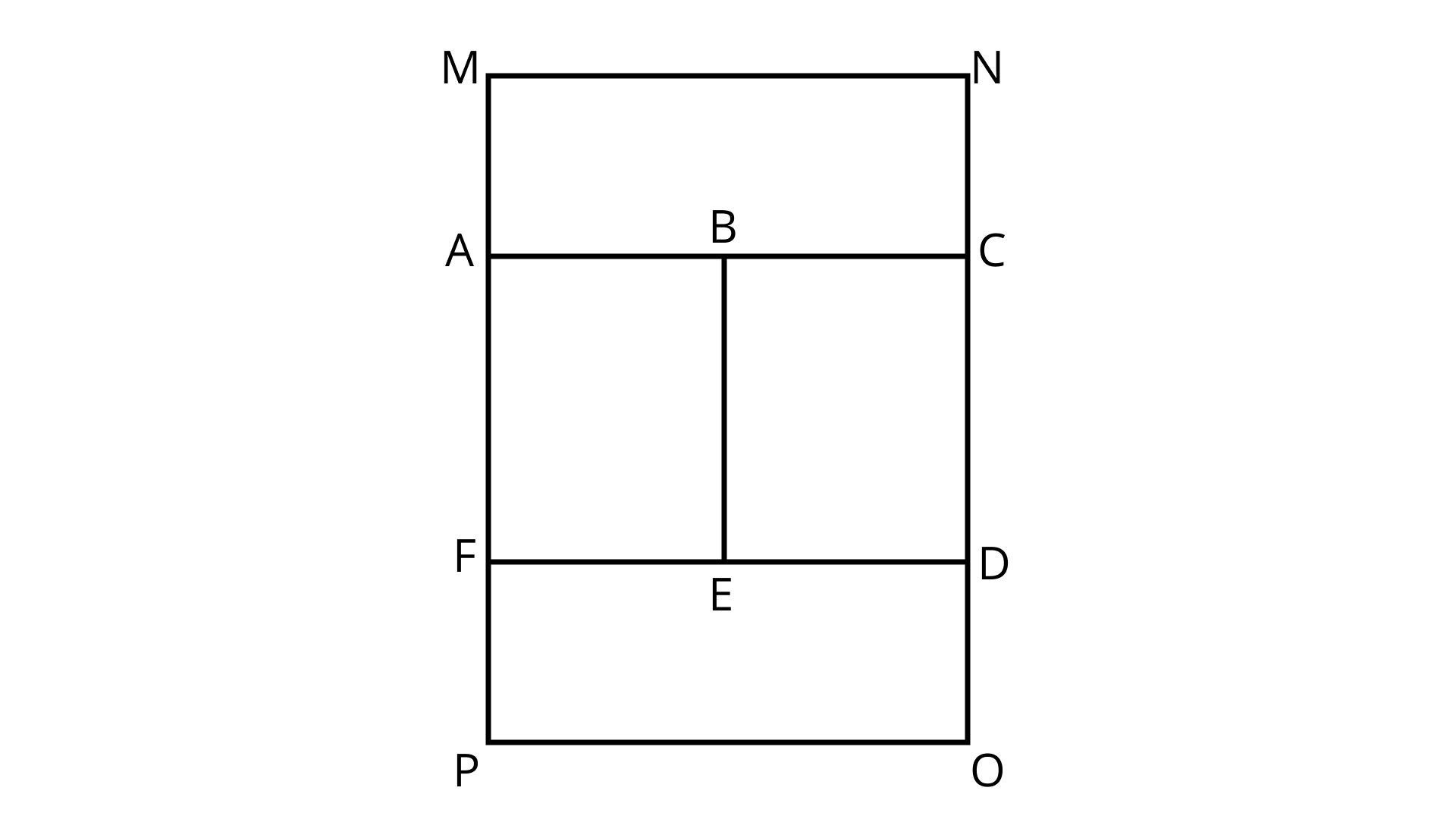
So,
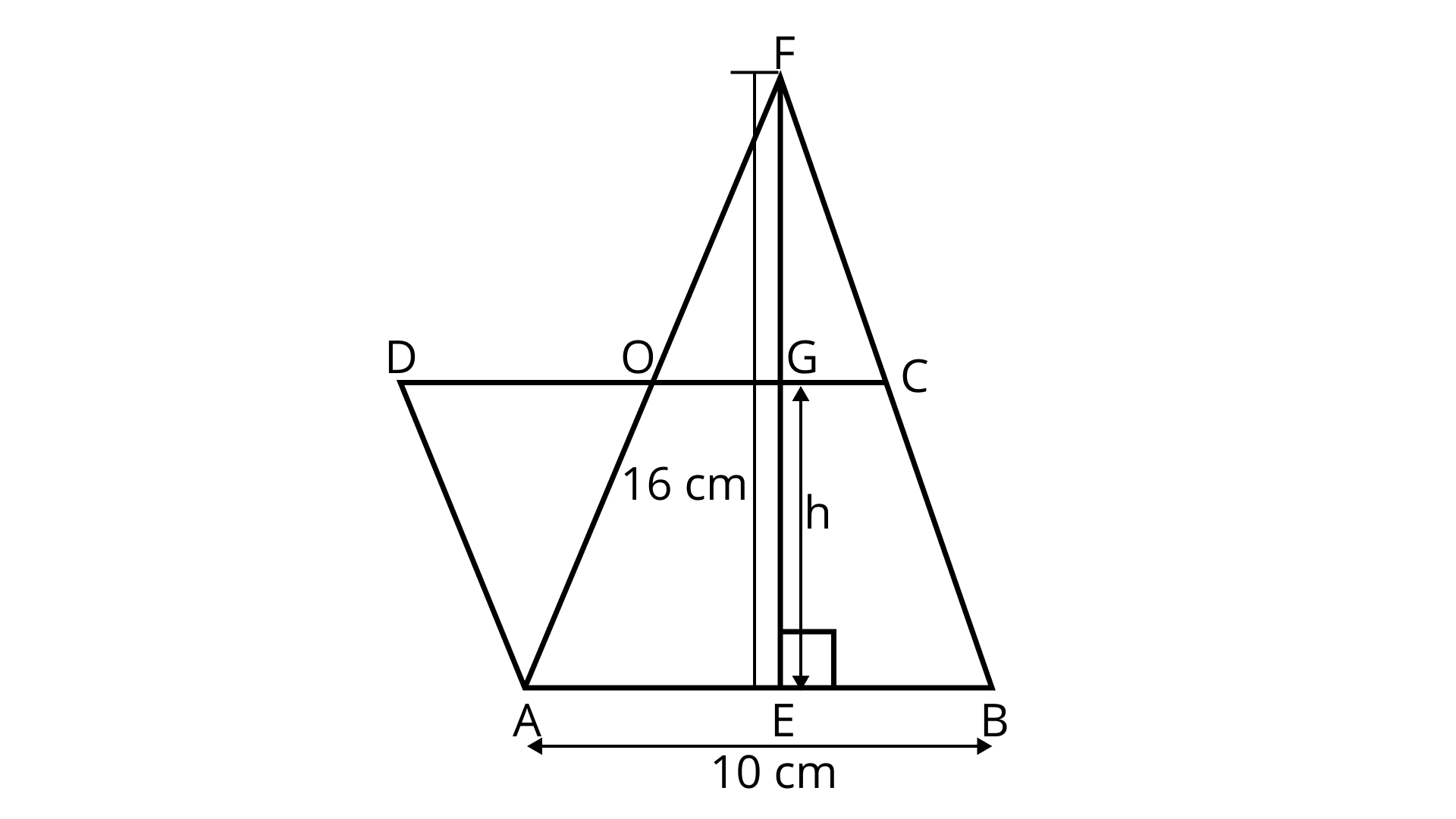
Thus, radius is
So, area of the circle
Thus, the area of the circle is
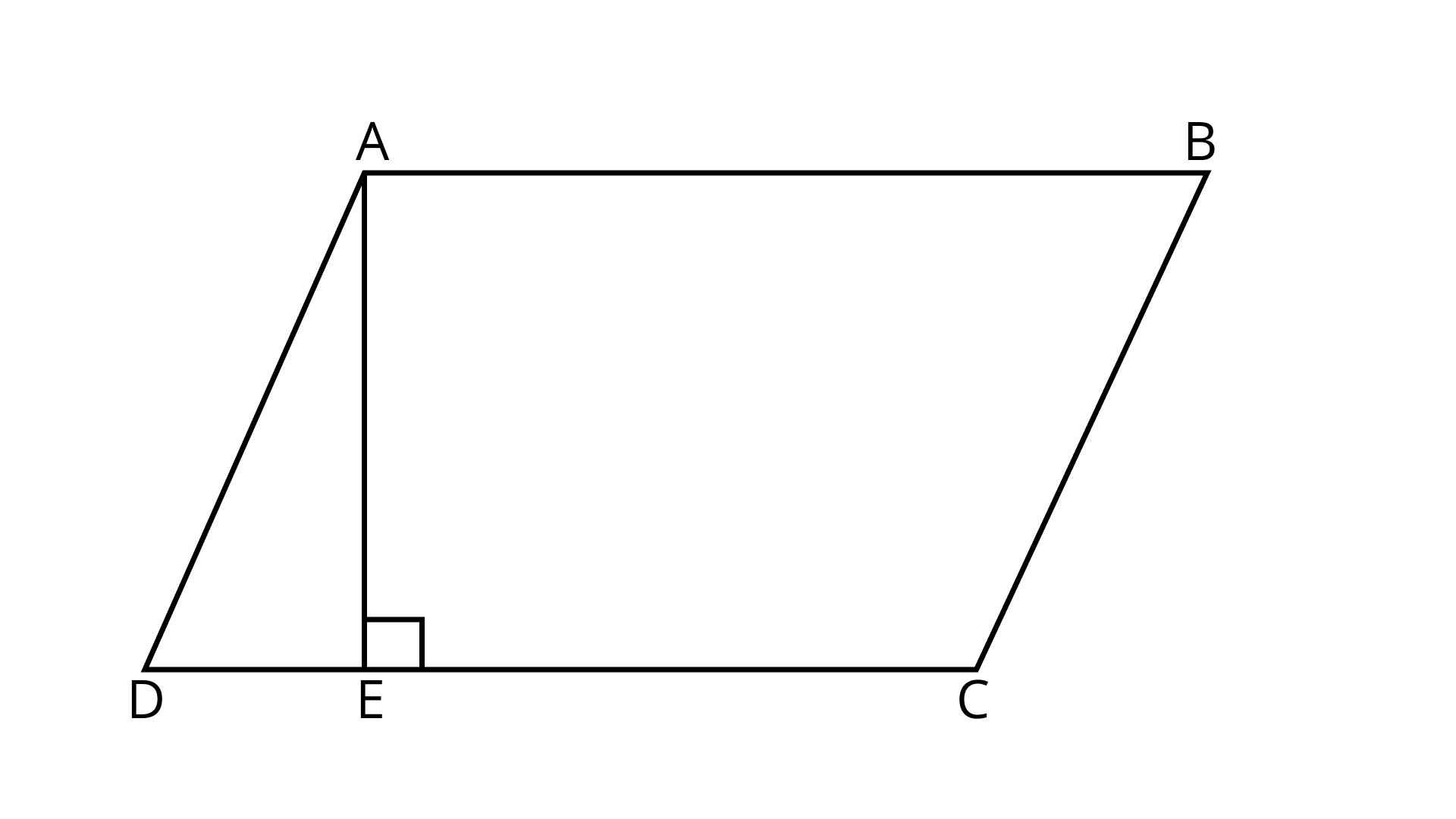
14. Rectangle
Ans:
DE is the radius of the circle.
Also, DB is the radius of the circle.
Next,
Therefore,
From
So,
Thus, length of
Hence, perimeter of the rectangle
15. Find the area of a parallelogram-shaped shaded region of Fig. 9.13. Also, find the area of each triangle. What is the ratio of the area of the shaded portion to the remaining area of the rectangle?

Ans: In DEBF the base is FB and height is AD.
We know area of a parallelogram is base
Area of DEBF = FB
In DAF the base is AF and height is AD
Area of a triangle =
In BCE the base is EC and height is CB
Area of a triangle =
Area of each triangle is
Area of unshaded part
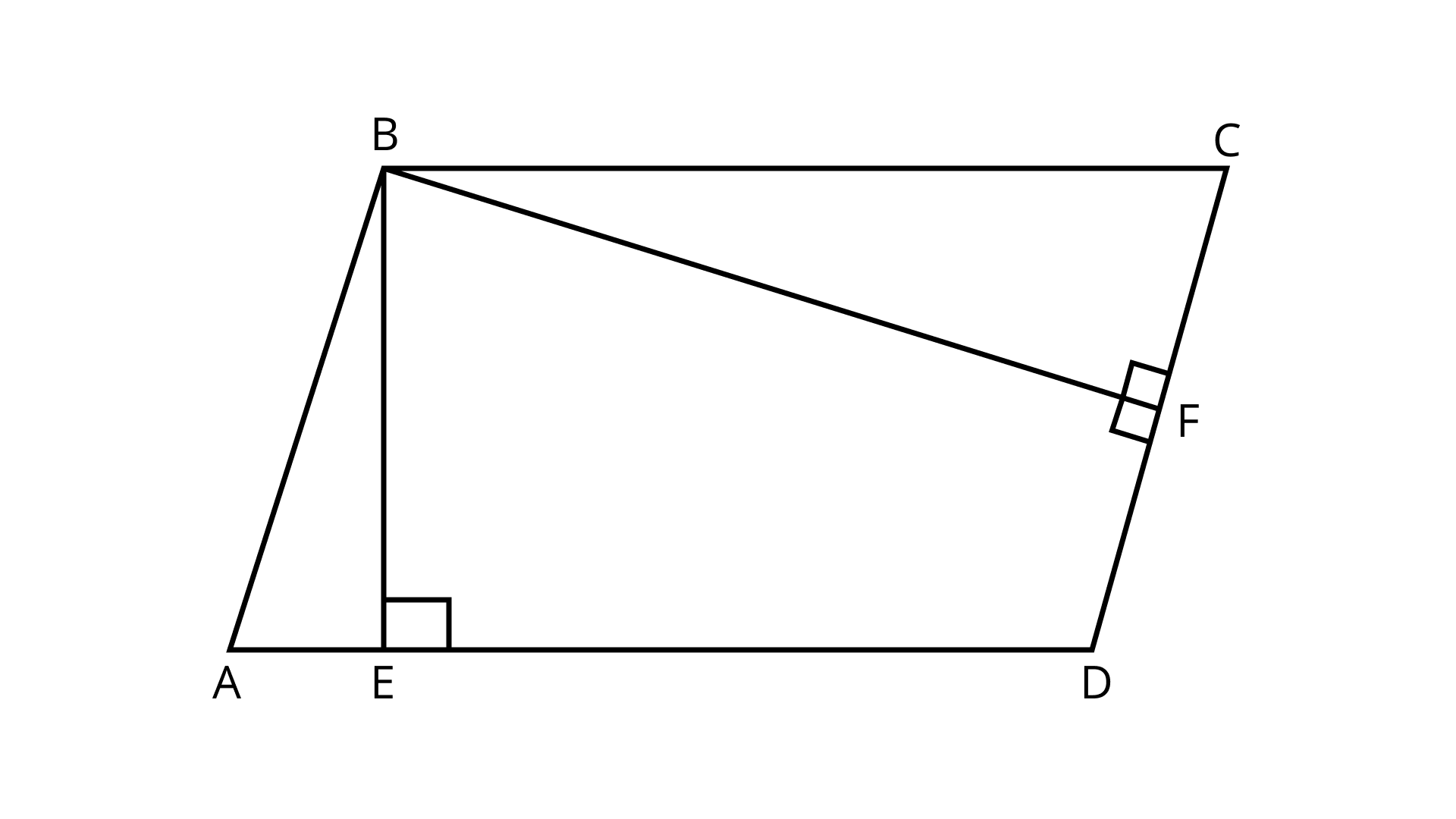
The ratio of shaded part to the leftover area in the rectangle is
Exercise
In the Questions 1 to 37, there are four options, out of which one is correct. Choose the correct one.
1. Observe the shapes

(a) Shapes 1,3 and 4 have different areas and different perimeters.
(b) Shapes 1 and 4 have the same area as well as the same perimeter.
(c) Shapes 1,2 and 4 have the same area.
(d) Shapes 1,3 and 4 have the same perimeter.
Ans: Option (a) is correct.

Perimeter of the shape 1
Therefore, area is
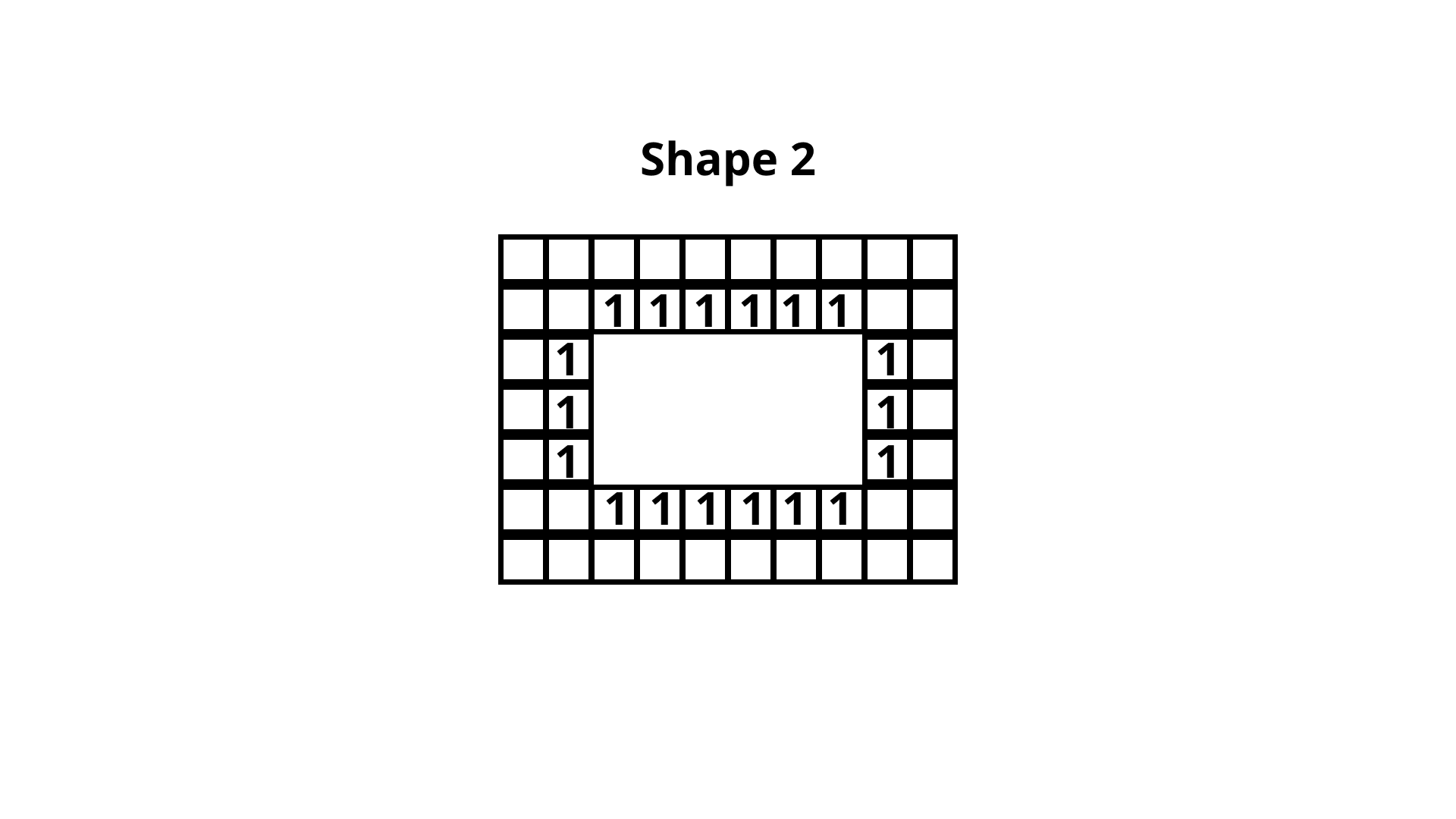
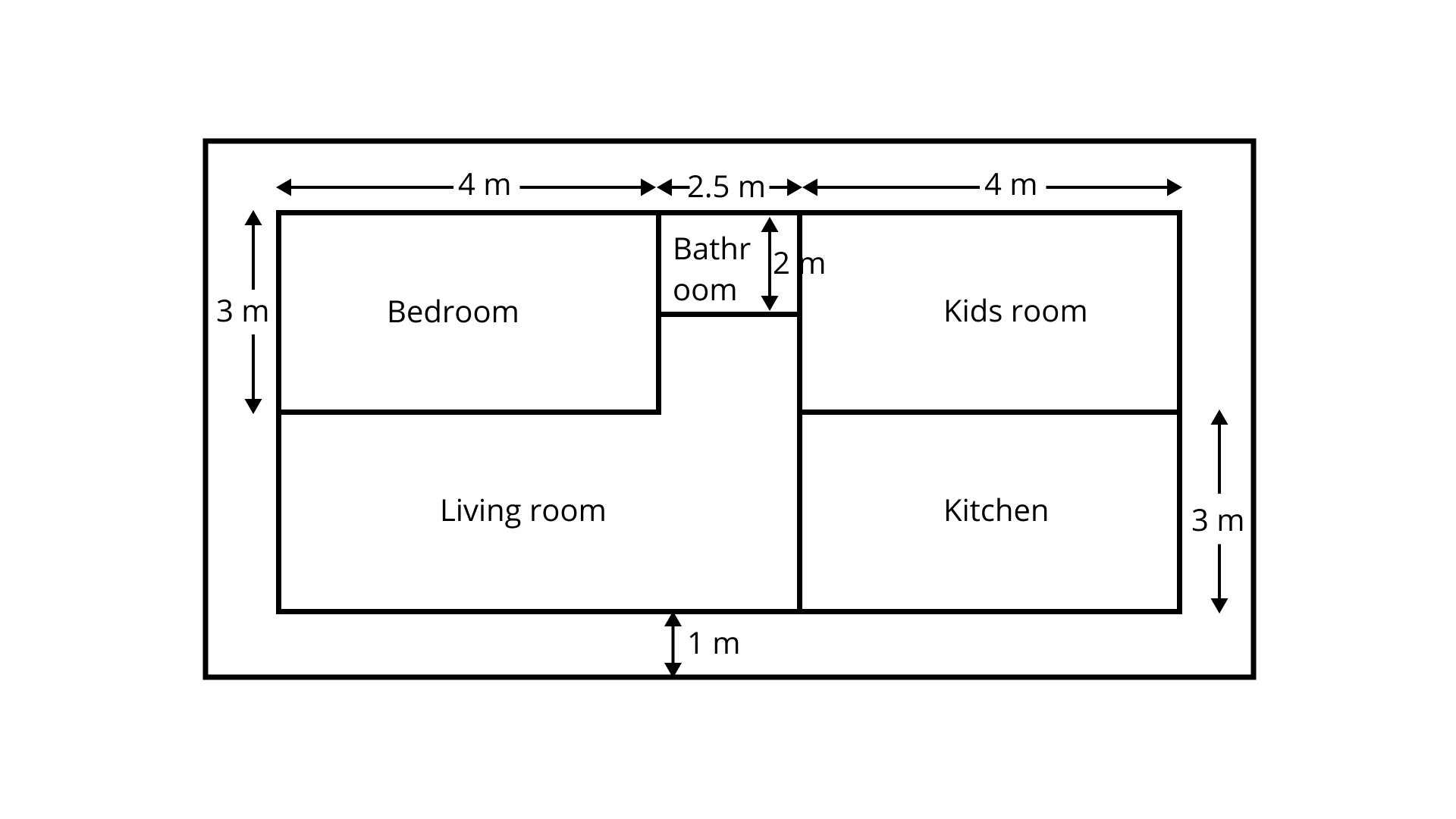
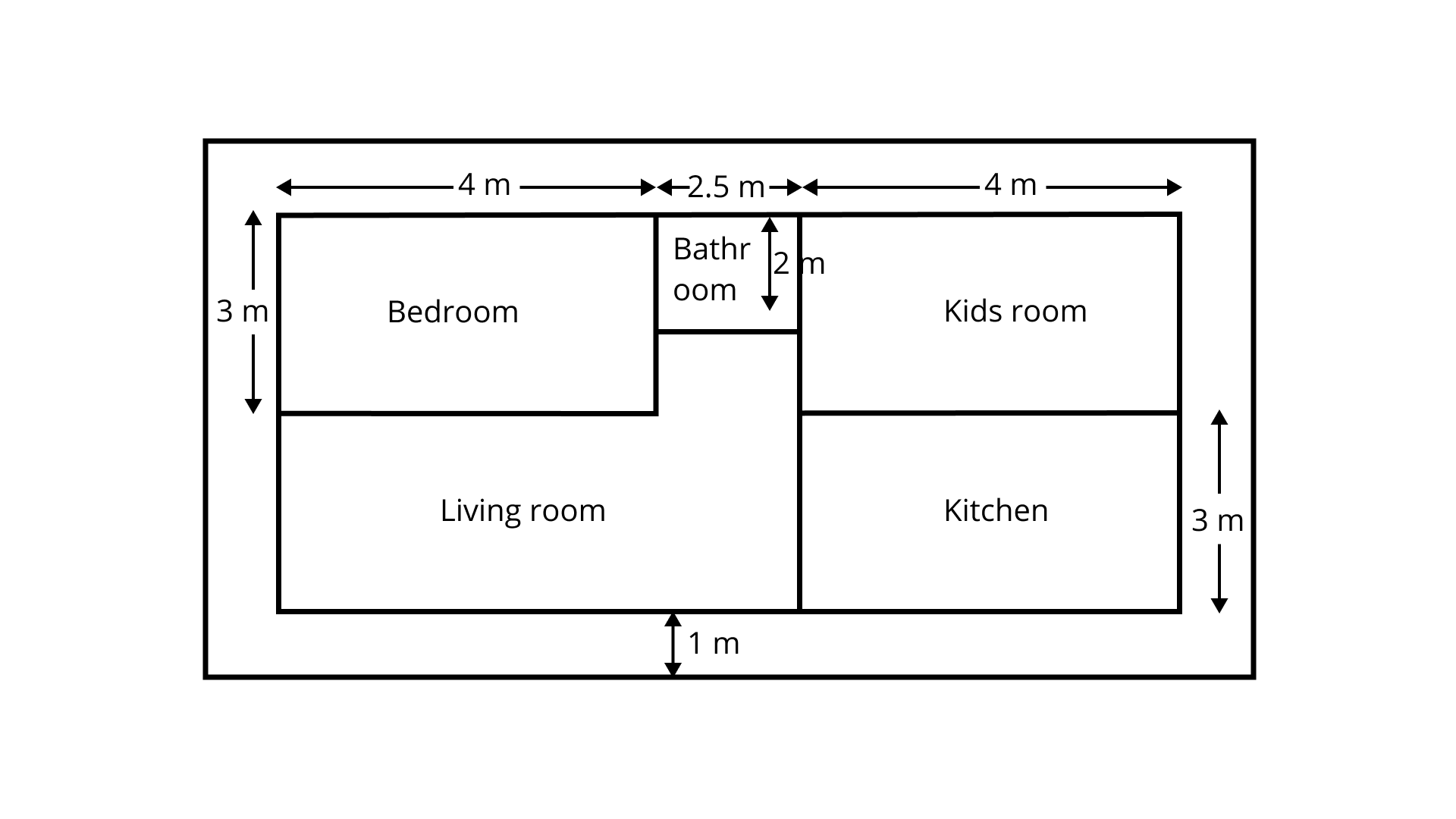
Perimeter of the shape 2
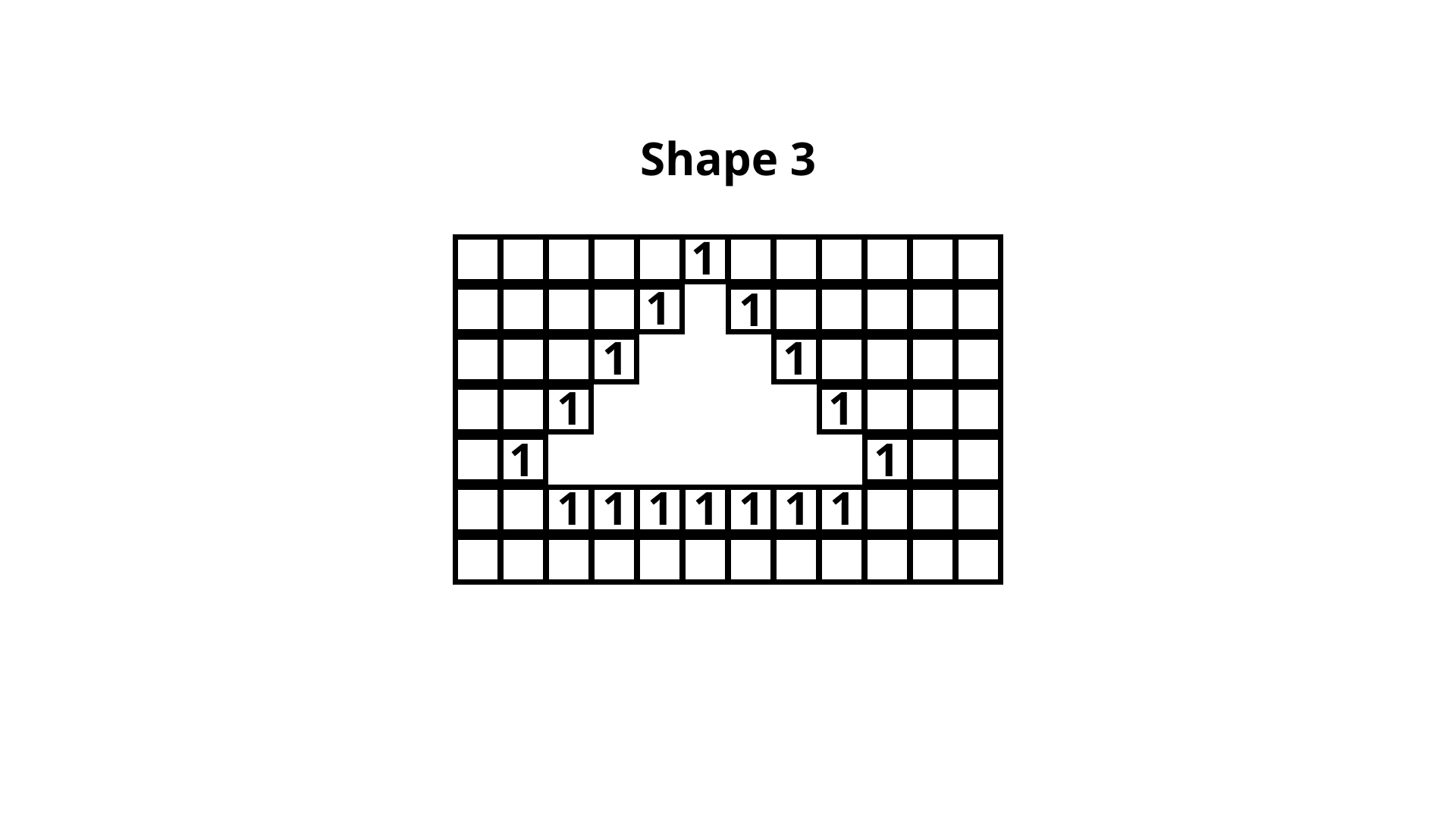
Perimeter of the shape 3
Therefore, area is
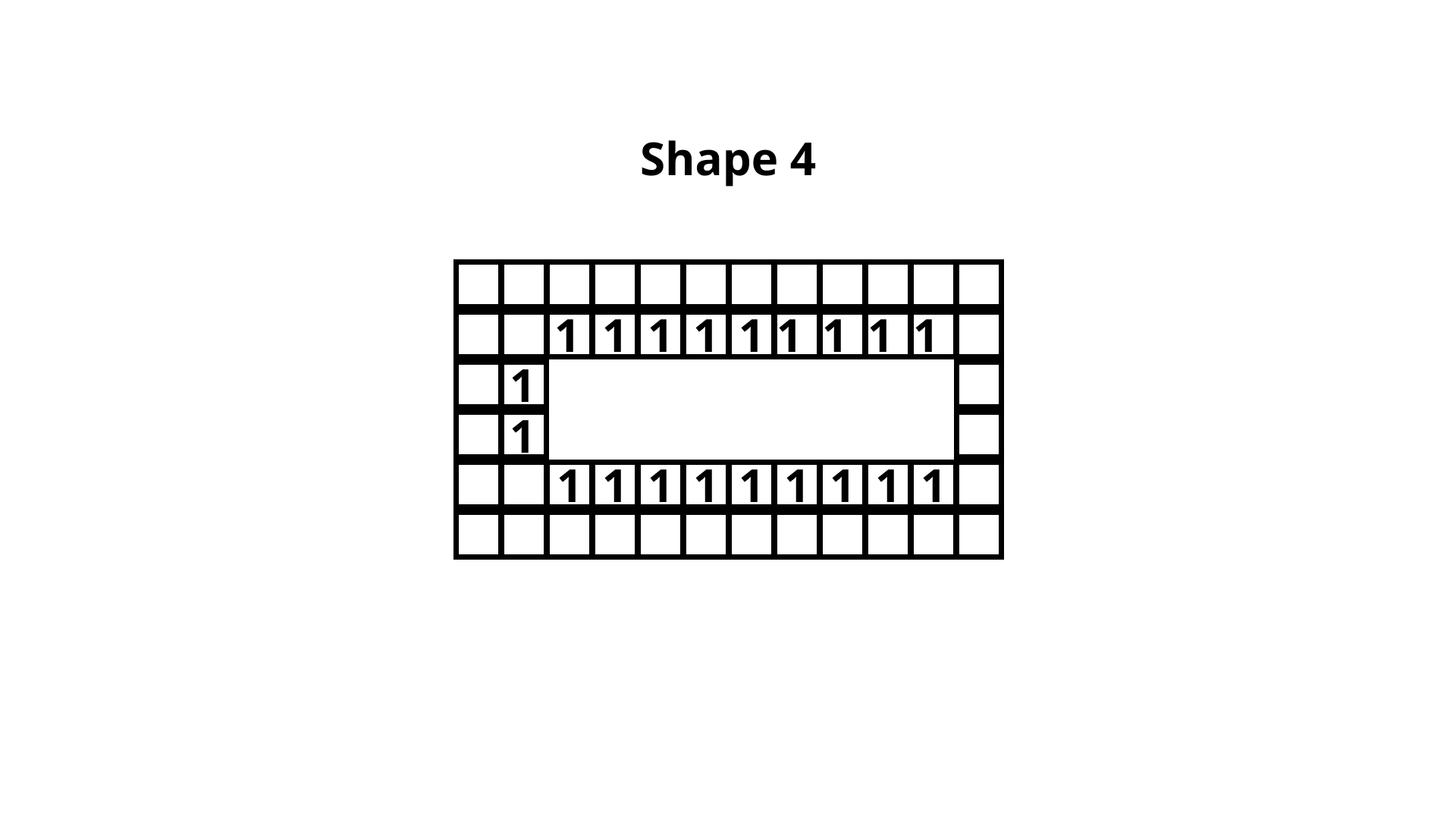
Perimeter of the shape 4
Therefore, area is
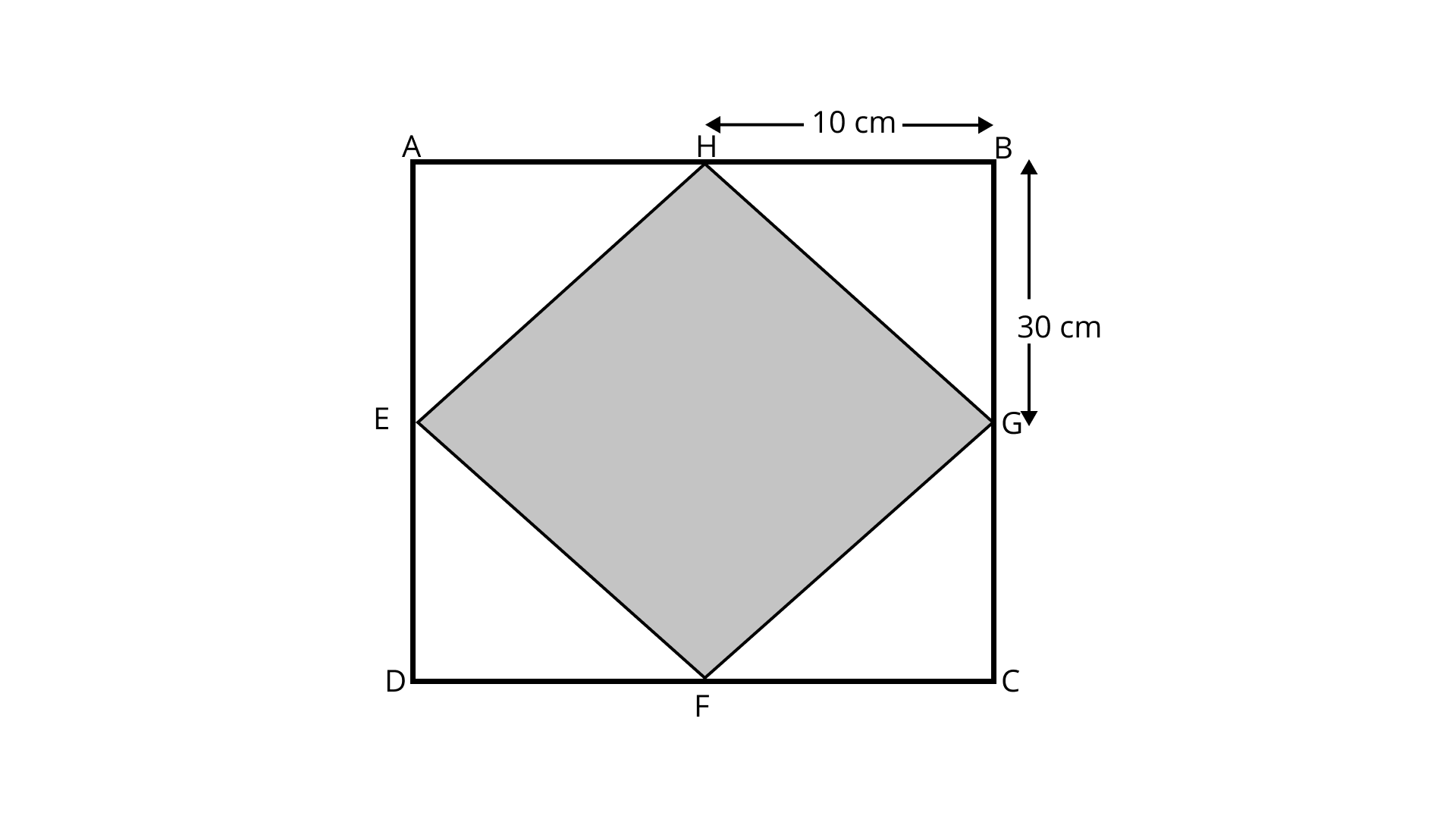
2. A rectangular piece of dimensions
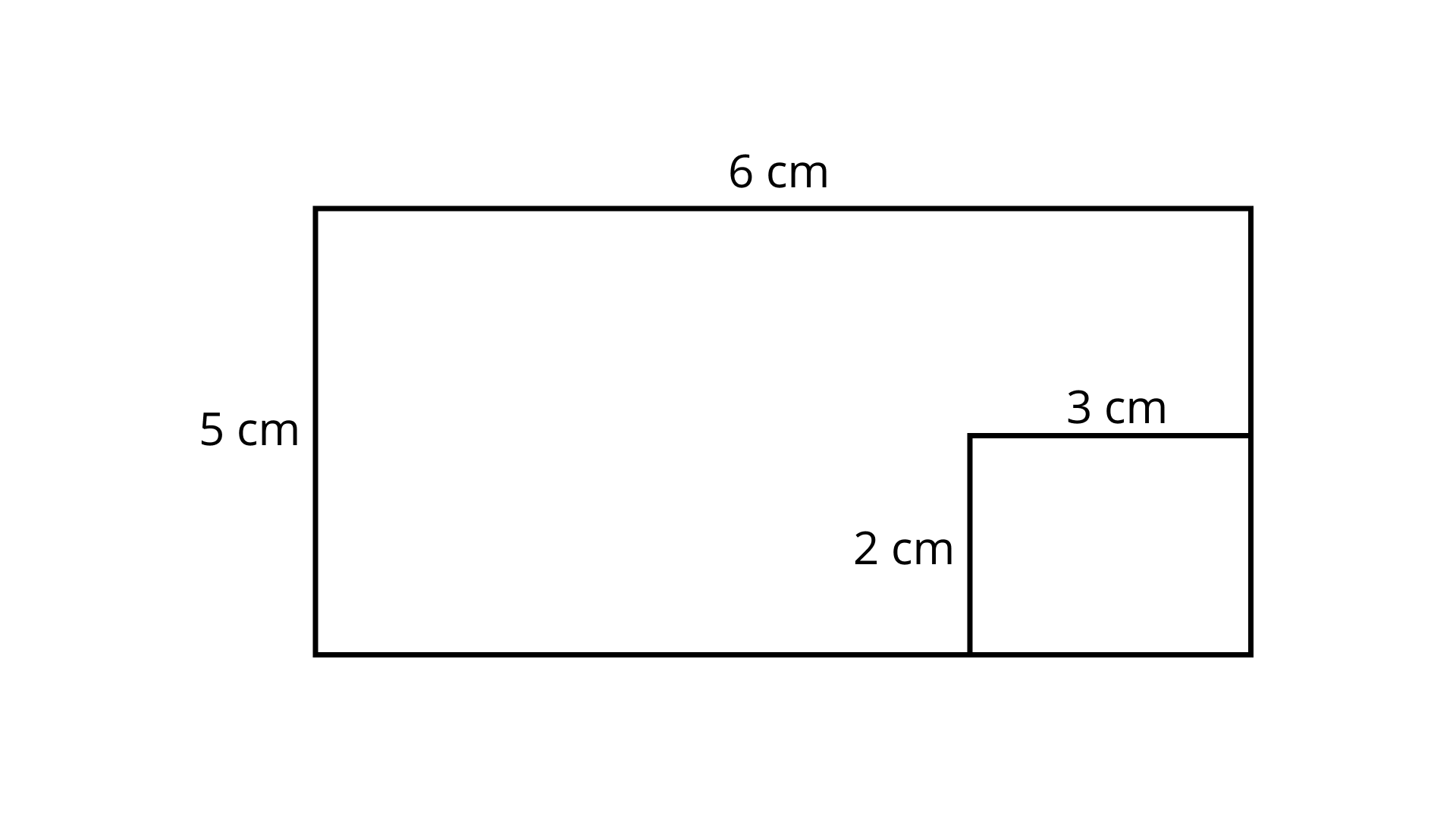
Area of remaining sheet of paper is
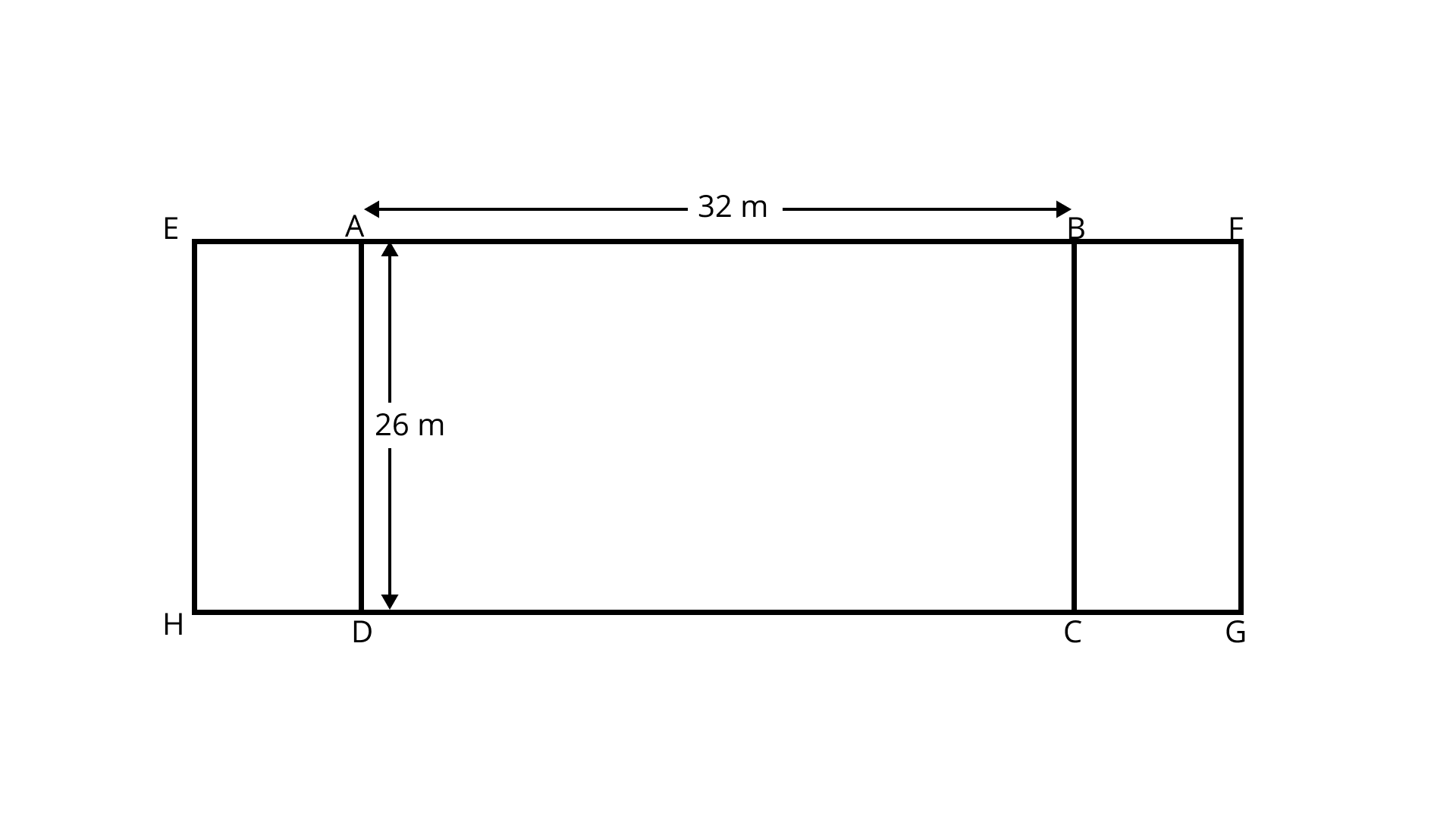
(a)
(b)
(c)
(d)
Ans: Option (c) is correct.
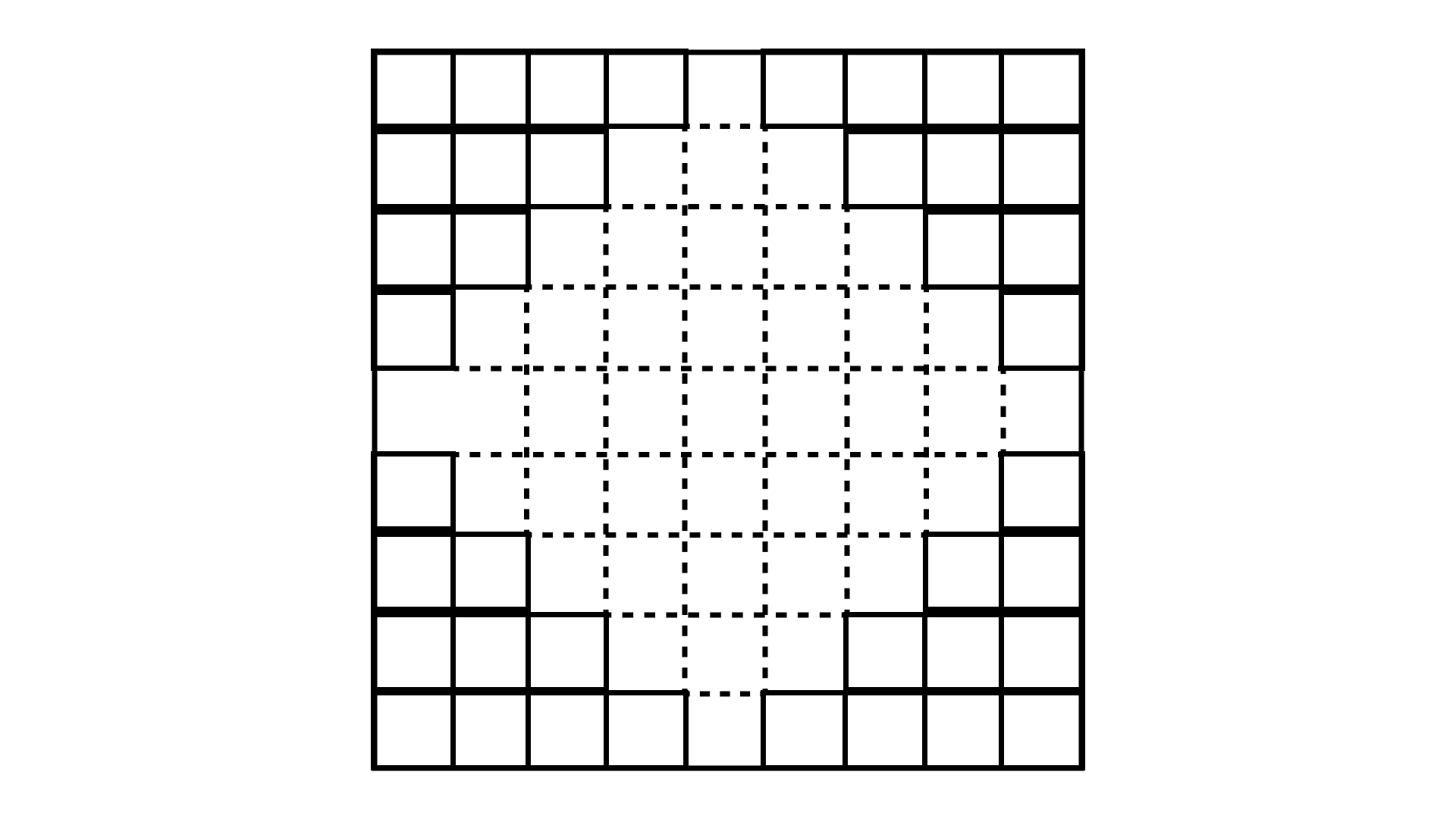
Given,
Area of bigger rectangle
Area of smaller rectangle
So, area of remaining sheet of paper
3.
(a)
(b)
(c)
(d)
Ans: Option (b) is correct.
Area of rectangle is
Therefore, the sides of a rectangle are
Perimeter
4. A wire is bent to form a square of side
(a)
(b)
(c)
(d)
Ans: Option (b) is correct.
Given, side of a square is
The wire has the same length.
Hence, the perimeter of the square and circumference of the circle are equal.
Circumference of circle
So,
5. Area of the circle obtained in Question 4 is
(a)
(b)
(c)
(d)
Solution: Option (c) is correct.
Area of the circle
6. Area of a rectangle and the area of a circle are equal. If the dimensions of the rectangle are
(a)
(b)
(c)
(d)
Ans: Option (d) is correct.
Given,
Length of rectangle
Breadth of rectangle
Area of circle
7. Area of shaded portion in Fig. 9.15 is
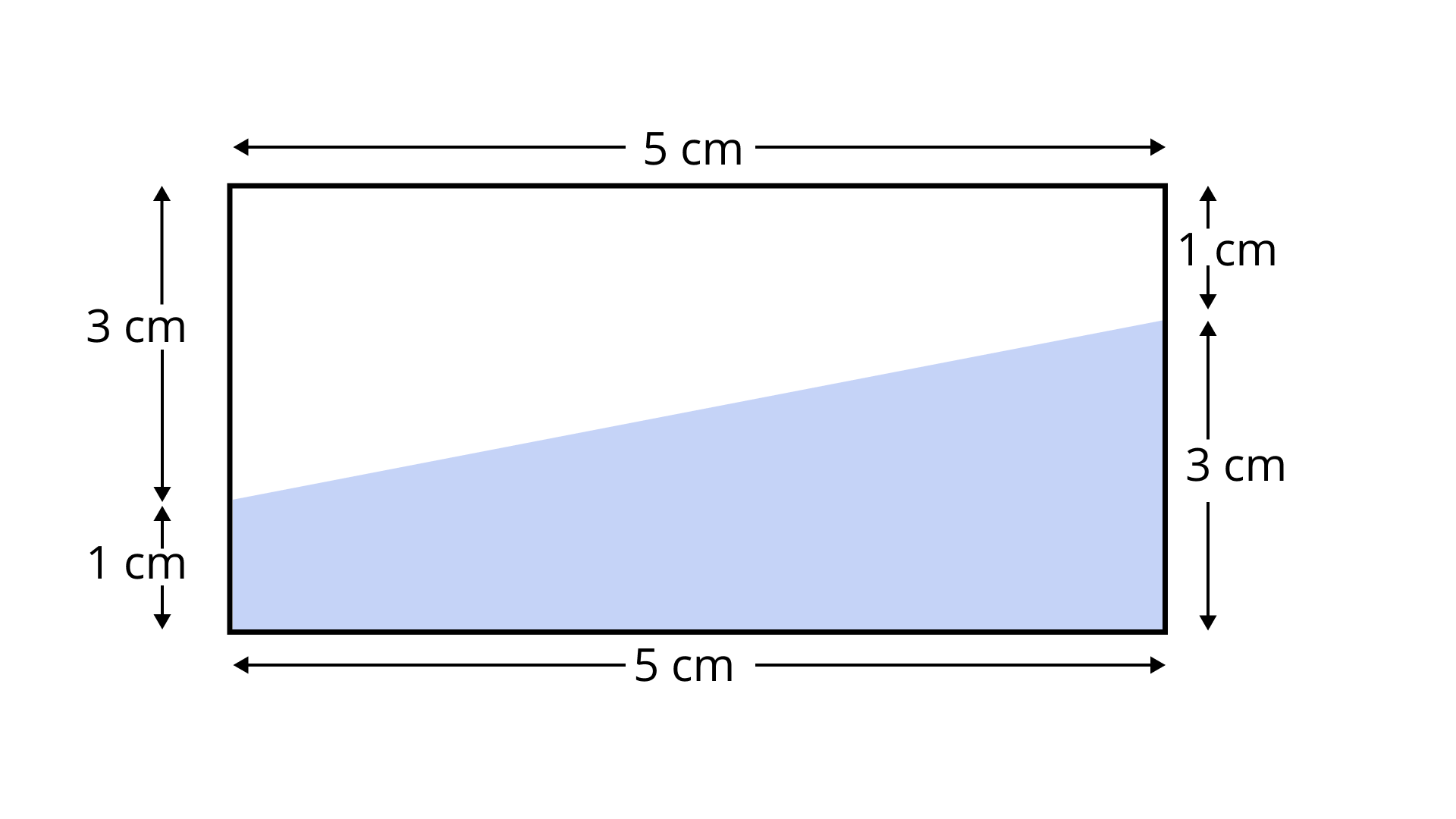
(a)
(b)
(c)
(d)
Ans: Option (d) is correct.
From the given figure,
Length of rectangle
Breadth of rectangle
Thus, area of shaded portion
8. Area of parallelogram
(a)
(b)
(c)
(d)
Ans: Option (a) is correct.
Area of parallelogram = Base
Here, the perpendicular dropped on the side from the opposite vertex is known as height
So, the area of parallelogram
Hence, (a) is the correct answer.
9. Area of triangle MNO of Fig is
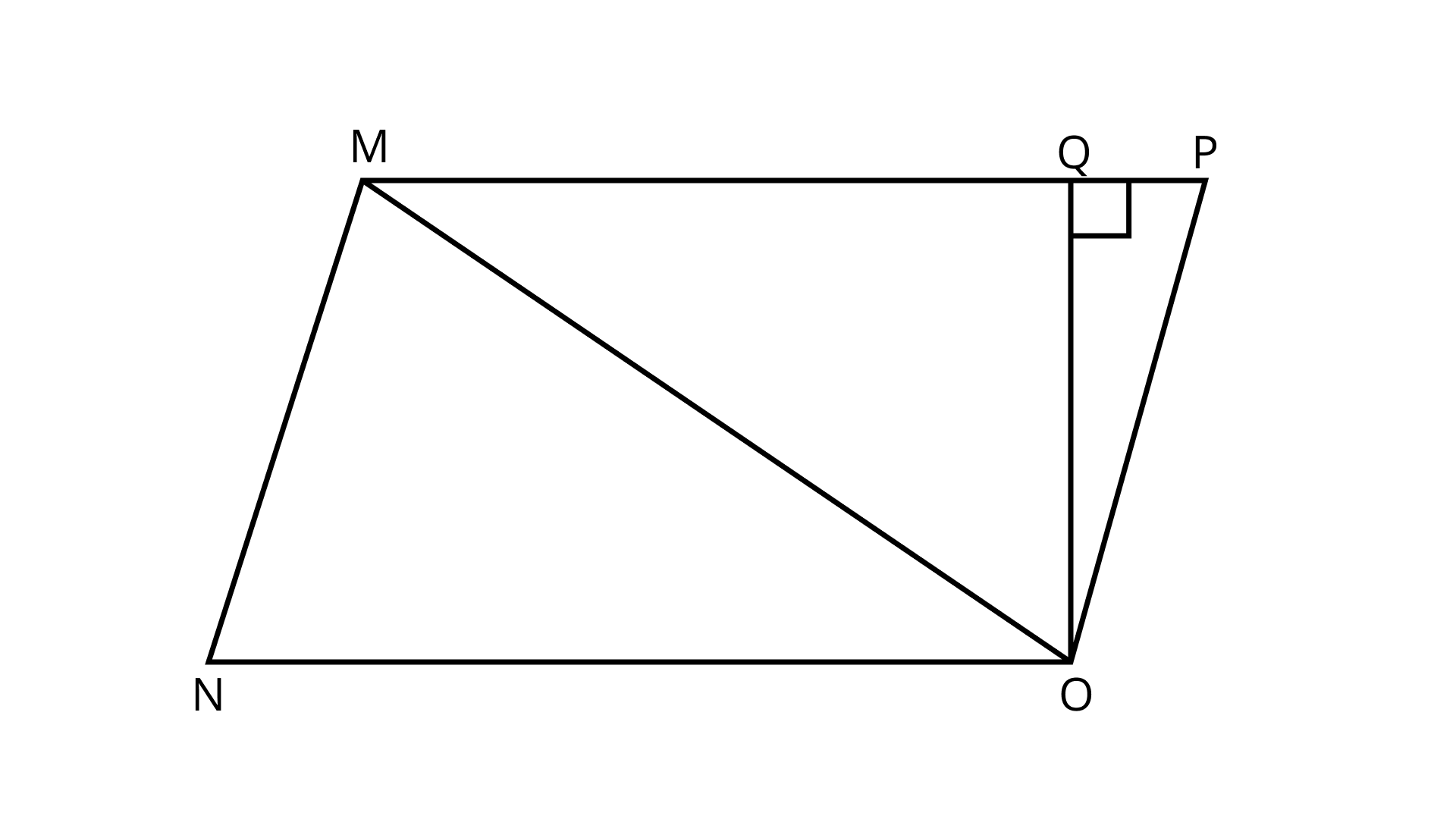
(a)
(b)
(c)
(d)
Ans: Option (d) is correct
As we know that,
Area of triangle
10. Ratio of area of triangle MNO to the area of parallelogram MNOP in the same figure is

(a)
(b)
(c)
(d)
Ans: Option (c) is correct.
Area of triangle MNO: Area of parallelogram MNOP
Therefore,
11. Ratio of areas of
(a)
(b)
(c)
(d)
Ans: Option (a) is correct.
Area of
Area of
Now, Area of
Hence, (a) is the correct answer.
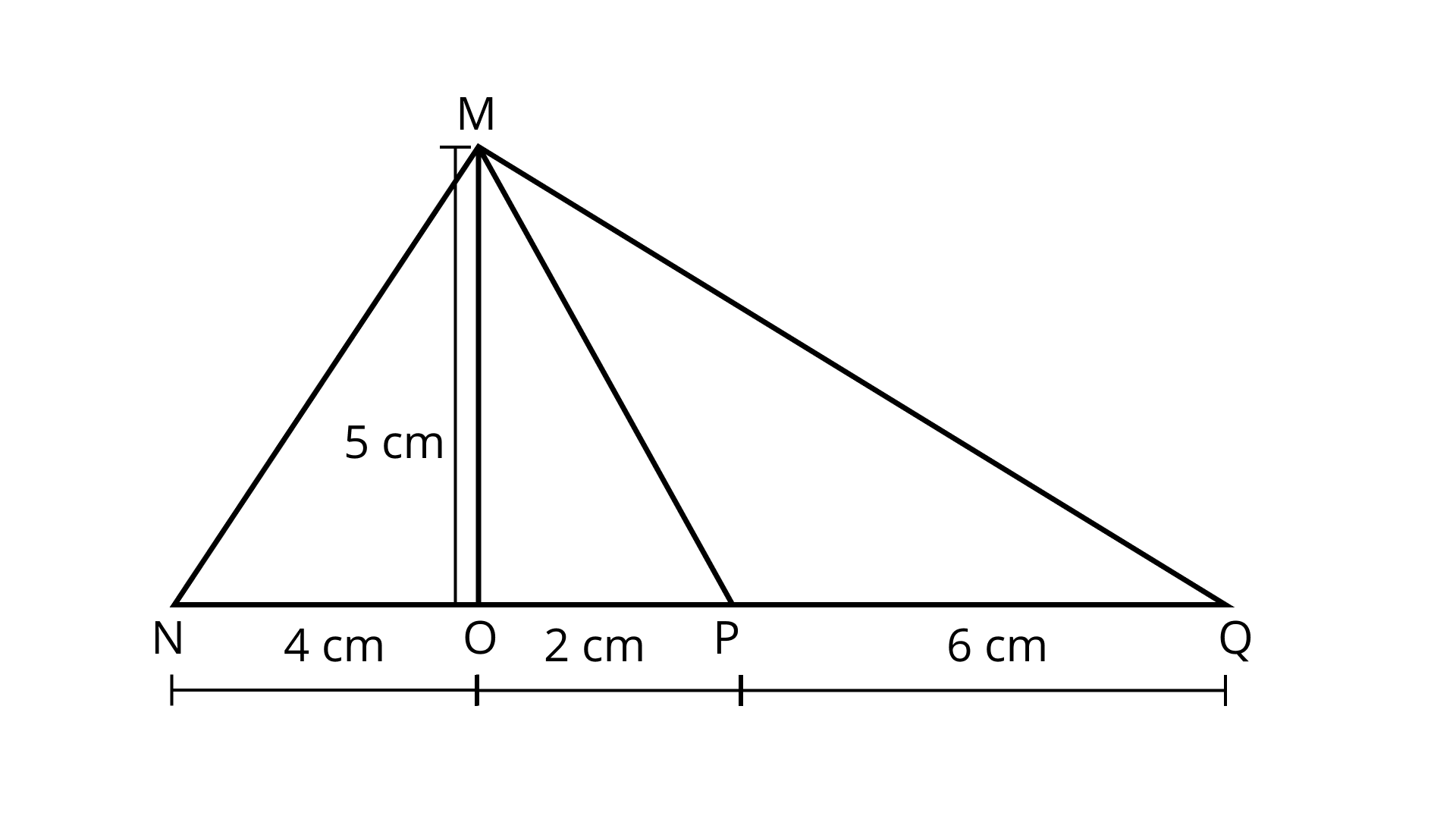
12. In Fig.
(a)
(b)
(c)
(d)
Ans: Option (c) is correct.
Area of parallelogram
13. In reference to a circle the value of
(a)
(b)
(c)
(d)
Ans: Option (c) is correct.
As we know,
Circumference of a circle
Circumference
14. Circumference of a circle is always
(a) more than three times of its diameter
(b) three times of its diameter
(c) less than three times of its diameter
(d) three times of its radius
Ans: Option (a) is correct.
As we know that,
Circumference of a circle
Circumference
Circumference
Therefore, Circumference of a circle is more than three of its diameter.
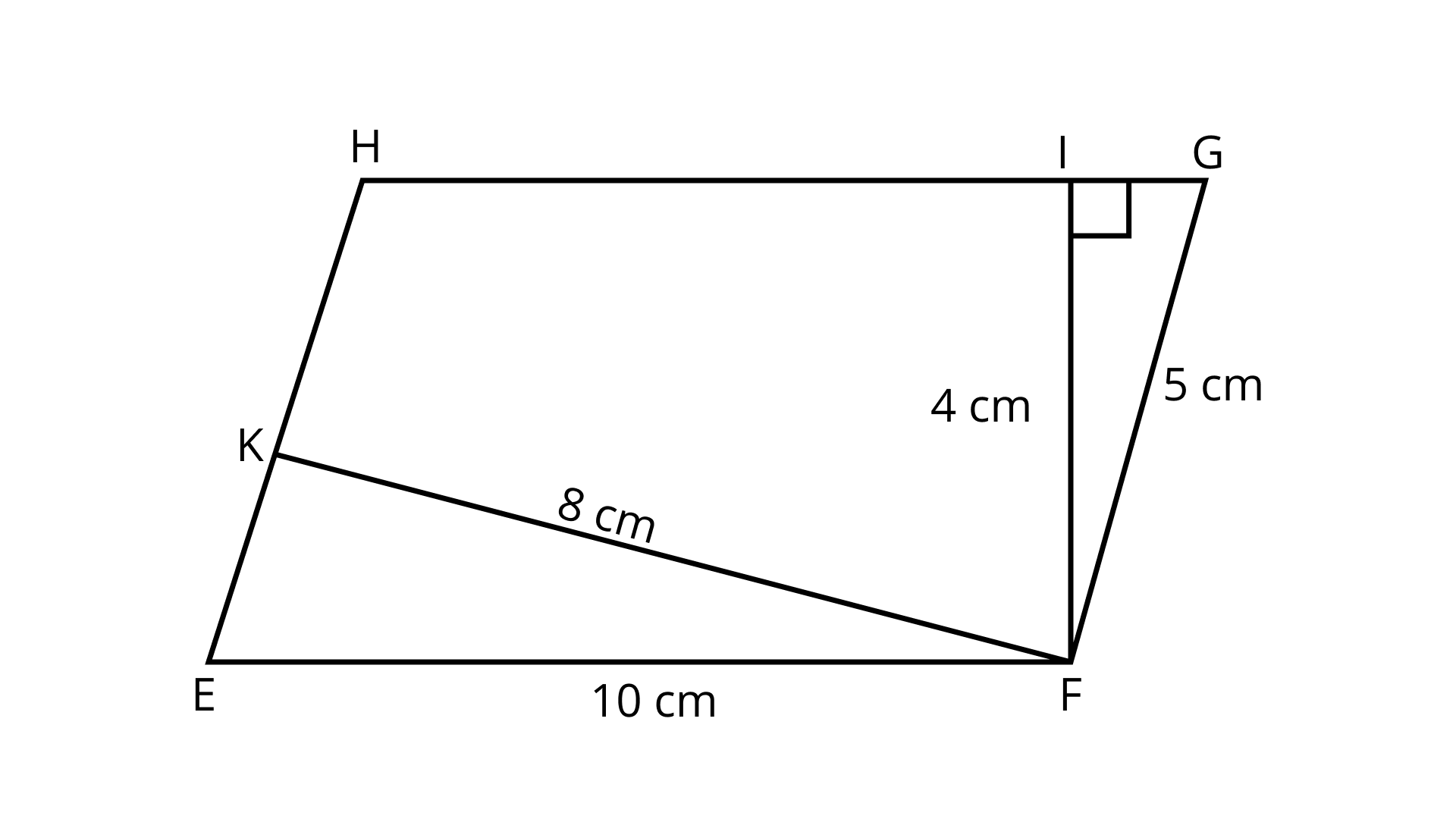
15. Area of triangle
(a)
(b)
(c)
(d)
Ans: Option (a) is correct.
Given, area of triangle
We know that,
Area of triangle
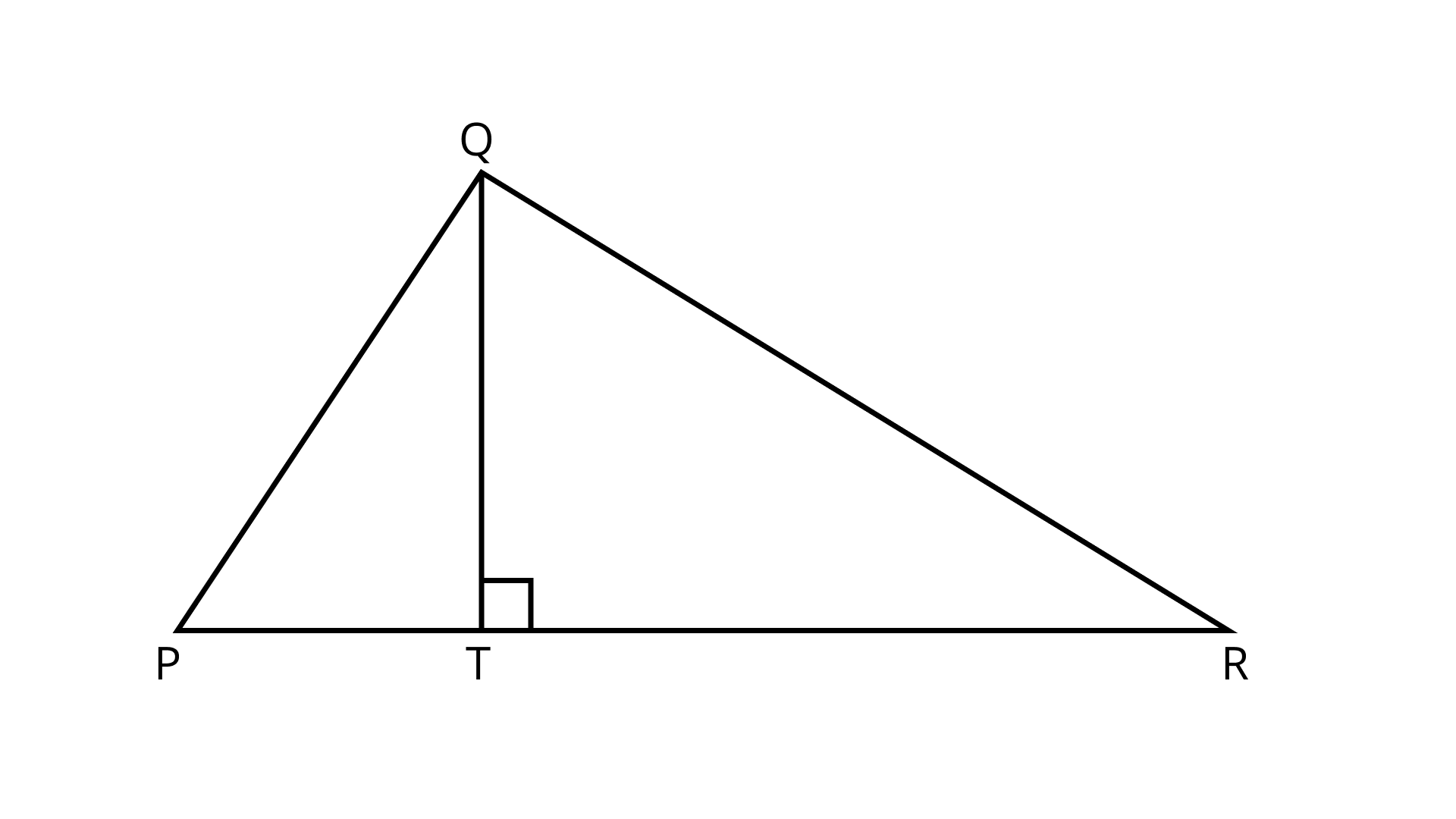
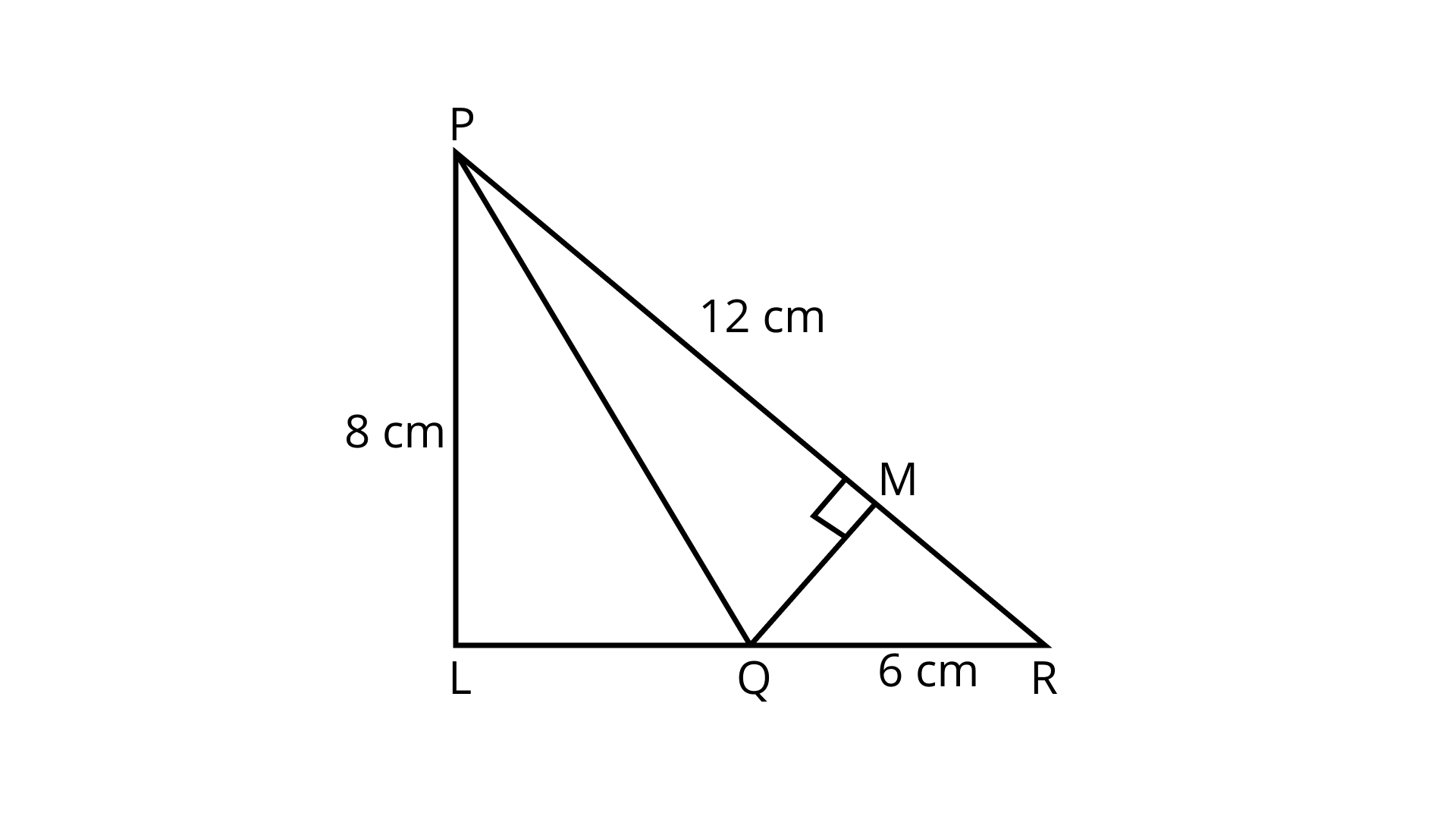
16. In Fig. 9.21, if
(a)
(b)
(c)
(d)
Ans: Option (c) is correct.
Given that,
Now, in right-angled triangle PLR Using Pythagoras theorem,
Now, area of triangle PLR
Now, area of triangle PLQ
Hence, area of triangle
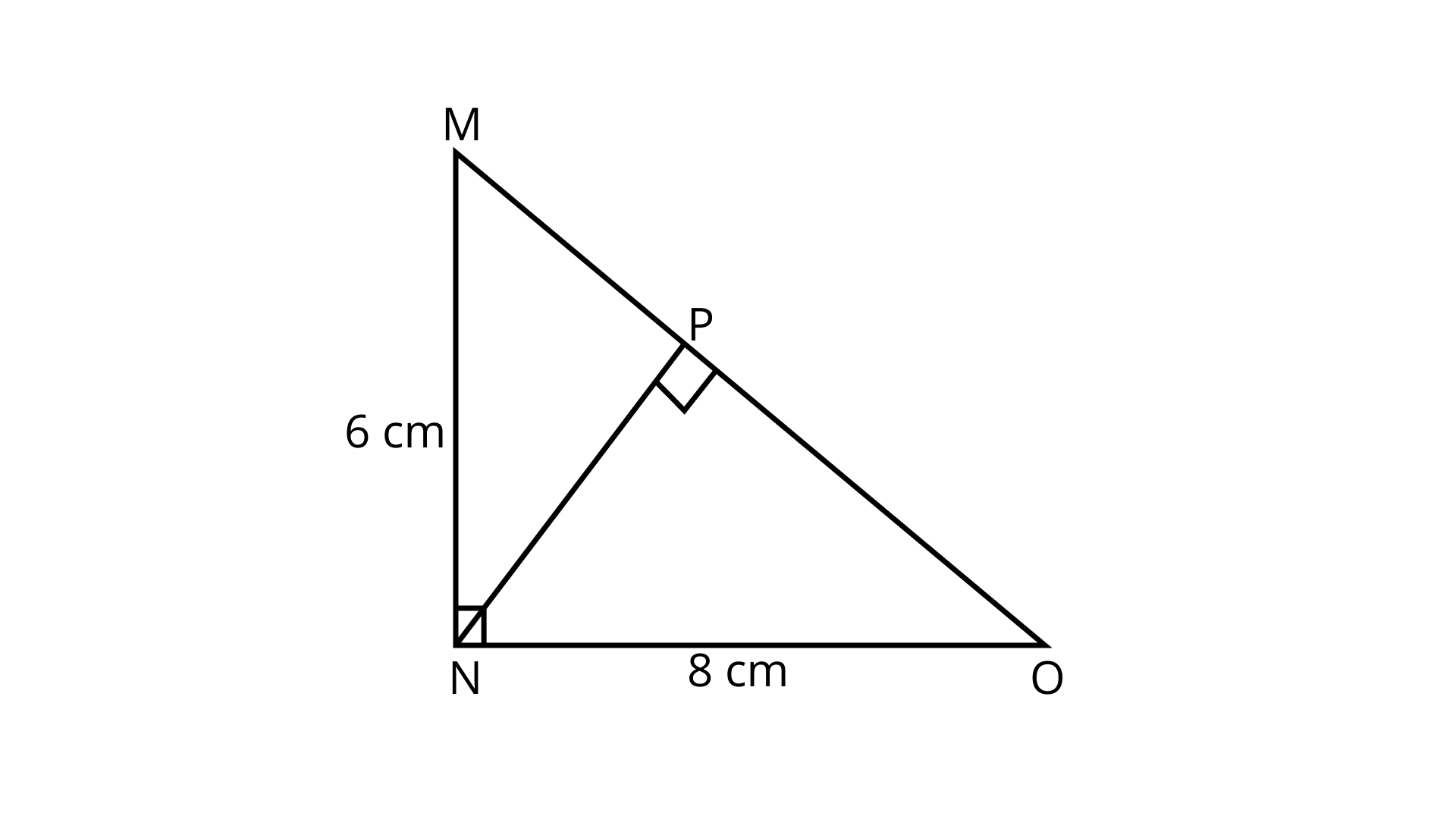
17. In Fig.
(a)
(b)
(c)
(d)
Ans: Option (a) is correct.
Given, triangle MNO is a right- angled triangle.
Area of triangle
18. Area of a right-angled triangle is
(a)
(b)
(c)
(d)
Ans: Option (b) is correct.
Given, area of right angled triangle
Smallest side
We know that,
Area of triangle
Height
According to the Pythagoras theorem,
Hypotenuse
19. Circumference of a circle of diameter
(a)
(b)
(c)
(d)
Ans: Option (c) is correct.
Given,
Diameter
Therefore, Radius
Since, circumference
20. Circumference of a circle disc is
(a)
(b)
(c)
(d)
Ans: Option (c) is correct.
Circumference
Hence,
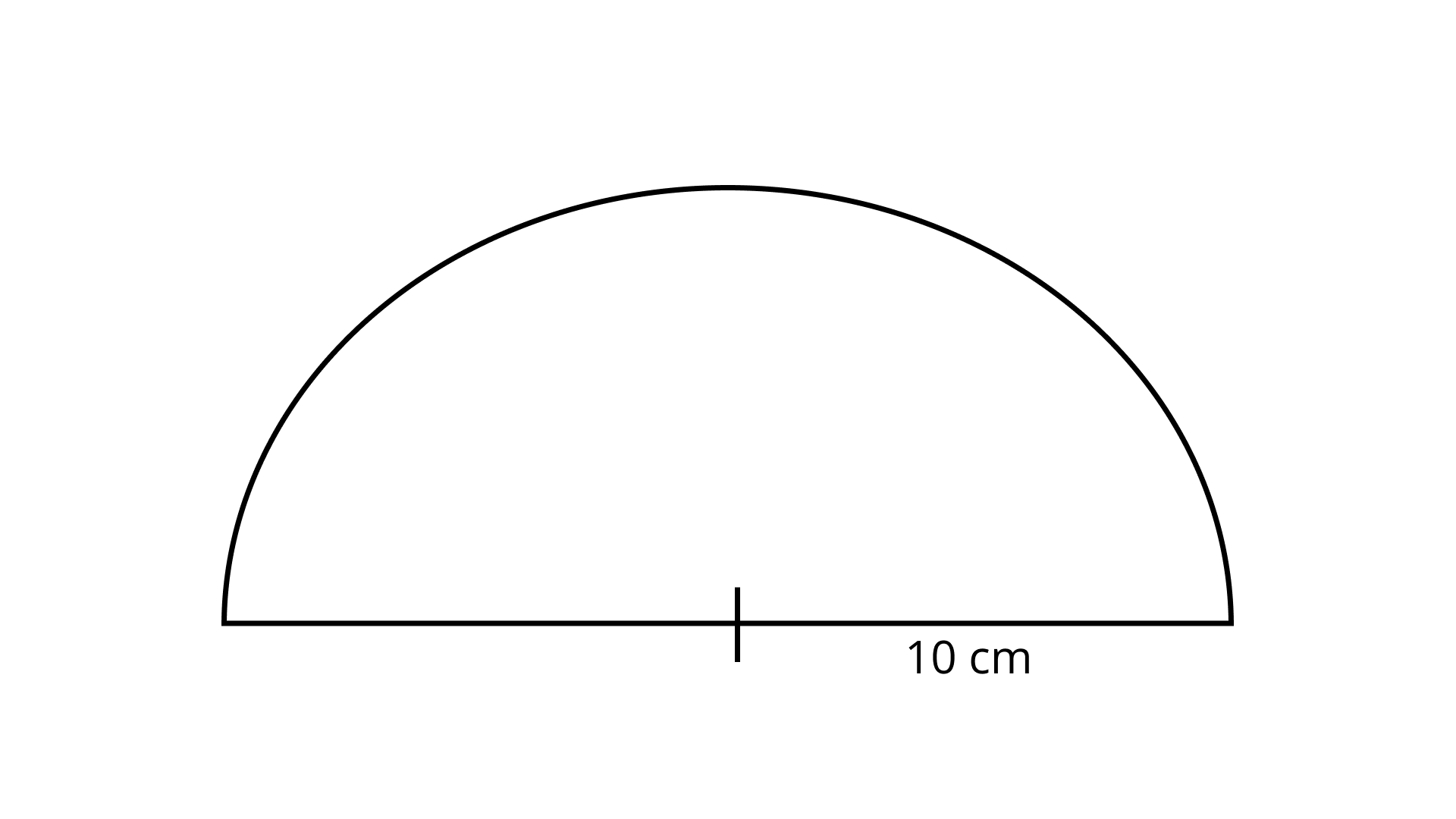
21. Length of tape required to cover the edges of a semicircular disc of radius
(a)
(b)
(c)
(d)
Ans: Option (b) is correct.
Perimeter of the semi-circle
circumference of semi-circle
Therefore, total tape required
22. Area of circular garden with diameter
(a)
(b)
(c)
(d)
Ans: Option (a) is correct.
Given, diameter is
So, radius
Hence, area of the circular garden
23. Area of a circle with diameter '
(a)
(b)
(c)
(d)
Ans: Option (d) is correct.
Given,
Diameter
Radius
Circumference
Hence, area of circle
24. A table top is semicircular in shape with diameter
(a)
(b)
(c)
(d)
Ans: Option (a) is correct.
Given,
Diameter
Radius
Hence,
Area of table top
25. If
(a)
(b)
(c)
(d)
Ans: Option (d) is correct.
Given,
26. If
(a)
(b)
(c)
(d)
Ans: Option (b) is correct.
Since, area of one square having side of
Area of the square having side of
27.
(a) a square with side
(b)
(c)
(d)
Ans: Option (b) is correct.
According to the option (b)
Area of square
28. If each side of a rhombus is doubled, how much will its area increase?
(a)
(b)
(c)
(d)
Ans: Option c is correct.
Let the side of rhombus be
New side of rhombus
Area of rhombus
New area of rhombus
Hence increase in the area of rhombus
Hence the increase in the area is 3 times the original area.
29. If the sides of a parallelogram are increased to twice its original lengths, how much will the perimeter of the new parallelogram?
(a)
(b)
(c)
(d)
Ans: Option (b) is correct.
Let 1 be the length and
Then, perimeter
If both sides of the parallelogram are doubled, then the breadth and length become 2l and
Now, perimeter
Therefore, the perimeter of the parallelogram will be increased by 2 times.
30. If the radius of a circle is increased to twice its original length, how much will the area of the circle increase?
(a)
(b)
(c)
(d)
Ans: Option (d) is correct.
Let
Then, area of circle
If radii are doubled, then radii become
Now, area of circle
The area of the circle has increased by 4 times.
31. What will be the area of the largest square that can be cut out of a circle of radius
(a)
(b)
(c)
(d)
Ans: Option (b) is correct.
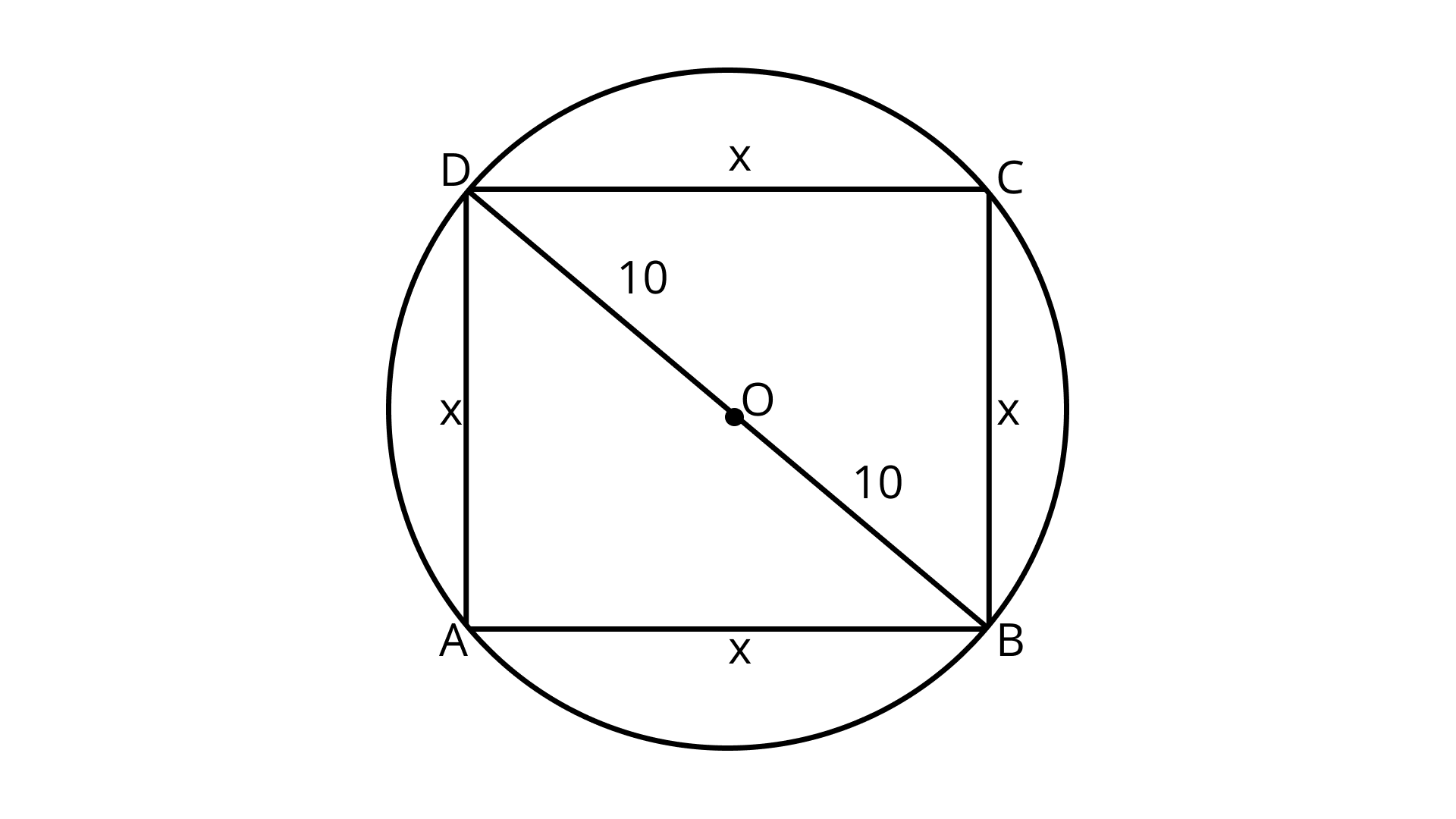
Given, radius of circle
The diagonal of the square will be equal to the diameter of the circle.
Now, in right-angled triangle
So,
32. What is the radius of the largest circle that can be cut out of the rectangle measuring
(a)
(b)
(c)
(d)
Ans: Option (a) is correct
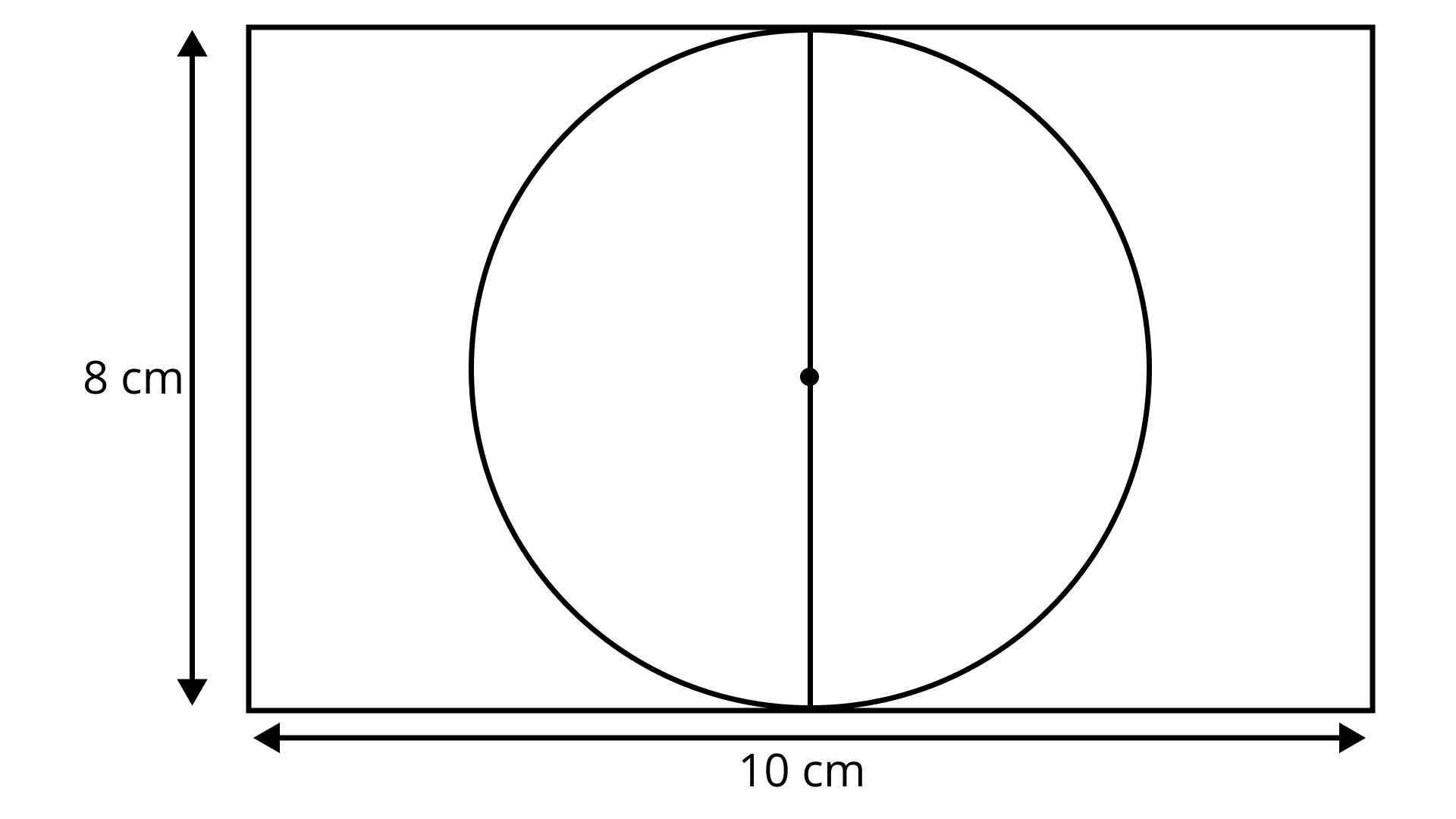
It is clear that the largest circle will have diameter equals the smaller side that is
Hence, Radius
33. The perimeter of the figure
(a)
(b)
(c)
(d)
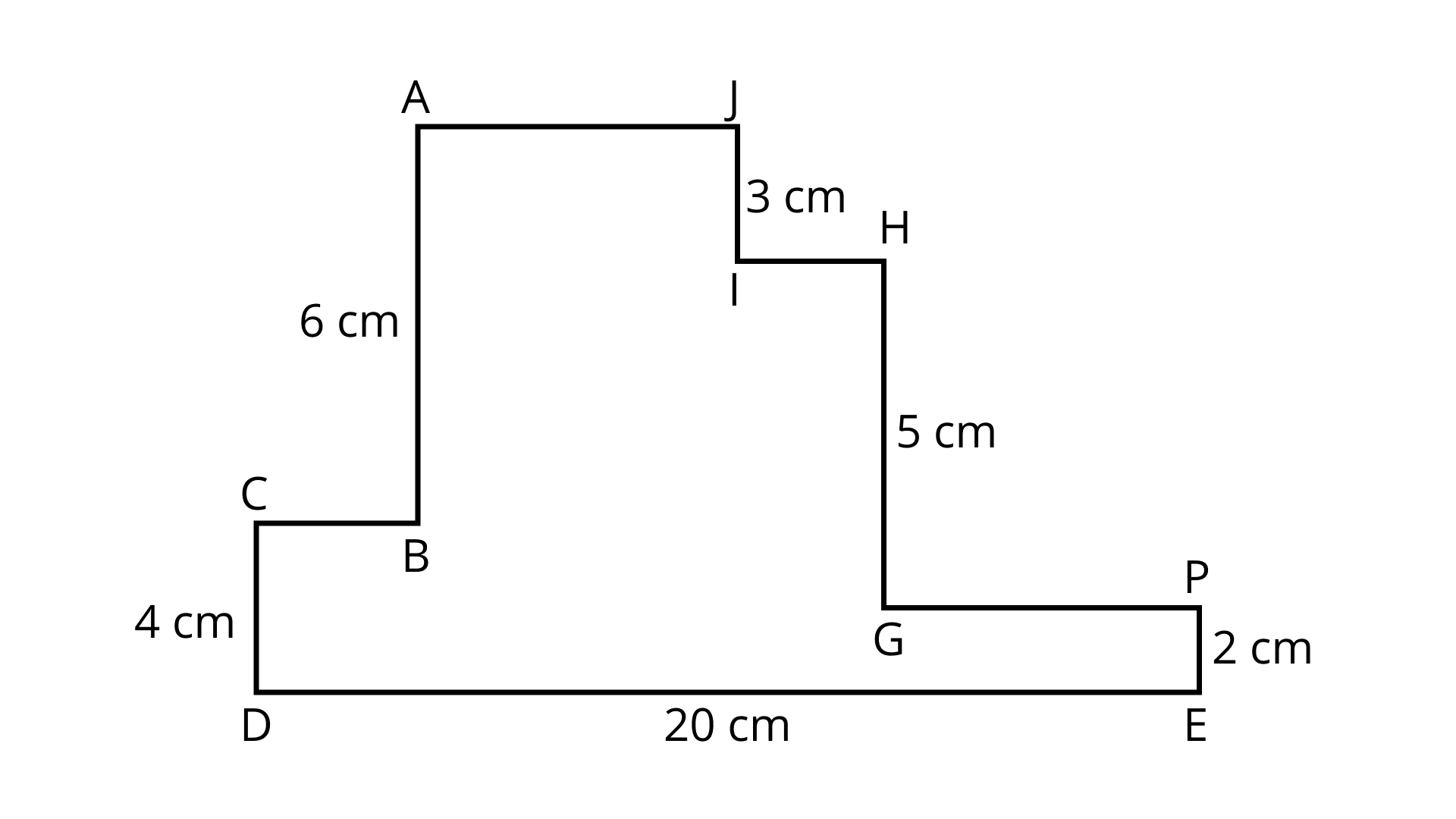
Ans: Option (a) is correct.
Sum of all side
34. The circumference of a circle whose area is
(a)
(b)
(c)
(d)
Ans: Option (a) is correct.
Given, area of the circle
Hence, radius is
Circumference of a circle
Therefore, the circumference of the circle is
35. The area of a square is
(a)
(b)
(c)
(d)
Ans: Option (a) is correct.
Let a be the side of the square.
Given, area of square
Now, for the largest circle in the square,
Hence, side of a square
Therefore, circumference of the circle
36. If the radius of a circle is tripled, the area becomes
(a)
(b)
(c)
(d)
Ans: Option (a) is correct.
The area of a circle with radius r is
So, if the radius is tripled, the new radius is 3r
The new area is
Hence, if the radius of a circle is tripled, the area becomes nine times.
37. The area of a semicircle of radius
(a)
(b)
(c)
(d)
Ans: Option (a) is correct.
Area of semicircle
Then, the area of a semicircle of radius
In Questions 38 To 56, Fill in the Blanks to Make the Statements True.
38. Perimeter of a regular polygon
Ans: Perimeter of a regular polygon
39. If a wire in the shape of a square is rebent into a rectangle, then the_____of both shapes remain same, but_____ may vary.
Ans: If a wire in the shape of a square is rebent into a rectangle, then the Perimeter of both shapes remain same, but area may vary.
40. Area of the square MNOP of Fig. 9.24 is

Ans: Given, Area of the square MNOP is
There are 8 triangles in the given squares MNOP
Hence, area of each triangle
Area of each triangle is
41. In Fig. 9.25, area of parallelogram BCEF is ___
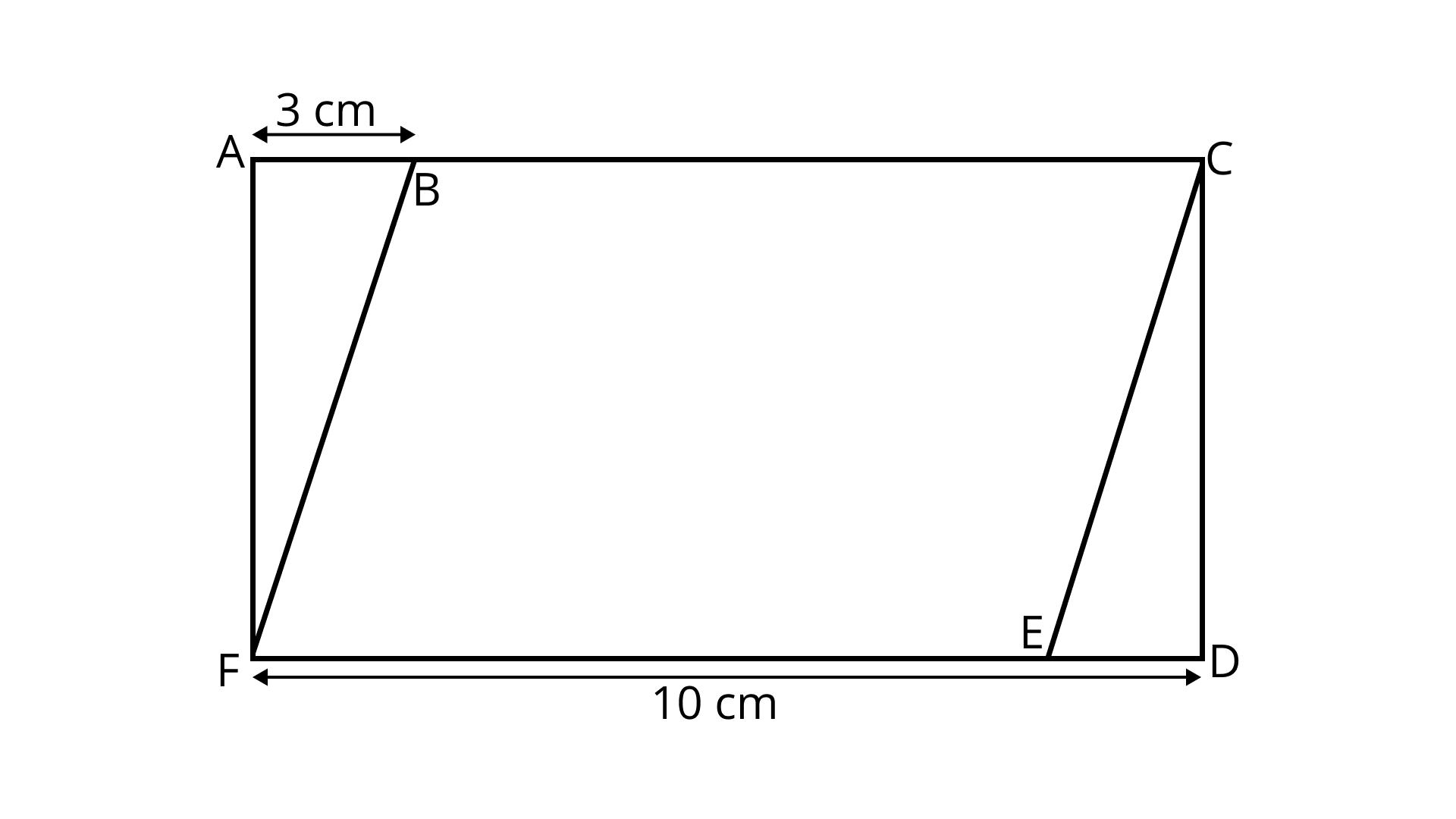
Ans: Area of parallelogram
Area of parallelogram BCEF is
42. To find an area, any side of a parallelogram can be chosen as____ of the parallelogram.
Ans: To find an area, any side of a parallelogram can be chosen as Base of the parallelogram.
43. Perpendicular dropped on the base of a parallelogram from the opposite vertex is known as the corresponding____ of the base.
Ans: Perpendicular dropped on the base of a parallelogram from the opposite vertex is known as the corresponding Height of the base.
44. The distance around a circle is its___
Ans: The distance around a circle is its Circumference
45. Ratio of the circumference of a circle to its diameter is denoted by symbol___
Ans: Circumference
Ratio of the circumference of a circle to its diameter is denoted by symbol
46. If area of a triangular piece of cardboard is
Ans: Area of triangle
If the area of a triangular piece of cardboard is
47. Value of
Ans:
Value of
48. Circumference '
Ans: Circumference
diameter
Therefore,
Circumference '
49. Circumference '
Ans: radius
Circumference '
50.
Ans:
51.
Ans:
52. 1 hectare
Ans: 1 hectare
53. Area of a triangle
Ans: Area of triangle
54.
Ans:
55. Area of a square of side
Ans: Area of side
56.
Ans:
In Questions 57 to 72, state whether the statements are True or False.
57. In Fig. 9.26, the perimeter of (ii) is greater than that of (i), but its area is smaller than that of (i).

Ans: The statement is True
Perimeter of the figure (ii) is greater than that of figure (i) because some part is being cut and that part has created a dip in the figure. So the perimeter is increased because now we have to add the height of the dip along with the perimeter of the figure (i).
58. In Fig. 9.27,
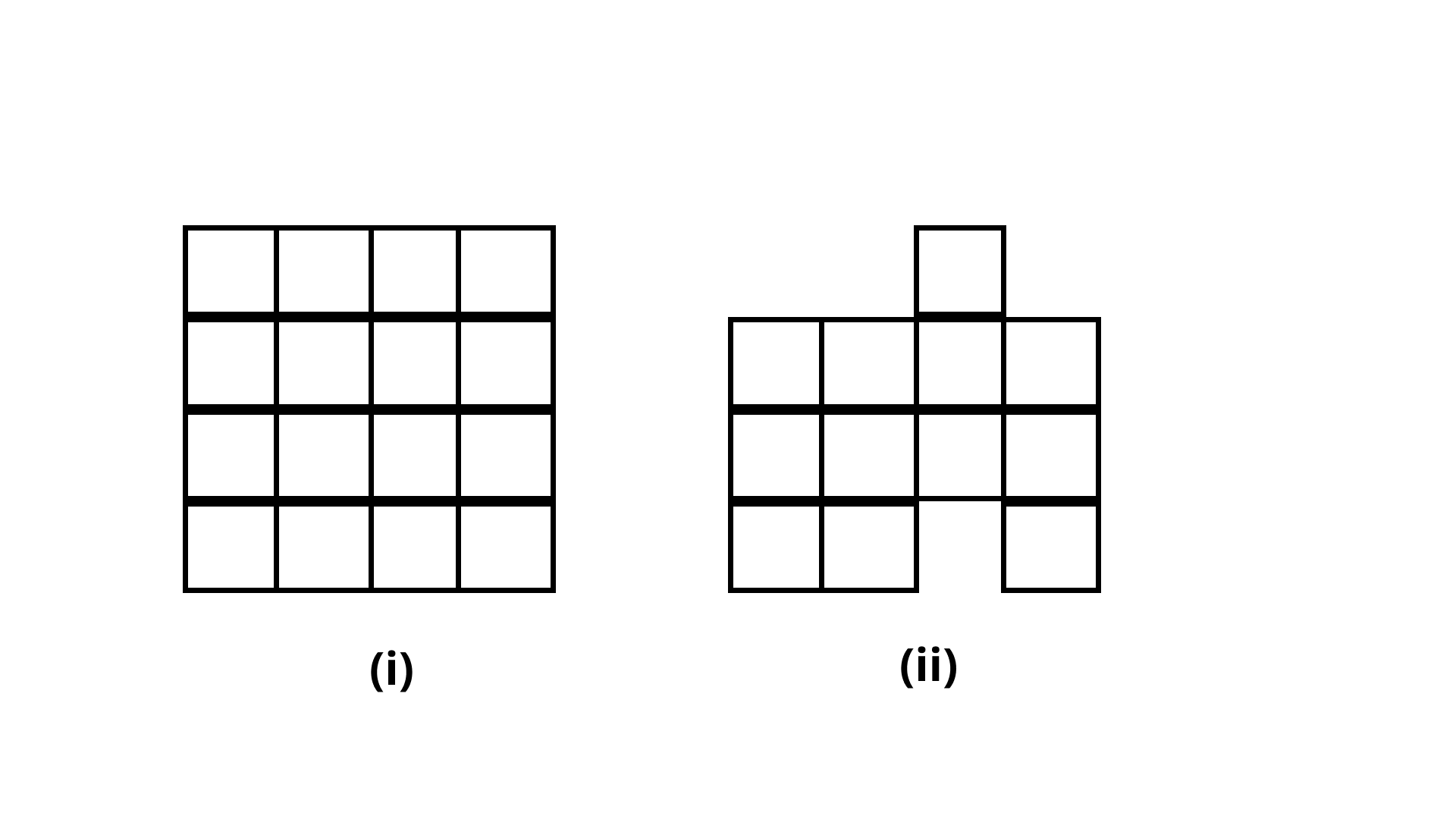
(a) area of (i) is the same as the area of (ii).
Ans: The statement is True
In the given figure, the numbers of blocks are the same.
Therefore, the area of both figures is the same.
(b) Perimeter of (ii) is the same as (i).
Ans: The statement is False
Two new sides are added in figure (ii). Hence, the perimeter of figure (ii) is greater than figure (i).
(c) If (ii) is divided into squares of unit length, then its area is
Ans: The statement is False
Area of 1 squares
Number of squares
Therefore, total area
(d) Perimeter of (ii) is
Ans: The statement is True
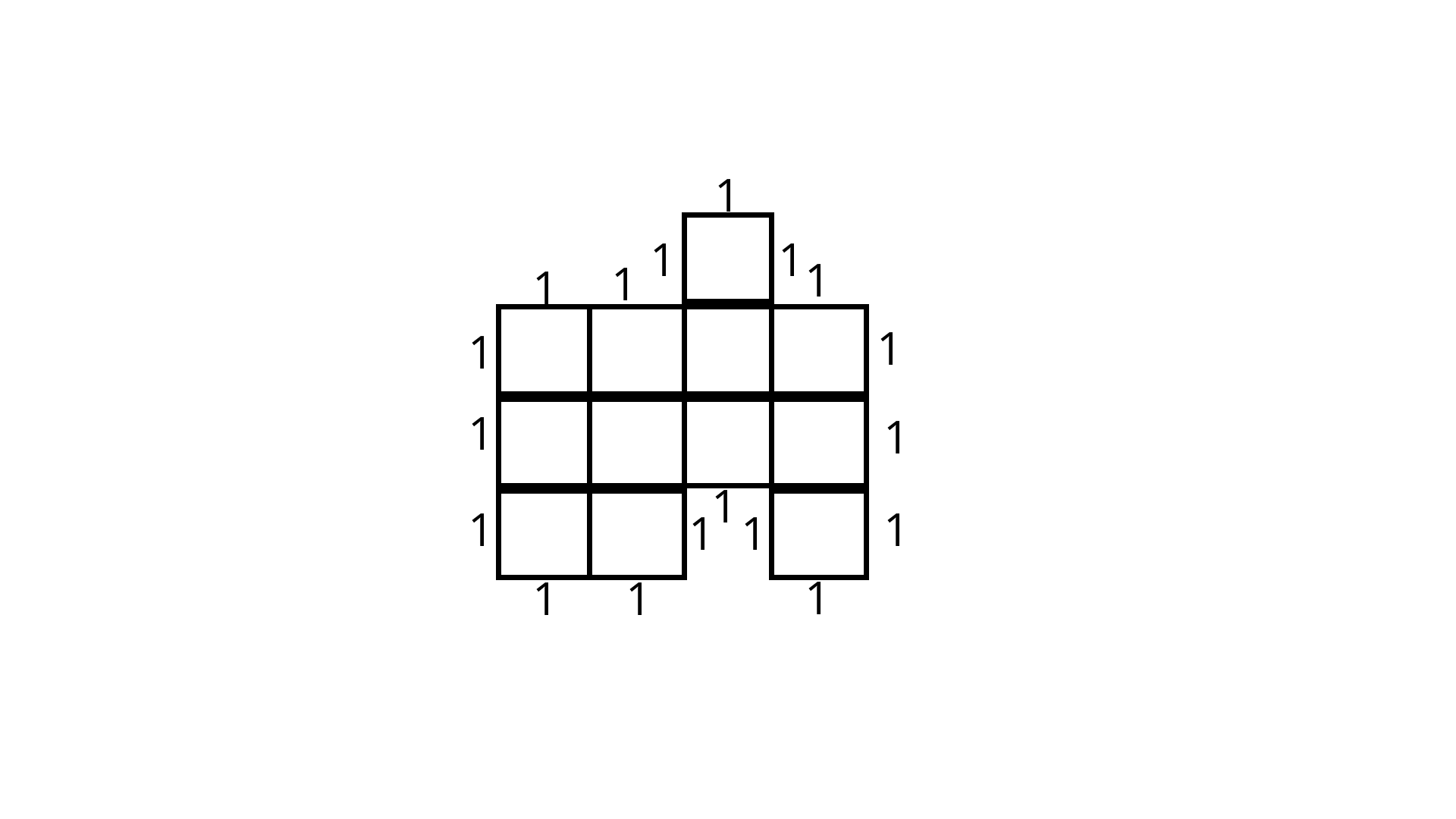
So, the perimeter is the sum of all sides. Hence, it is
59. If the perimeter of two parallelograms are equal, then their areas are also equal.
Ans: The statement is False
Their corresponding height and sides may be different.
So, the area cannot be equal.
60. All congruent triangles are equal in area.
Ans: The statement is True
Congruent triangles have equal size and shape.
Therefore, their areas are also equal.
61. All parallelograms having equal areas have the same perimeters.
Ans: The statement is False
Because it may be possible their height and base can be different, in this case not all parallelograms have equal areas.
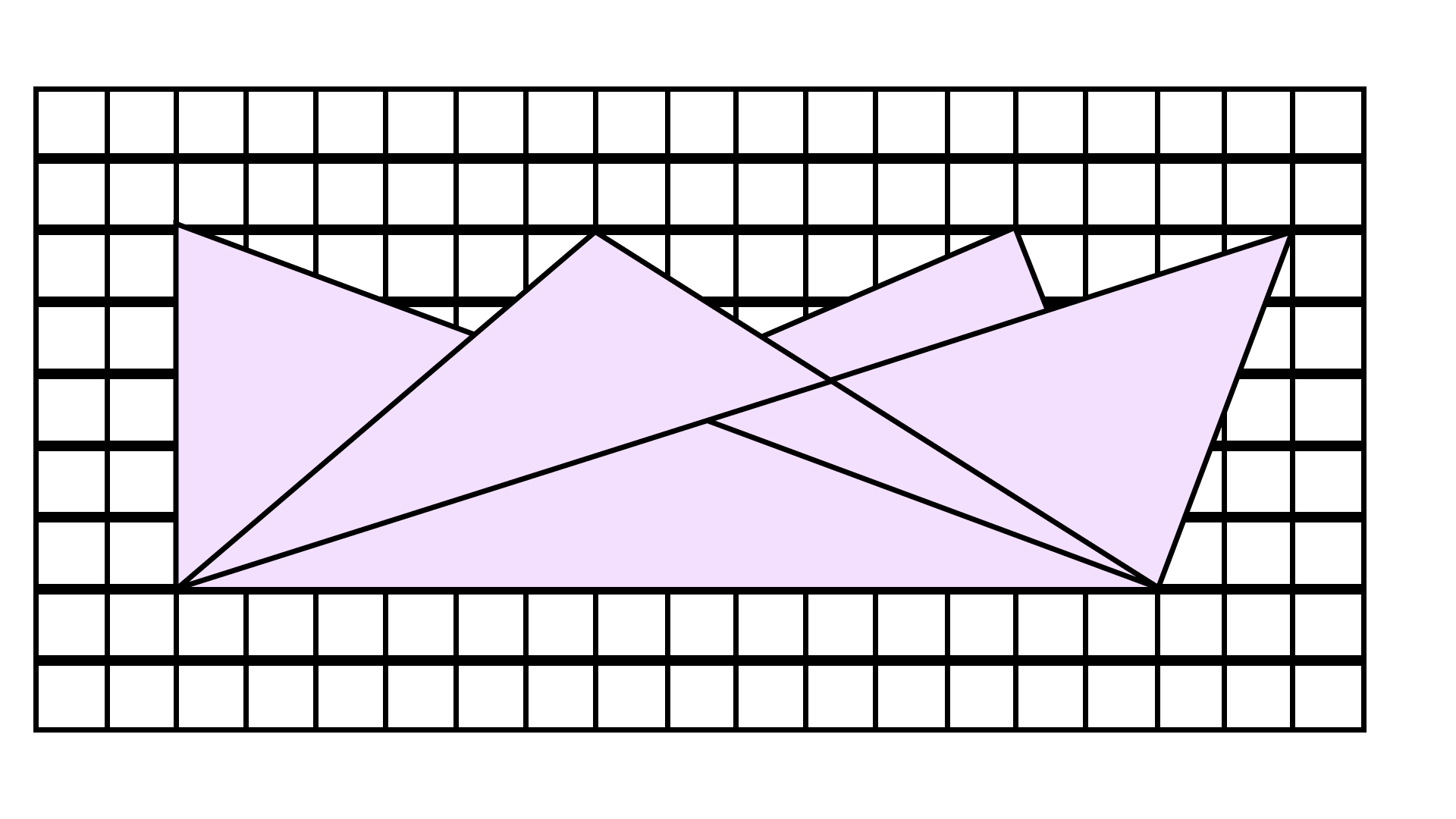
Observe all the four triangles
62. All triangles have the same base and the same altitude.
Ans: The statement is True
It is clear from the figure that all triangles have the same base
63. All triangles are congruent.
Ans: The statement is False
In the given figure, all triangles have only the baseline is equal and no such other lines are equal to each other.
64. All triangles are equal in area.
Ans: The statement is True
Because the triangles between, the same parallel lines and the same base are equal in the area.
65. All triangles may not have the same perimeter.
Ans: The statement is True
In the given figure, it is clear that not all triangles may have the same perimeter.
66. In Fig. 9.29 ratio of the area of triangle
Ans: The statement is True
Area of triangle
67. Triangles having the same base have equal area.
Ans: The statement is False
Area of triangle
From the formula, it is clear that the area of triangles depends on base and height not only on the base.
68. Ratio of the circumference of a circle to its radius is always
Ans: The statement is True
Circumference: Radius
69.
Ans: The statement is False
1 hectare
70. An increase in perimeter of a figure always increases the area of the figure.
Ans: The statement is False
It is not necessary, because the perimeter is the sum of all sides of closed shapes or polygons while the area is just bounded space insides.
71. Two figures can have the same area but different perimeters.
Ans: The statement is True
Yes, because the perimeter is the sum of all sides of closed shapes or polygons while the area is just bounded space insides. Therefore, it can be different.
72. Out of two figures if one has a larger area, then its perimeter need not to be larger than the other figure.
Ans: The statement is True
73. A hedge boundary needs to be planted around a rectangular lawn of size
Ans: Length of rectangular lawn
Breadth of rectangular lawn
Perimeter of rectangle
Perimeter of rectangle
If three shrubs can be planted in a metre of hedge.
Then,
Number of shrubs
74. People of Khejadli village take good care of plants, trees and animals. They say that plants and animals can survive without us, but we can not survive without them. Inspired by her elders Amrita marked some land for her pets (camel and ox) and plants. Find the ratio of the areas kept for animals and plants to the living area.
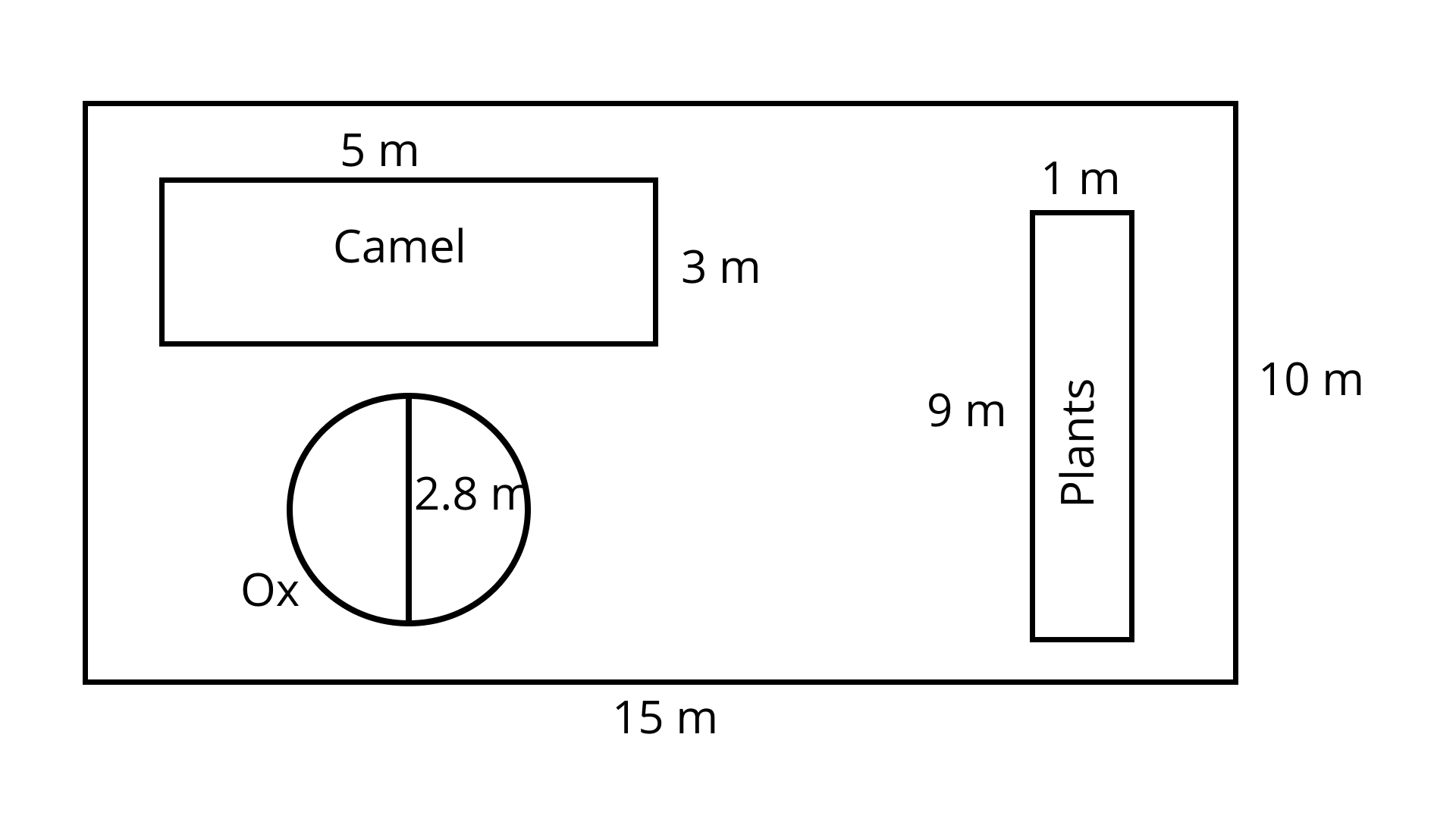
Ans: Area of covered land by plants
Area of rectangular land
Area of covered land by camel
Hence, the region of land covered by ox in a circular area.
Hence, diameter,
Radius
Region of land covered by ox
Total area covered by Camels, plants and ox
So, remaining land
Ratio of areas kept for animals and plants to the living areas
We should save our environment and balance the environment.
75. The perimeter of a rectangle is
Ans: Let, breadth of rectangle is
Perimeter of rectangle
Breadth
Hence, length
So, area of rectangle
76. A wall of a room is of dimensions
Ans: Given,
Length of the room
Breadth of the room
Area of the room
Also,
Length of the window
Breadth of the window
Area of the window
Now,
Length of the door
Breadth of the door
Area of the door
Now, area of the wall to be painted
77. Rectangle MNOP is made up of four congruent rectangles (Fig. 9.31). If the area of one of the rectangles is
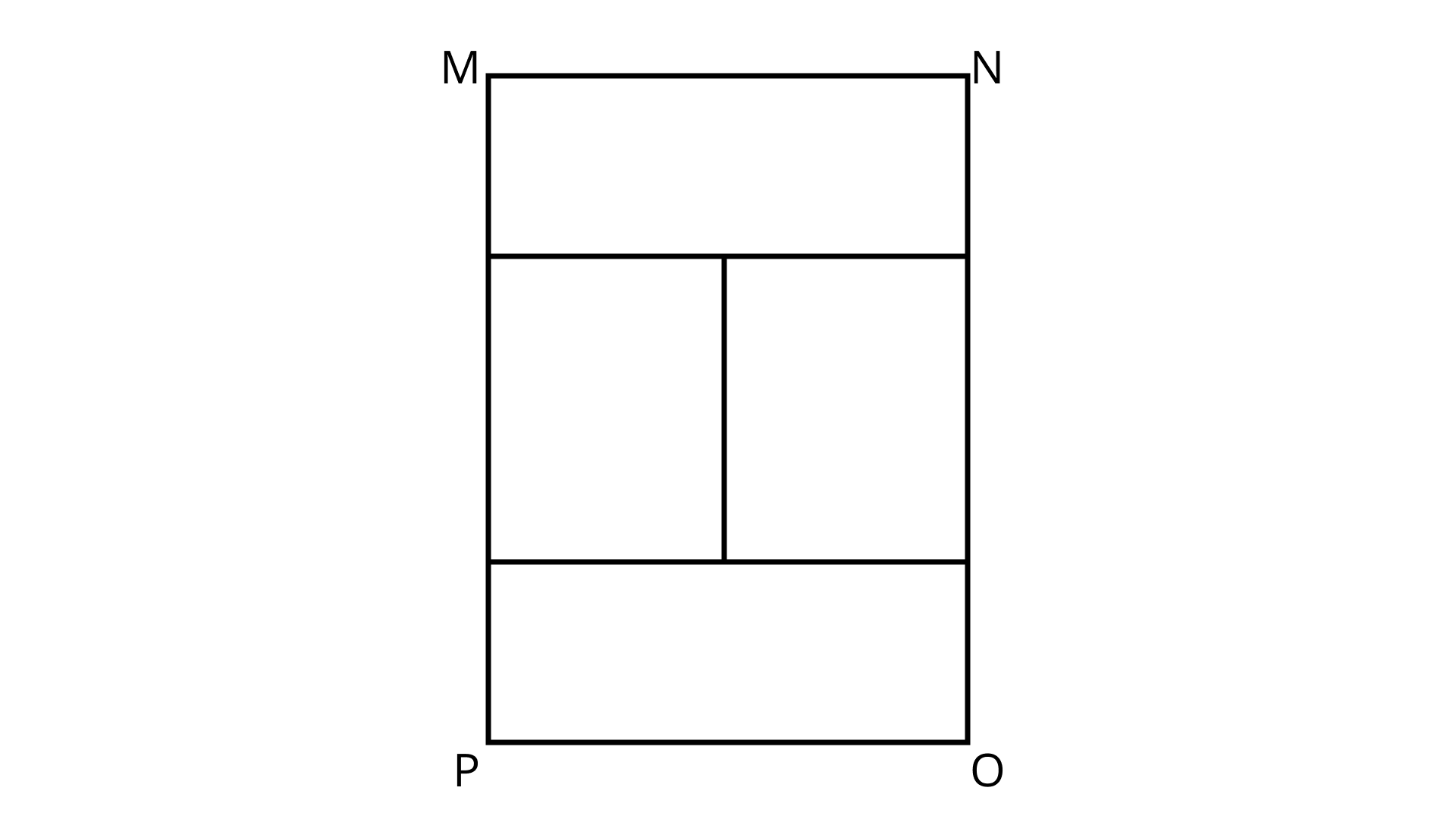
Ans: Given, Area of one rectangle
Breadth
Now, perimeter of rectangle MNOP
So, the perimeter of MNOP is
78. In Fig. 9.32, the area of
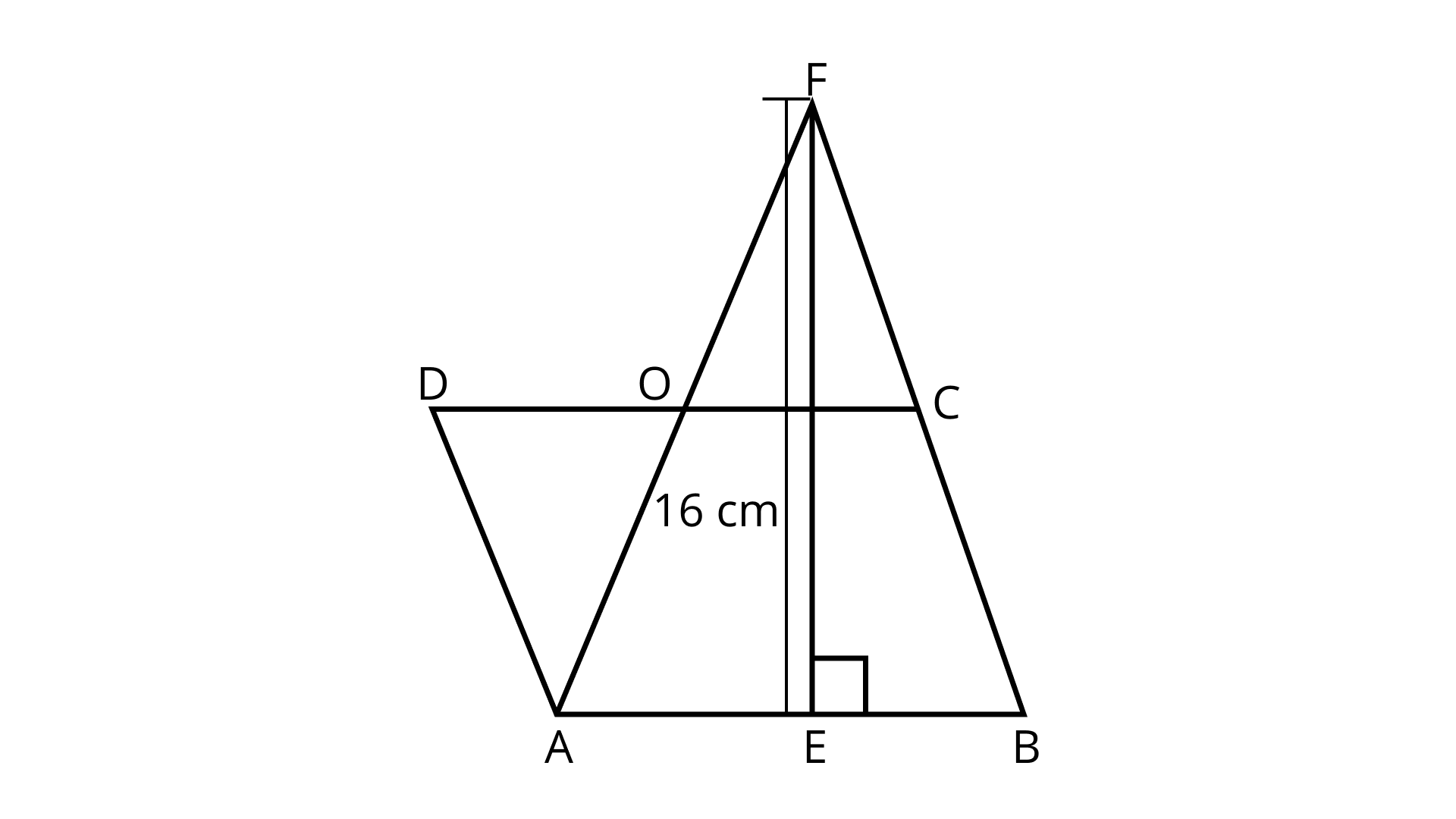
Ans: Given, Area of triangle
Let
In triangle DAO,
DO
Area of triangle
79. Ratio of the area of
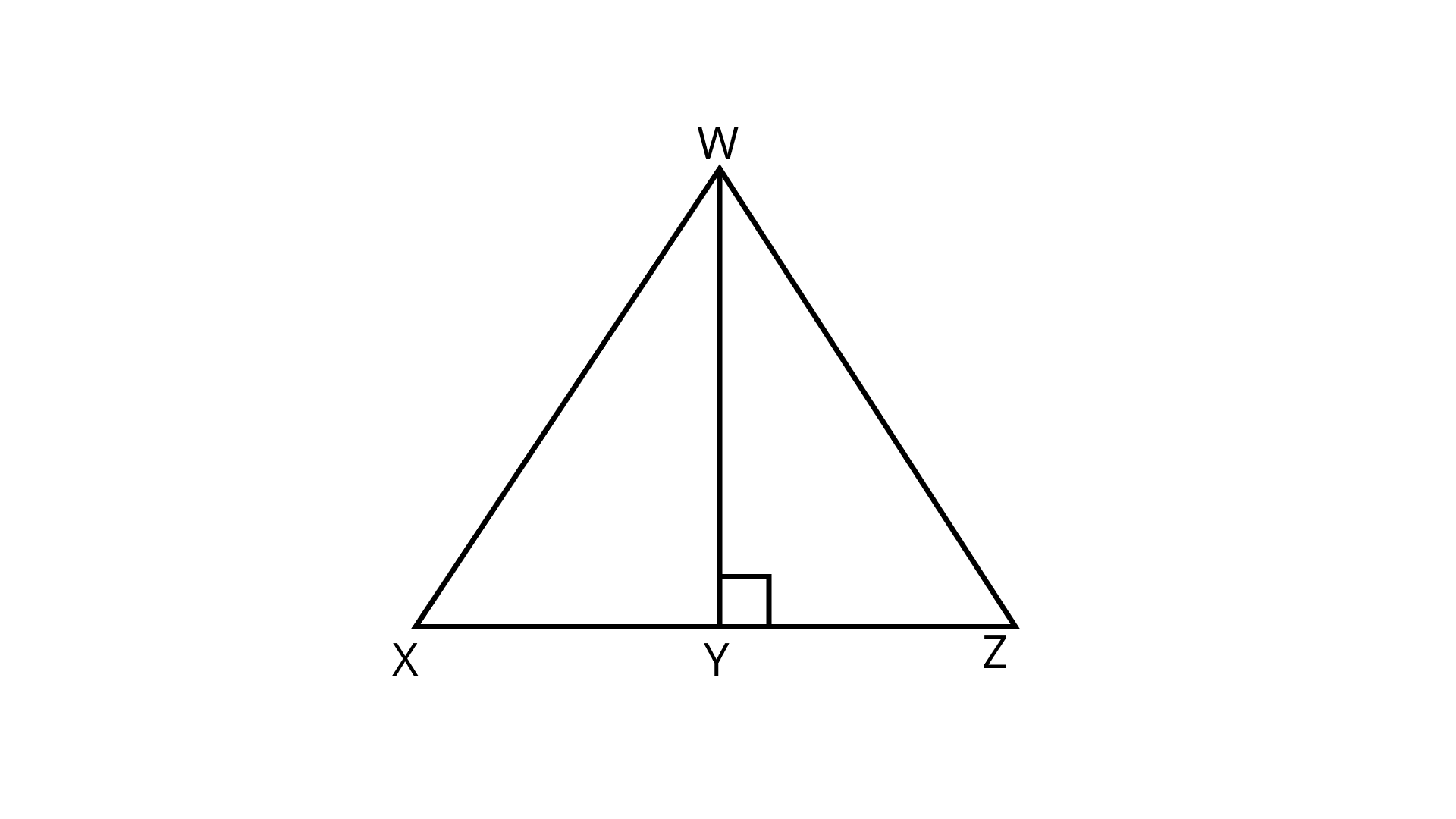
Ans: Area of triangle
Area of triangle
Hence,
Hence,
80. Rani bought a new field that is next to one she already owns (Fig. 9.34). This field is in the shape of a square of side
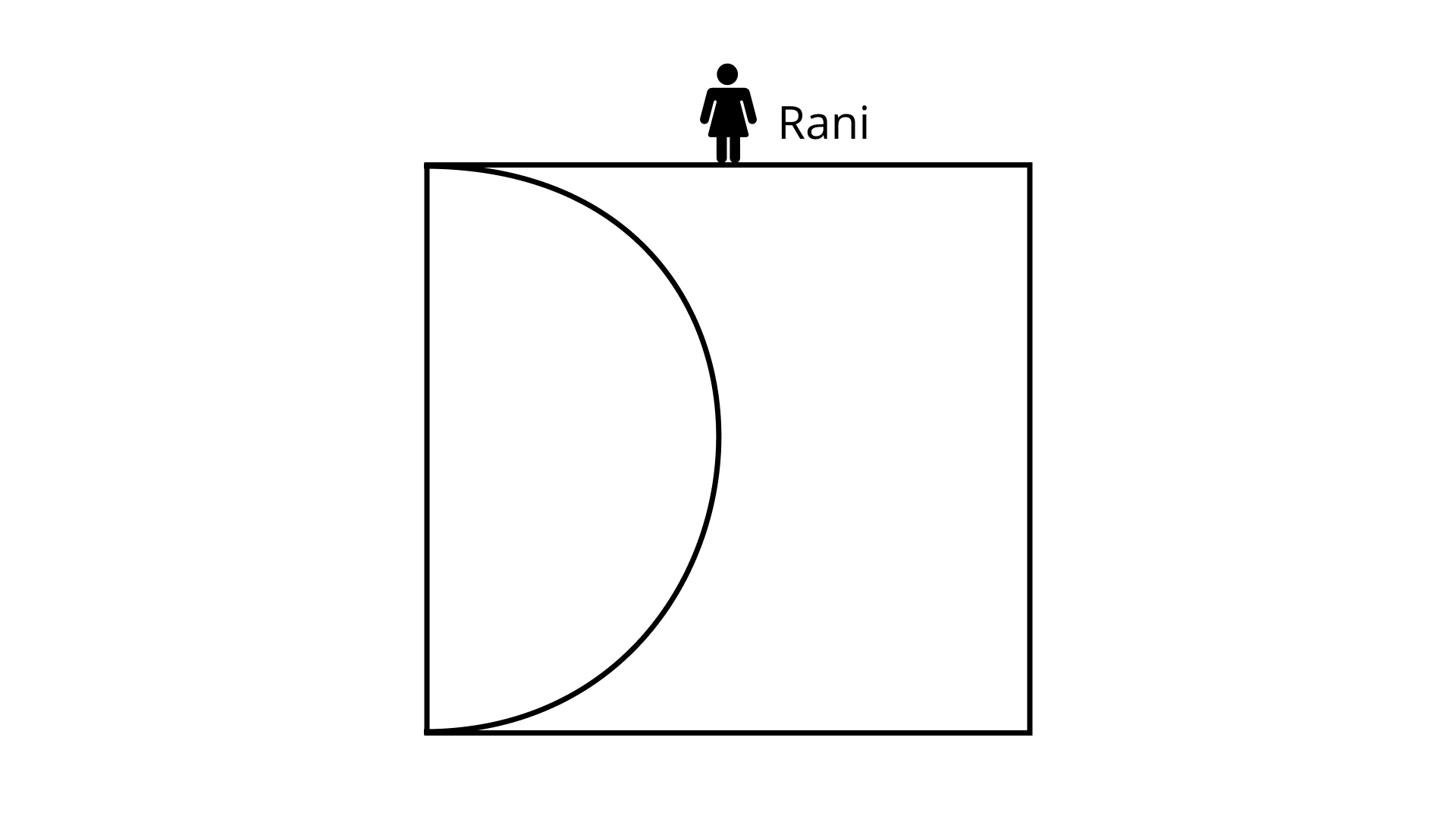
(i) Find the perimeter of the lawn.
Ans: Given,
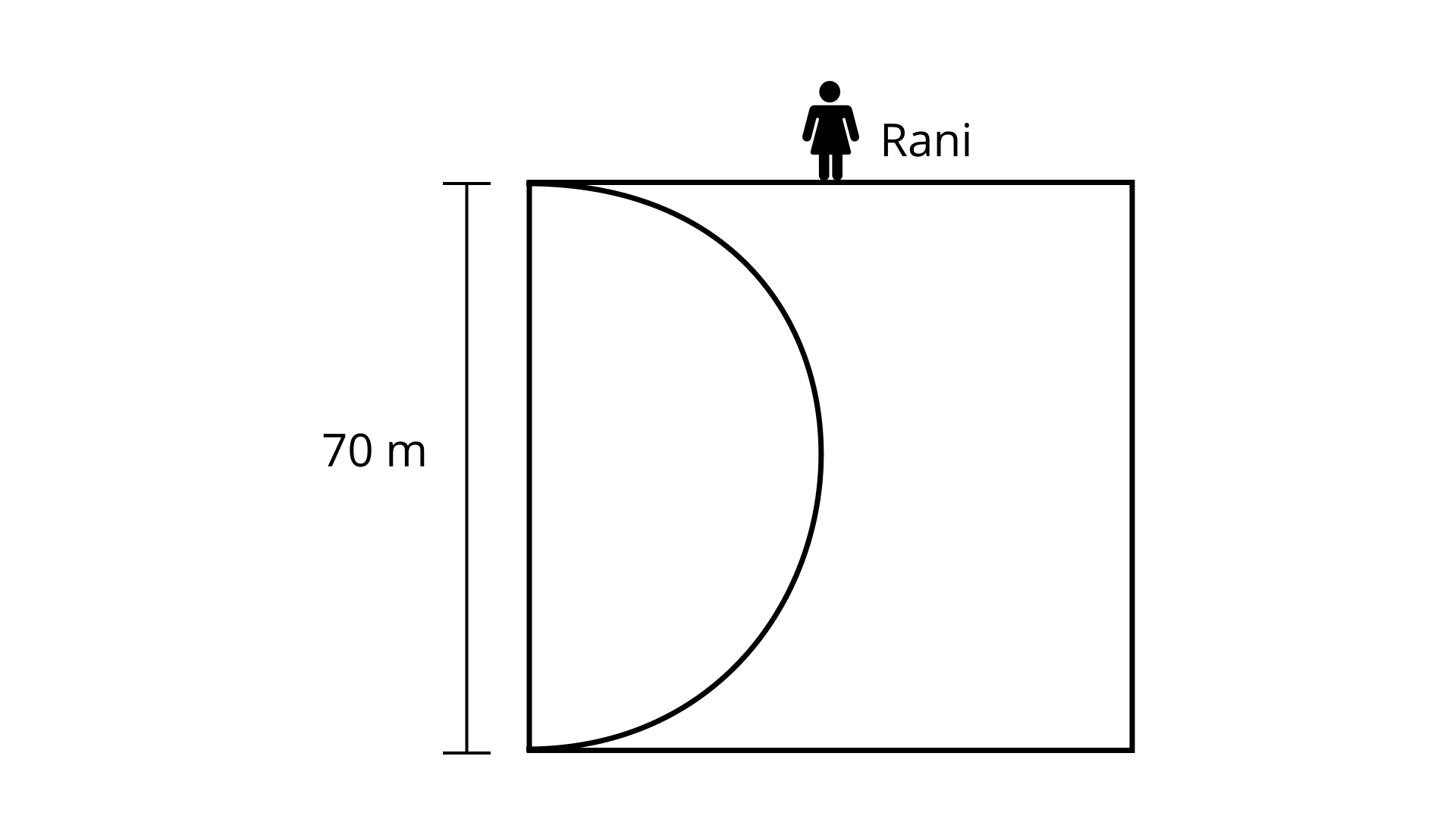
Side of a square field
So, diameter of semi circular lawn = Side of square field
Radius of semi circular lawn
Perimeter of semi circular lawn
Hence, perimeter of the lawn
(ii) Find the area of the square field excluding the lawn.
Ans: Area of square
Hence, required area
81. In Fig. 9.35, find the area of parallelogram
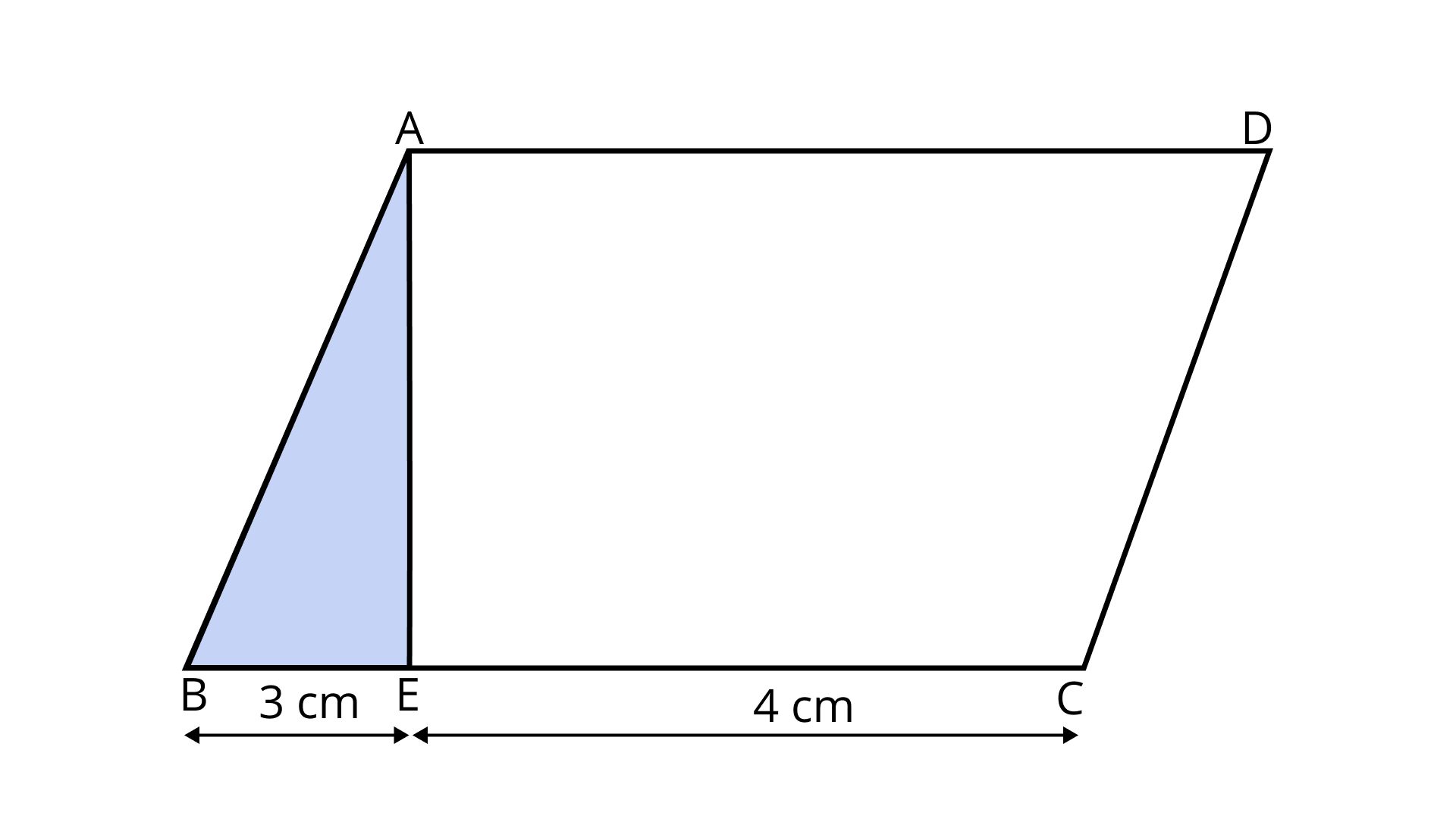
Ans: Given,
Area of shaded portion
Base of triangle
Now, area of triangle,
Area of parallelogram
82. Pizza factory has come out with two kinds of pizzas. A square pizza of side
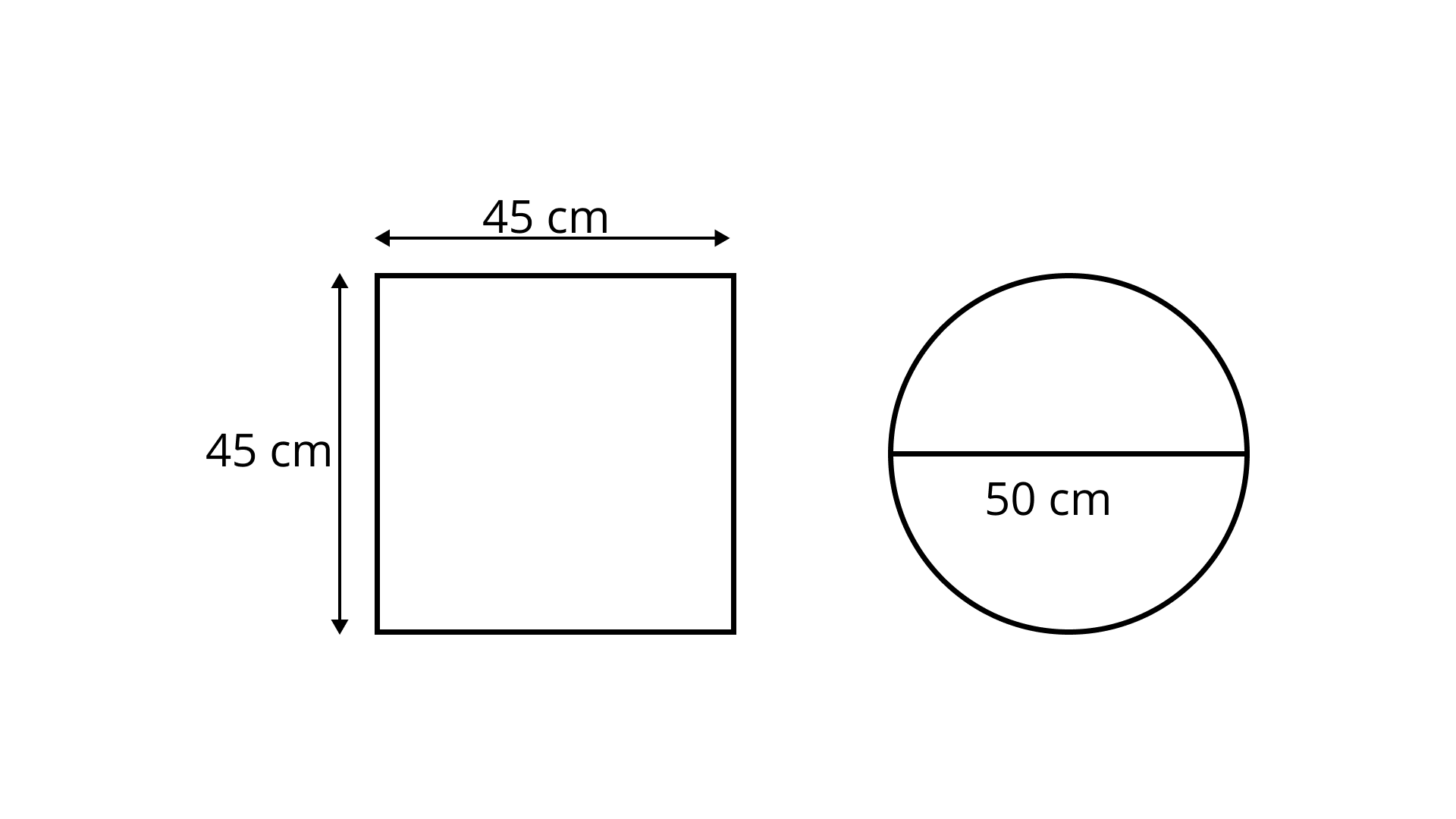
Ans: Given,
Side of square pizza
Diameter of circular pizza
Radius
Area of square pizza
Area of circular pizza
Therefore, price of
Price of
Hence, the best deal is circular pizza.
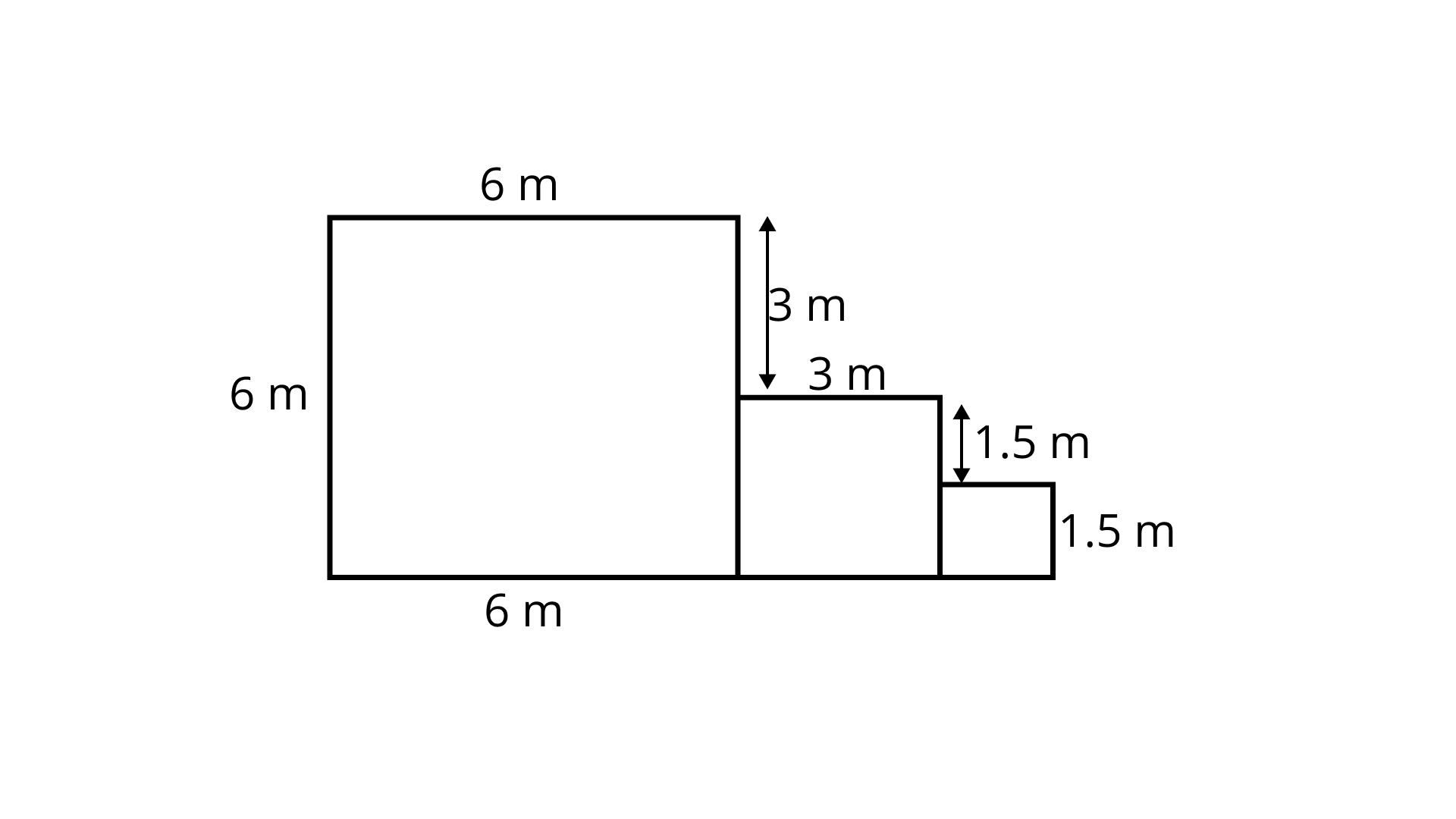
83. Three squares are attached to each other as shown in Fig. 9.37. Each square is attached at the midpoint of the side of the square to its right. Find the perimeter of the complete figure.
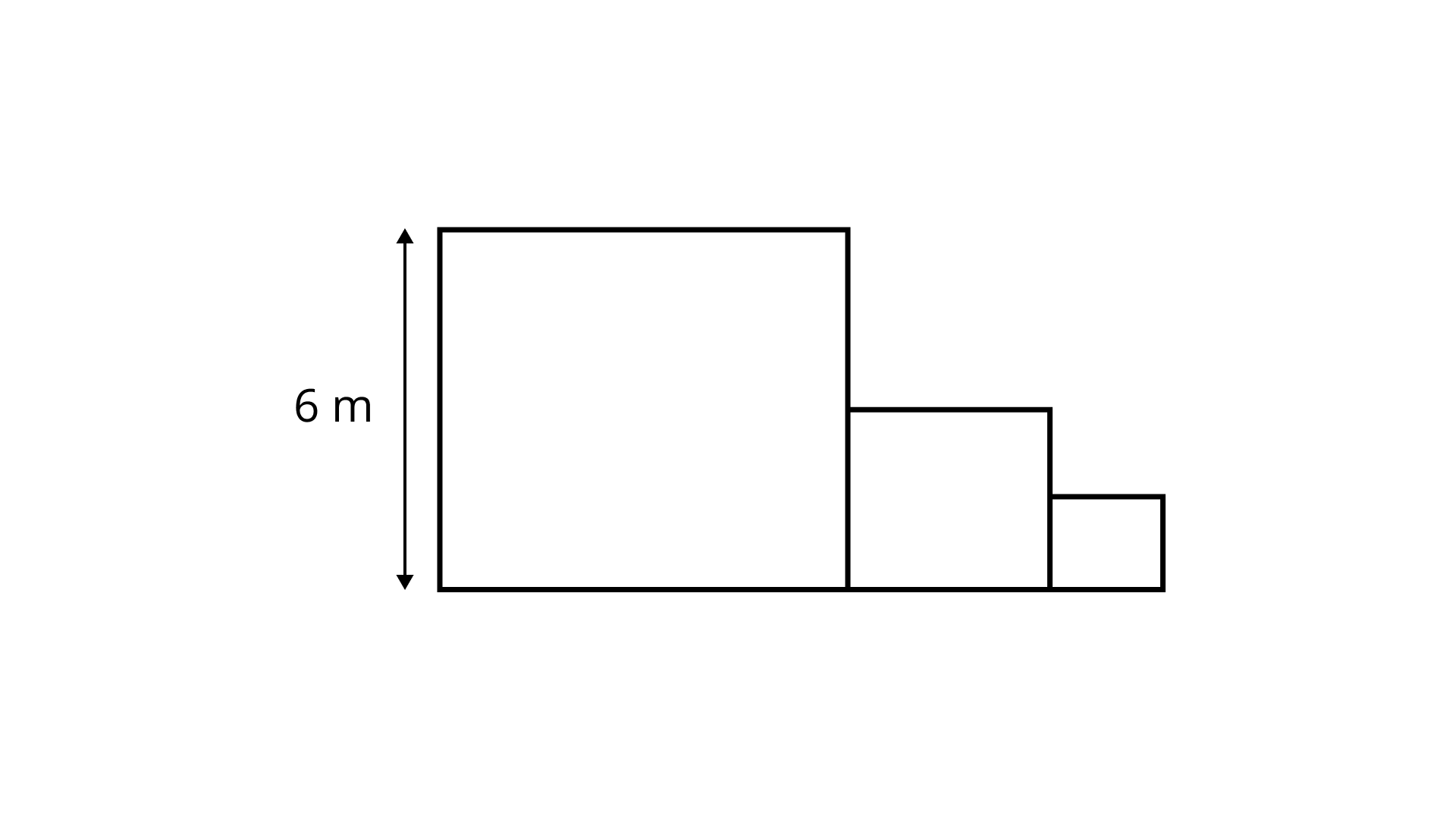
Ans: Perimeter of the complete figure
84. In Fig. 9.38,
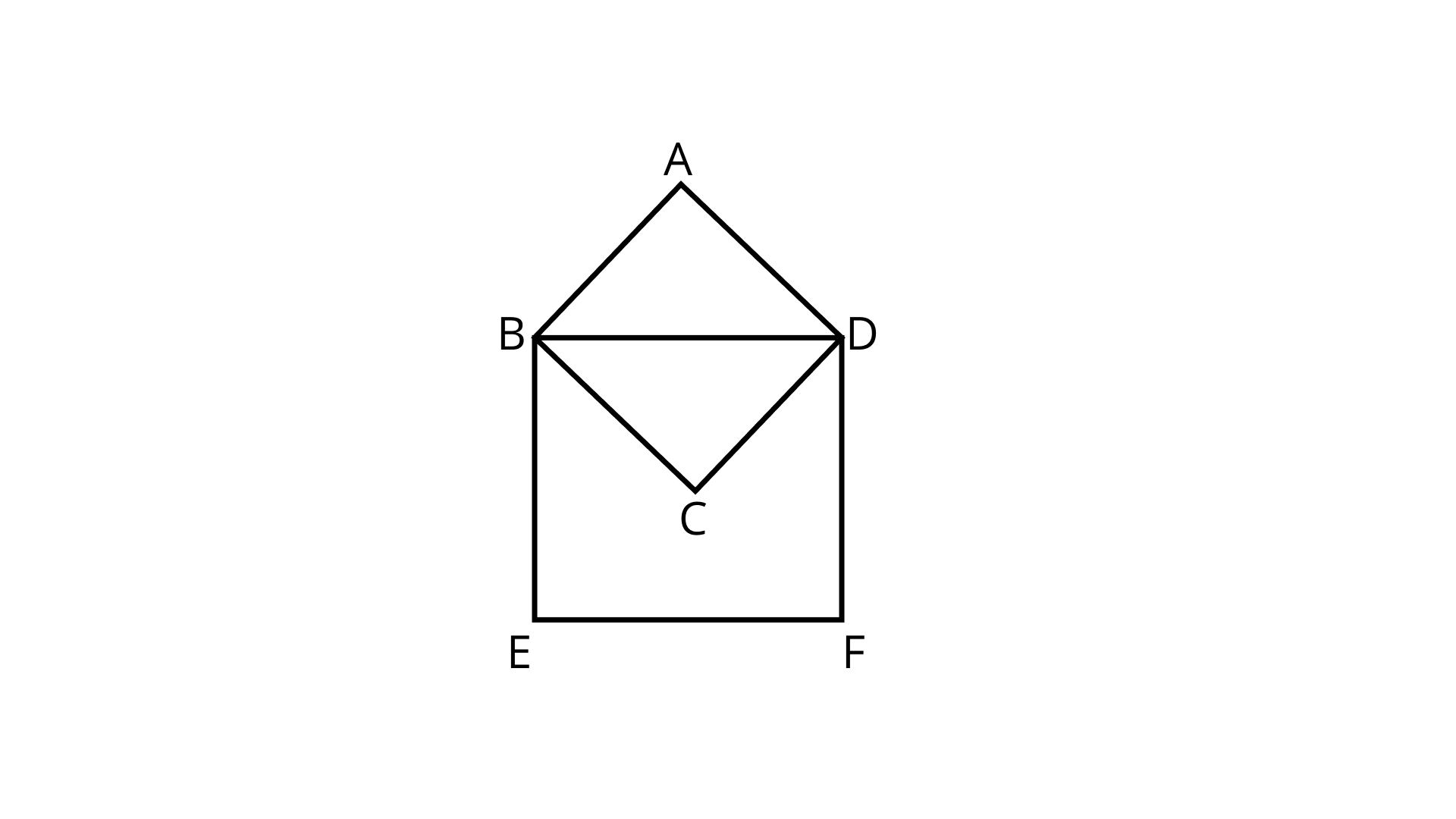
Ans: Given,
Diagonal of square
From the figure,
Diagonal of square
Therefore, area of the square
85. In the given triangles of Fig. 9.39, perimeter of
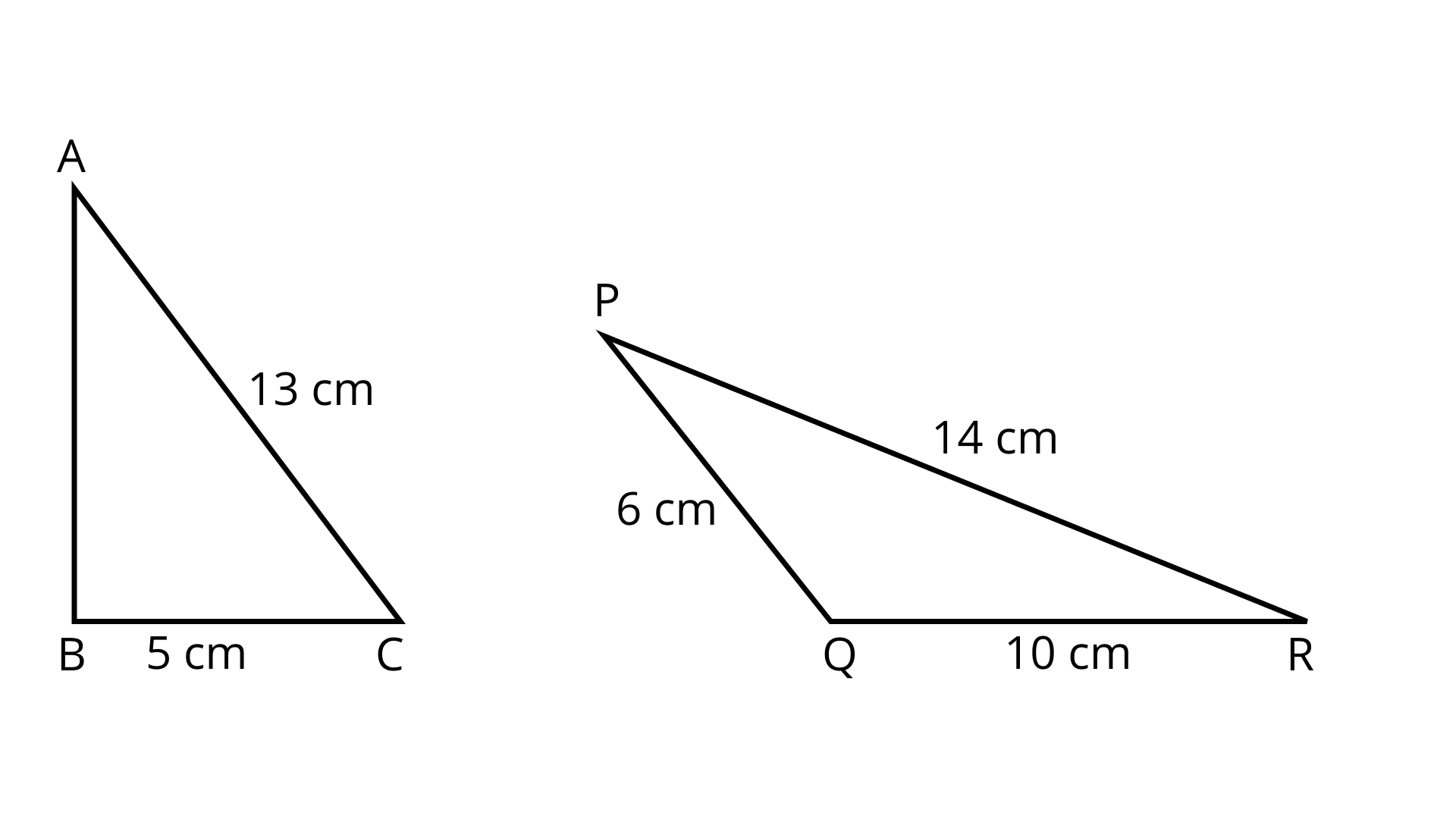
Ans: Given,
Perimeter of triangle
Perimeter of triangle
Now,
Perimeter of triangle
Hence, area of triangle
86. Altitudes MN and MO of parallelogram MGHK are
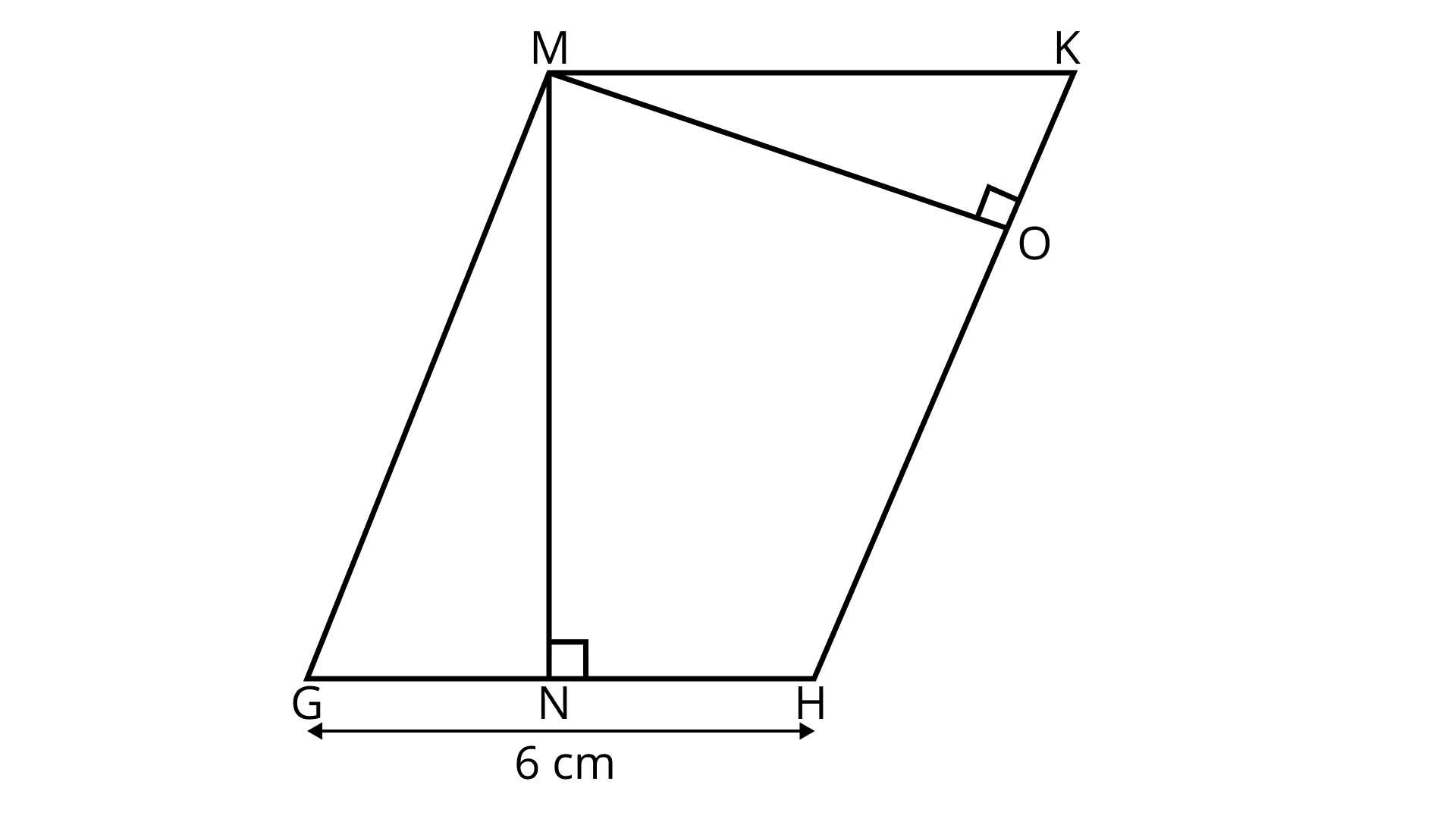
Ans: Given,
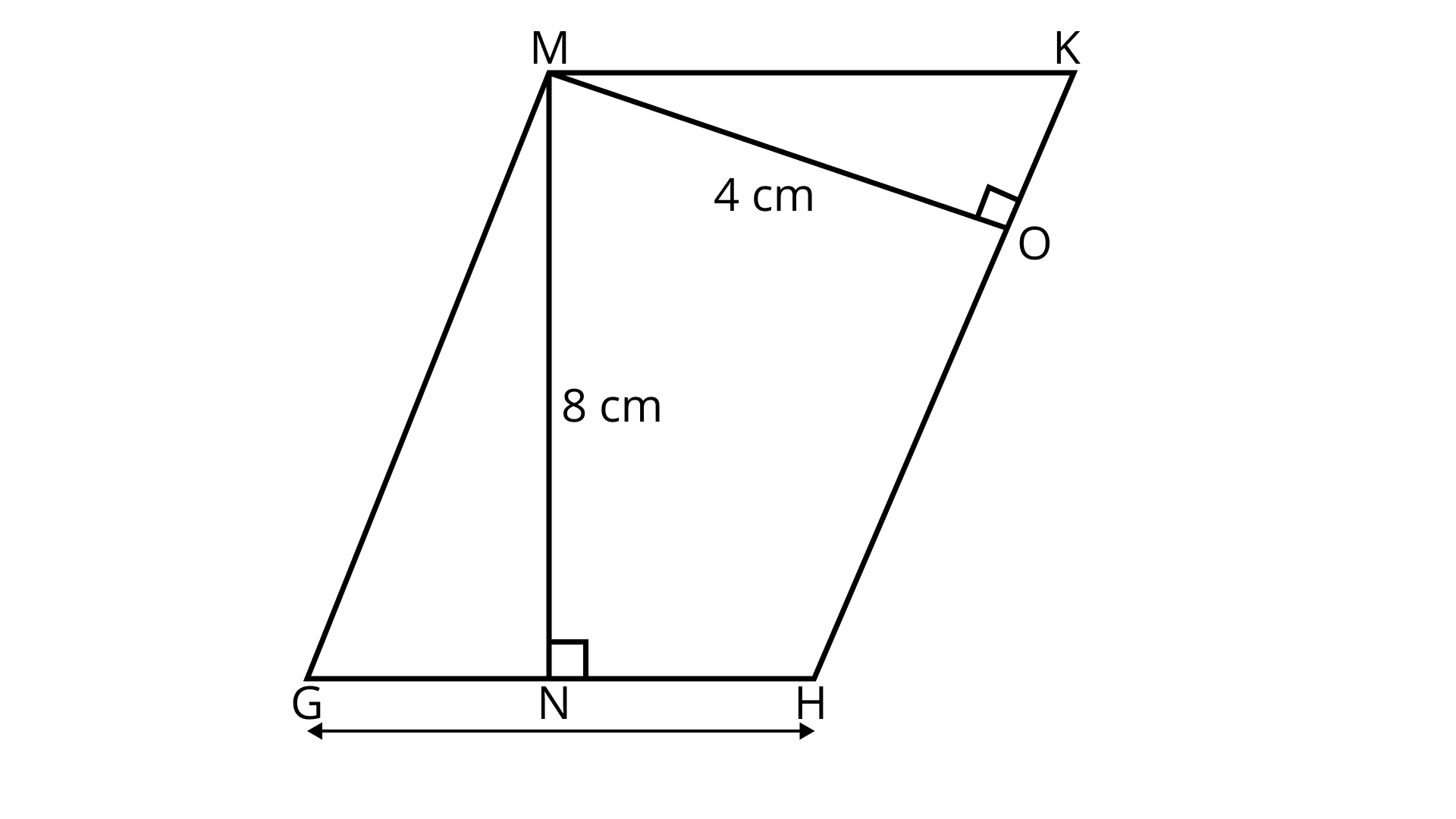
Now, area of parallelogram MGHK, when base is GH
Now, area of parallelogram MGHK, when base is HK
In parallelogram, opposite sides are equal
Thus,
Therefore, perimeter of parallelogram
87. In Fig. 9.41, area of
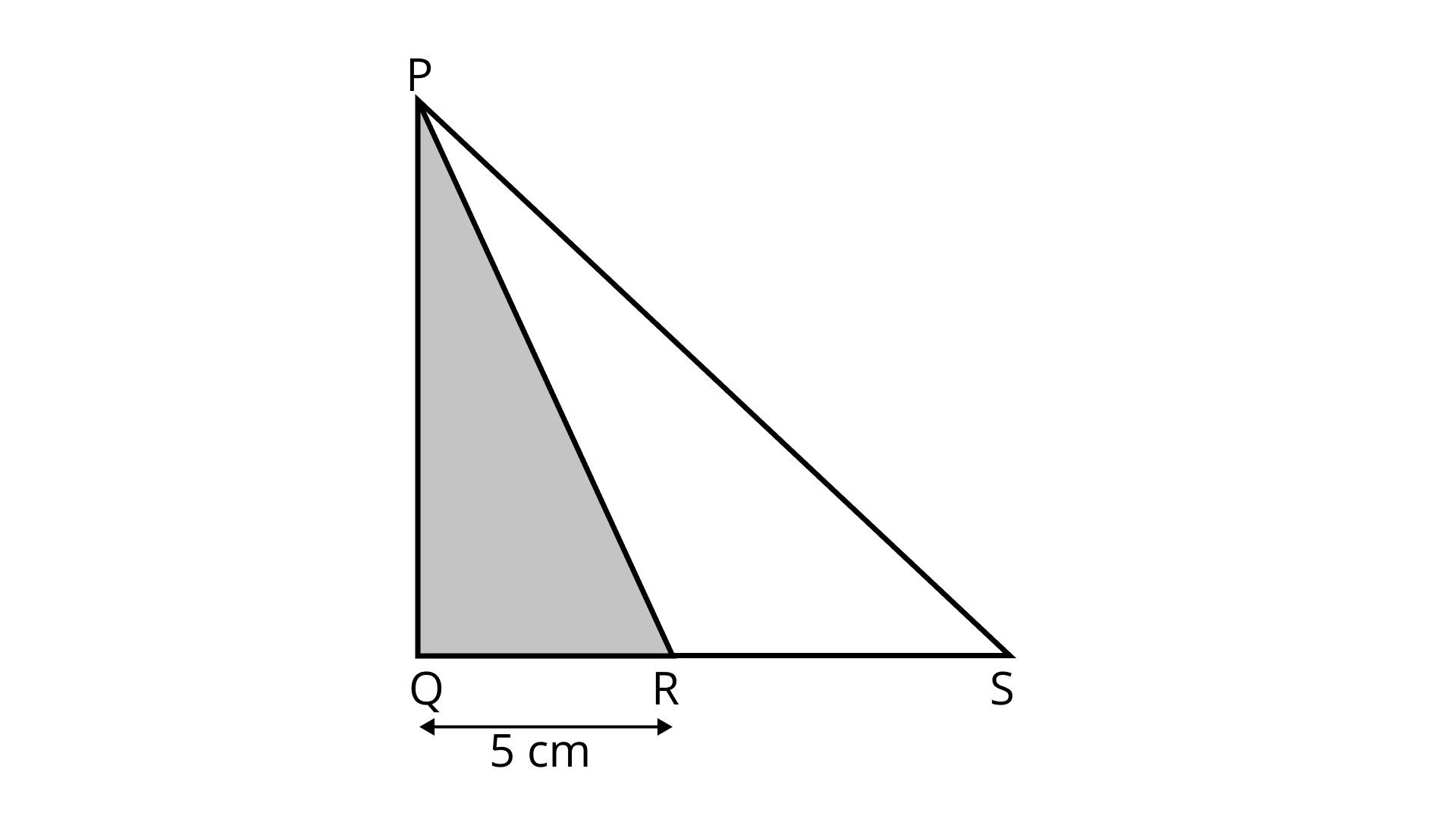
Ans: Given,
Area of triangle PQR
Area of triangle PQS
Area of triangle PQR,
Area of triangle PQS,
Now,
88. Area of an isosceles triangle is
Ans:
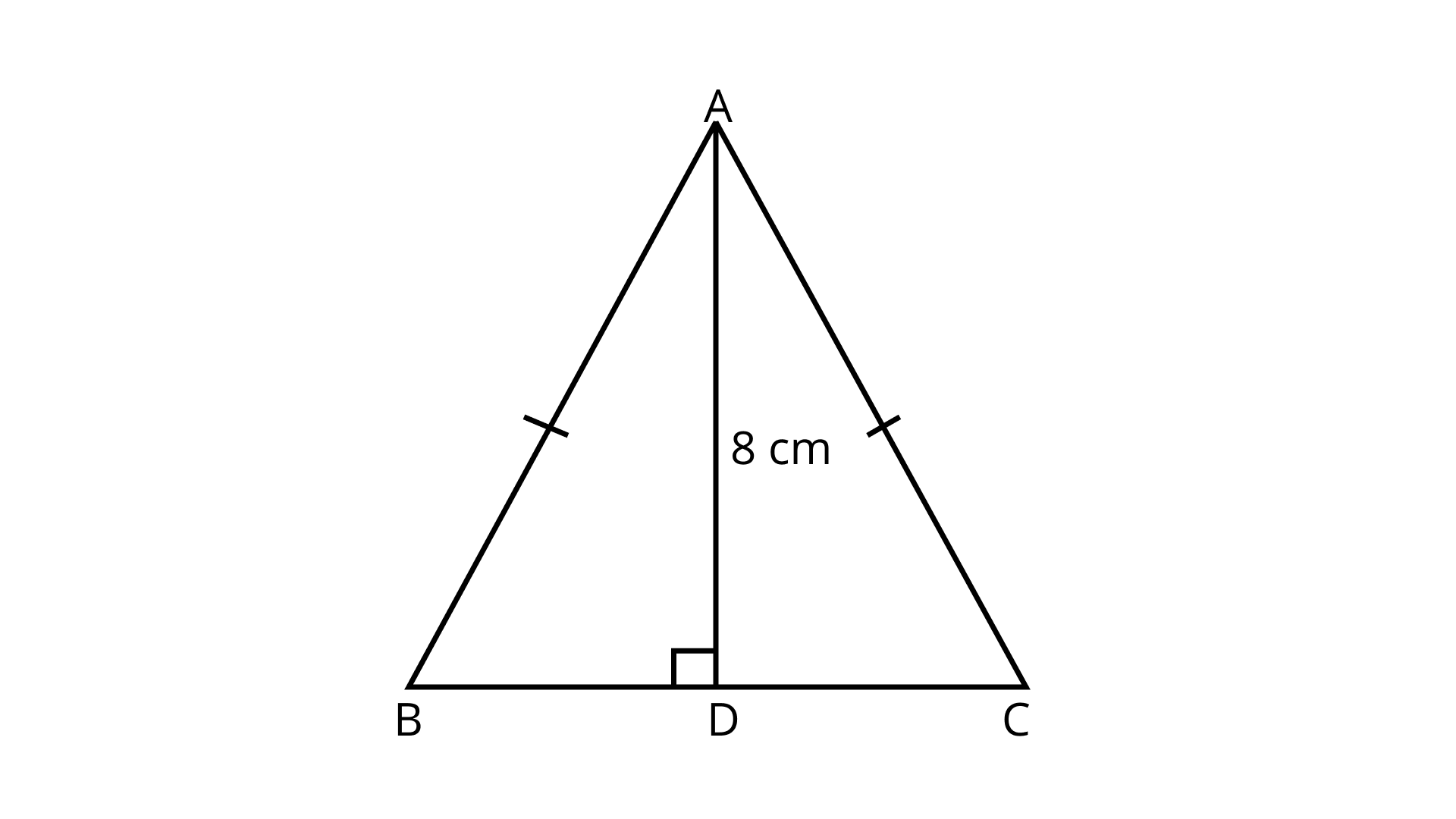
Given,
Area of triangle
Height
Area of triangle
Now, in a isosceles triangle,
Applied Pythagoras theorem in triangle ADB
Now, perimeter of triangle
89. Perimeter of a parallelogram shaped land is

Ans: Given,
Perimeter of parallelogram
Area of parallelogram
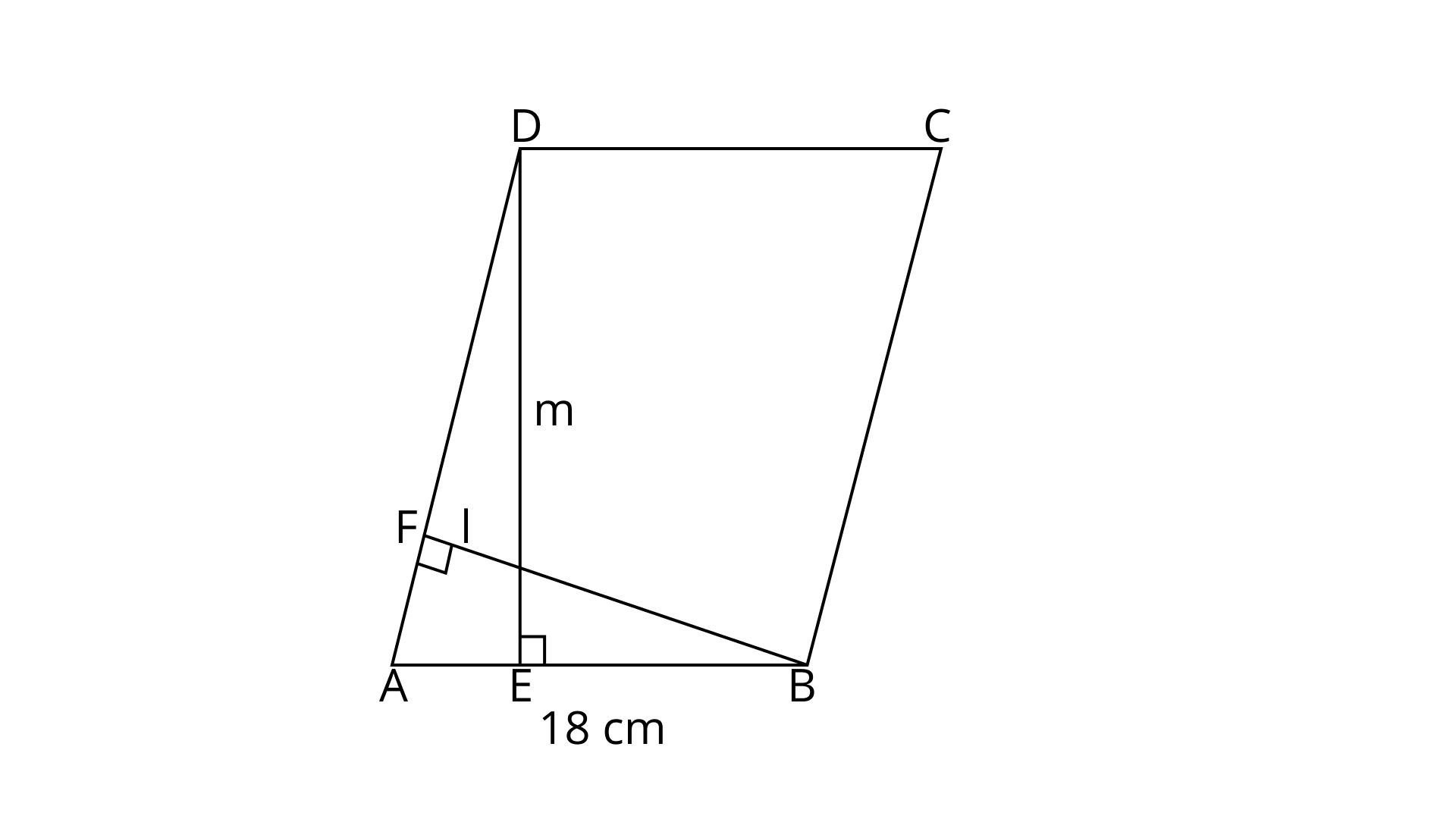
In parallelogram
As we know perimeter of a parallelogram
Now, area of parallelogram ABCD,
Now, area of parallelogram
90. Area of a triangle
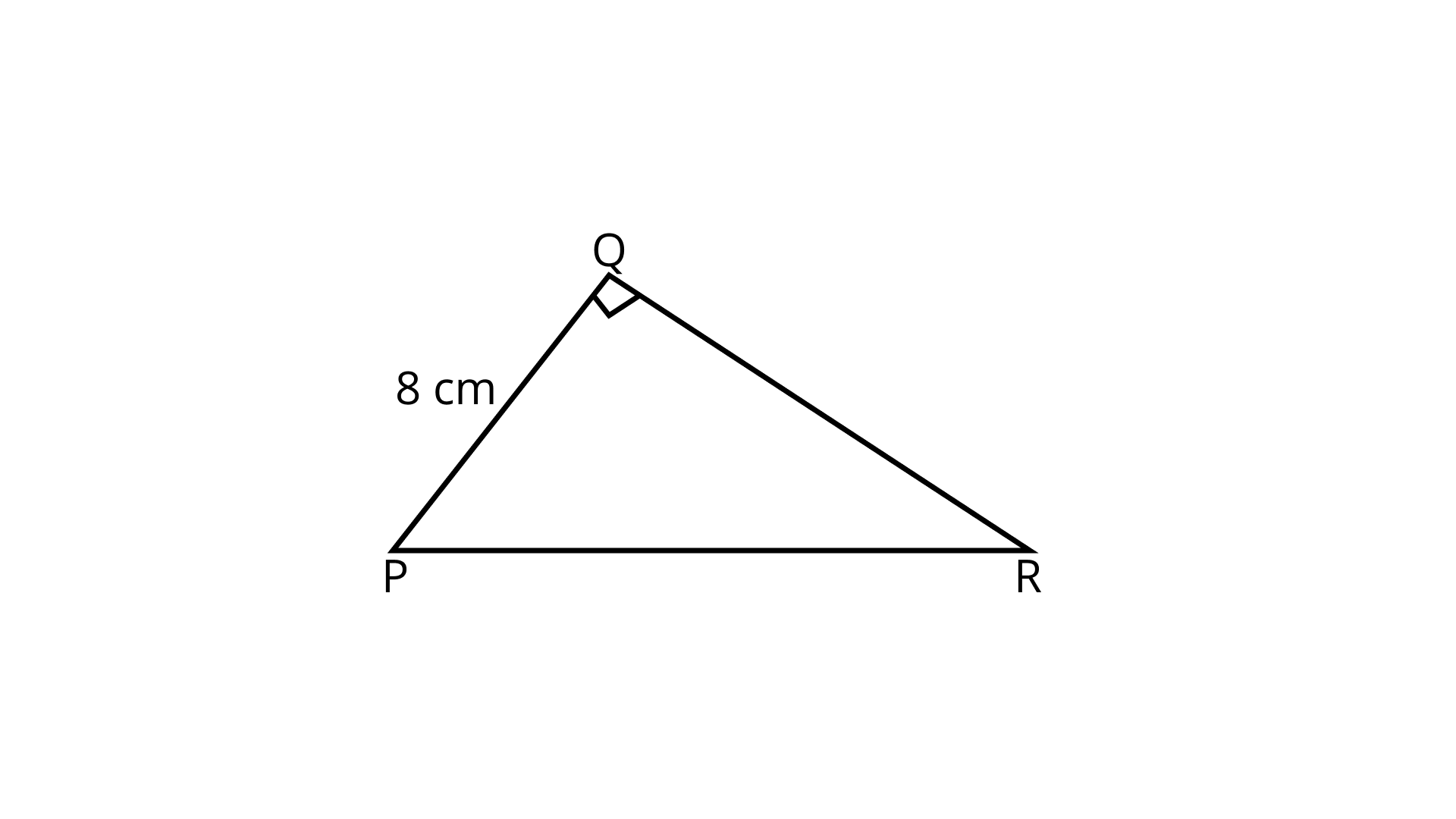
Ans: Given,
Area of triangle
Side
Area of triangle PQR,
Applied Pythagoras theorem in triangle PQR
Therefore,
91. In Fig. 9.44 a rectangle with perimeter
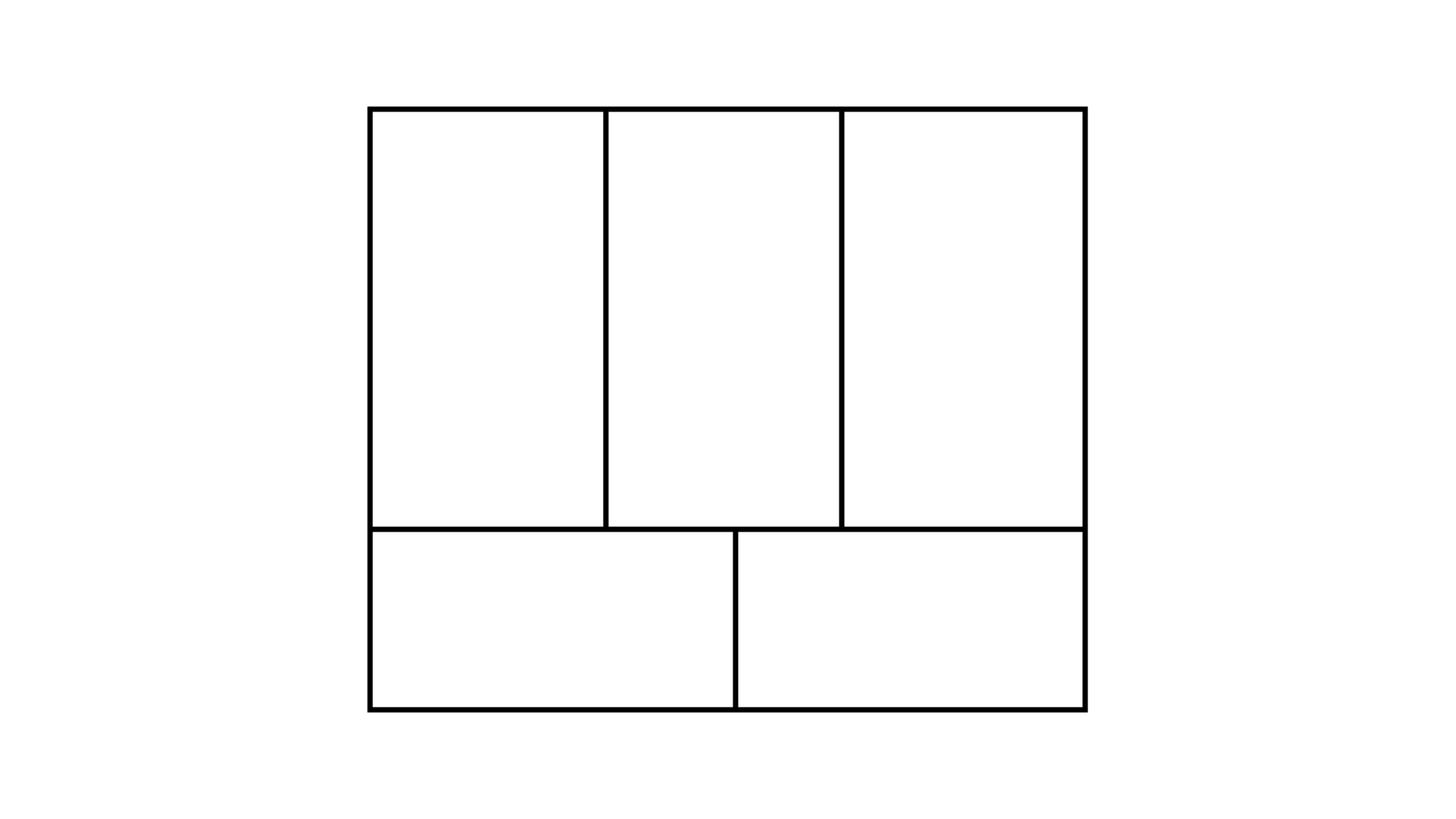
Ans: Let
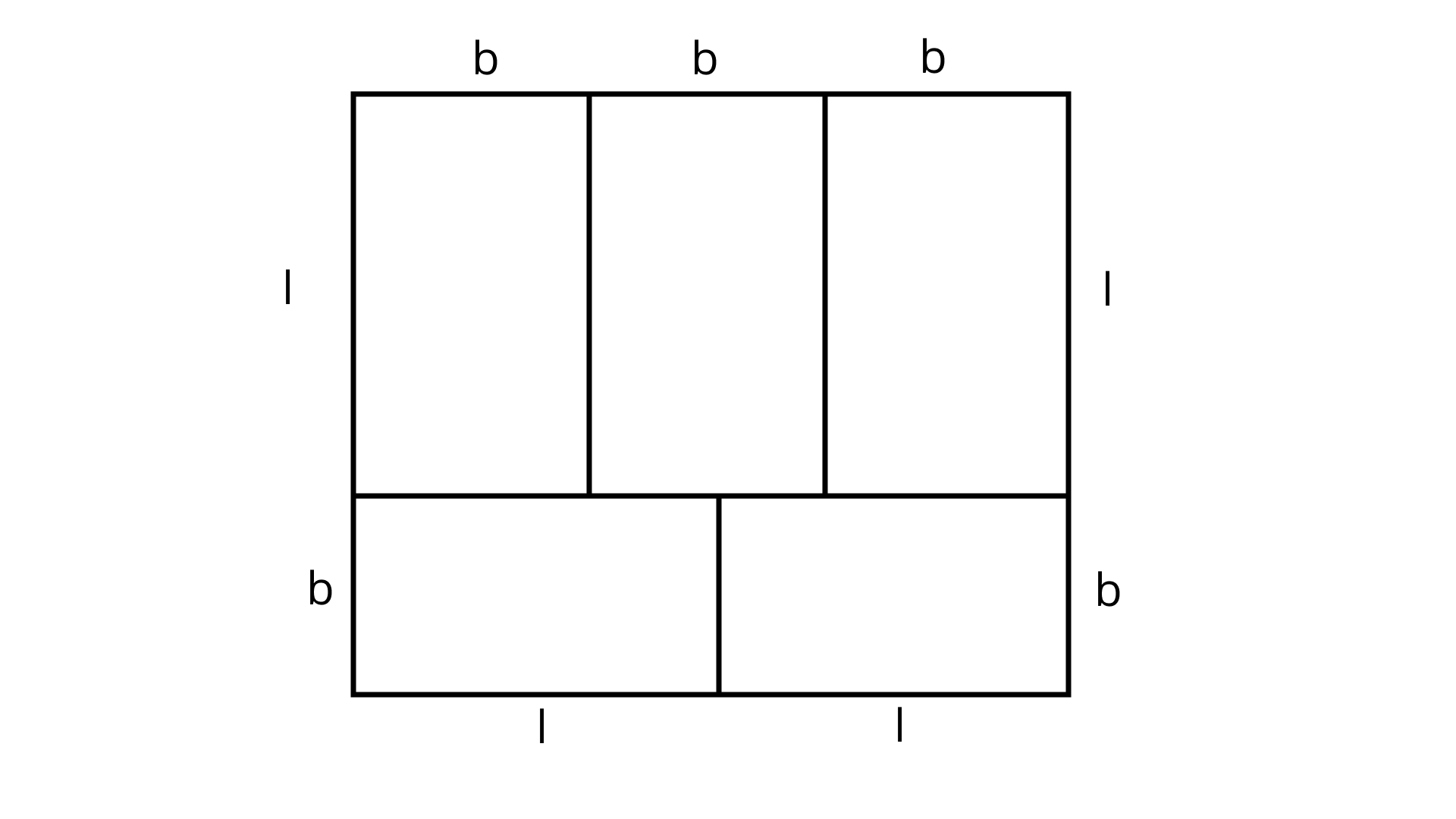
According to the figure,
Now, put the value of
Therefore, perimeter of the rectangle
92. Find the area of a square inscribed in a circle whose radius is
Hint: Four right-angled triangles joined at right angles to form a square
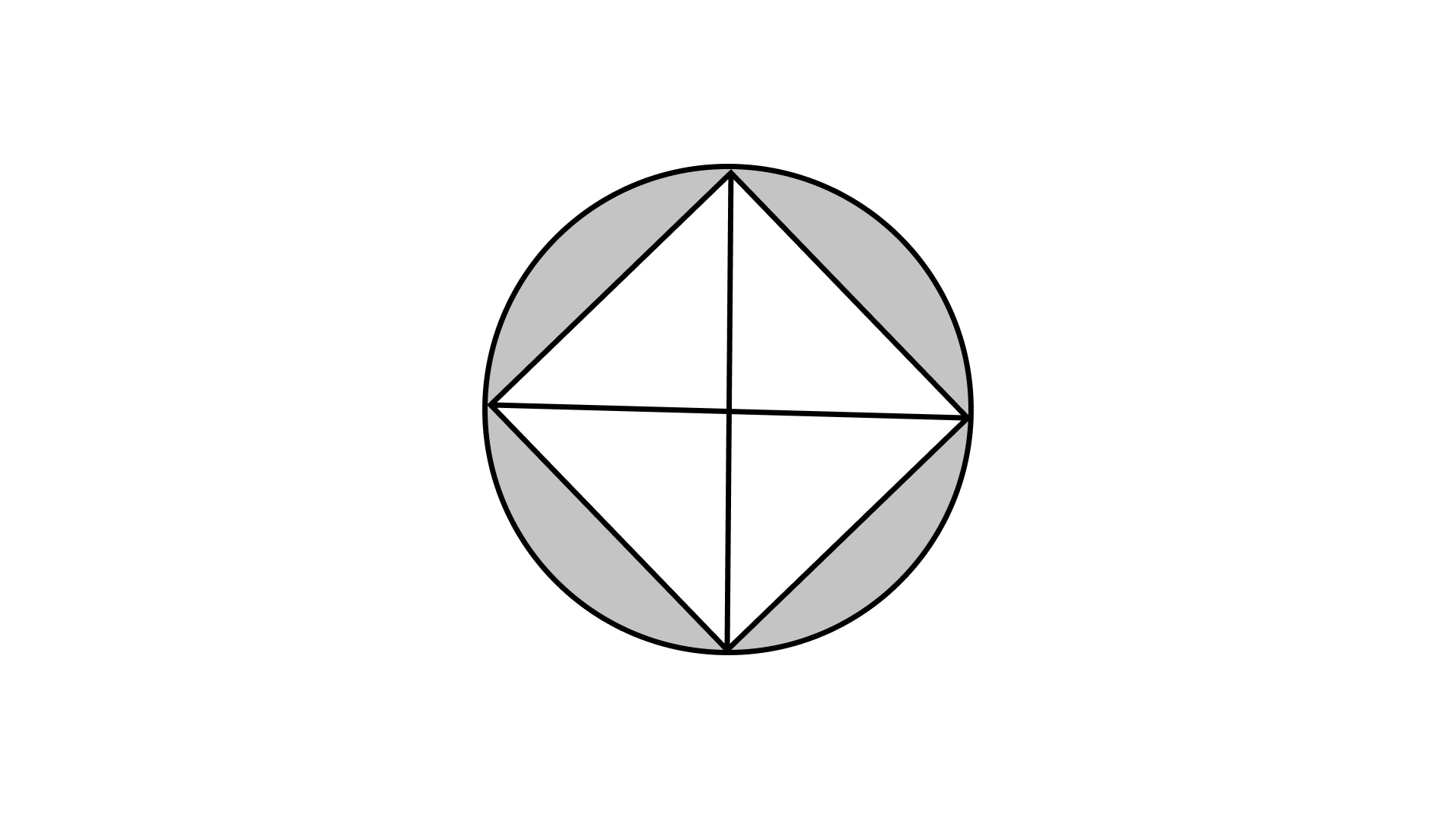
Ans:
Area of square
Now, Area of square
Therefore, the area of the inscribed square is
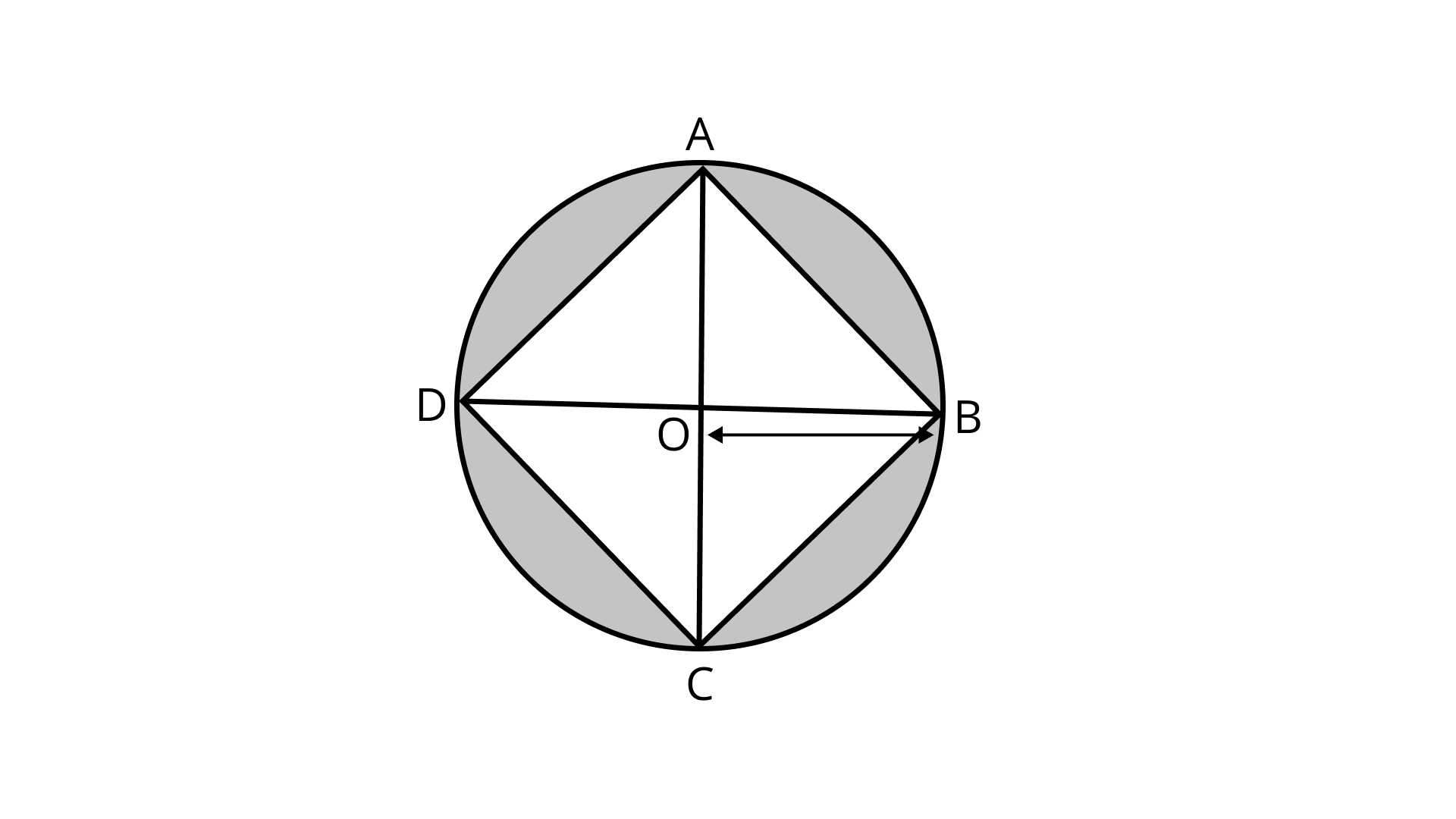
93. Find the area of the shaded portion in question 92.

Ans:
Area of square
Now, Area of square ABCD
Now, area of shaded portion
In Questions 94 to 97 find the area enclosed by each of the following figures:
94.
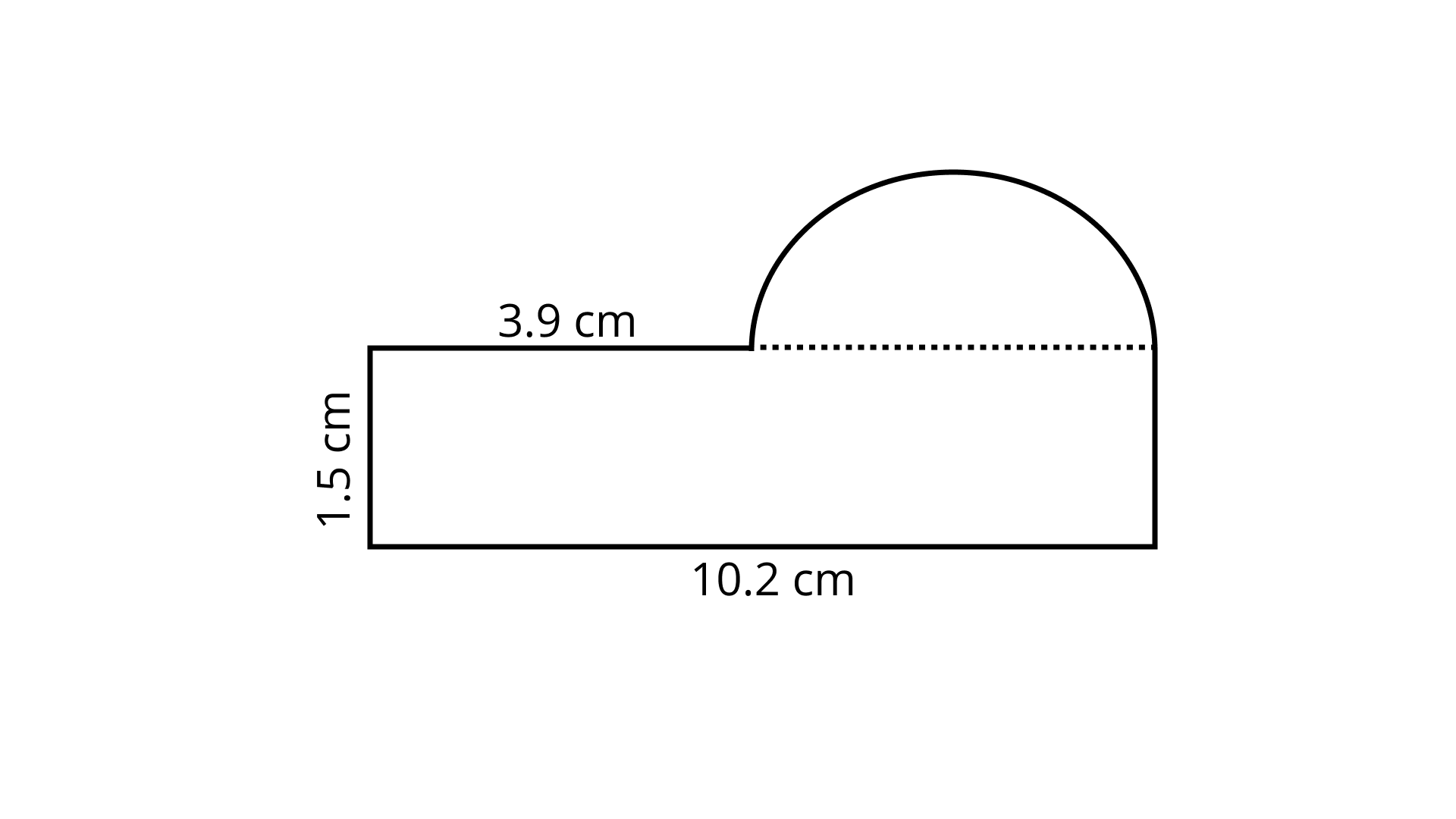
Ans: The Sample contains a semi-circle and a rectangle.
Area of rectangle
Diameter of semi-circle
Radius
Area of semi-circle
Therefore, total area
95.
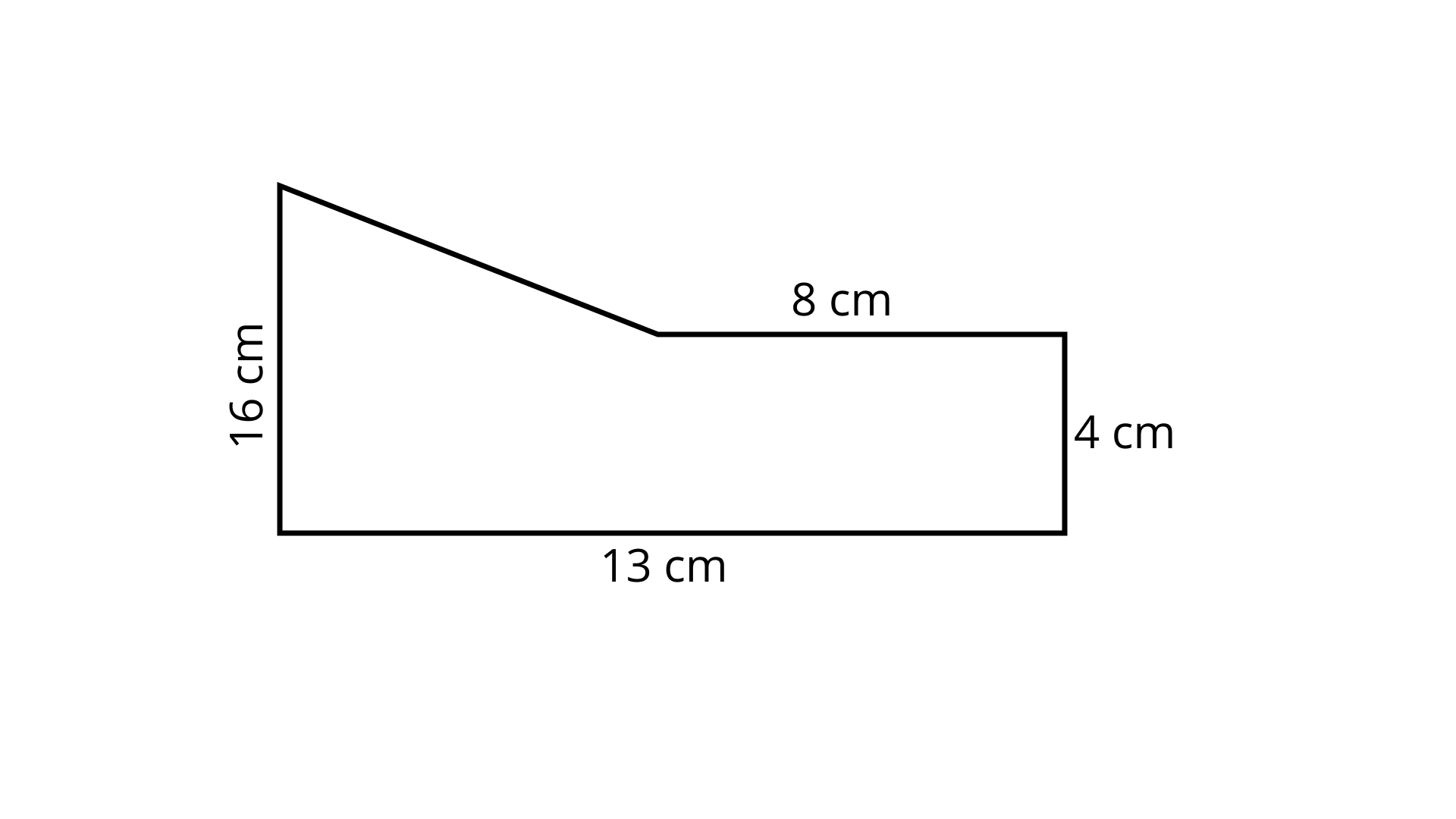
Ans: The Sample contains a triangle and a rectangle.
Area of rectangle
Area of triangle
Therefore, the total area enclosed in shape
96.
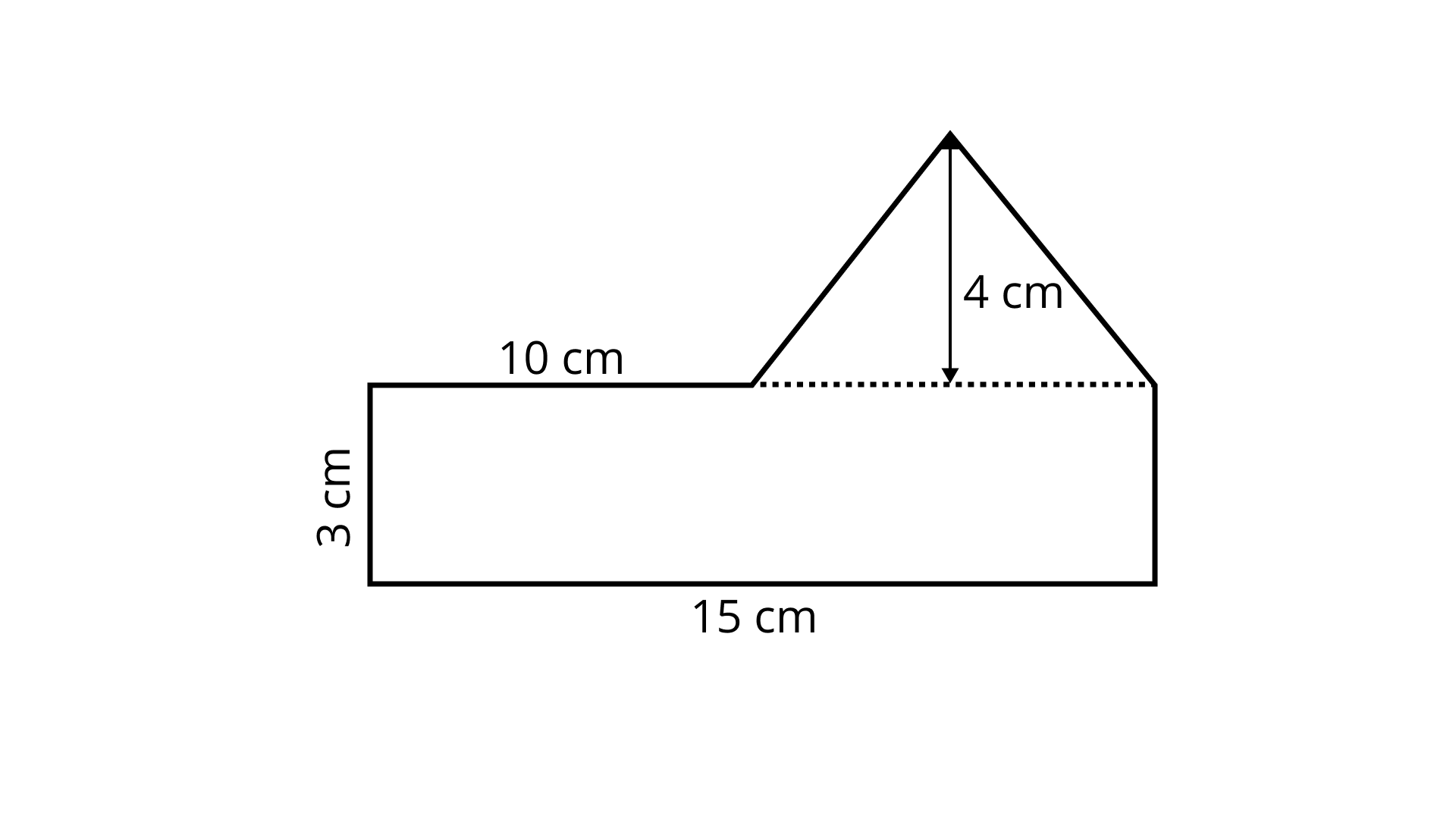
Ans: The Sample contains a triangle and a rectangle.
Area of rectangle
According to the figure the base
Now, area of triangle
Therefore, the total area enclosed in shape
97.
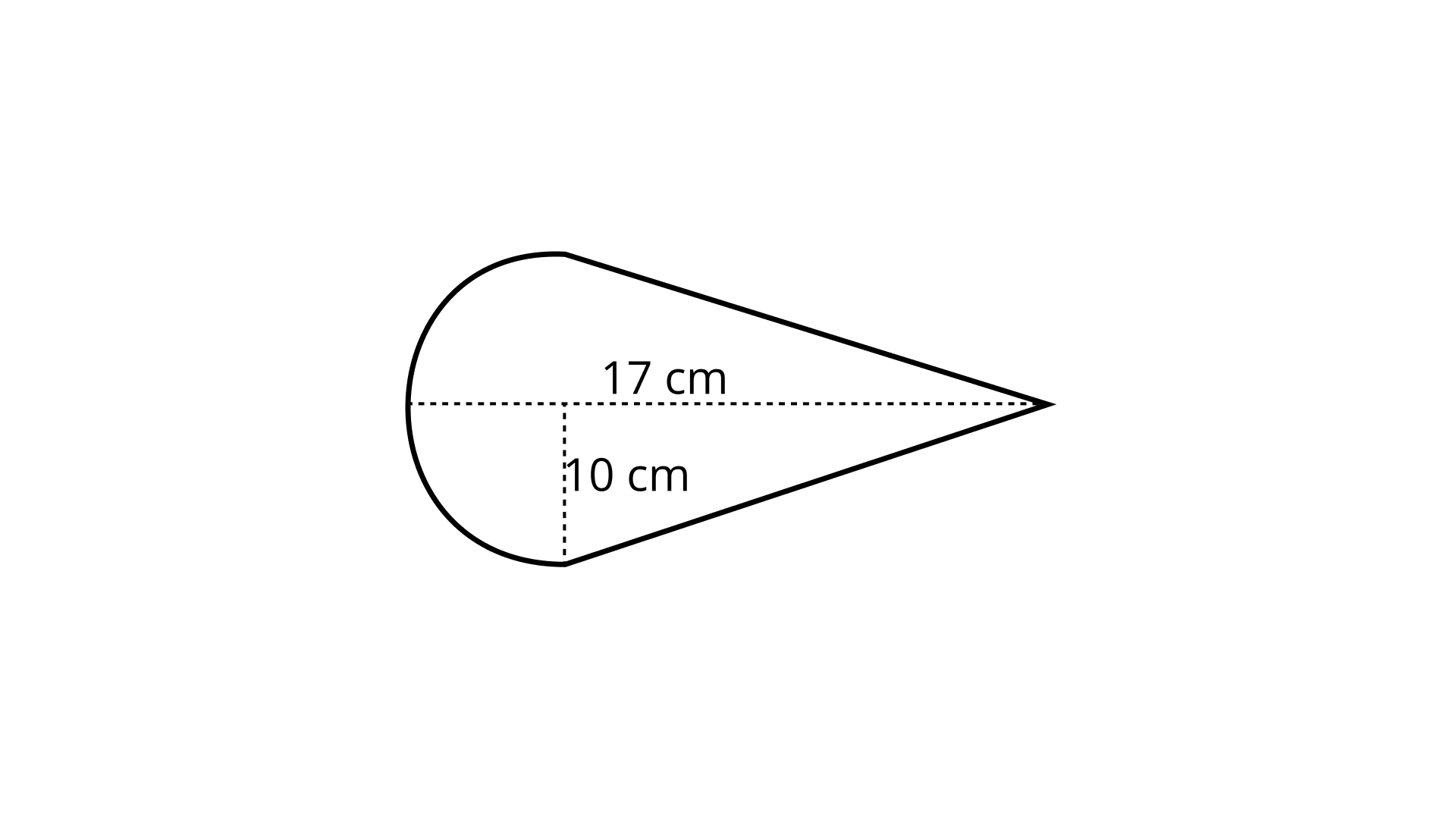
Ans: The Sample contains a semi-circle and a triangle.
Area of semi-circle
Now, area of triangle
Therefore, total area enclosed in shape
In Questions 98 and 99 find the areas of the shaded region:
98.
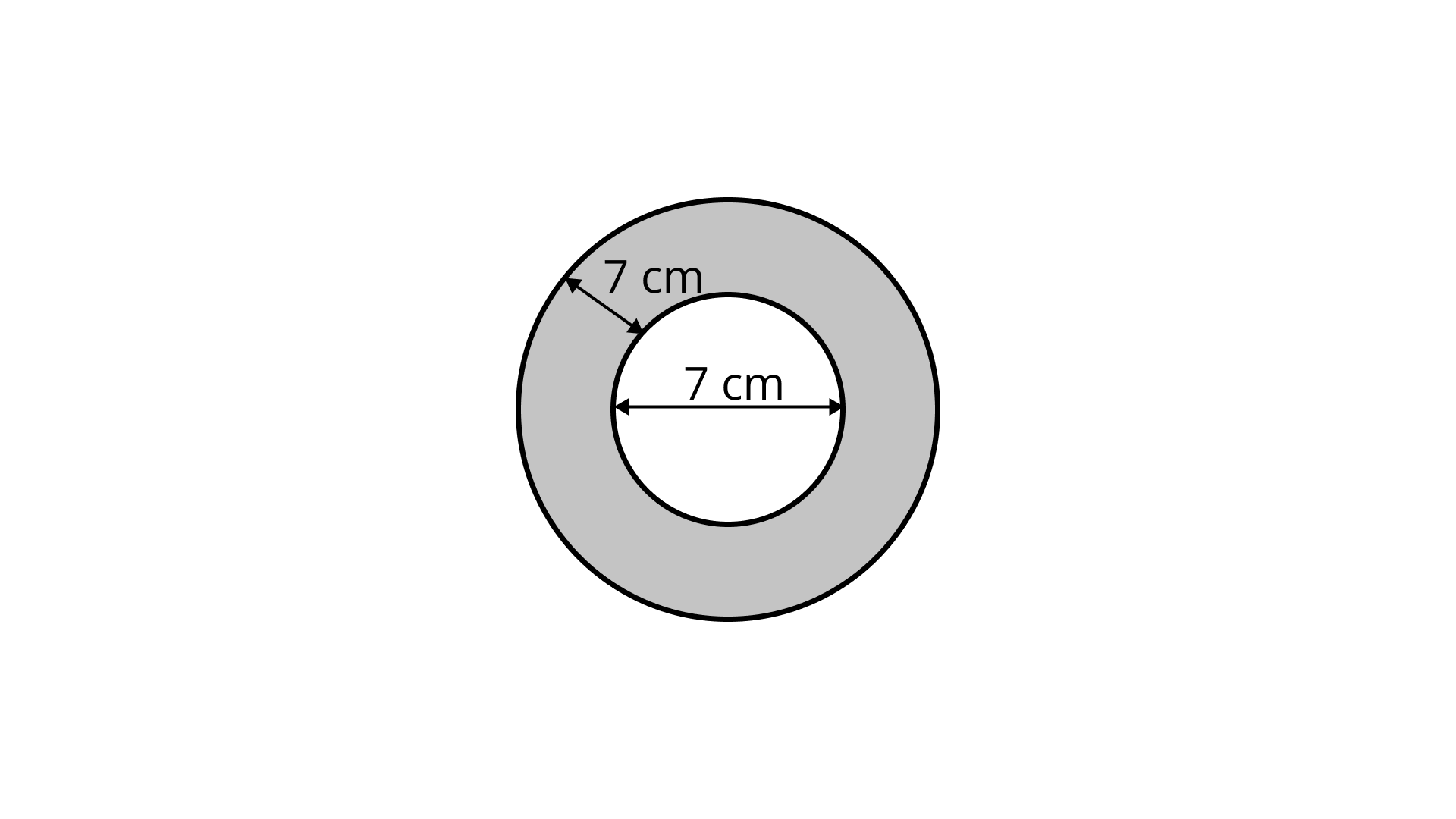
Ans: Let
From the figure,
Now, area of shaded region
Therefore,
99.
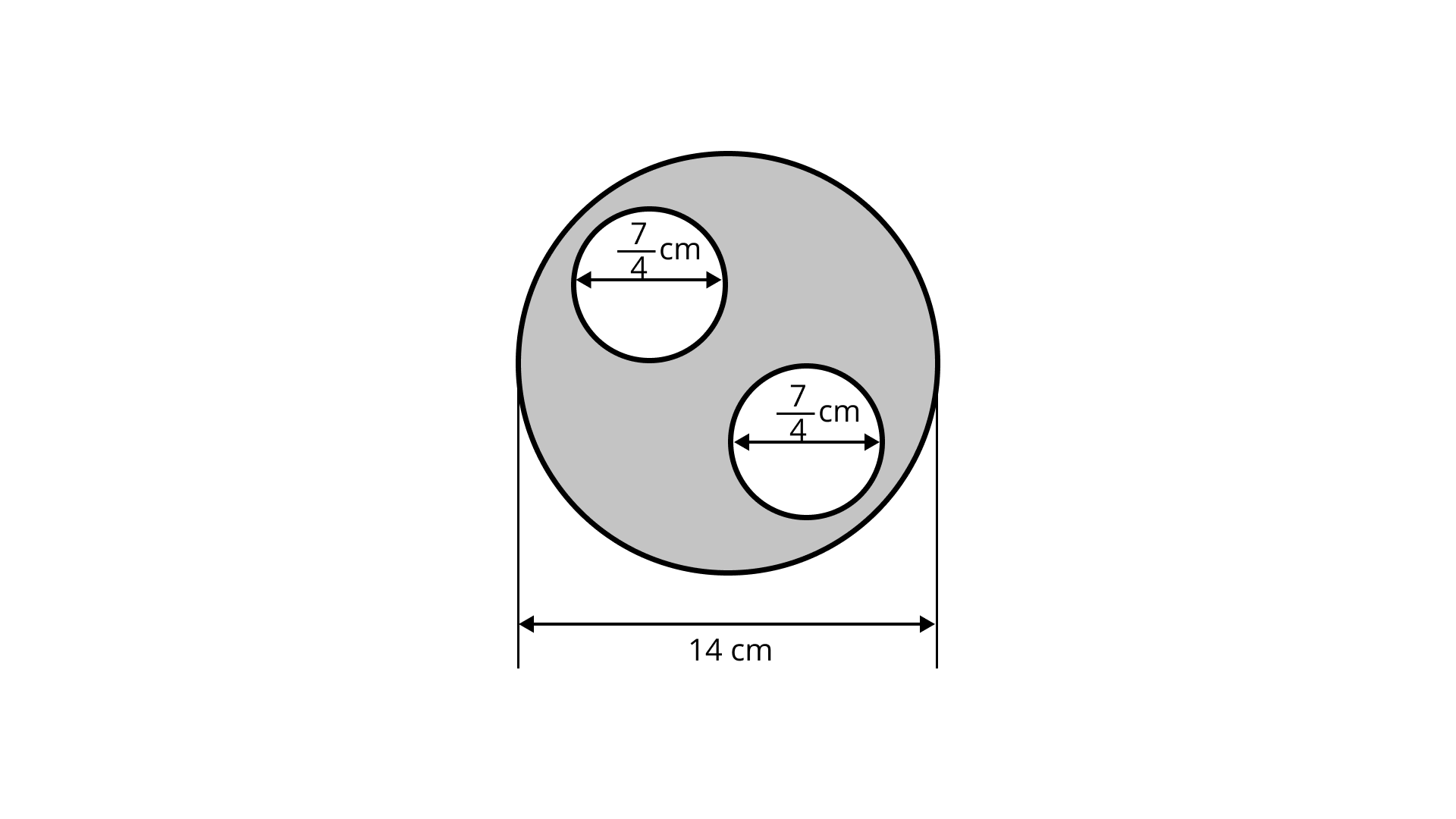
Ans: Diameter of the complete circle
Radius
From the figure,
Area of the complete circle
Diameter of the smaller circle
Area of two smaller circles
Now, area of shaded portion = area of complete circle area of two smaller circles
Therefore,
100. A circle with radius
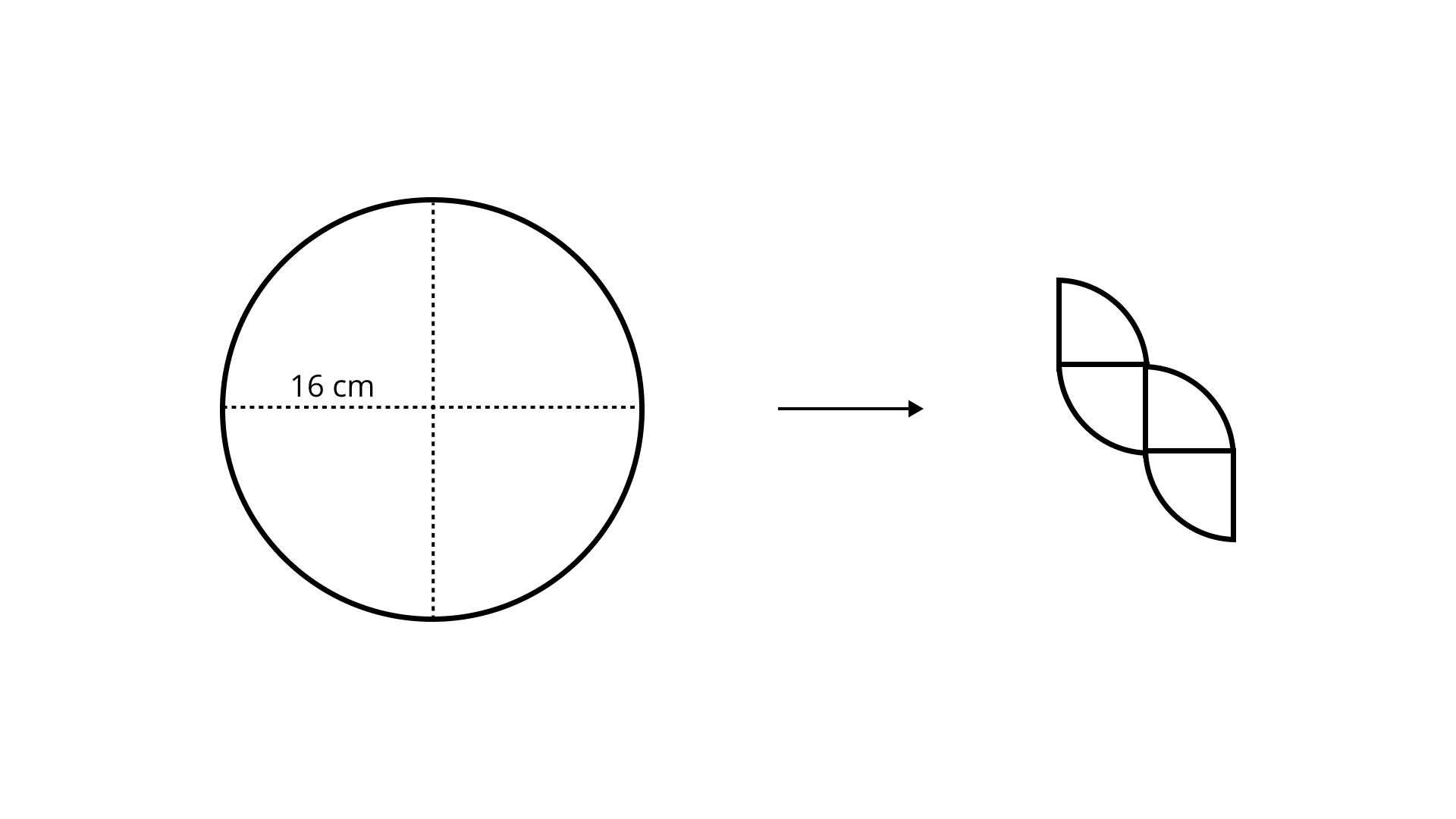
Does the perimeter change? If it does change, by how much does it increase or decrease?
Ans: Yes, the perimeter changes.
Radius of circle
Perimeter of the circle
Perimeter of new figure
= Perimeter of circle
Now the increase in perimeter
101. A large square is made by arranging a small square surrounded by four congruent rectangles as shown in Fig. If the perimeter of each of the rectangles is
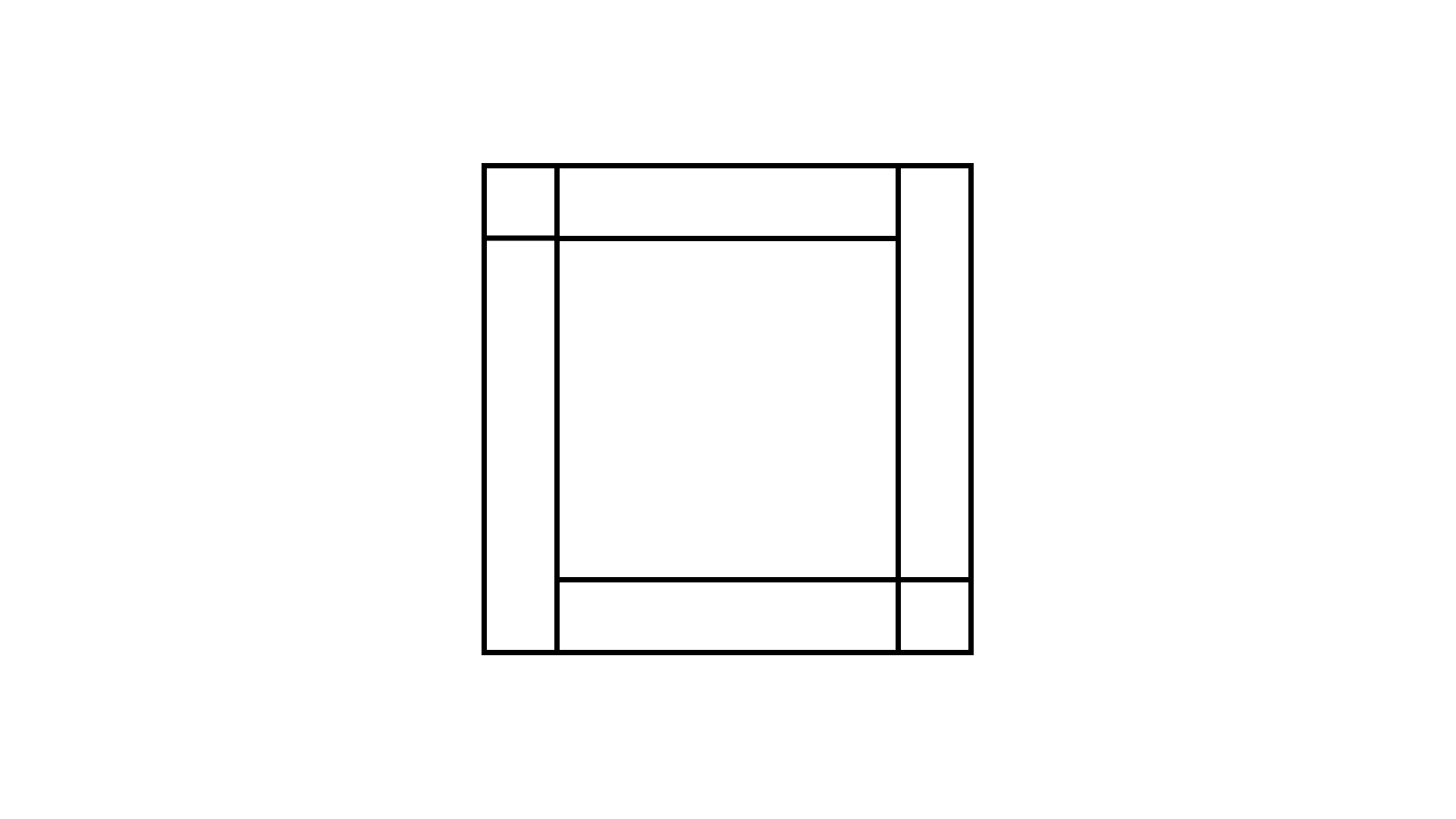
Ans:
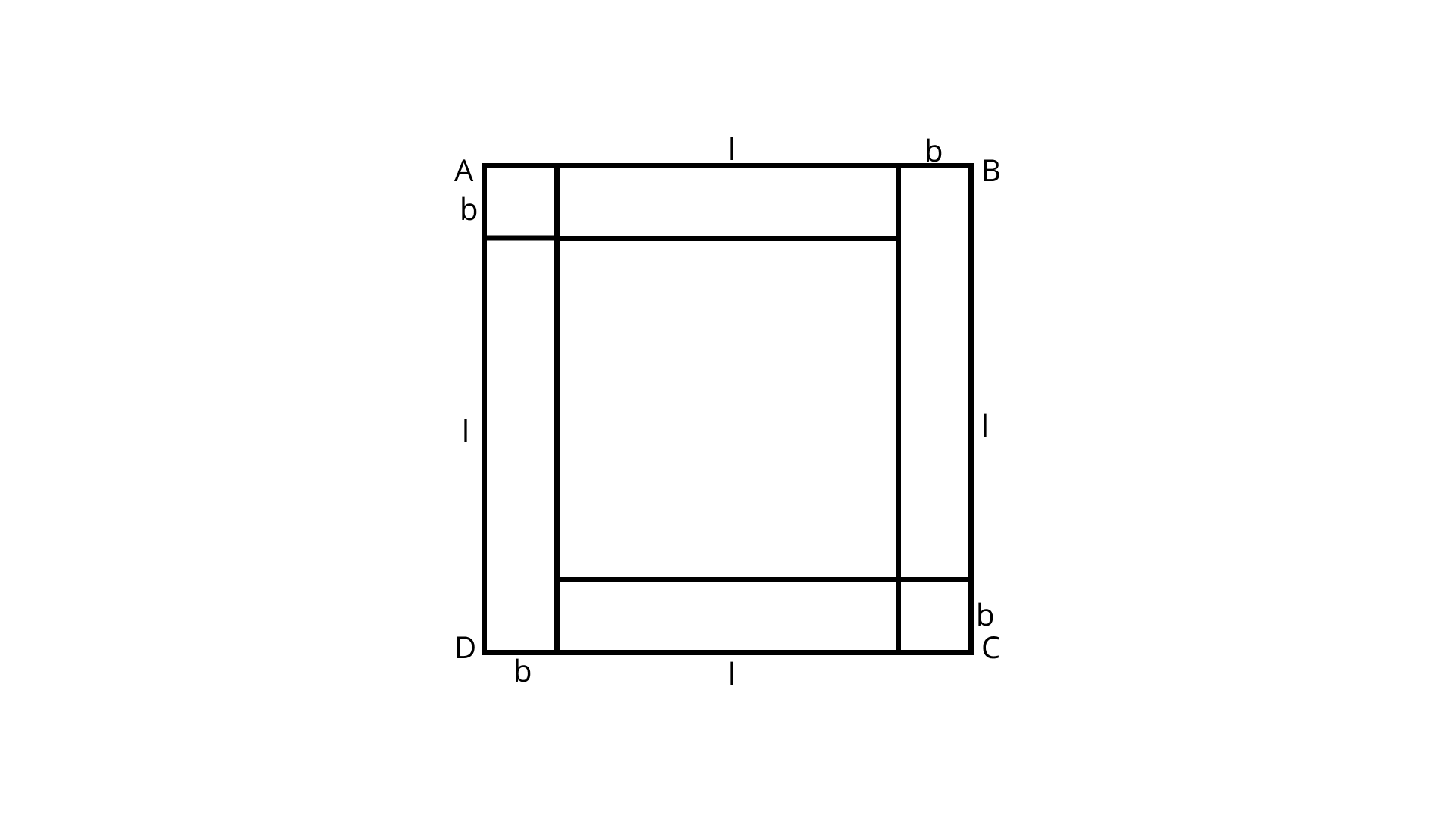
Let
Given, perimeter of one rectangle
As the side of larger square is
Therefore, area
102.
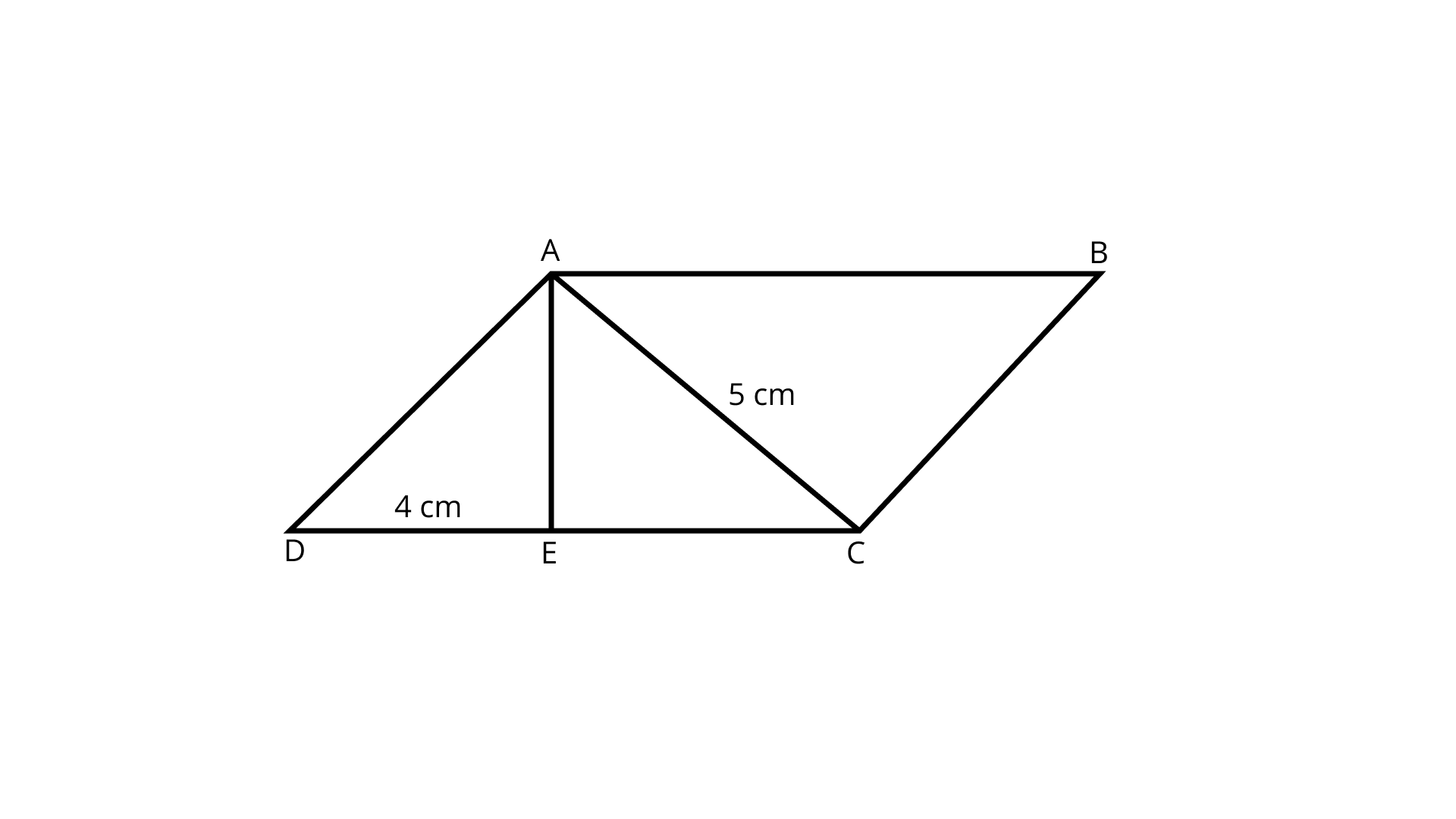
Ans: Given that,
Area of triangle
Area of triangle
Now, area of right-angled triangle
Since,
Now, applied Pythagoras theorem in triangle
Thus,
Perimeter of parallelogram
Area of parallelogram
103. Ishika has designed a small oval race track for her remote control car. Her design is shown in the figure 9.55. What is the total distance around the track? Round your answer to the nearest whole cm.
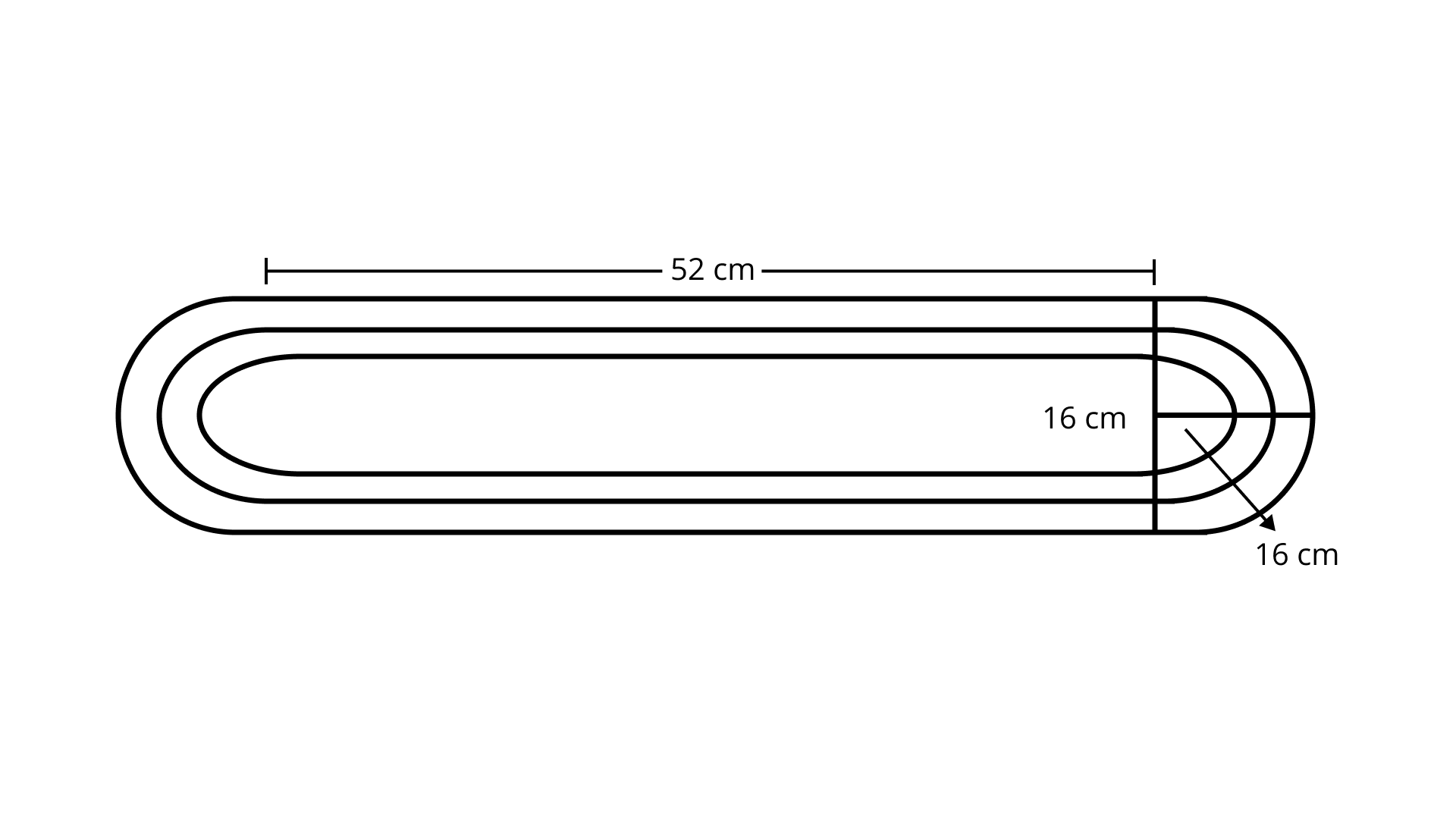
Ans: Total distance around the track
104. A table cover of dimensions
Ans:
To find the cost of polishing the table top, we have to find out its area,
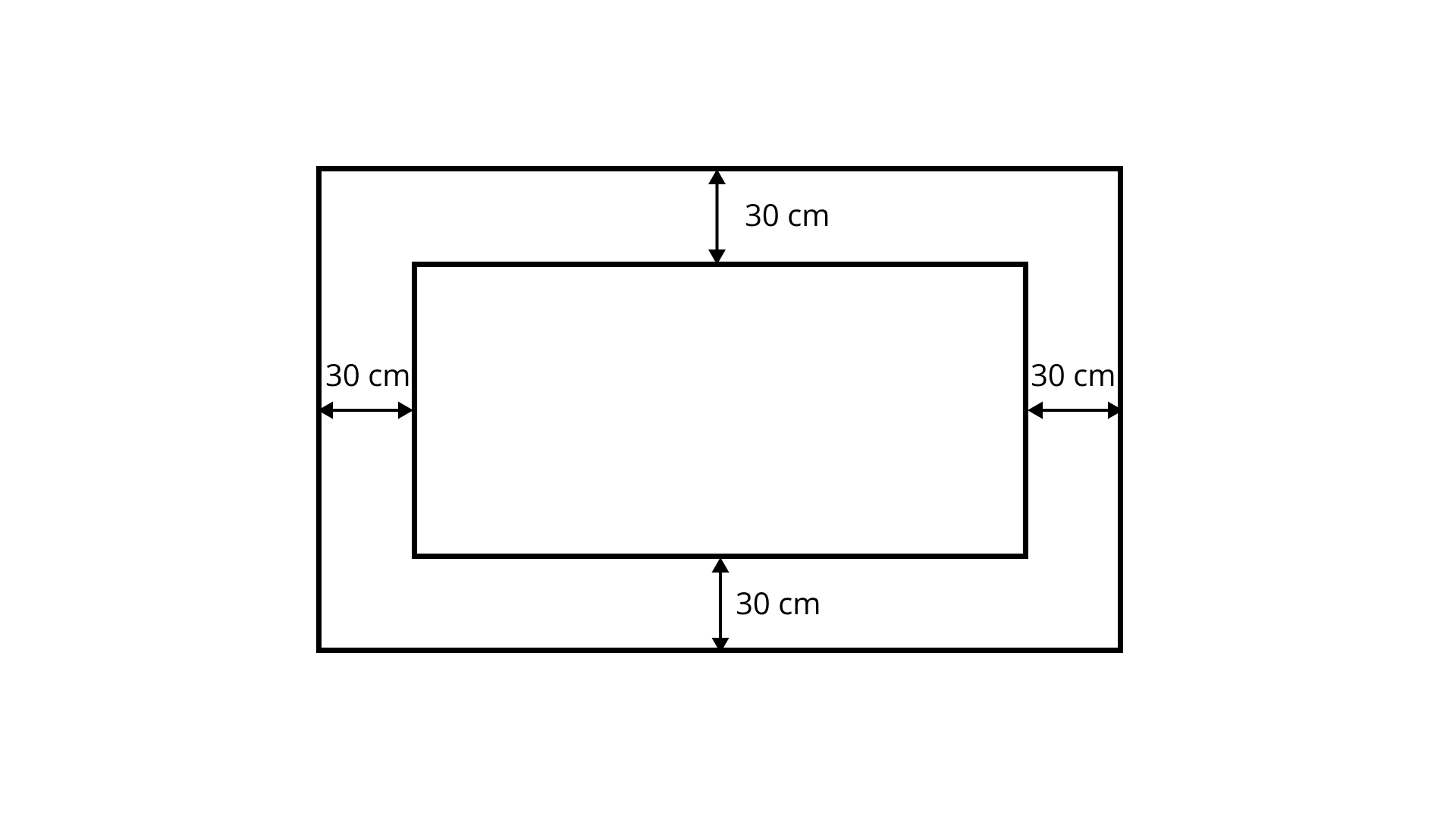
Given,
Length of cover
Breadth of cover
Now, area of the table cover
Given,
Hence, length of table
Breadth of table
Now, area of the top of the table
Now,
Area of hanging cover of table
It has been given that Rs.
of polishing the table.
105. The dimensions of a plot are
Ans:
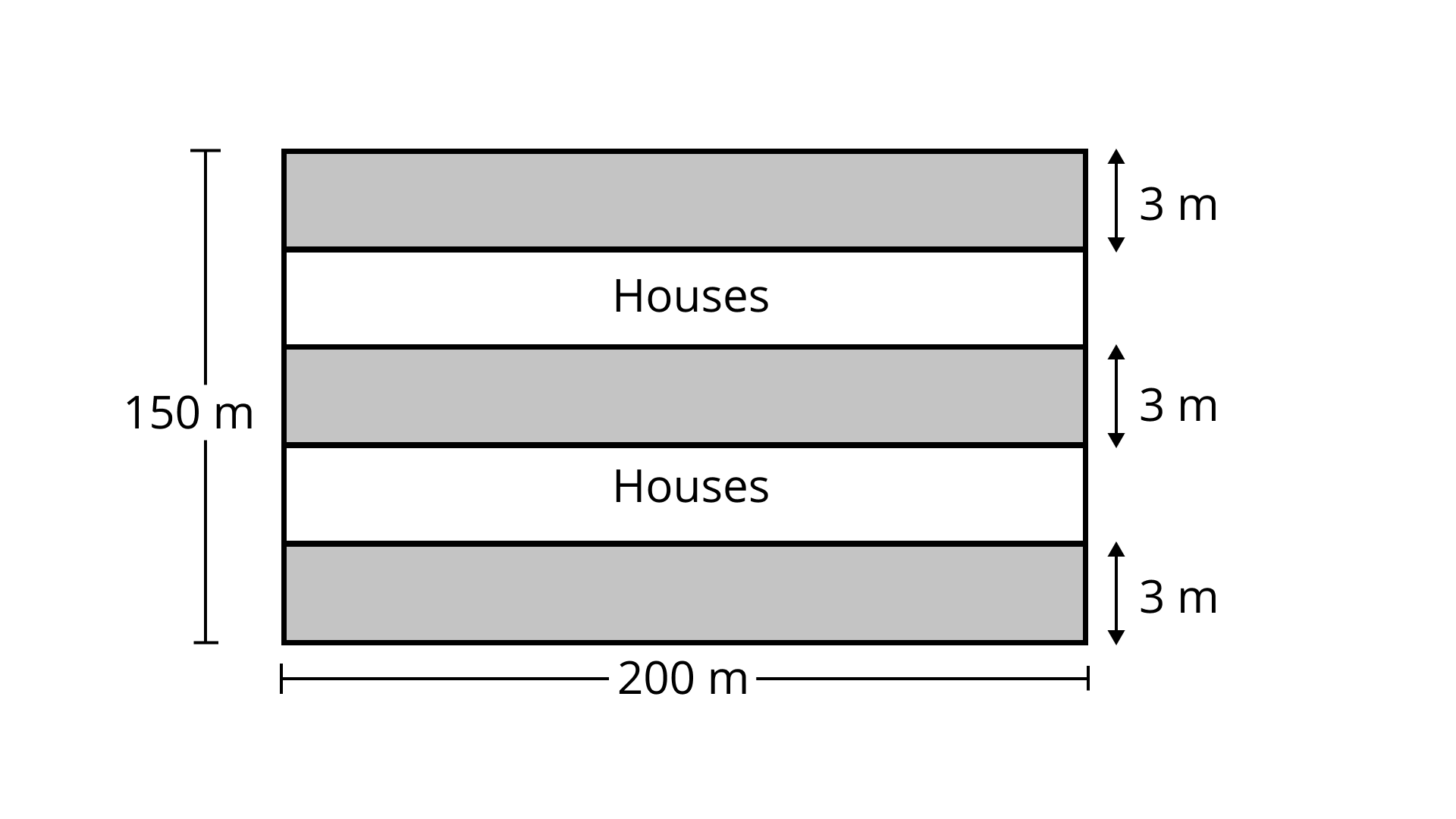
Given,
Dimension of plot
Width of road
Total area required for houses
106. A room is
Ans: Given that,
Length of room
Size of tiles
Width of room
Area of room
Area of one tile
Number of tiles =
Therefore, cost of covering the floor with tiles
107. Find the total cost of wooden fencing around a circular garden of diameter
Ans: Given,
Diameter of circular garden
Length of the fencing
Total cost of fencing
108. Priyanka took a wire and bent it to form a circle of radius
Ans: Given that,
Radius of circle
Length of rectangle
Hence, length of the wire
Let
Since, the wire is rebent in the form of rectangle
Hence, perimeter of rectangle
Area of rectangle
Therefore, the circle encloses more area than the rectangle.
109. How much distance, in metres, a wheel of
Ans: Given that,
Radius of wheel
Distance travelled in one rotation
Distance travelled in
Therefore, the wheel covers
110. A circular pond is surrounded by a
Ans:
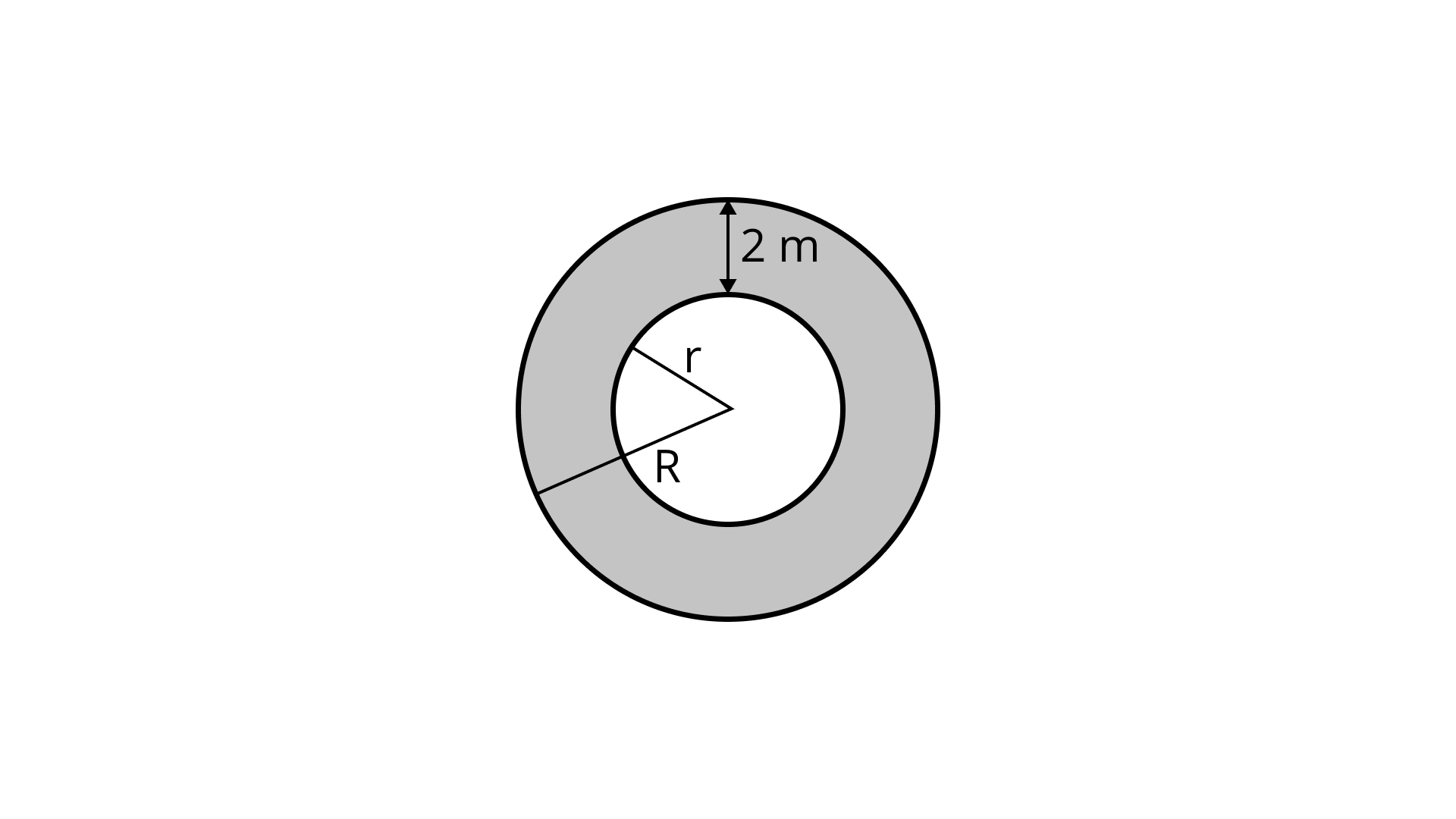
Let
It has been given that
Since,
Therefore, area of the path
111. A carpet of size
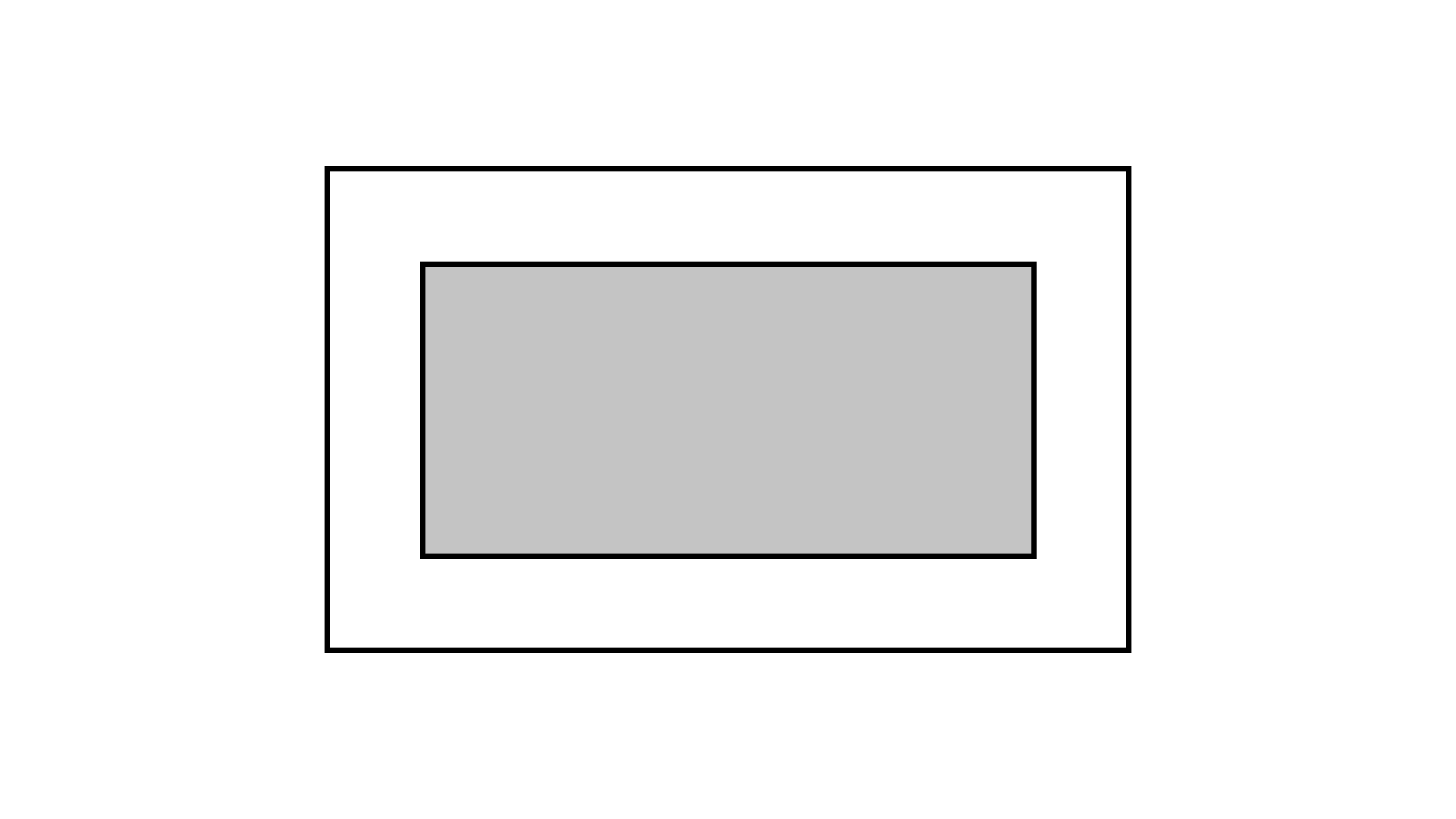
Ans:
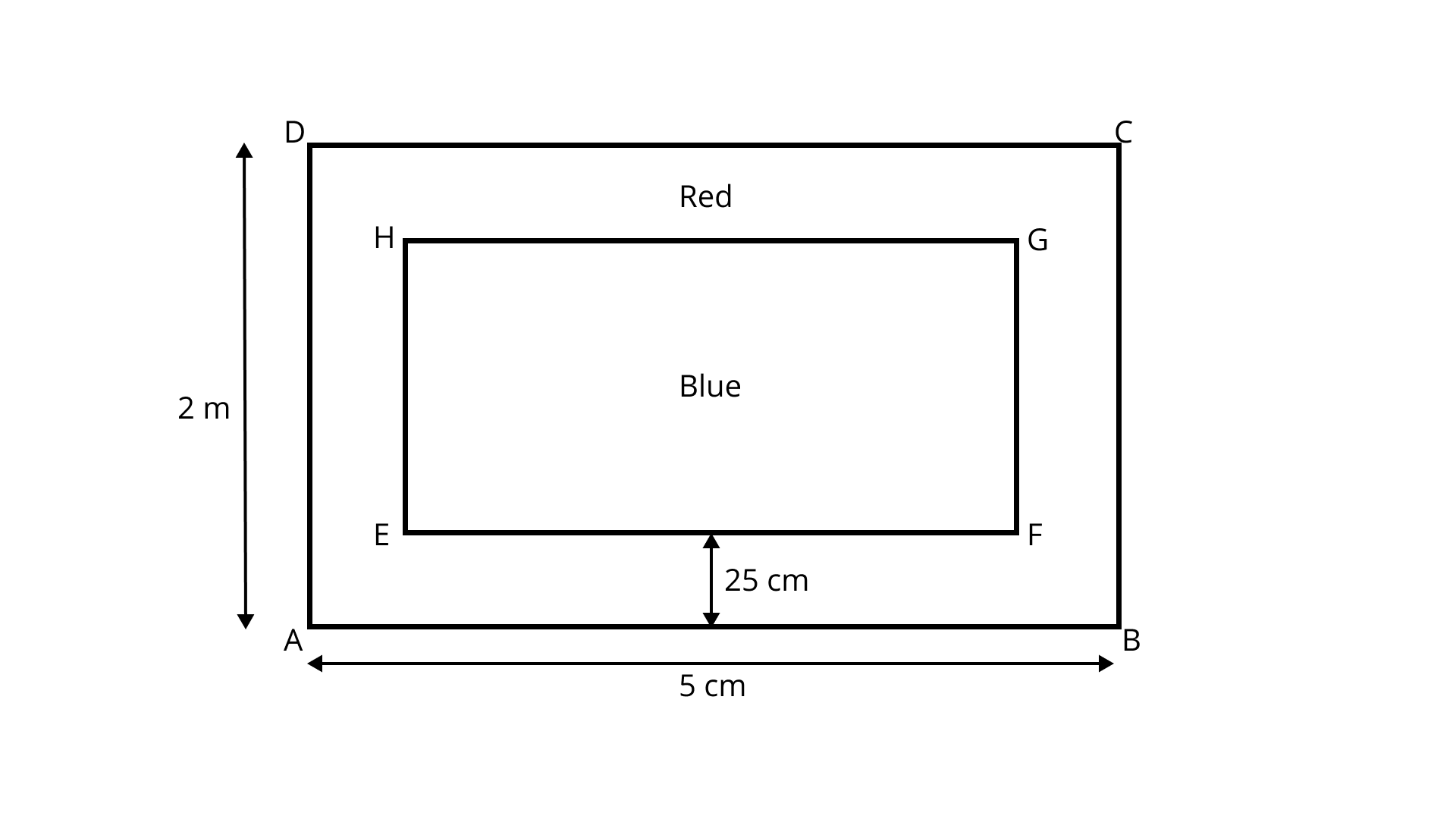
Given that,
Size of carpet
Width of border
Area of carpet
Thus, length of inner blue portion,
Breadth of inner blue portion
Area of blue portion
Now,
Area of red portion
Therefore, the ratio of area of red and blue portions
112. Use the Fig. 9.57 showing the layout of a farmhouse:
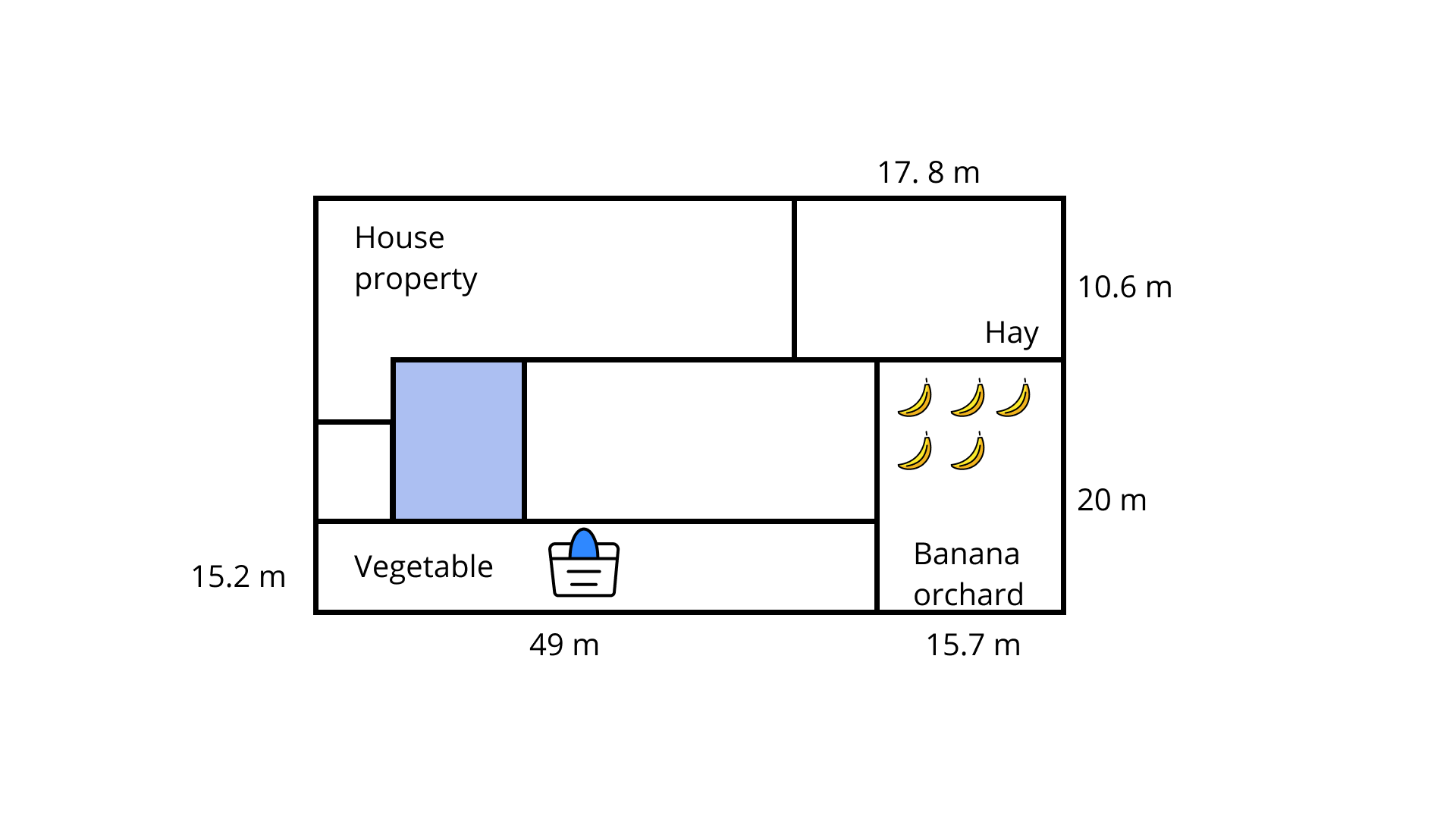
(a) What is the area of land used to grow hay?
Ans: Area of land used to grow hay
(b) It costs ₹
Ans: Area of vegetable garden
Since, cost to fertilise
Hence, cost to fertilise
(c) A fence is to be enclosed around the house. The dimensions of the house are
Ans: Fence is of dimension
Perimeter of the house
Hence, total lengths of the fence
(d) Each banana tree required
Ans: Area covered by banana orchard =
Since,
113. Study the layout given below in Fig. 9.58 and answer the questions:
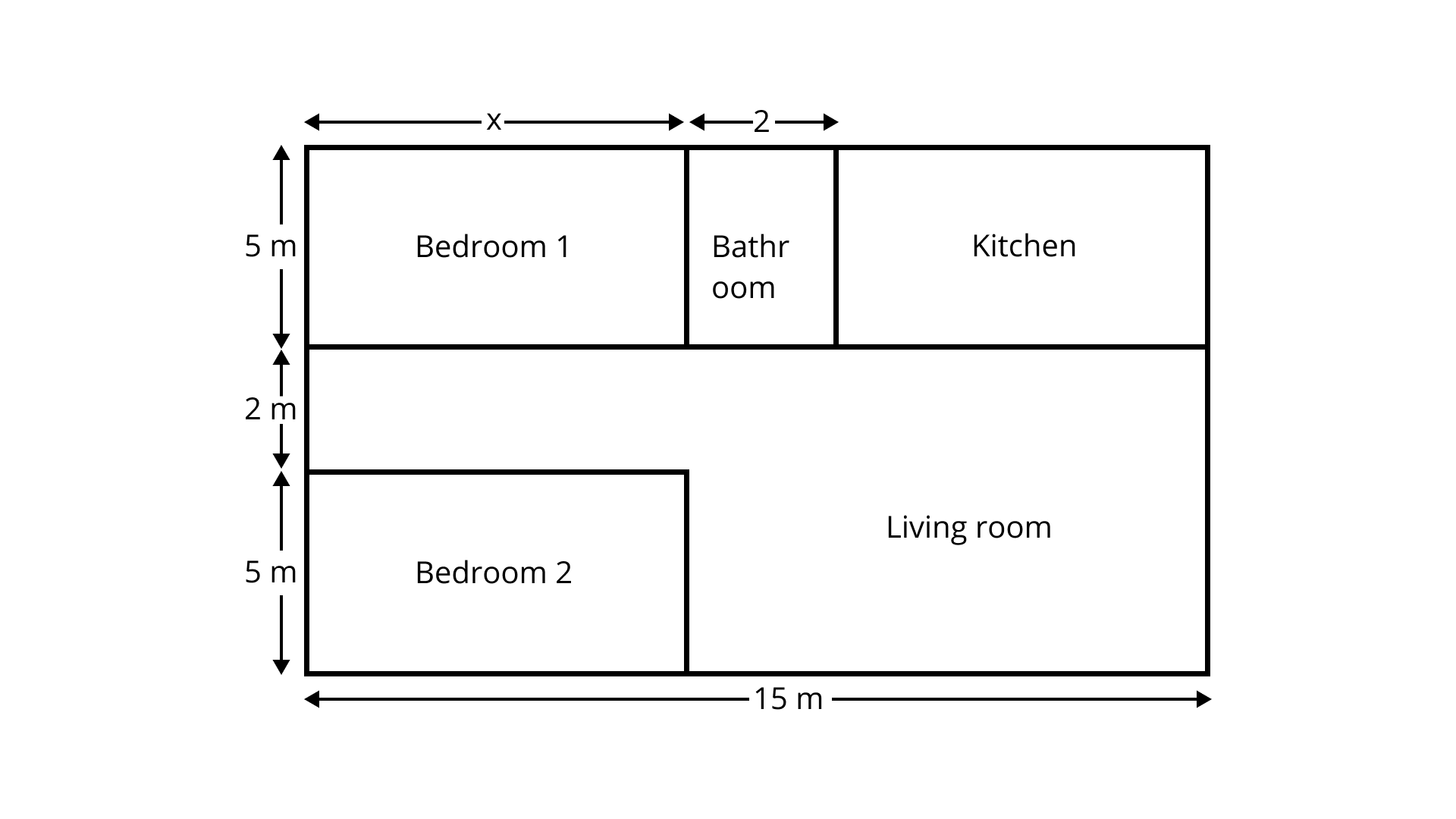
(a) Write an expression for the total area covered by both the bedrooms and the kitchen.
Ans: Area of both kitchen and bedrooms
(b) Write an expression to calculate the perimeter of the living room.
Ans: Perimeter of the living room
(c) If the cost of carpeting is Rs.
Ans: Total area of both living the room and the bedrooms
Therefore, total cost of carpeting
(d) If the cost of tiling is Rs.
Ans: Total area of bathroom and kitchen
Therefore, total cost of tilling
(e) If the floor area of each bedroom is
Ans: Given,
Area of floor of each bedroom
Area of one bedroom
114. A
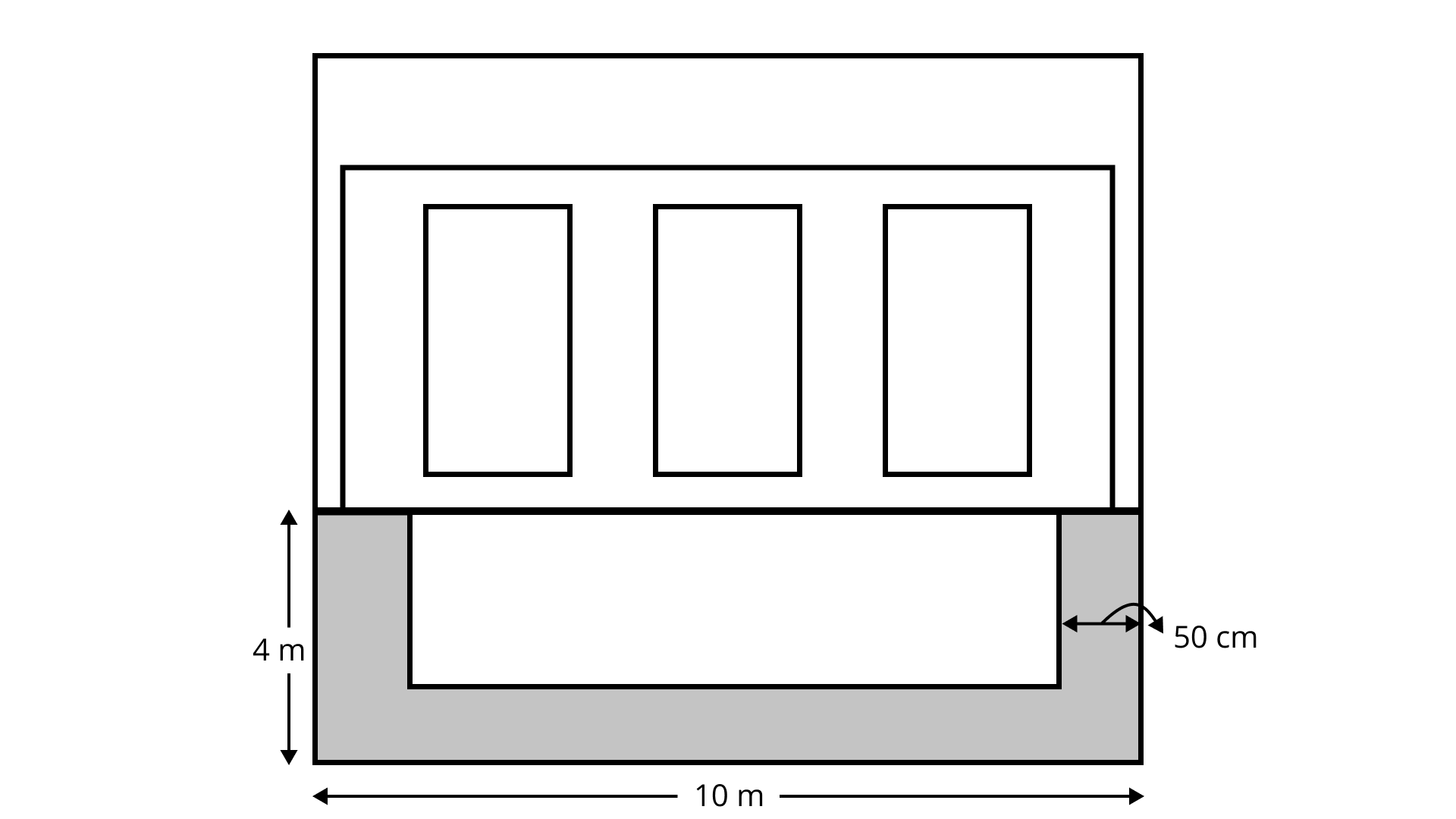
Ans:
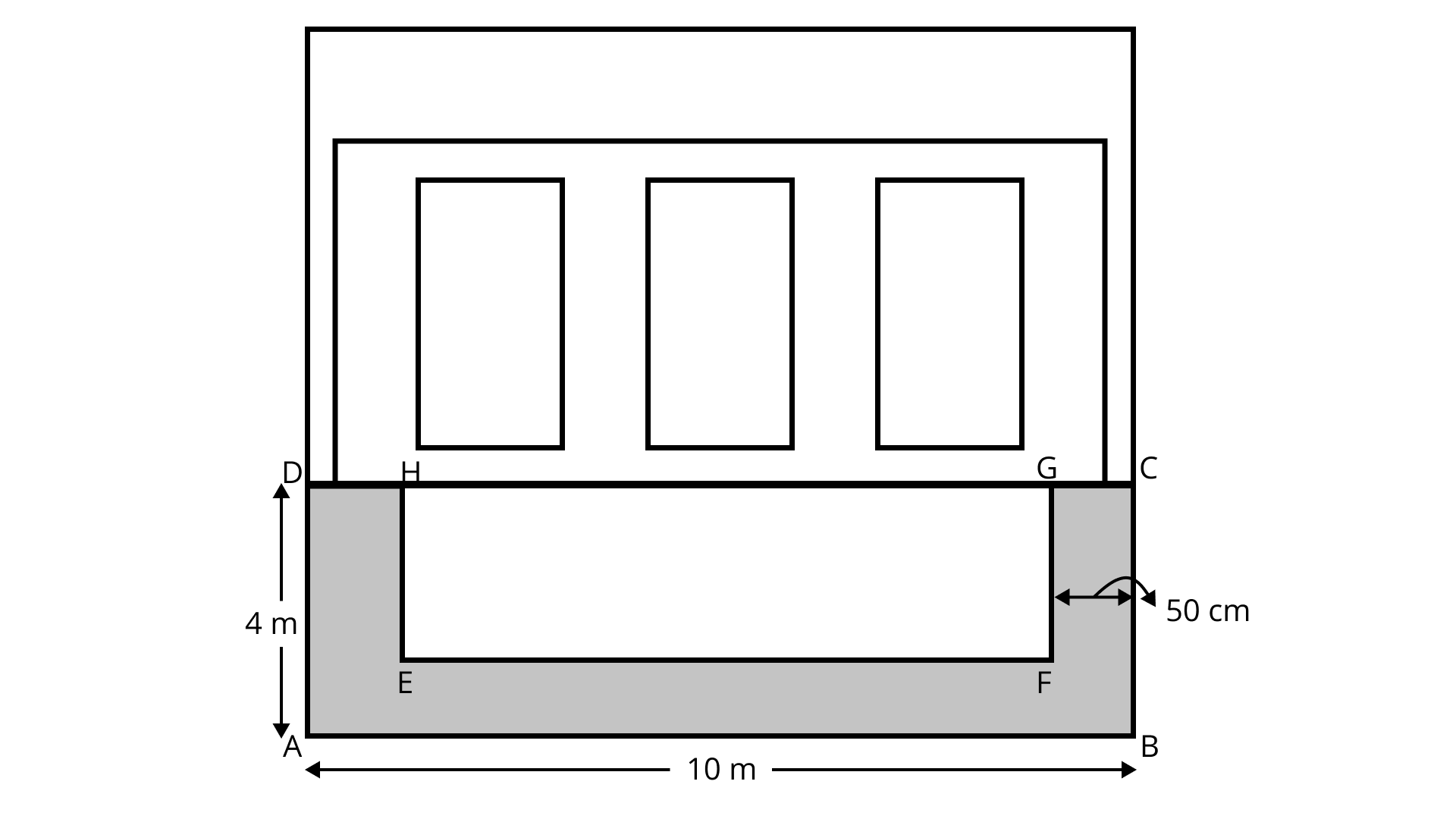
Given that,
Dimension of rectangular lawn
Width of flowerbed
Length of remaining portion
Breadth of remaining region,
Therefore, required area
115. A school playground is divided by a
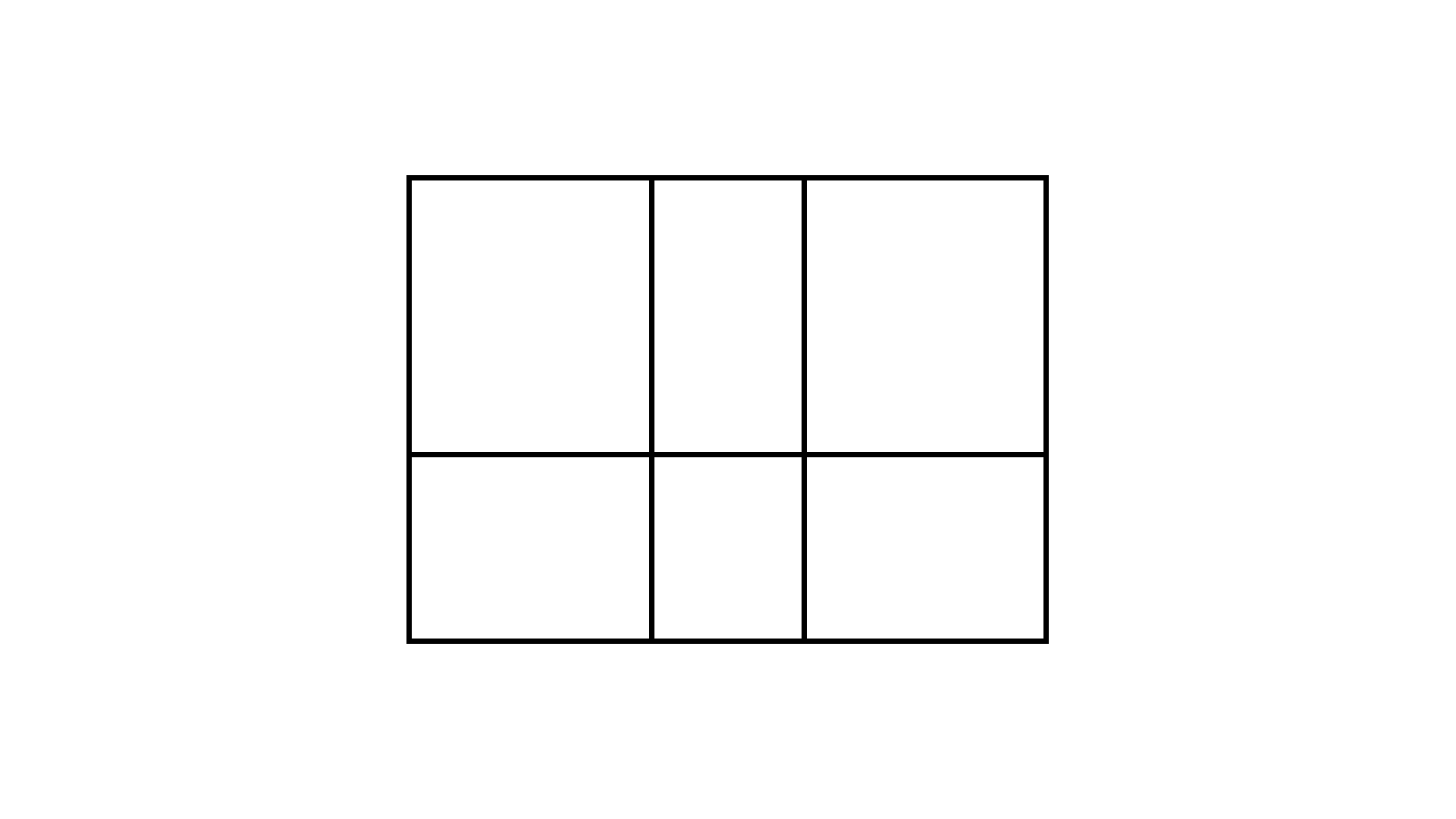
Ans:
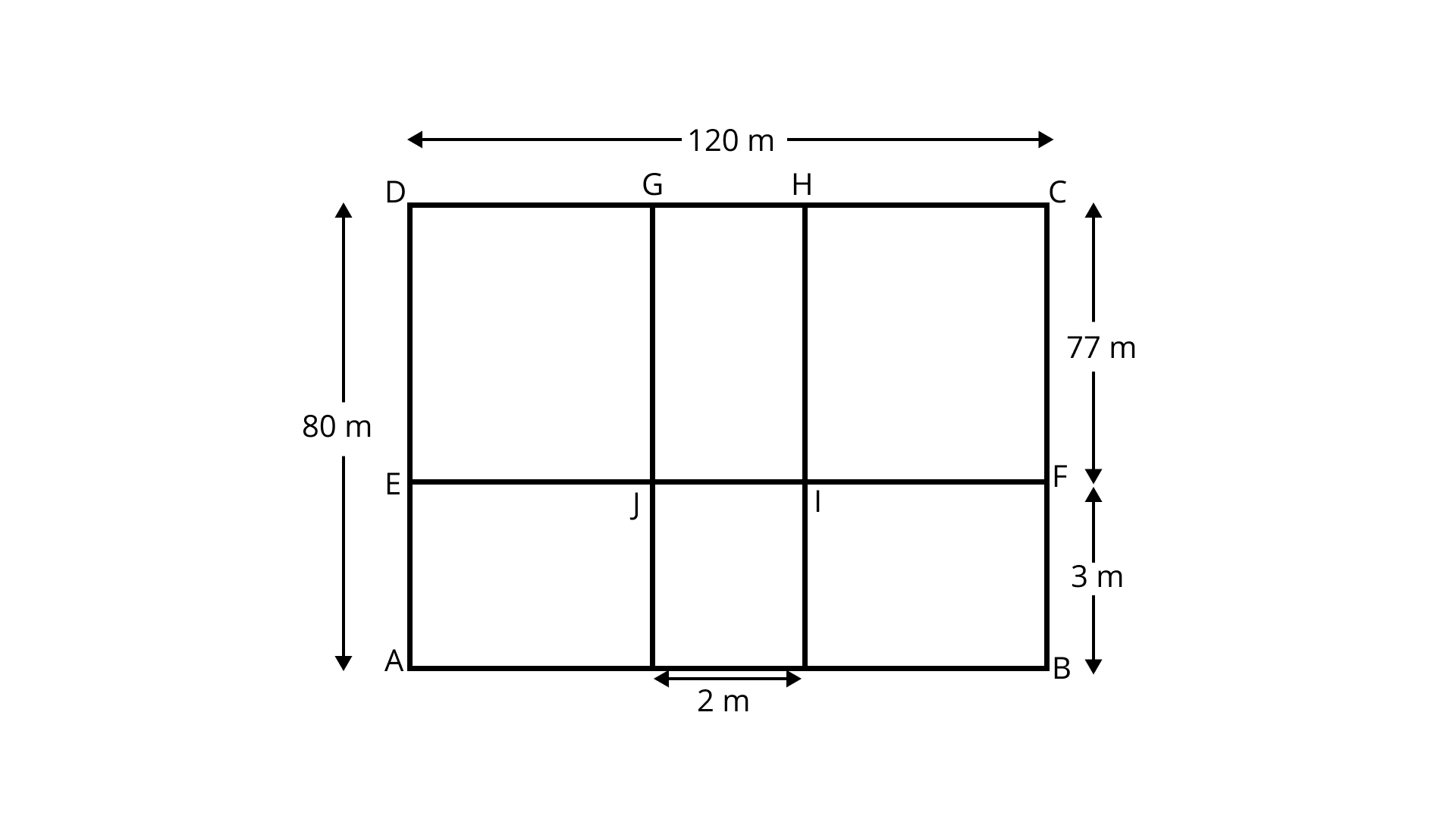
Given that,
Dimension of playground
Area of rectangle
Area of rectangle
Area of rectangle
Area of Remaining ground rectangle
116. In a park of dimensions

Ans:
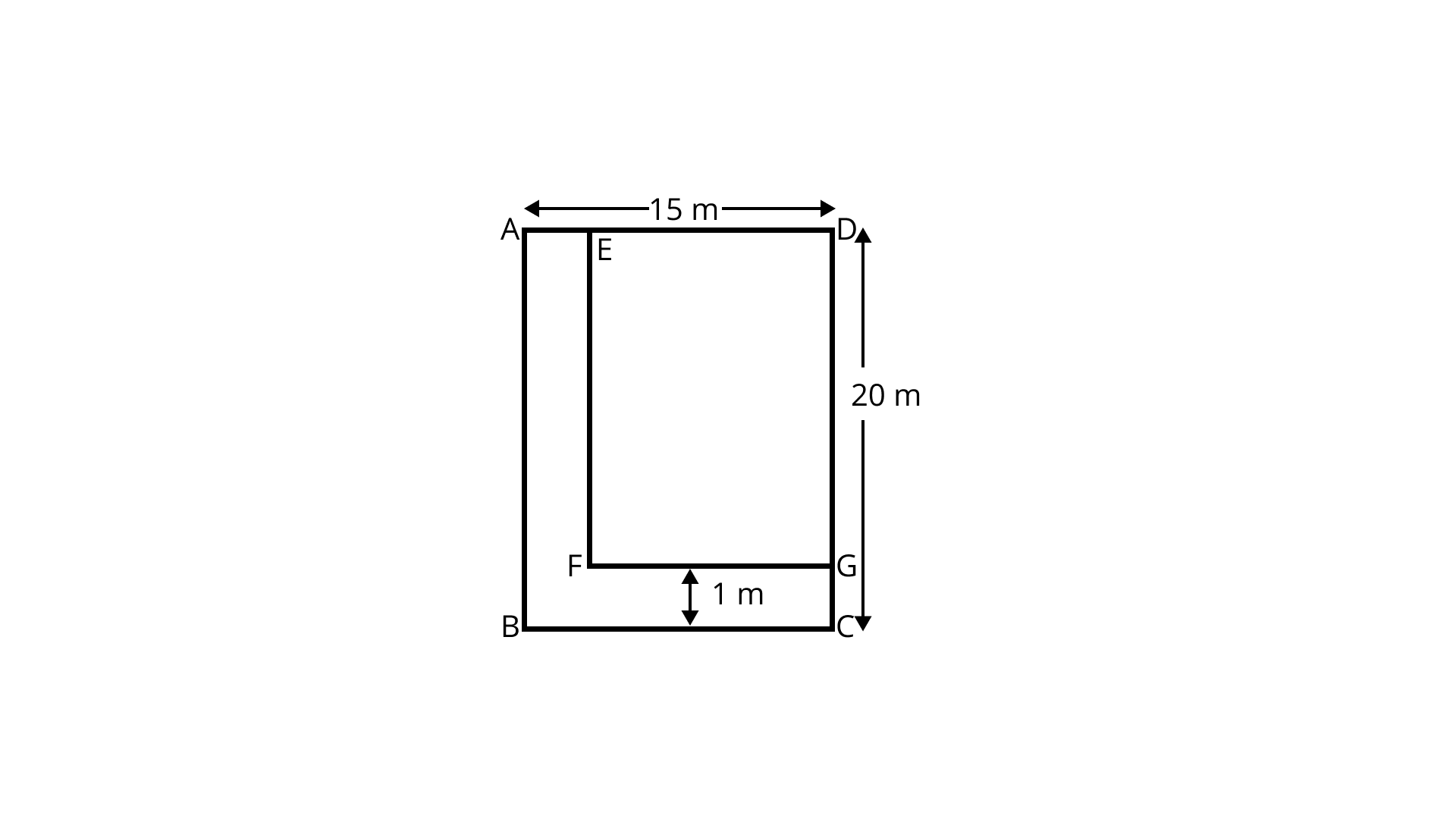
Given,
Dimensions of a given park
Width of flowerbed
From the figure,
Therefore, area of flower bed = Area of rectangle
Rs.
Therefore, the cost of manuring of
117. Dimensions of a painting are
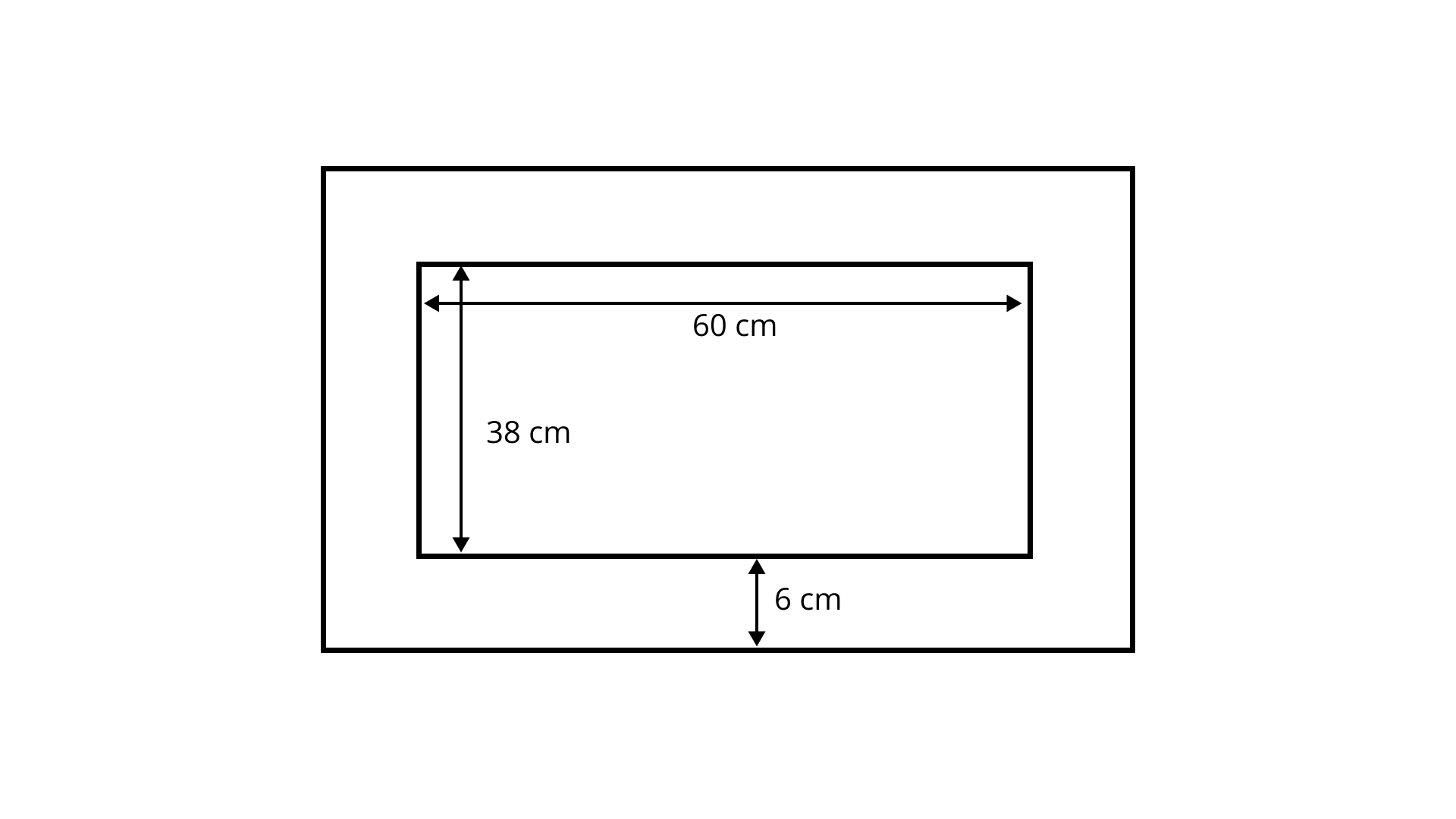
Ans: Given,
Length of inner rectangle
Breadth of inner rectangle
Thus, area of inner rectangle
Length of outer rectangle
Breadth of outer rectangle
Thus, area of outer rectangle
Now,
Area of wooden frame
118. A design is made up of four congruent right triangles as shown in Fig. 9.63. Find the area of the shaded portion.
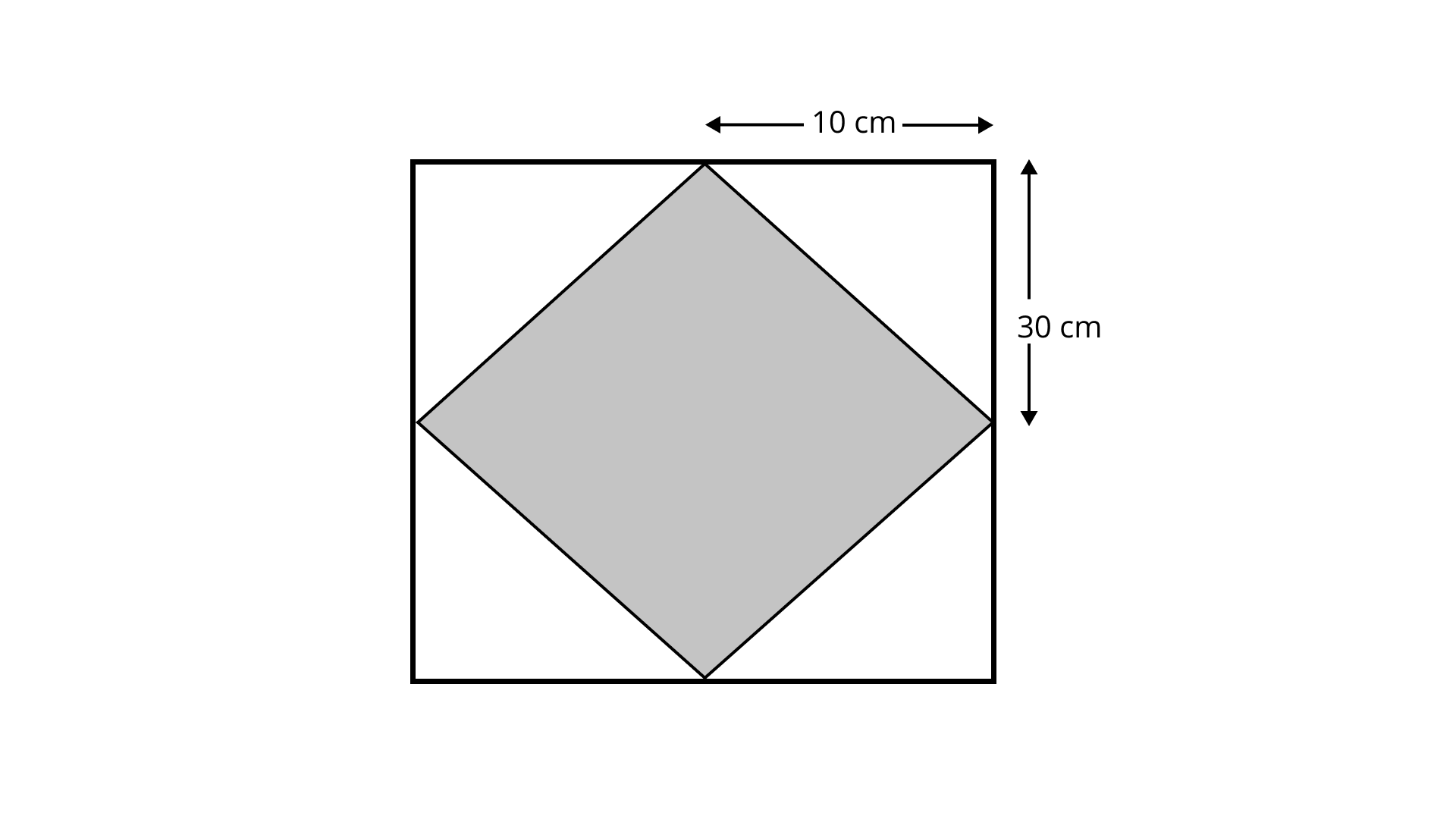
Ans: Area of one right-angled triangle
Thus, area of 4 right-angled triangles
Area of portion
Therefore, area of shaded portion
119. A square tile of length

Ans: Figure 1
Area of shaded portion
Figure ii
Area of shaded portion =
Therefore, both cases have equal areas.
120. A rectangular field is
Ans: Given,
Dimensions of field
Dimension of right-angled triangle
Therefore, number of right-angled triangular flowerbeds
121. Ramesh grew wheat in a rectangular field that measured
Ans: Given,
Dimensions of field
Increased area
So, increased area of wheat field
Therefore, increased length of wheat field is
122. In Fig. 9.65, triangle AEC is right-angled at E, B is a point on EC,
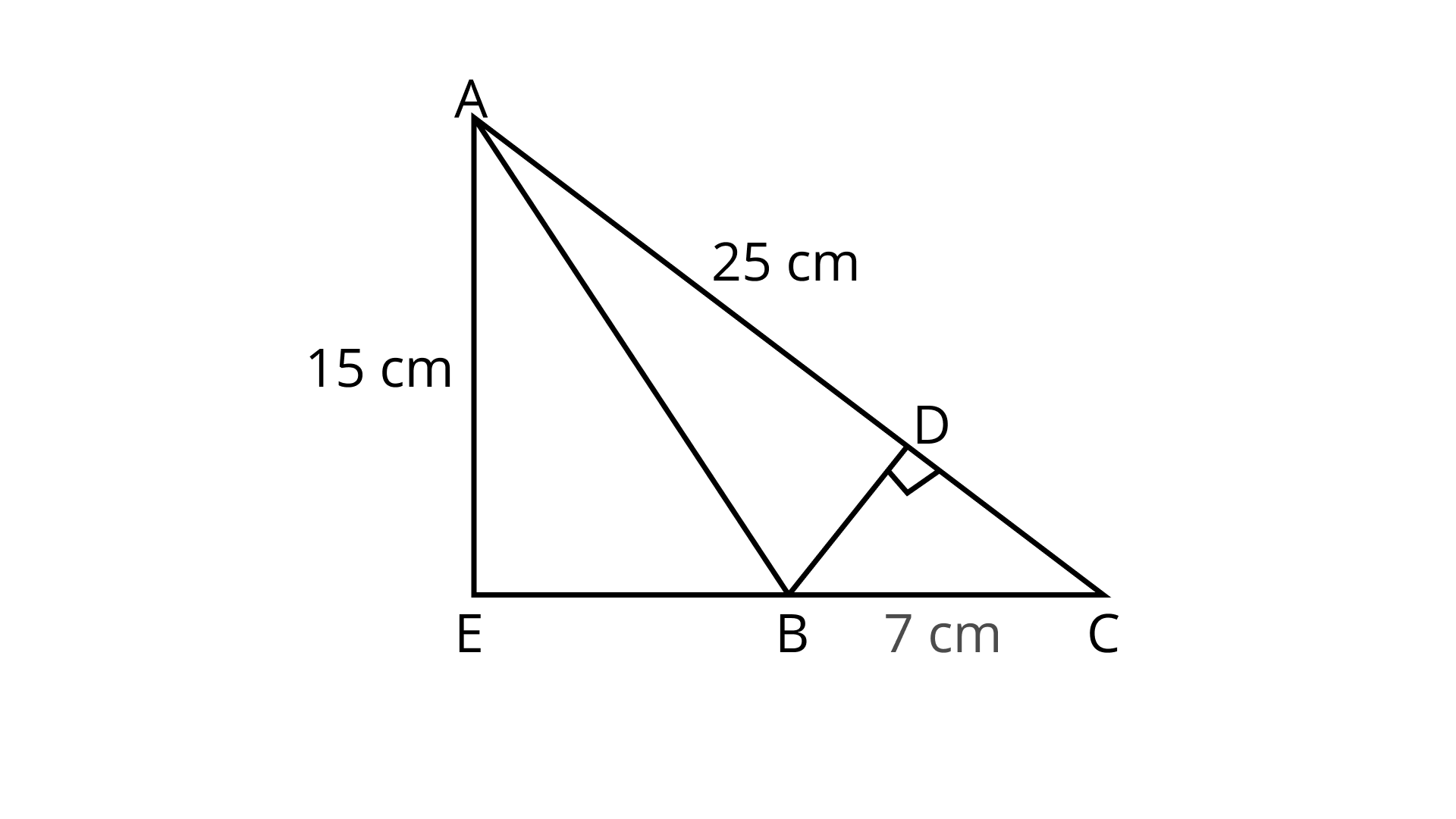
Ans: Given,
Applying Pythagoras theorem in triangle
Now,
Area of triangle AEC,
Now, Area of triangle AEB,
Thus, area of triangle
Now, again area of triangle ABC
Therefore,
123.

Ans: Number of pieces of chocolate=
Therefore, the number of pieces of chocolate are
124. Calculate the area of the shaded region in Fig. 9.66, where all of the short line segments are at right angles to each other and
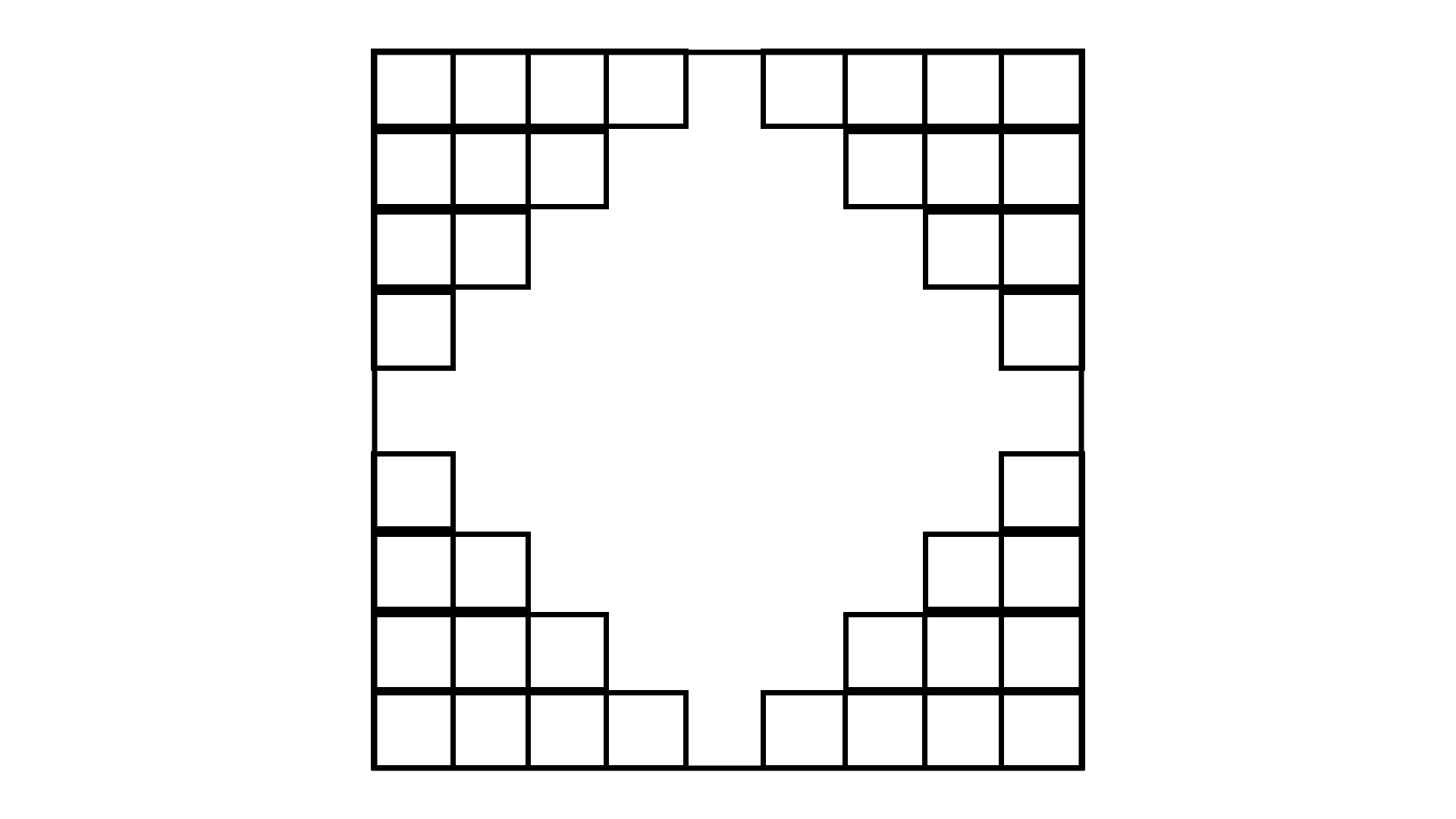
Ans: Length of the larger rectangle
Breadth of the larger rectangle
Thus, area of shaded portion
Thus, the area of the shaded portion is
125. The plan and measurement for a house are given in Fig. 9.67. The house is surrounded by a path
Find the following:
(i) Cost of paving the path with bricks at rate of ₹
Ans: Area of path = Area of rectangle PQRS - area of rectangle ADFH
Given,
By putting these values in equation (i);
Now,
Cost of paving the path with bricks
(ii) Cost of wooden flooring inside the house except the bathroom at the cost of ₹
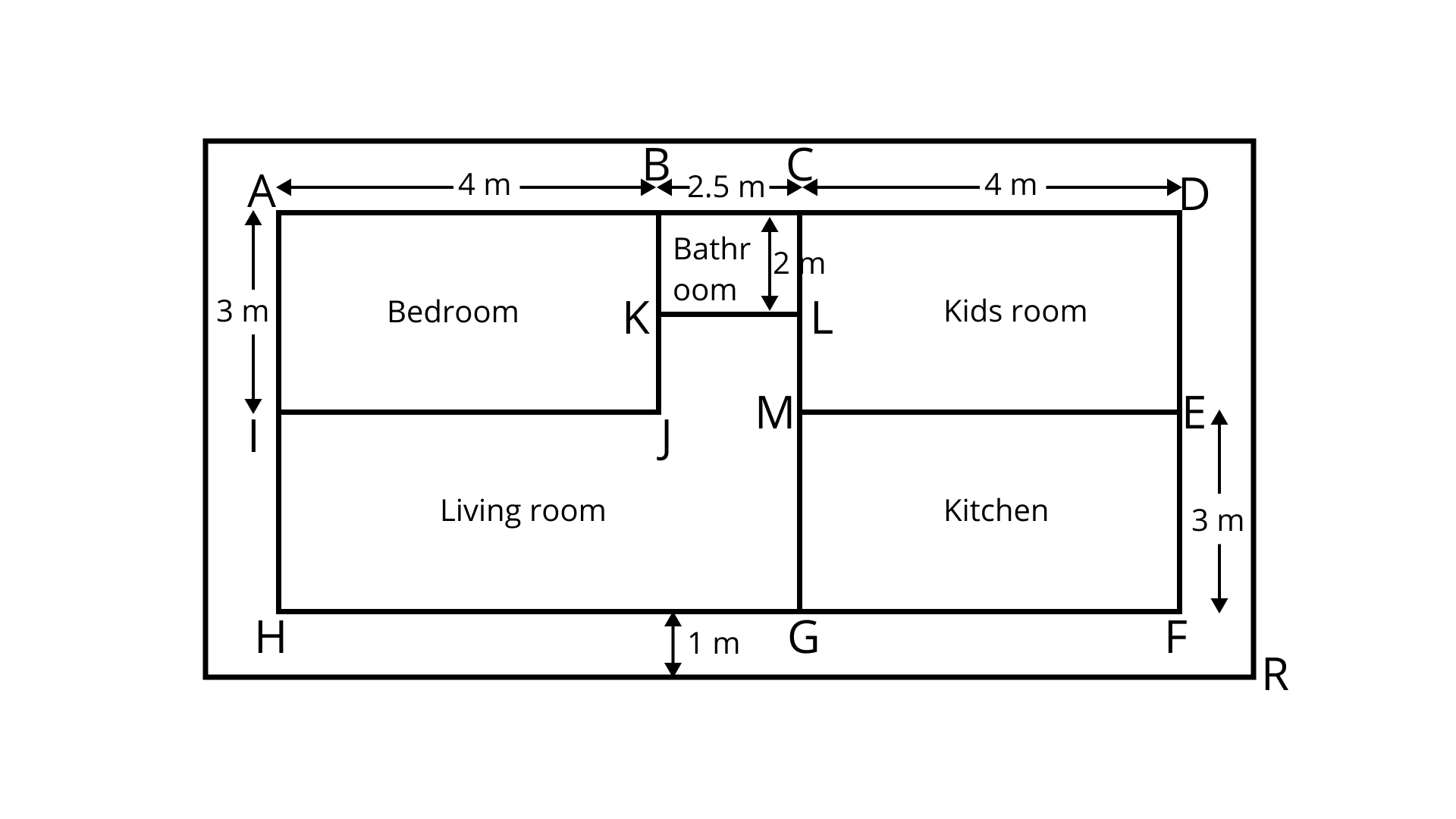
Ans: Area of house except bathroom = area of house
= Area of rectangle
Given, Area of rectangle
By putting these values in equation (ii);
Now,
The cost of flooring = cost per unit
(iii) Area of Living Room.
Ans: Area of living room
= Area of rectangle
Given, Area of rectangle
Area of rectangle
Area of rectangle
By putting these values in equation (iii), we get
126. Architects design many types of buildings. They draw plans for houses, such as the plan shown in Fig. 9.68:
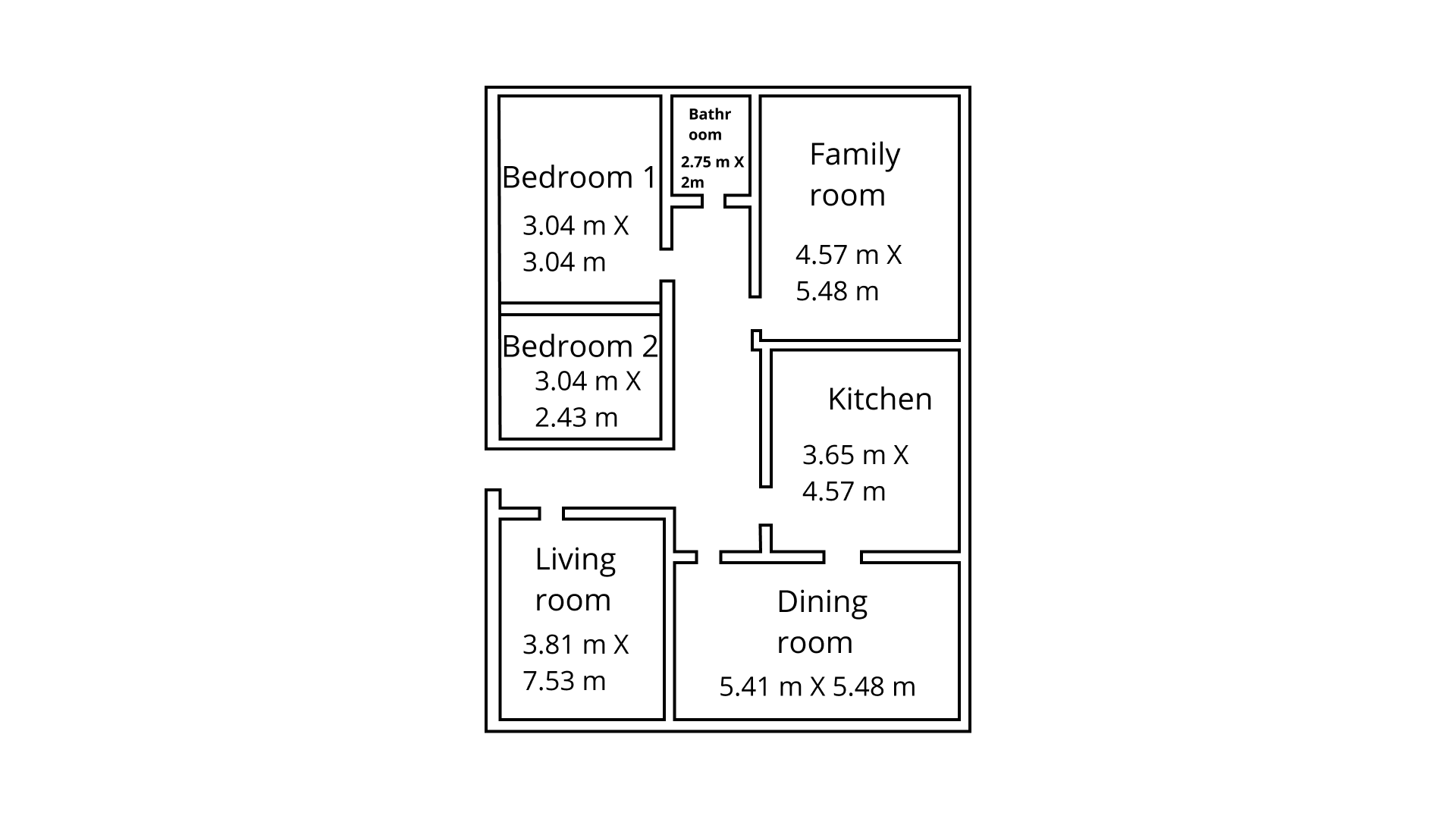
An architect wants to install decorative moulding around the ceilings in all the rooms. The decorative moulding costs Rs
(a) Find how much moulding will be needed for each room.
(i) family room
(ii) living room
(iii) dining room
(iv) bedroom 1
(v) bedroom 2
Ans:
(i) Perimeter of family room
Given, length
breadth
By putting these values in above formula, we get
ii. Perimeter of living room
breadth
By putting these values in above formula, we get
iii. Perimeter of dining room
By putting these values in above formula, we get
iv. Perimeter of bedroom
By putting these values in above formula, we get
v. Perimeter of bedroom
Given, length
By putting these values in above formula, we get
(b) The carpet costs Rs
Ans:
For bedroom 1,
Given, length of bedroom
Area of bedroom
Area of bedroom
Cost of carpeting
Cost of carpeting
For bedroom 2 ,
Given, length of bedroom
and breadth of bedroom
Area of bedroom
Cost of carpeting
Cost of carpeting
For living room,
Given, length of living room
breadth of living room
Area of living room
Cost of carpeting of living room
Cost of carpeting
For dining room,
Given, length of dining room
Area of dining room
Cost of carpeting
Cost of carpeting
For the family room,
Given, length of family room
Area of family room
Therefore, cost of carpeting family room
(c) What is the total cost of moulding for all the five rooms.
Ans: Total perimeter of all the five rooms,
Given, cost of moulding each room = Rs. 500 per m
Now, the total cost of moulding all 5 rooms
127.
Ans: Here,
Now,
Area of triangle APS
Area of portion PQRS = Area of rectangle ABCD -4 times of triangle APS
Area of circular rangoli,
Area of shaded portion,
128. 4 squares each of side
Ans: Area of each square
Area of rectangular sheet
Area of an isosceles right triangle
Area of remaining part of paper
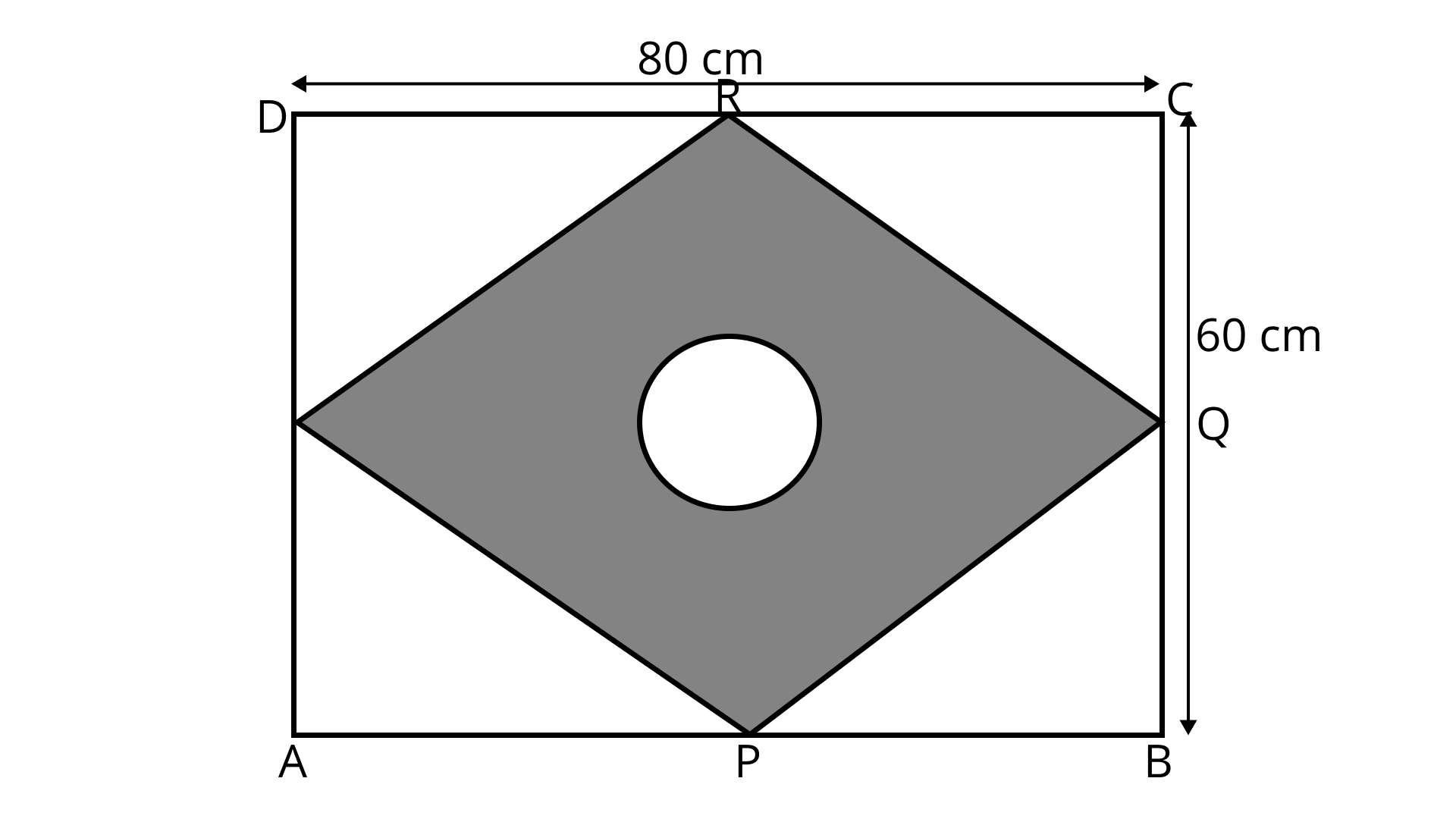
129. A dinner plate is in the form of a circle. A circular region encloses a beautiful design as shown in Fig. 9.70. The inner circumference is
Ans: Let
It has been given that
In addition,
Therefore, width of circular design
130. The moon is about
Ans: Length of path described by moon in one complete revolution
131. A photograph of Billiard/Snooker table has dimensions as

The portion excluding six holes each of diameter
Ans: Actual breadth
Actual length
Area of table
Radius on one hole
Area of 6 holes,
Area of excluding holes,
Therefore, cost of polishing
Easy Solutions for NCERT Exemplar Class 7 Chapter 9
Vedantu offers the NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area to the students which are completely free of cost. This NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area is provided in PDF format, which can be downloaded by the students to study offline.
The subject experts at Vedantu had prepared these solutions: The NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area appropriately. All these solutions are made following the latest NCERT syllabus and guidelines. These solutions are made to help and guide students and get them prepared for examinations. These solutions assist students in their homework and assignments too. Students can certainly do well in the final exams and ace the subject by practising these solutions.
All the sections of this chapter and exercises have been properly covered in this solution file. The prime aim of the experts of Vedantu is to provide the ideal solutions that students can easily grab and work on. They can refer to these solutions to understand how to approach such problems and solve them within a shorter period. To become efficient in solving problems related to perimeter and area, download this file today and add it to your study material.
FAQs on NCERT Exemplar for Class 7 Maths Solutions Chapter 9 Perimeter & Area
1. Are NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area good to score better in class 7 examinations?
Yes, NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area can help students to learn more and more of the subject and thus score better grades in the class 7 examination. This is because, when students practice the solutions mentioned, they learn about all the concepts of the subject deeply. So they are well prepared for the examination. They don't need to be in chaos in the days leading up to the examination. Also, the learning power of the students is enhanced.
2. Where can I find useful study resources for NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area?
You can find NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area on the Vedantu app or website. You can find the NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area at Vedantu. It offers these free resources which are created by the subject experts. They are masters in their respective fields and that's why the content is accurate and reliable. Students can find revision notes, important questions, question papers, etc on the site. These study materials are completely free and there is no cost involved. To access these useful study resources, all students have to sign in, and then they will be able to download what they want in pdf format online or offline.
3. Do you have to pay anything for studying NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area?
No, NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area is completely free of cost. Students can study it only by logging in to the Vedantu site which is absolutely free and just preparing for your examination. They don't need to pay anything to access this NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area. These are made for the convenience of the students. They are made not to loot students, but to make students prepare for the examination, to make them more adaptive, and make learning fun and enjoyable for them.
4. How much time does studying NCERT Exemplar for Class 7 Maths Solutions Chapter - 9 Perimeter & Area involve?
Well it takes just some time to go through and understand these solutions thoroughly and it truly varies from student to student. It also depends upon the difficulty of the subjects and the chapter's concepts. Those with deeper concepts can be difficult to go through by the students while the normal easy-to-understand concepts and chapters revision notes can be easily gone through within a short time. This is where NCERT Exemplar solutions can be used to make all these concepts easy to understand. You can easily use them to write answers correctly and score better in exams.
5. What is NCERT Exemplar?
NCERT Exemplars are practice books that include extra questions of a higher level. These NCERT Exemplars are made to help students help in in-depth learning. The NCERT exemplar books contain conceptual sums, which cover CBSE board exams and competitive exams (JEE Main and JEE advanced). CBSE board exams sometimes contain in-depth sums. They are just made for the convenience of the students.






















