Physics Average Value and RMS Value JEE Main 2025

Understanding Average Value and RMS Value is crucial for mastering topics in physics for JEE Main 2025. These concepts are widely applied in alternating current, wave motion, and electrical circuits. The average value gives the mean of a function over a specific interval, while the RMS value measures the effective value of a varying quantity.

This page provides comprehensive explanations, step-by-step calculations, and practical examples to help students to learn how to find RMS and average value of a waveform. By mastering these concepts, students can easily solve related problems and perform confidently in the exam.

Students can visit and download the other important study resources from the Vedantu website as this content is created by our master teachers and experts keeping in mind that it is updated according to JEE Main Syllabus.

JEE Main Physics Syllabus JEE Main Physics Revision Notes JEE Main Physics Important Questions JEE Main Physics Difference Between JEE Main Physics Question Papers

What are Average Value and RMS Value?

Average Value and RMS value are important expressions for sinusoidal waves. As we know that there are two types of sources and currents: the AC source and DC source; AC and DC. The AC or Alternating Currents is the one that repeats itself after 2π, whereas the DC current source is the one that does not alternate. Some important terms associated with both AC and DC are: RMS value, instantaneous value and the average value. 

RMS value is the amount of heat produced by a resistor when at a time both AC and DC are passed through it. Average value is the area of the one cycle of sinusoidal wave to the time period. However, instantaneous value is the value at a particular instant.  Let us further understand these concepts below.

RMS Value of Current

RMS or the root mean square value of current is the amount of heat produced by a resistor or circuit when an AC and DC pass through it. If we assume the RMS value of currents as Irms and its peak value is Im, then the RMS value formula is given as: 

$ I_{r m s}=\dfrac{I_{m}}{\sqrt{2}}$ 

This is also referred to as the RMS value of alternating current, where Im is the maximum value of the sine wave. 

RMS Value of Sine Wave

We have already seen the expressions for the RMS values but we will now derive the RMS value using the analytical method. Let’s first derive the RMS value of current and then we can generalise it for voltage too. Since the sine wave is symmetrical, we can calculate the RMS value by considering the half cycle only.

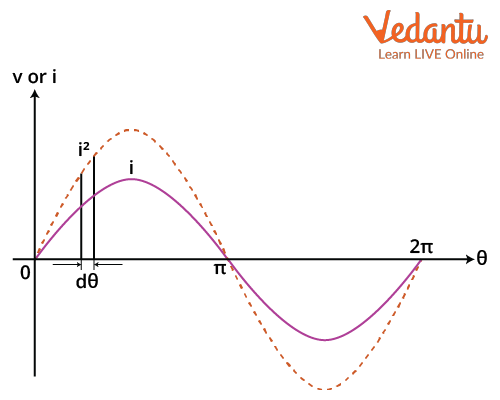
Sinusoidal Wave

We know that the equation of a sinusoidal alternating current i is given as

$i=I_{m} \sin \theta$.

Since the current changes from positive to negative in a n AC signal, we will use the squared current wave because it is always positive for our calculations.

Let’s consider i2 to be the average height of the strip in the above figure. The area of this strip A will be

$A=i^{2} d \theta$

The area of the half cycle of i2 wave will be given as

$\begin{align} &\int A_{h}=\int_{0}^{\pi} i^{2} \mathrm{~d} \theta \\ &A_{h}=\int_{0}^{\pi} I_{m}^{2} \sin ^{2} \theta \mathrm{d} \theta \\ &A_{h}=\dfrac{I_{m}^{2}}{2} \int_{0}^{\pi}(\cos 2 \theta+1) \mathrm{d} \theta \\ &A_{h}=\dfrac{I_{m}^{2}}{2}\left[\theta-\dfrac{\sin 2 \theta}{2}\right]_{0}^{\pi} \\ &A_{h}=\dfrac{\pi I_{m}^{2}}{2} \end{align}$

The mean i.2 or the mean square current can be found by dividing the area by the period or in this case $\pi$. So the mean square current will be

$\begin{align} \overline{i^{2}} &=\dfrac{\dfrac{\pi I_{m}^{2}}{2}}{\pi} \\ \overline{i^{2}} &=\dfrac{I_{m}^{2}}{2} \end{align}$

The root mean square current will be given by the root of the mean square current. This will be

$\begin{align} &I_{R M S}=\sqrt{\dfrac{I_{m}{ }^{2}}{2}} \\ &I_{R M S}=\dfrac{I_{m}}{\sqrt{2}} \end{align}$

This is the expression for the root mean square current. This whole derivation can be repeated for the voltage too and the root mean square voltage will also be

$V_{R M S}=\dfrac{V_{m}}{\sqrt{2}}$

As discussed above the RMS value of a sine wave is denoted as $I_{r m s}=\dfrac{I_{m}}{\sqrt{2}}$ or for sinusoidal voltage it is $V_{r m s}=\dfrac{V_{m}}{\sqrt{2}}$

Alternating Current or AC

Im and Vm here are the peak value of current and voltage respectively. Vm or Im  from above can be calculated as Vrms $\cdot \sqrt{2}$

RMS Value of Square Wave

As we have calculated the RMS value of sinusoidal waves, similarly, we will calculate the RMS value of a square wave. The RMS value is basically just the root of the mean square value. Suppose we have a square wave as given below.

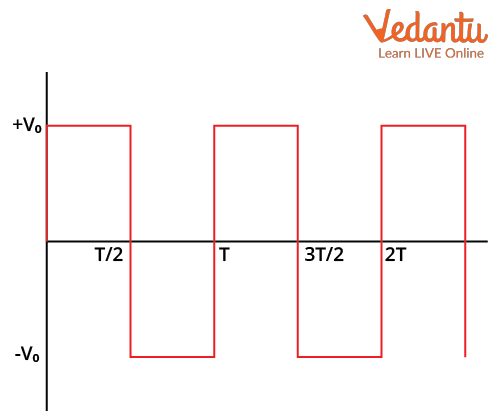
A square wave

The mean square of this wave form can be given as

$\overline{V^{2}}=\dfrac{\int_{0}^{T} V^{2} \mathrm{~d} t}{T}$

We can solve this integral by breaking it into two parts from 0 to $\dfrac{T}{2}$ and then from $\dfrac{T}{2}$ to T. This will give

$\begin{align} &\overline{V^{2}}=\dfrac{1}{T}\left(\int_{0}^{\dfrac{T}{2}} V_{0}^{2} \mathrm{~d} t+\int_{0}^{\dfrac{T}{2}}\left(-V_{0}\right)^{2} \mathrm{~d} t\right) \\ &\overline{V^{2}}=\dfrac{1}{T}\left(V_{0}^{2}\right)\left([t]_{0}^{T / 2}+[t]_{T / 2}^{T}\right) \\ &\overline{V^{2}}=\dfrac{V_{0}^{2}}{T}\left(\left[\dfrac{T}{2}-0\right]+\left[T-\dfrac{T}{2}\right]\right) \\ &\overline{V^{2}}=\dfrac{V_{0}^{2}}{T^{2}} \cdot T \\ &\overline{V^{2}}=V_{0}^{2} \end{align}$

This is the mean square, now the root mean square voltage will be given as

$\begin{align} &V_{R M S}=\sqrt{V_{0}^{2}} \\ &V_{R M S}=V_{0} \end{align}$

This means that the rms value of a square wave is the same as its magnitude. Suppose the magnitude of a square wave is A, then its rms value will also be equal to A. 

A square wave

Average Value of AC

When AC is converted to DC using a rectifier, this converted value of AC is known as the average value of AC. The average value of a sinusoidal wave can be calculated graphically or using the standard sinusoidal equation. By using the standard sinusoidal equation, the average value of AC comes out to be $I_{\text {average }}=\dfrac{2 I_{m}}{\pi}$, where Im is the peak value of the sinusoidal wave. 

Average Value of AC Formula

We will now find the average value for any AC signal. Let’s consider the graph of an AC sinusoidal waveform.

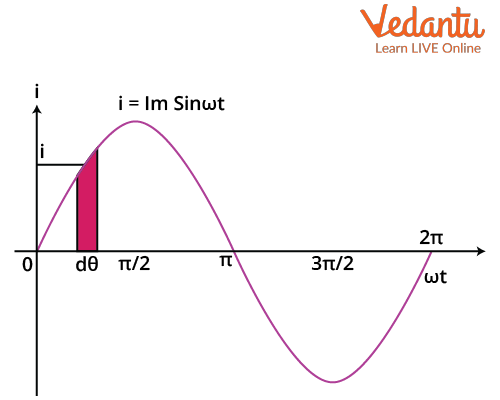
AC Sinusoidal Waveform

Now the average can be found by dividing the area under the graph of the sine wave by the total time period for which the area has been found.

The average for the full cycle will be given as

$\begin{align} &\bar{i}=\int_{0}^{T} i \mathrm{~d} t \\&\bar{i}=\int_{0}^{T} I_{m} \sin \omega t \mathrm{~d} t \\ &\bar{i}=I_{m}\left[\frac{-\cos \omega t}{\omega}\right]_{0}^{T} \\ &\bar{i}=\dfrac{I_{m}}{\omega}[-\cos \omega T-(-\cos 0)]\end{align}$

Now we know that the time period is related to the angular frequency as

$T=\dfrac{2\pi}{\omega}$

Substituting this in the above expression gives,

$\begin{align} &\bar{i}=\dfrac{I_{m}}{\omega}[-\cos 2 \pi+1] \\ &\bar{i}=0 \end{align}$

So the average value of AC for a full cycle is zero. 

For half cycle similarly we can find the average as

$\begin{align} &\bar{i}=\int_{0}^{\dfrac{T}{2}} i \mathrm{~d} t \\&\bar{i}=\int_{0}^{\dfrac{T}{2}} I_{m} \sin \omega t \mathrm{~d} t \\&\bar{i}=I_{m}\left[\dfrac{-\cos \omega t}{\omega}\right]_{0}^{\dfrac{T}{2}} \end{align}$

$\begin{align} &\bar{i}=\dfrac{I_{m}}{\omega}\left[-\cos \left(\omega \dfrac{T}{2}\right)-(-\cos 0)\right]\\ &\bar{i}=\dfrac{I_{m}}{\omega}\left[-\cos \left(\omega \dfrac{2 \pi}{2 \omega}\right)+1\right] \\&\bar{i}=\dfrac{I_{m}}{\omega}[-\cos (\pi)+1] \\&\bar{i}=\dfrac{I_{m}}{\omega}[-(-1)+1] \\ &\bar{i}=\dfrac{2 I}{\omega} \end{align}$

The average value of the AC formula is thus given as $I_{\text {average }}=\dfrac{2 I_{m}}{\pi}$. We calculated that the average value for a full sine wave is zero. As there is the same amount of current in the positive and negative cycle, and flowing in opposite directions thus, it neutralises the effect and the average value of a full sinusoidal wave is zero. The same formula holds true for an alternating voltage. Thus, the average value of the voltage formula is given as $V_{\text {average }}=\dfrac{2 V_{m}}{\pi}$.

W

It is true for a half cycle, for a full cycle, however, the average value of voltage is zero. 

Instantaneous Value

The instantaneous value of AC, on the other hand, is its value at a particular instant. In one cycle, there are multiple instances of a wave. Look at the figure below.

Instantaneous Value of a Sine Wave

Now, we know that the current in a sine wave can be expressed in terms of its amplitude Im as

$i=I_{m} \sin \omega t$

For any value of t we can find the value of the current. This means that this is the formula for the instantaneous current at a particular value of t. If we want to find the value of current for a particular angle $\theta=\omega t$, then we can use the relation between the time period and angular frequency which is given as

$T=\dfrac{2\pi}{\omega}$.

This way we can find the instantaneous value of AC.

Significance of Average Value and Root Mean Square (RMS) Value in JEE Main 2025 

• Fundamental Concepts: These values are essential in analysing alternating currents, waveforms, and signal processing, forming the basis for various topics.

• Problem-Solving Skills: Mastery of these concepts enables efficient tackling of numerical problems involving periodic functions and electrical circuits.

• Application Across Topics: Knowledge of average and RMS values is applicable in multiple subjects, enhancing overall performance.

• Scoring Potential: Questions related to these concepts are common in JEE Main, offering opportunities to secure marks with proper understanding.

 Tips for JEE Main 2025 preparation

1. Know the Syllabus: Focus on high-weightage topics in Physics, Chemistry, and Maths.

2. Make a Study Plan: Allocate time for concept building, practice, and revision.

3. Strengthen Basics: Use NCERT textbooks to build a strong foundation.

4. Practise Regularly: Solve past papers and take topic-wise mock tests.

5. Time Management: Learn to balance time between subjects and questions.

6. Revise Often: Maintain short notes and revise formulas frequently.

7. Use Quality Resources: Refer to trusted books and platforms like Vedantu for guidance.

8. Mock Tests: Take weekly mock tests and work on weak areas.

9. Consistency: Stick to your plan and maintain discipline.

10. Self-Care: Get enough rest, eat healthily, and stay positive.

Benefits of Vedantu Study Resources for JEE Main 2025 

• Expert-designed study materials aligned with the JEE Main syllabus.

• Live classes for real-time doubt-solving and better understanding.

• Mock tests with detailed analysis to improve performance.

• Free PDFs, notes, and practice questions for easy revision.

• Flexible learning with recorded sessions and self-paced courses.

• Personalised doubt-clearing sessions for focused preparation.

• Comprehensive coverage of Physics, Chemistry, and Mathematics.

Conclusion

AC and DC are fundamental supply sources in an electric circuit, be it the respective voltage or the currents. AC as we know is important as the supply to our homes is AC, as well as the appliances. However, it does not neglect the DC supply as it too is used for various applications. Electronic circuits such as TV Clock, laptop, LED strip etc all use DC supply. Moreover, solar panels themselves produce DC. With different sources comes the ways to denote them. Thus, average , rms and instantaneous values play an important role. Each one of these quantities have their own significance which are as discussed above. 

JEE Main 2025 Subject-wise Important Chapters

The JEE Main 2025 subject-wise important chapters provide a focused strategy for Chemistry, Physics, and Maths. These chapters help students prioritise their preparation, ensuring they cover high-weightage topics for better performance in the exam.

Important Links for JEE Main and Advanced 2025

Access a curated collection of study materials and tips to excel in JEE Main and Advanced 2025, helping you prepare effectively for these prestigious engineering entrance exams.