Question

A rod of length $L$ is sliding on a frictionless surface as shown in the figure. Velocity of end $A$ is $4$$m/s$ along the wall. Find the velocity of end $B$, when and $B$ makes ${30^0}$ with wall $PQ$.

A) $8$$m/s$
B) $2\sqrt 3 $$m/s$
C) $4\sqrt 3 $$m/s$
D) $\dfrac{4}{{\sqrt 3 }}$$m/s$

Answer 1

Hint: In this question the rod of length is sliding horizontally on the frictional surface. The velocity of one end that is A is given. We need to find the velocity of the other end that is B in which angle is given. First find the angle of A and then find the velocity.

Complete step by step answer:
The rod is sliding on a horizontal frictionless surface then the gravity will be in downward and uniform. The force of friction is exerted by the object and the inclined plane is each other which is parallel to the surface of the plane but the normal force is perpendicular to the plane.

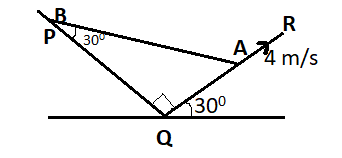
At the position A the angle is ${60^0}$then it becomes, ${V_A}\cos {60^0}$
At position B the angle is ${30^0}$, then we need to find the velocity at the end B
So,
$ \Rightarrow $ \[{V_A}\cos {60^0} = {V_B}\cos {30^0}\]…………(1)
The value of ${V_A}$ is $4$$m/s$
Substitute ${V_A}$ in the equation (1)
Then we get,
$ \Rightarrow $ $4 \times \cos {60^0} = {V_B} \times \cos {30^0}$
The value of $\cos {60^0} = \dfrac{1}{2}$ ,
And the value of $\cos {30^0} = \dfrac{{\sqrt 3 }}{2}$
If we substitute the value we get,
$ \Rightarrow $ $4 \times \dfrac{1}{2} = {V_B} \times \dfrac{{\sqrt 3 }}{2}$
$ \Rightarrow $ $2 = {V_B} \times \dfrac{{\sqrt 3 }}{2}$
$ \Rightarrow $ ${V_B} = 2 \times \dfrac{2}{{\sqrt 3 }}$
$ \therefore $ ${V_B} = \dfrac{4}{{\sqrt 3 }}$
The velocity of end B is $\dfrac{4}{{\sqrt 3 }}$$m/s$

So therefore option (D) is correct.

Note: If you put a grease into the kitchen sink, the grease all sticks inside the pipe and if you put the food particle it will stick into the grease and water backs up behind the blockage so all the pipes are in a frictionless coating.