Question

What does the slope of a distance-time graph indicate?

Answer 1

Hint: The slope of any two quantities (let’s say for graph A-B) gives the change in A with respect to change in B. Find what change in distance with respect to change in time indicates.

Complete step by step solution:
A distance-time is a graph between, distance travelled by an object in a given time. Distance is considered on Y-axis and time is considered on X-axis. The motion of the object is said to be uniform when the graph is a straight line. The slope of the straight-line graph is the same for any two sets of points.
As per the given question, let’s check what the change in distance with respect to change in time indicates:
Consider the graph below:

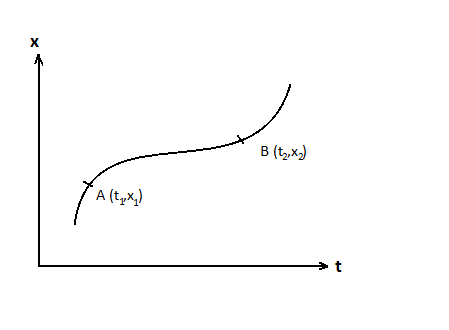
The above graph indicates the motion of an object. The change in displacement with respect to time is depicted in the graph.
We know that velocity is defined as the rate of change of displacement. And rate of change means change of some quantity with respect to time.
Now from the graph, it is clear that position of particle initially is $A({x_1},{y_1})$ and final position is $B({x_2},{y_2})$
Change in displacement will be given as:
$dx = {x_2} - {x_1}$ and $dy = {y_2} - {y_1}$
Now the rate of change in X and Y will be given as:
$\dfrac{{dx}}{{dt}} = \dfrac{{{x_2} - {x_1}}}{{dt}}$ and $\dfrac{{dy}}{{dt}} = \dfrac{{{y_2} - {y_1}}}{{dt}}$
Rate of change of displacement is velocity.

Note: The graph of displacement-time can be linear, non-linear, continuous or discontinuous depending on the motion of the particle. It must also be noted, the rate of change of velocity is known as acceleration. Please remember that for particles having constant velocity, its acceleration is zero.