Question

In the figure shown, battery 1 has emf =6V ,internal resistance =1Ω . Battery 2 has emf= 2V and internal resistance =3Ω. The wire has negligible resistance. What is the potential difference across the terminals of battery 2 ?

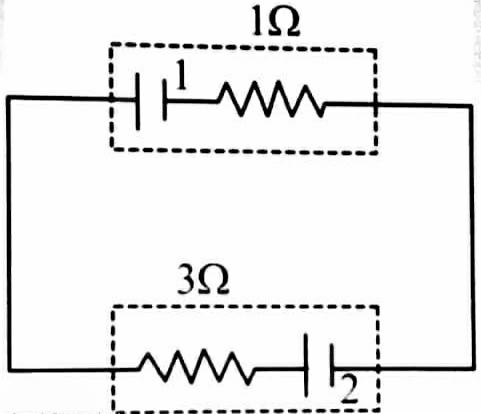
A) 4V
B) 1.5V
C) 5V
D) 0.5V

Answer 1

Hint: Using Kirchhoff’s Second Law (Voltage Law) , first we will calculate the value of current across the circuit. After obtaining the value of current we will calculate the voltage drop across the second battery.

Complete step by step solution:
We will solve this question using Kirchhoff’s Second Law ,which states-“ The algebraic sum of change in potential around a closed loop is zero”. Mathematically,\[\sum {V = 0} \].
Applying second law,
1. Choose a loop. The loop should be closed. The loop can involve any number of cells, resistors, capacitors etc.
2. Draw current from one cell( usually with a larger emf) , and follow Kirchhoff’s Current Law for distribution of current.
3. Decide the direction of current –clockwise/anticlockwise as per your convenience.
4. Take voltage as positive or negative i.e. if the current is moving from higher potential to lower potential ,take voltage drop NEGATIVE. If the current is moving from lower potential to higher potential, take voltage drop POSITIVE.
5. Drawing the circuit diagram as per above steps, we get-
6. Using loop rule, let the current in the circuit be $i$. Using battery 1 as source battery( the current is being withdrawn from the first battery).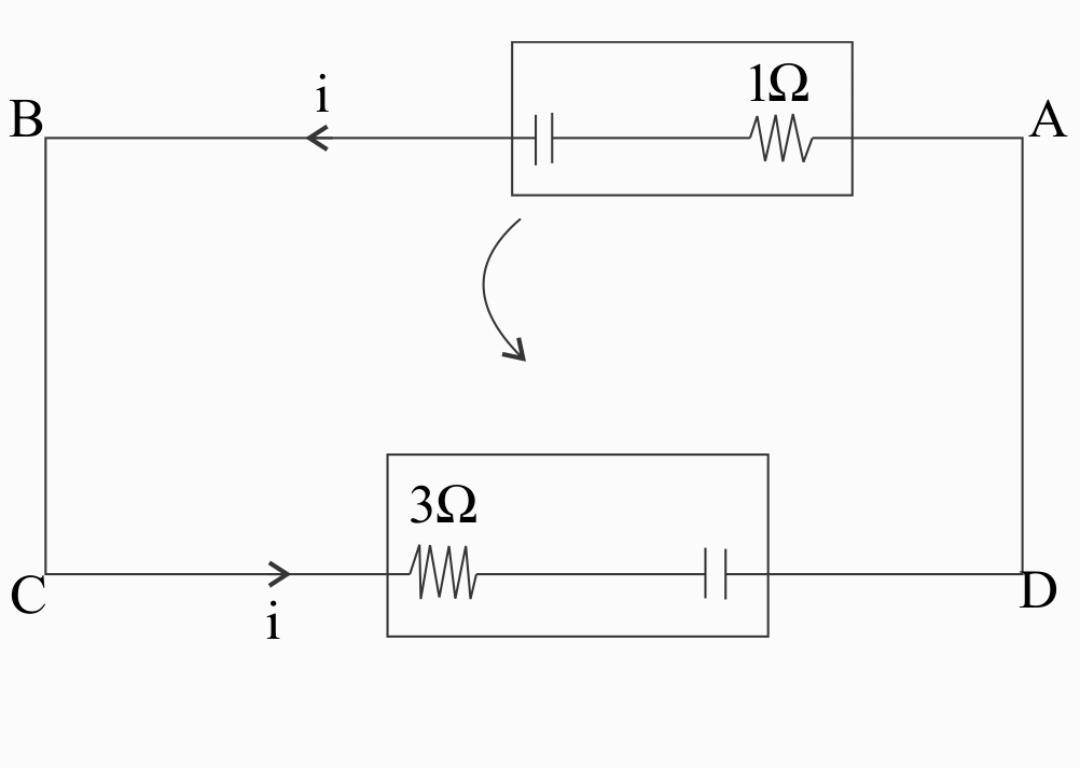
We have a value of 1A.
7. Potential difference across battery 2 is given by-
${V_2} = {E_2} + i{R_2}$ ( getting charged)
   $
  {V_{2 = }}2 + 1{R_2} \\
  {V_2} = 2 + 3 \\
  {V_2} = 5 \\
 $
The voltage drop across battery 2 is 5V.

Hence, option (C) is correct.

Note: Kirchhoff’s Second Law is based on the principle of conservation of energy.
Kirchhoff’s First Law , generally known as KCL( Kirchhoff’s Current Law ) is based on the principle of conservation of charge.