Answer
109.8k+ views
Hint: For a blackbody the relation between emissive power and temperature is given by Stefan’s law which states that emissive power is directly proportional to the fourth power of temperature.
$E \propto {T^4}$
Where, $E$ is the emissive power and $T$ is the temperature.
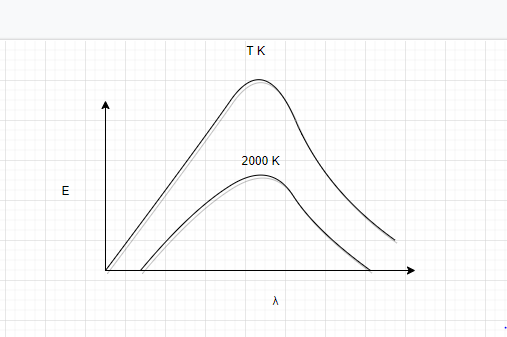
The area under the graph between energy density ${E_\lambda }$ and wavelength $\lambda $ gives the emissive power.
Complete step by step answer:
For a blackbody the relation between emissive power and temperature is given by Stefan’s law which states that emissive power is directly proportional to the fourth power of temperature.
$E \propto {T^4}$
Where, $E$ is the emissive power and $T$ is the temperature
The area under the graph between energy density ${E_\lambda }$ and wavelength $\lambda $ gives the emissive power.
Let the area under the curve at $T\,K$ be ${A_1}$.Then
${A_1} \propto {T^4}$ …… (1)
That is,${A_1} = \sigma {T^4}$
Let area under the curve at $2000\,K$ be ${A_2}$ ;then,
${A_2} \propto {\left( {2000} \right)^4}$ …….. (2)
That is,
${A_2} = \sigma {\left( {2000} \right)^4}$
Divide equation (1) by (2)
$\dfrac{{{A_1}}}{{{A_2}}} = \dfrac{{{T^4}}}{{{{\left( {2000} \right)}^4}}}$ ……. (3)
Ratio of area is given as $16:1$
Substituting this in equation (3), we get
$
\dfrac{{16}}{1} = \dfrac{{{T^4}}}{{{{2000}^4}}} \\
\left( {\dfrac{T}{{2000}}} \right) = {\left( {16} \right)^{\dfrac{1}{4}}} \\
T = 2000 \times 2 \\
= 4000K \\
$
So, the correct answer is option D.
Note: A black body is a body that absorbs all the radiation falling on it and it has emissivity equal to 1. It is important in this problem that we consider the power of the temperature and just don’t solve it assuming a linear relationship.
$E \propto {T^4}$
Where, $E$ is the emissive power and $T$ is the temperature.
The area under the graph between energy density ${E_\lambda }$ and wavelength $\lambda $ gives the emissive power.
Complete step by step answer:
For a blackbody the relation between emissive power and temperature is given by Stefan’s law which states that emissive power is directly proportional to the fourth power of temperature.
$E \propto {T^4}$
Where, $E$ is the emissive power and $T$ is the temperature
The area under the graph between energy density ${E_\lambda }$ and wavelength $\lambda $ gives the emissive power.
Let the area under the curve at $T\,K$ be ${A_1}$.Then
${A_1} \propto {T^4}$ …… (1)
That is,${A_1} = \sigma {T^4}$
Let area under the curve at $2000\,K$ be ${A_2}$ ;then,
${A_2} \propto {\left( {2000} \right)^4}$ …….. (2)
That is,
${A_2} = \sigma {\left( {2000} \right)^4}$
Divide equation (1) by (2)
$\dfrac{{{A_1}}}{{{A_2}}} = \dfrac{{{T^4}}}{{{{\left( {2000} \right)}^4}}}$ ……. (3)
Ratio of area is given as $16:1$
Substituting this in equation (3), we get
$
\dfrac{{16}}{1} = \dfrac{{{T^4}}}{{{{2000}^4}}} \\
\left( {\dfrac{T}{{2000}}} \right) = {\left( {16} \right)^{\dfrac{1}{4}}} \\
T = 2000 \times 2 \\
= 4000K \\
$
So, the correct answer is option D.
Note: A black body is a body that absorbs all the radiation falling on it and it has emissivity equal to 1. It is important in this problem that we consider the power of the temperature and just don’t solve it assuming a linear relationship.
Recently Updated Pages
If x2 hx 21 0x2 3hx + 35 0h 0 has a common root then class 10 maths JEE_Main

The radius of a sector is 12 cm and the angle is 120circ class 10 maths JEE_Main

For what value of x function fleft x right x4 4x3 + class 10 maths JEE_Main

What is the area under the curve yx+x1 betweenx0 and class 10 maths JEE_Main

The volume of a sphere is dfrac43pi r3 cubic units class 10 maths JEE_Main

Which of the following is a good conductor of electricity class 10 chemistry JEE_Main

Other Pages
If a wire of resistance R is stretched to double of class 12 physics JEE_Main

The adjoining diagram shows the spectral energy density class 11 physics JEE_MAIN

In a steady state of heat conduction the temperature class 11 physics JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main