Question

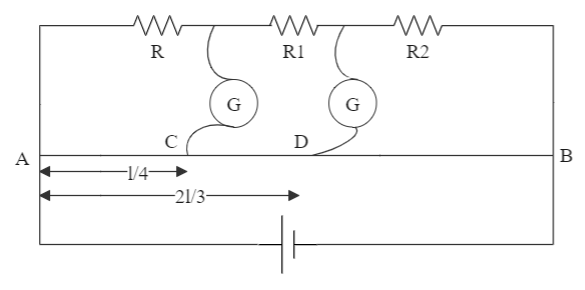
The diagram below shows a modified meter bridge which is used for measuring two unknown resistances at the same time. The first galvanometer gives the balance point at C and the second one fives the balance point at D. Using the details given in the diagram, find out the values of \[{R_1}\] and\[{R_2}\] [This question may have multiple correct options].

(A) \[{R_1} = \dfrac{{5R}}{3}\]
(B) \[{R_2} = \dfrac{{4R}}{3}\]
(C) \[{R_1} = \dfrac{{4R}}{3}\]
(D) \[{R_2} = \dfrac{{5R}}{3}\]

Answer 1

Hint: When a meter bridge is balanced at a certain point, it is used to find the unknown resistances in its setup. In this question, the two galvanometers work independently. So, the two unknown resistances can be found by solving linear equations made with the help of balance.
Formula used: \[\dfrac{{{R_1}}}{{{R_2}}} = \dfrac{l}{{total - l}}\] where \[{R_1}\] and \[{R_2}\] are two resistances on either side of the galvanometer jockey, $l$ is the length at which the jockey is touched on the scale and total is the full length of the meter scale.

Complete step by step answer:
In a meter bridge, the galvanometer is connected between the resistances to the wire using a jockey. As the jockey touches the taut wire at some point, an electrical connection is established that renders a balance between the total resistance per unit length on both sides of the point.
In this question, the galvanometer touches two points and we are asked to find two unknown resistances. Let us first calculate the distances AC, CB and AD, DB so that the two sides can be easily balanced.
\[AC = \dfrac{l}{4}\]
\[CB = l - \dfrac{l}{4} = \dfrac{{3l}}{4}\]
\[AD = \dfrac{{2l}}{3}\]
\[DB = l - \dfrac{{2l}}{3} = \dfrac{l}{3}\]
Now, using the balance equation at point C we try to find the values of unknown resistances as:
\[\dfrac{R}{{{R_1} + {R_2}}} = \dfrac{{AC}}{{CB}}\]
Putting the values of AC and CB, we get:
\[\dfrac{R}{{{R_1} + {R_2}}} = \dfrac{{\dfrac{l}{4}}}{{\dfrac{{3l}}{4}}}\]
\[ \Rightarrow \dfrac{R}{{{R_1} + {R_2}}} = \dfrac{1}{3}\] [By dividing and cancelling $l$]
Cross-multiplying and rearranging the two gives us:
\[
  3R = {R_1} + {R_2} \\
   \Rightarrow {R_1} = 3R - {R_2} \\
 \] [Eq. 1]
Similarly, using the balance equation at point D we get,
\[\dfrac{{R + {R_1}}}{{{R_2}}} = \dfrac{{AD}}{{DB}}\]
Putting the values of AD and DB gives us:
\[\dfrac{{R + {R_1}}}{{{R_2}}} = \dfrac{{\dfrac{{2l}}{3}}}{{\dfrac{l}{3}}}\]
\[ \Rightarrow \dfrac{{R + {R_1}}}{{{R_2}}} = 2\][By dividing and cancelling $\dfrac{l}{3}$]
Upon cross-multiplying and rearranging, we get:
\[R + {R_1} = 2{R_2}\]
\[ \Rightarrow {R_1} = 2{R_2} - R\] [Eq. 2]
We can see that both Eq. 1 and Eq.2 have\[{R_1}\]on their LHS. Hence, we can equate to two to get:
\[3R - {R_2} = 2{R_2} - R\]
\[3{R_2} = 4R\] [Using linear algebra]
This gives us \[{R_2} = \dfrac{{4R}}{3}\]
We put this value in Eq. 1:
\[{R_1} = 3R - \dfrac{{4R}}{3}\]
Taking the LCM and simplifying we get,
\[ \Rightarrow {R_1} = \dfrac{{9R - 4R}}{3} = \dfrac{{5R}}{3}\]

Hence, we can see that the options A and B are correct.

Note: A meter bridge works on the principle of a Wheatstone bridge. It is mostly used to identify unknown resistance when a set of known resistances are provided, just like the Wheatstone. The meter bridge provides very accurate measurements for resistance because the ammeter and voltmeter are not involved and their intrinsic resistances do not affect the readings.