
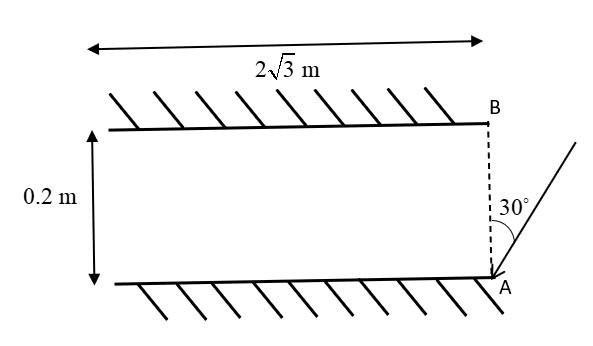
Two plane mirrors A and B are aligned parallel to each other, as shown in the figure. A light ray is incident at an angle of ${30^ \circ }$ at a point just inside one end of A. The plane of incidence coincides with the plane of the figure. What is the maximum number of times the ray undergoes reflection (including the first one) before it emerges out?
A. 28
B. 30
C. 32
D. 34
Answer
133.8k+ views
Hint: The total distance that the ray will cover before emerging out will be equivalent to the length of the plane mirrors. To solve the above question, first calculate the distance that the ray will cover in one reflection and then proceed further to calculate the maximum number of reflections.
Complete answer:
The length of the mirrors is the distance that the ray should cover in order to emerge out.
Separation between the mirrors, $d = 0.2{\text{ m}}$
Let us calculate the distance that the ray covers in the first reflection.
For plane mirrors, the angle of incidence is equivalent to the angle of reflection.
Hence, angle of reflection $ = {30^ \circ }$ .
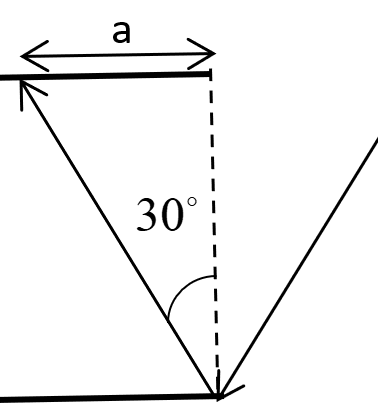
In the above triangle,
$\tan {30^ \circ } = \dfrac{a}{d}$
Substituting the values,
$\dfrac{1}{{\sqrt 3 }} = \dfrac{a}{{0.2}}$
This gives $a = \dfrac{{0.2}}{{\sqrt 3 }}$
First reflection onwards, the distance covered by each ray would be $\dfrac{{0.2}}{{\sqrt 3 }}$ .
Let the total number of times the ray was reflected including the first one be $n$ , then
$n\left( {\dfrac{{0.2}}{{\sqrt 3 }}} \right) = 2\sqrt 3 $
On simplifying, we get $n = 30$ .
Hence, the maximum number of times the ray undergoes reflection before it emerges is 30.
Thus, the correct option is B.
Note:Angle of incidence is the angle that the incident ray makes with the normal of the mirror and angle of reflection is the angle that the reflected ray makes with the normal of the mirror. In the case of plane mirrors, the angle of incidence is equivalent to the angle of reflection. Use this property of plane mirrors and trigonometric relations to solve the given question.
Complete answer:
The length of the mirrors is the distance that the ray should cover in order to emerge out.
Separation between the mirrors, $d = 0.2{\text{ m}}$
Let us calculate the distance that the ray covers in the first reflection.
For plane mirrors, the angle of incidence is equivalent to the angle of reflection.
Hence, angle of reflection $ = {30^ \circ }$ .

In the above triangle,
$\tan {30^ \circ } = \dfrac{a}{d}$
Substituting the values,
$\dfrac{1}{{\sqrt 3 }} = \dfrac{a}{{0.2}}$
This gives $a = \dfrac{{0.2}}{{\sqrt 3 }}$
First reflection onwards, the distance covered by each ray would be $\dfrac{{0.2}}{{\sqrt 3 }}$ .
Let the total number of times the ray was reflected including the first one be $n$ , then
$n\left( {\dfrac{{0.2}}{{\sqrt 3 }}} \right) = 2\sqrt 3 $
On simplifying, we get $n = 30$ .
Hence, the maximum number of times the ray undergoes reflection before it emerges is 30.
Thus, the correct option is B.
Note:Angle of incidence is the angle that the incident ray makes with the normal of the mirror and angle of reflection is the angle that the reflected ray makes with the normal of the mirror. In the case of plane mirrors, the angle of incidence is equivalent to the angle of reflection. Use this property of plane mirrors and trigonometric relations to solve the given question.
Recently Updated Pages
JEE Main 2025 Session 2 Form Correction (Closed) – What Can Be Edited

Sign up for JEE Main 2025 Live Classes - Vedantu

JEE Main Books 2023-24: Best JEE Main Books for Physics, Chemistry and Maths

JEE Main 2023 April 13 Shift 1 Question Paper with Answer Key

JEE Main 2023 April 11 Shift 2 Question Paper with Answer Key

JEE Main 2023 April 10 Shift 2 Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Wheatstone Bridge for JEE Main Physics 2025

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Diffraction of Light - Young’s Single Slit Experiment

Dual Nature of Radiation and Matter Class 12 Notes: CBSE Physics Chapter 11

If a wire of resistance R is stretched to double of class 12 physics JEE_Main

JEE Advanced 2024 Syllabus Weightage

Current Loop as Magnetic Dipole and Its Derivation for JEE




