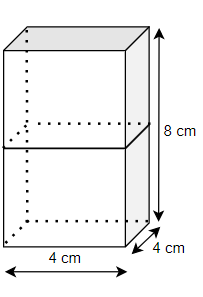
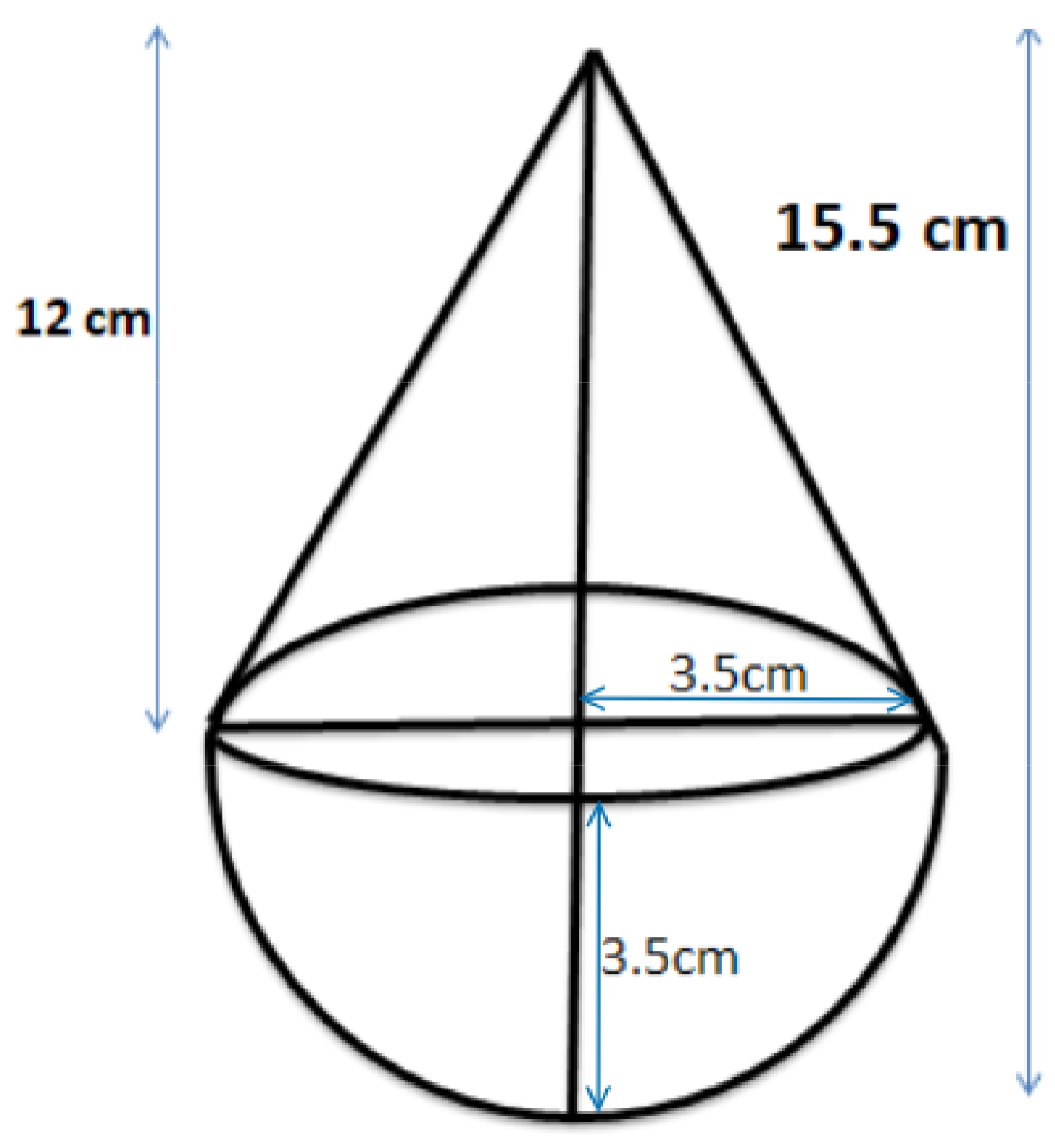
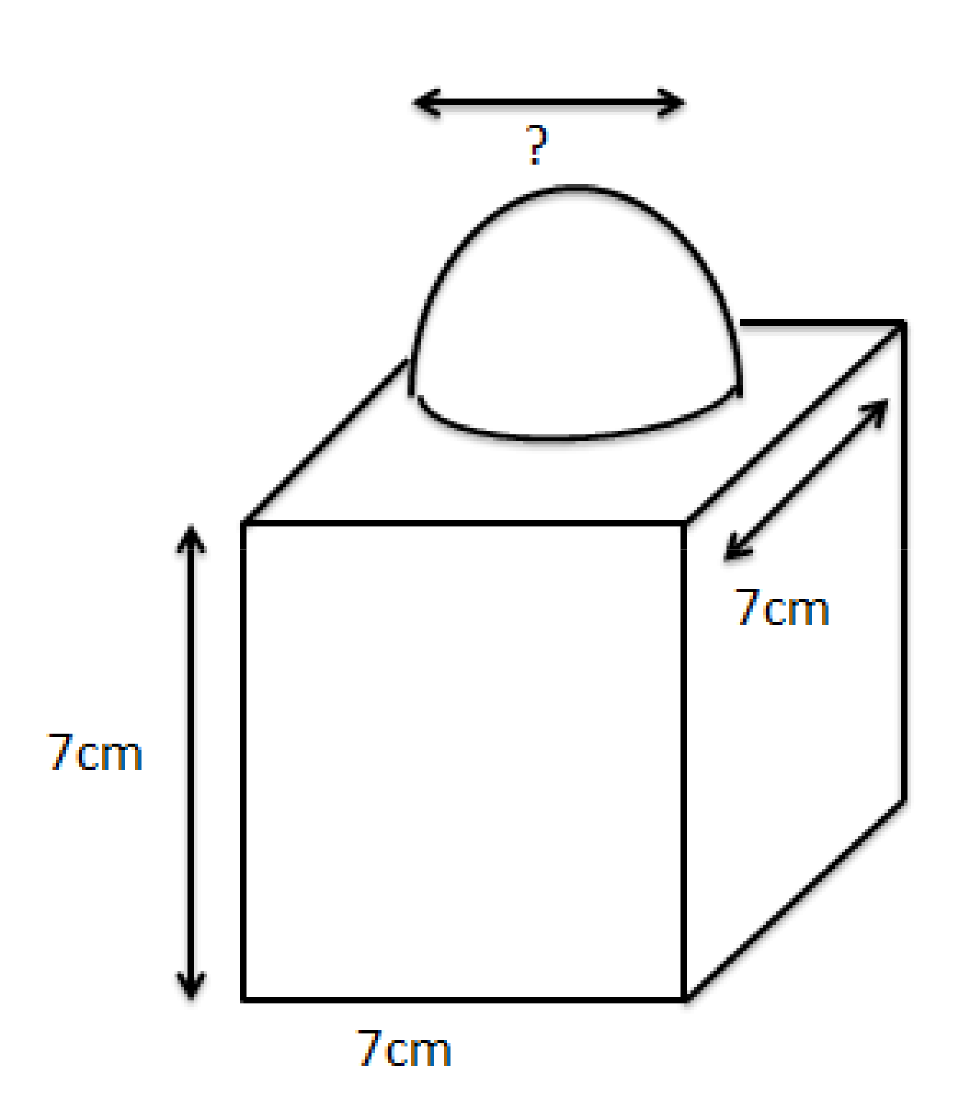
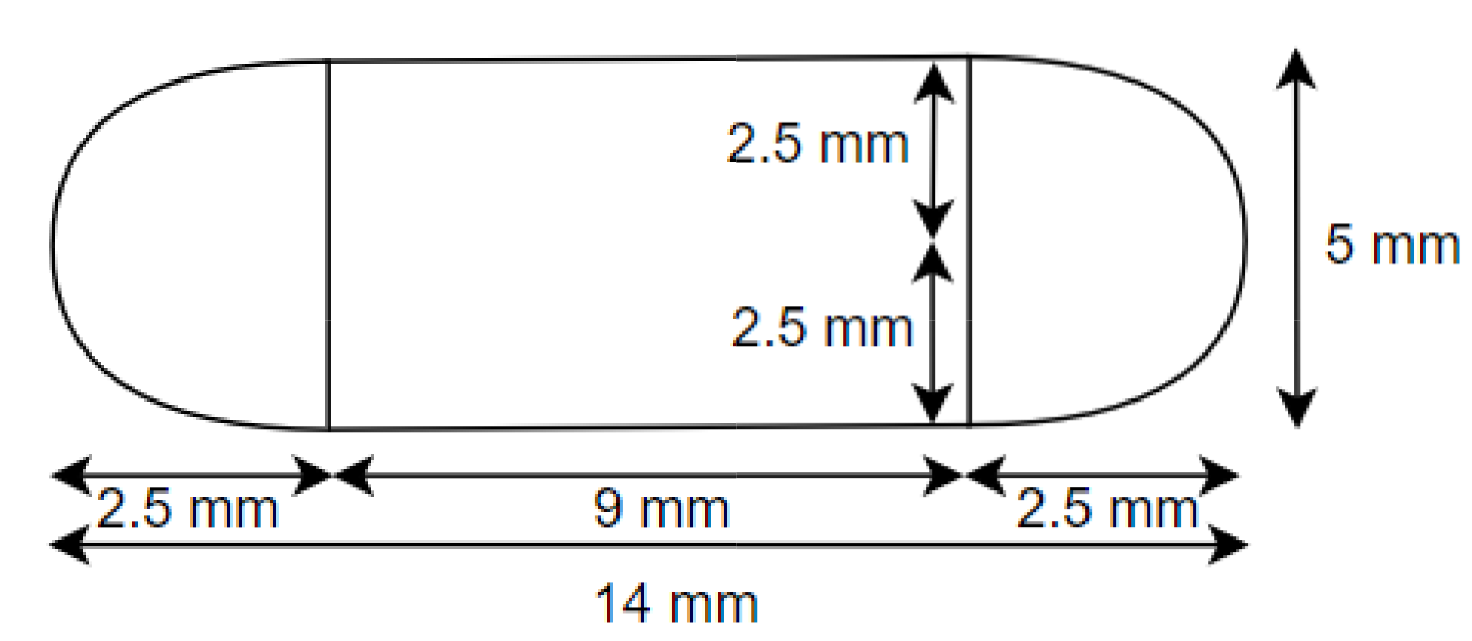
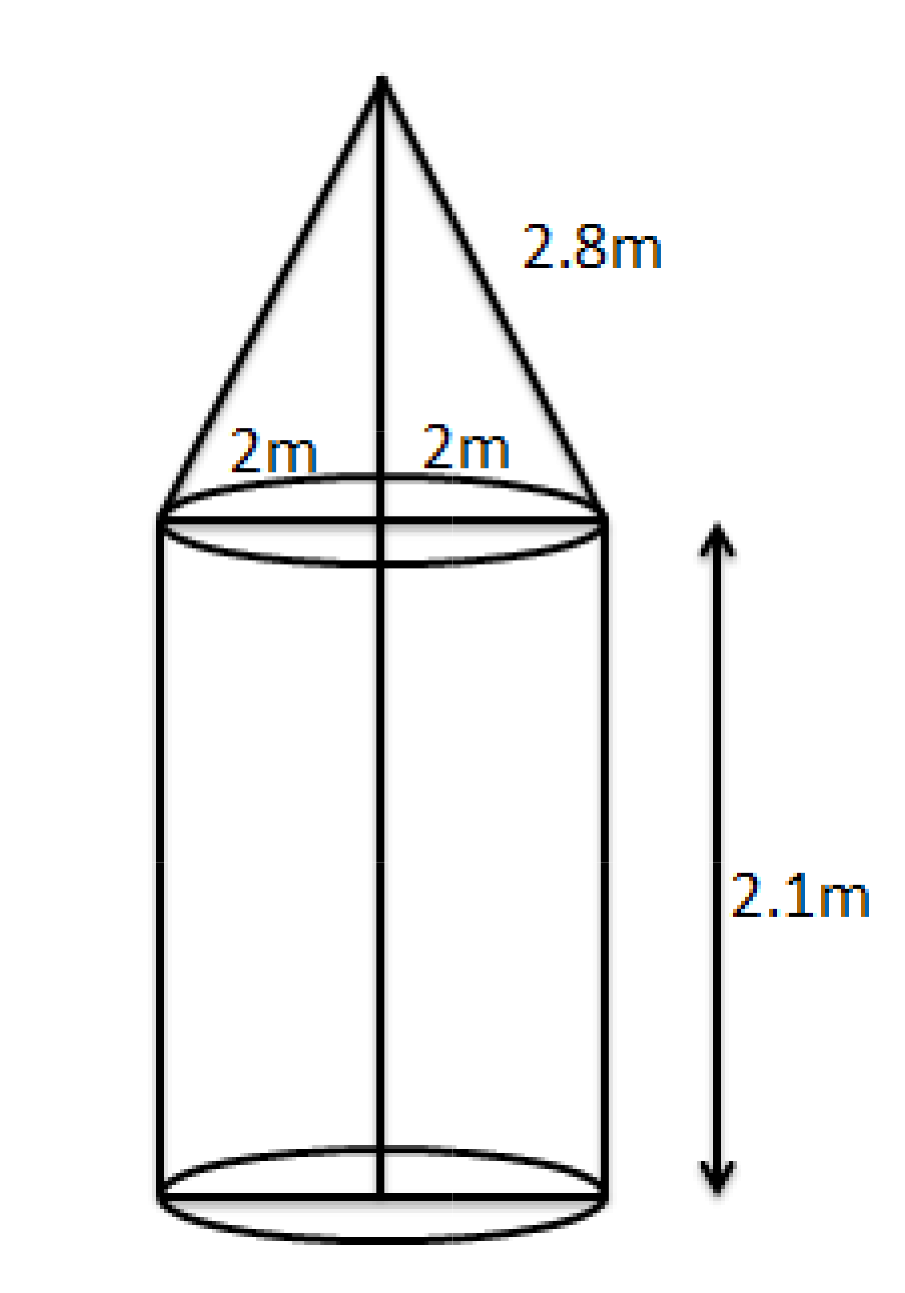
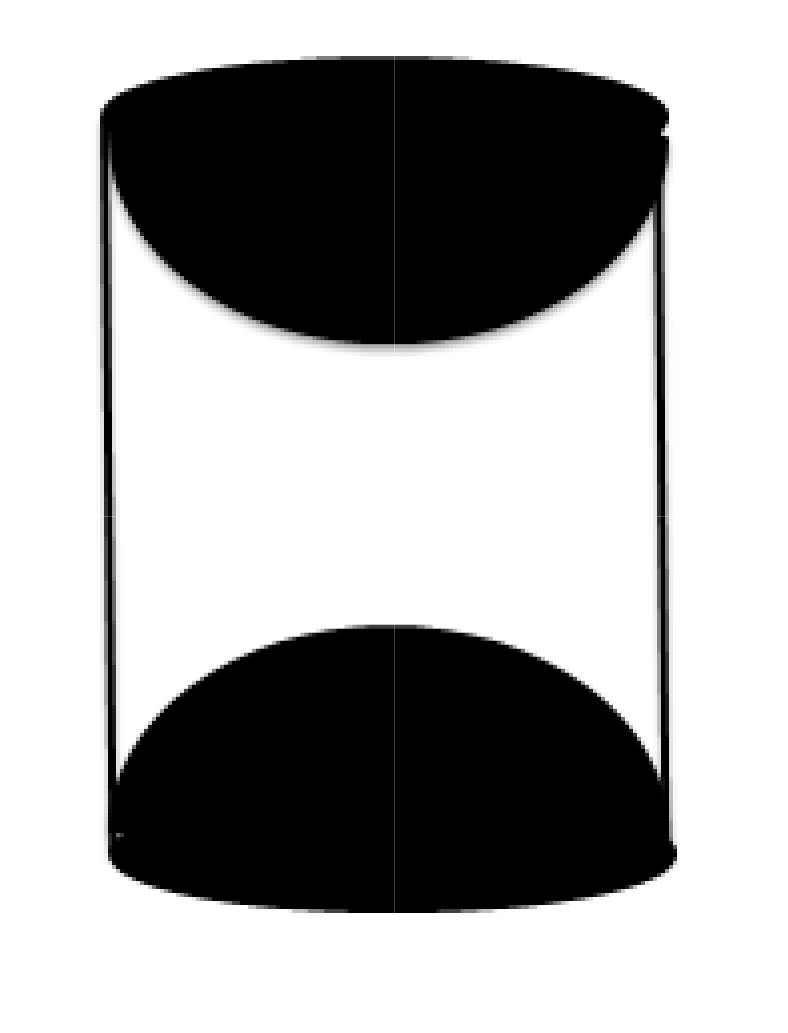
NCERT Solutions for Class 10 Maths Chapter 12 Exercise 12.1 - FREE PDF Download
NCERT Solutions for Class 10 Maths Chapter 12 Surface Areas And Volumes Ex 12.1
FAQs on NCERT Solutions for Class 10 Maths Chapter 12 Surface Areas And Volumes Ex 12.1
1. What are the topics and sub-topics of the Class 10 Maths Chapter 12?
There are quite a few topics and sub-topics of this chapter. They are- 1) Surface Areas and Volumes, 2) Introduction, 3) Surface Area of a Combination Of Solid, 4) Volume of a combination of Solids, 5) Conversion of Solid From One Shape to Another, 6) Frustum of a Cone.
2. How many questions are there in Class 10 Maths Chapter 12 Exercise 12.1 of the NCERT textbook?
Chapter 12 Exercise 12.1 of Class 10 Mathematics NCERT book contains a total of seven long and two short questions. We, at Vedantu, provide the answers for the same as well in our solutions PDF, which you will find on our website and mobile application. You can download these solutions without spending a penny and use them for better exam preparation.
3. What is a frustum of a cone?
Here goes the definition- when we cut through a cone or cone-shaped object with a plane parallel to the base and remove the cone that is formed on one side of the plane, now the part which is now leftover on the other side of the plane is called as a frustum of a cone.
4. How is Vedantu’s NCERT Solutions for Class 10 Maths Chapter 12 Exercise 12.1 prepared?
Class 10 students who are looking for a better NCERT Solutions for Mathematics should definitely look into the solutions PDF provided by Vedantu, India’s no.1 ed-tech company. All the answers provided in these solutions are 100% accurate. The problems on this exercise have been answered by our Maths expertise, to make students understand the theories in a better way. Topics covered in these materials are according to NCERT syllabus and guidelines. Therefore, solutions for Maths class 10, are very helpful for students who are doing the preparation for the exams and to score good marks.




















 Watch Video
Watch Video