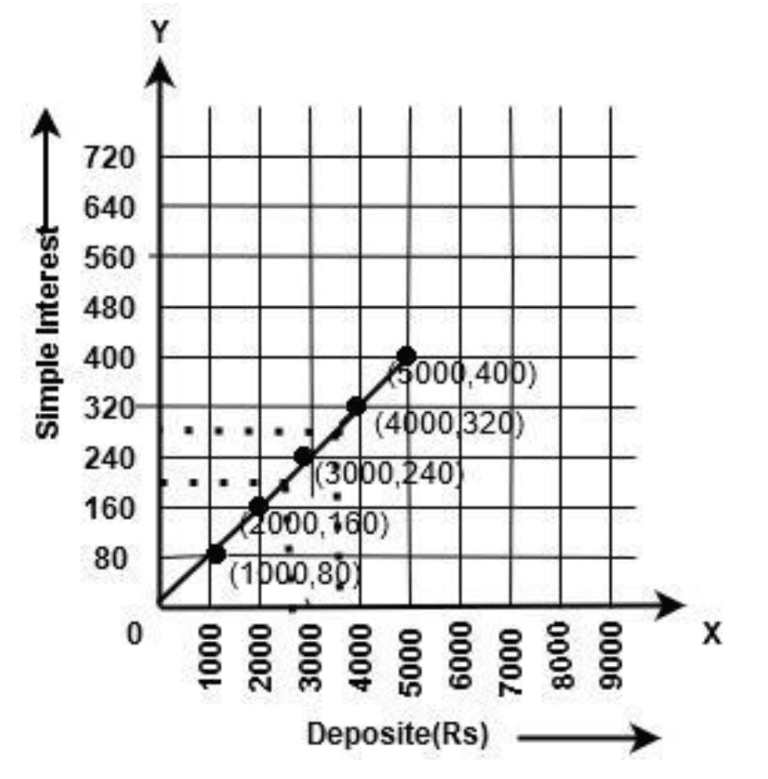
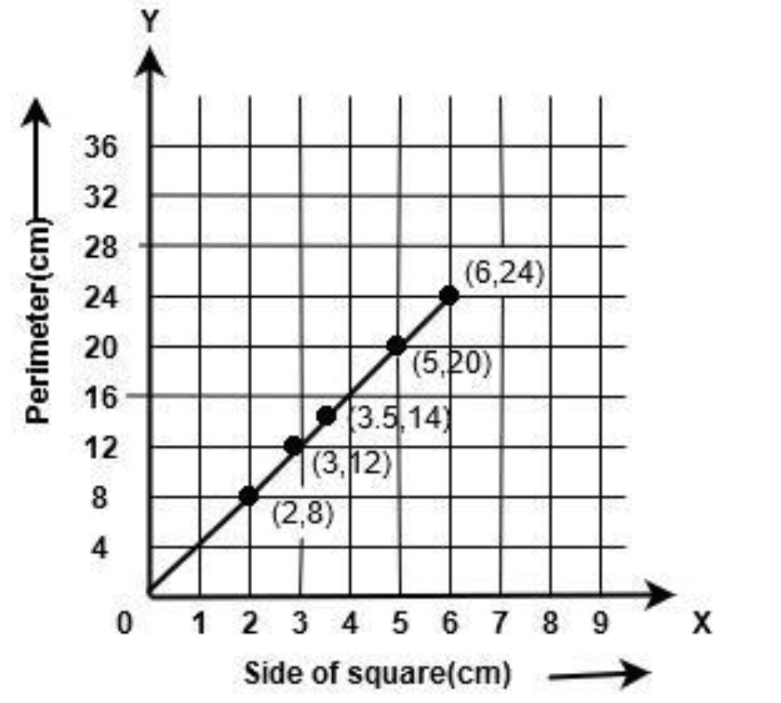
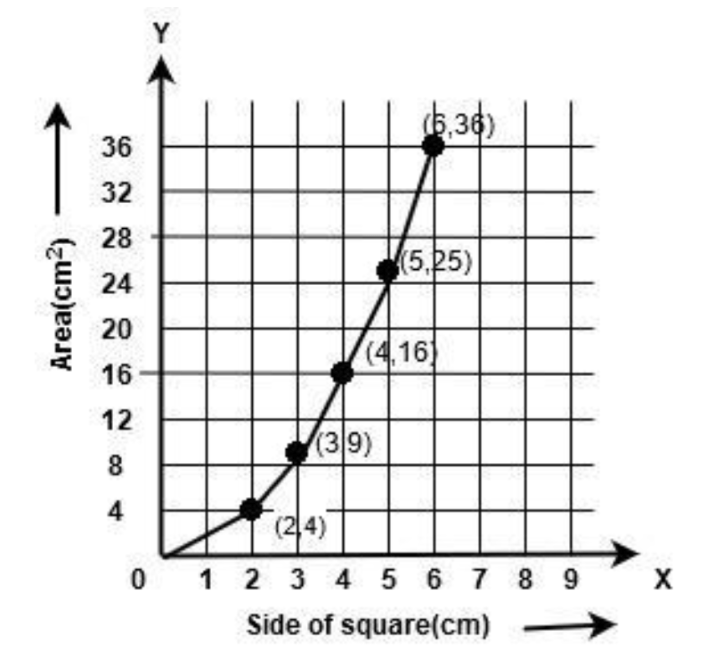
NCERT Solutions for Chapter 13 Maths Class 8 Introduction To Graphs - FREE PDF Download
FAQs on NCERT Solutions for Class 8 Maths Chapter 13 Introduction To Graphs
1. What are the five essential things that a graph needs?
There are five important things that students should keep in mind while drawing a graph, and they are:
Axes and Background
Grid lines
Visual structures
Scales and tick marks
Text
2. What are the crucial elements of a useful graph?
Among different important elements, five principal features are explained below:
The experiment which describes the title
The graphical representation should fill the space allocated for the graph
Every data point should be arranged in a proper position
Each axes should be categorised with the measured quantity and the measuring units
A best-fitted line is required
3. What is a useful chart?
To make comparisons, Bar charts are the best option, whereas line charts are good for trends. For relationships and distribution, Scatter plot charts work better, but Pie charts are only utilised for compositions, not for any comparisons or distributions.
4. Where can I download the latest NCERT Solutions for Chapter 15 “Introduction to Graphs” of Class 8 Maths?
To download Vedantu's NCERT Solutions for Chapter 15 of Class 8 Maths follow these direct steps:
Visit the page NCERT Solutions for Chapter 15 of Class 8 Maths.
Scroll down and click on the option to "Download PDF."
The subsequent page will open that carries the link to download the PDF automatically.
These solutions are regularly updated as per the CBSE guidelines and syllabus. Hence, these are the latest solutions you can find online. Moreover, they are available to be downloaded free of charge.
5. Does Vedantu provide answers to all the questions of Chapter 15 “Introduction to Graphs” of Class 8 Maths?
In Chapter 15 "Introduction to Graphs," there are three exercises in total. Vedantu provides detailed answers to each question of these exercises. The solutions are explained step by step with graphs wherever required. Vedantu's NCERT Solutions for Class 8 Chapter 15 "Introduction to Graphs" provide solutions that are well suited for examinations. These are prepared after a painstaking process by our experts and hence are reliable. Moreover, Vedantu provides additional explanations of the chapter's concepts to allow students to comprehend the chapter better. These solutions are available free of cost on the Vedantu website and the Vedantu app.
6. How many types of graphs are there in Chapter 15 “Introduction to Graphs” of Class 8 Maths?
Chapter 15 "Introduction to Graphs" teaches the students the basic types and functions of graphs. The various types of graphs introduced in this chapter are as follows:
Bar graphs: to compare various categorical data
Histograms: to represent data in intervals
Pie graphs: to compare parts of a whole
Line graphs: to show continuous change over a certain duration
Linear graphs: a whole unbroken line graph
These graphs have several real-time applications, and thus students should pay close attention to this chapter.
7. How do you draw a linear graph?
To draw a linear graph, concepts of plotting points on graph paper, coordinates, and location of points must be clear in your mind.
Choose a suitable scale for the x and y-axis.
Mark the required quantities accordingly on the x and y-axis.
As per the quantities given in the question, plot the points on the graph.
Join the plotted points. This should provide you with a straight line on the graph.
8. Why do we use graphs according to Chapter 15 of Class 8 Maths?
Graphs have infinite uses in our lives. They are used for visually representing accurate data for various purposes. Such a graphical representation of collected data is convenient to comprehend. Graphs can easily represent large quantities that might be difficult to display in text.
You might have often come across various graphs on news channels, magazines, newspapers, etc. They can be used to depict temperature and weather changes, display annual reports, profits, losses, scores in sports, etc.
9. What is the basic introduction to graphs in Class 8?
In class 8 introduction to graphs, graphs are visual representations of data that show the relationship between different sets of information. They help in understanding and interpreting data quickly and easily. In Class 8, students learn about plotting points on the Cartesian plane and various types of graphs used to represent data.
10. How many types of graphs are there in Maths Class 8 introduction to graphs?
In the class 8 maths chapter introduction to graphs, there are several types of graphs, including:
Line Graphs: Used to show trends over time.
Bar Graphs: Used to compare quantities across different categories.
Histograms: Used to represent the distribution of data over a continuous interval.
Pie Charts: Used to show proportions of a whole.
Scatter Plots: Used to show the relationship between two variables.
11. Who introduced graphs in the class 8 maths chapter introduction to graphs?
Graphs discussed in class 8 maths chapter introduction to graphs were introduced by the Scottish mathematician William Playfair in the late 18th century. He is credited with inventing several types of graphs, including the line graph, bar graph, and pie chart, which are commonly used today.
12. What is the origin of the graph in the introduction to Graphs class 8 pdf solutions?
The origin in a graph is the point where the x-axis and y-axis intersect. It is denoted by the coordinates (0,0)(0, 0)(0,0) and serves as the reference point for plotting other points on the Cartesian plane.
13. What is a line graph in the introduction of graph class 8?
A line graph shown in the introduction of Graph Class 8 is a type of graph used to display information that changes over time. It consists of points plotted on the graph that are connected by straight lines. Line graphs are useful for showing trends and patterns in data.
14. What are graphs class 8 introduction to graphs?
In the introduction to Graphs class 8 pdf solutions, graphs are introduced as tools for representing data visually. Students learn about different types of graphs, how to plot points on the Cartesian plane, and how to interpret data from graphs. Graphs in Introduction to Graphs Class 8 pdf solutions introduction to graphs help in understanding the relationship between variables and making data-driven decisions.
15. What is an example of a graph mentioned in the introduction to graphs class 8?
An example of a graph in Introduction to Graphs class 8, is a line graph showing the temperature changes over a week. The x-axis represents the days of the week, and the y-axis represents the temperature. Points are plotted for each day's temperature and connected by lines to show the trend over the week.