
How many
Answer
491.7k+ views
Hint: The net resistance for two resistors of resistances
Complete step by step solution:
Let’s find out how a parallel resistance circuit works. A parallel resistance circuit is made below.
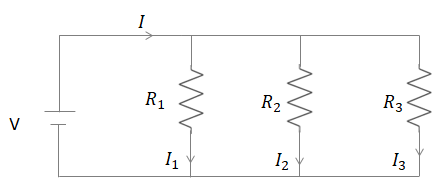
The voltage source is (V) and the net amount of current flowing through the circuit is (I). There are three resistances:
The values of the individual amount of current through each resistance, can be found through the Ohm’s law:
Hence,
Let’s consider a special case of the resistances connected in parallel. In this case, the resistances are of same value, that is:
Using the same formulation for N branches, we get:
For the current problem, we have multiple
Hence, 4 of the
Note: It’s important to remember that in a parallel resistance circuit connection, the voltage drawn by all the resistances are equal. An everyday example of this is the way electricity reaches all our houses. The constant 220V voltage is supplied to all the households in a locality, since all the houses are in a parallel connection to each other.
Complete step by step solution:
Let’s find out how a parallel resistance circuit works. A parallel resistance circuit is made below.

The voltage source is (V) and the net amount of current flowing through the circuit is (I). There are three resistances:
The values of the individual amount of current through each resistance, can be found through the Ohm’s law:
Hence,
Let’s consider a special case of the resistances connected in parallel. In this case, the resistances are of same value, that is:
Using the same formulation for N branches, we get:
For the current problem, we have multiple
Hence, 4 of the
Note: It’s important to remember that in a parallel resistance circuit connection, the voltage drawn by all the resistances are equal. An everyday example of this is the way electricity reaches all our houses. The constant 220V voltage is supplied to all the households in a locality, since all the houses are in a parallel connection to each other.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Franz thinks Will they make them sing in German even class 12 english CBSE




