Question

A lateral edge of a regular rectangular pyramid is a cm long. The lateral edge makes an angle $\alpha $ with the plane of the base. For what $\alpha $ is the volume of the pyramid the greatest?

Answer 1

Hint: In this question, we need to evaluate the value of $\alpha $ at which the volume of the pyramid is the greatest such that the lateral edge makes an angle $\alpha $ with the plane of the base. To solve this type of question, find the volume of the pyramid in terms of angle α and then equate the first derivative of the volume of the pyramid to zero and make sure the second derivative should be less than zero.

Complete step-by-step answer:
Given that there is a regular rectangular pyramid whose lateral edge makes an angle α with the plane base.

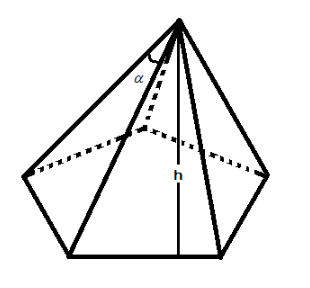
We have to find the value of α for which the volume of the regular rectangular pyramid has the greatest volume.
Suppose l, b, and h are the length breadth and the height if the regular rectangular pyramid
So, the Volume of rectangular pyramid is given as:
 \[V = \dfrac{{lbh}}{3}\]
Now applying the trigonometric ratio property, we get
 \[
  sin\alpha = \dfrac{h}{a} \\
   \Rightarrow h = asin\alpha \;
 \]
And, \[l = b = 2a\]
On putting the above values of length breadth and height, we get,
 \[V = \dfrac{{4{a^3}sin\alpha }}{3}\]
Now find the first derivative of the volume with respect to $\alpha $, we get
 \[\dfrac{{dV}}{{d\alpha }} = \dfrac{{4{a^3}cos\alpha }}{3}\]
For maxima, the second derivative should be less than zero. So, the second derivative is
 \[
  \dfrac{{{d^2}V}}{{d{\alpha ^2}}} = - \dfrac{{4{a^3}sin\alpha }}{3} \\
   \Rightarrow \dfrac{{{d^2}V}}{{d{\alpha ^2}}} < 0 \;
 \]
Which fulfills the criteria of maximum.
Now equate the first derivative of volume to zero and find the value α
∴ \[
  \dfrac{{dV}}{{d\alpha }} = 0 \\
   \Rightarrow \dfrac{{4{a^3}cos\alpha }}{3} = 0 \\
   \Rightarrow 4{a^3}cos\alpha = 0 \;
 \]
Here
 \[4{a^3} \ne 0\;\] then,
 \[cos\alpha = 0\]
We know \[cos\alpha \] can be zero when
 \[\therefore \alpha = \dfrac{\pi }{2}\;or\;\dfrac{{3\pi }}{4}\]
Hence, for \[\alpha = \dfrac{\pi }{2}\;or\;\dfrac{{3\pi }}{4}\] , the volume of regular rectangular pyramid will be greatest.
So, the correct answer is “ \[\alpha = \dfrac{\pi }{2}\;or\;\dfrac{{3\pi }}{4}\] ”.

Note: It is worth noting down here that the all the slant height of the regular pyramid will make an angle of $\alpha $ with the base of the pyramid. Here, \[cos\alpha = 0\] can be zero for n values of $\alpha $ but we have taken only two values because for a regular rectangular pyramid $\alpha $ can vary from 0 to $\pi $ only.