
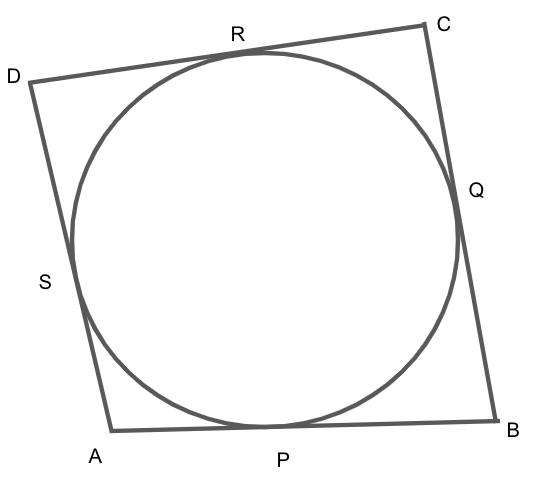
A quadrilateral ABCD is drawn to circumscribe a circle (see Figure). Prove that

Answer
494.7k+ views
Hint: First, we will use the tangents drawn from an exterior point to a circle are equal in length and then add the obtained equations. Then we will use the
Complete step by step answer:
We are given that a quadrilateral ABCD is drawn to circumscribe a circle.
We know that tangents drawn from an exterior point to a circle are equal in length, so we have
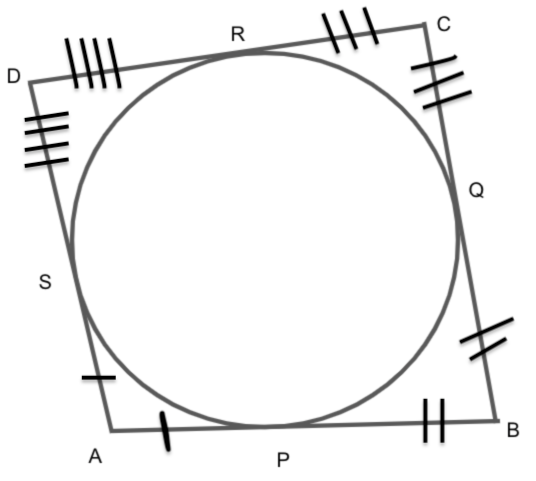
Adding the equations (1), (2), (3) and (4), we get
Rewriting the above equation, we get
Since we also know that AB is a line segments and when any point P cuts this line segments, then
From the same procedure, we get
Using these sums from the given diagram in the equation (5), we get
Hence, proved.
Note: In this question, we know that circumscribes a circle is to draw on the outside of just touching the corner points but never crossing. Here a quadrilateral is circumscribed around a circle. Students must crack the point of using that tangents drawn from an exterior point to a circle are equal in length. If we are able to crack this point, then the proof is very simple.
Complete step by step answer:
We are given that a quadrilateral ABCD is drawn to circumscribe a circle.
We know that tangents drawn from an exterior point to a circle are equal in length, so we have

Adding the equations (1), (2), (3) and (4), we get
Rewriting the above equation, we get
Since we also know that AB is a line segments and when any point P cuts this line segments, then
From the same procedure, we get
Using these sums from the given diagram in the equation (5), we get
Hence, proved.
Note: In this question, we know that circumscribes a circle is to draw on the outside of just touching the corner points but never crossing. Here a quadrilateral is circumscribed around a circle. Students must crack the point of using that tangents drawn from an exterior point to a circle are equal in length. If we are able to crack this point, then the proof is very simple.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




