Question

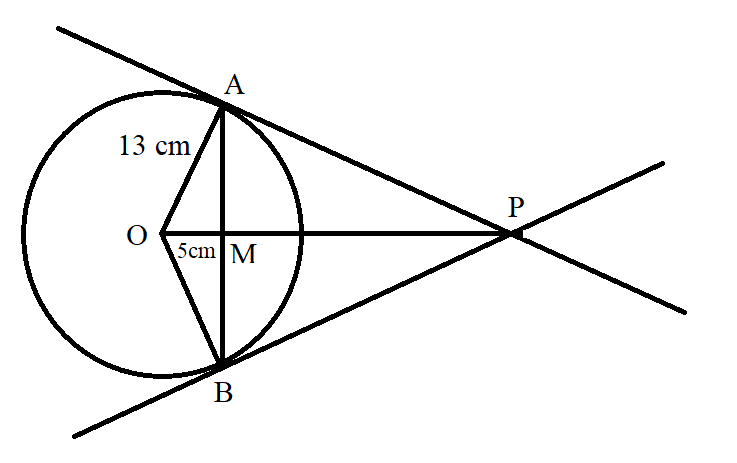
AB is a chord of circle with center ‘O’ having a radius of $13cm$. The perpendicular distance between the chord AB and the center ‘O’ is $5cm$. P is an external point in the plane of the circle where $PO = 85cm$. Find the perimeter of the triangle PAB.

Answer 1

Hint: The given question revolves around the concepts of analytical geometry and Pythagoras theorem. We have to solve two right angled triangles that are formed in the figure when the length of other two sides are given. We have to find the length of the third side of the given right angled triangle. This can be done easily using the Pythagoras theorem. However, we first need to know the position of the right angle in the right angled triangle before applying the Pythagoras theorem.

Complete step-by-step solution:
In the given question, we are given a circle with center O and radius $13cm$. The perpendicular distance between the chord and the center of the circle is given as $5cm$. So, we know the lengths of two sides of the triangle AMO. Also, we know that OM is perpendicular to AM. Hence, the angle $\angle AMO$ is a right angle. So, we can apply the Pythagoras theorem in the right angled triangle AMO.
So, according to Pythagoras theorem,
\[{\left( {Hypotenuse} \right)^2} = {\left( {Base} \right)^2} + {\left( {Altitude} \right)^2}\]
\[ \Rightarrow {\left( {AO} \right)^2} = {\left( {AM} \right)^2} + {\left( {OM} \right)^2}\]
Now, substituting in the lengths of the sides of triangle, we get
\[ \Rightarrow {\left( {13cm} \right)^2} = {\left( {AM} \right)^2} + {\left( {5cm} \right)^2}\]
Calculating the squares of the terms, we get,
\[ \Rightarrow 169c{m^2} = {\left( {AM} \right)^2} + 25c{m^2}\]
Simplifying the expression,
\[ \Rightarrow {\left( {AM} \right)^2} = 169c{m^2} - 25c{m^2} = 144c{m^2}\]
Taking square root on both sides of the equation, we get,
\[ \Rightarrow AM = 12cm\]
So, the length of AM is $12cm$.
Now, we know that the perpendicular from the center to a chord also bisects the chord. So, OM also bisects the chord AB. Hence, the length of AM and BM portions of the line segment are equal.
So, \[BM = AM = 12cm\]
Adding the lengths of both the portions, we get the length of AB as $24cm$.
Now, we have to find the length of the other two sides of the triangle PAB in order to find the perimeter.
Now, we are given that the length of PO is $85cm$.
So, in triangle POA, we know the lengths of two sides of the triangle. Also, PA is a tangent to the circle. We also know that the radius OA is perpendicular to the tangent PA. Hence, the angle $\angle OAP$ is a right angle. So, we can apply the Pythagoras theorem in the right angled triangle APO.
So, we get, \[{\left( {Hypotenuse} \right)^2} = {\left( {Base} \right)^2} + {\left( {Altitude} \right)^2}\]
\[ \Rightarrow {\left( {OP} \right)^2} = {\left( {AP} \right)^2} + {\left( {OA} \right)^2}\]
Now, substituting in the lengths of the sides of triangle, we get
\[ \Rightarrow {\left( {85cm} \right)^2} = {\left( {AP} \right)^2} + {\left( {13cm} \right)^2}\]
Calculating the square of the terms, we get,
\[ \Rightarrow 7225c{m^2} = {\left( {AP} \right)^2} + 169c{m^2}\]
Simplifying the equation,
\[ \Rightarrow {\left( {AP} \right)^2} = 7225c{m^2} - 169c{m^2}\]
\[ \Rightarrow {\left( {AP} \right)^2} = 7056c{m^2}\]
Taking square root on both sides of the equation, we get,
\[ \Rightarrow AP = 84cm\]
So, we get the length of the line segment AP as \[84cm\].
Now, AP and BP are the tangents from the same external point to the circle. We also know that the tangents to the circle from the same external point are equal in length. So, we get the length of AP and BP line segments as equal. So, $AP = BP = 84cm$.
Now the perimeter of triangle PAB is the sum of all sides of the triangle.
So, perimeter$ = AP + PB + BA$
$ = 84cm + 84cm + 24cm$
Adding up the terms, we get,
$ = 192cm$
So, the perimeter of the triangle PAB is $192cm$.

Note: For solving such type of question, where we need to find the third side of a triangle using the Pythagoras theorem, we need to know the position of right angle in the triangle beforehand since we need to know which of the three sides is the hypotenuse of the right angled triangle and then apply the Pythagoras theorem \[{\left( {Hypotenuse} \right)^2} = {\left( {Base} \right)^2} + {\left( {Altitude} \right)^2}\].