
A.
B.
C.
D.
Answer
492.3k+ views
Hint:
Lattice structures are topologically ordered, three-dimensional open-celled structures composed of one or more repeating unit cells [2,3]. These cells are defined by the dimensions and connectivity of their constituent strut elements, which are connected at specific nodes.This question can be solved by using the formula
Value of
Complete step by step answer:
Let’s deduce the expression
We know density of unit cell =mass of unit cell/volume of unit cell
Mass of a unit cell = number of atoms in unit cell
So,
From equation (1) & (2)
Mass of unit cell
Now we can write density of unit cell
So, equation (4) can be rewritten as
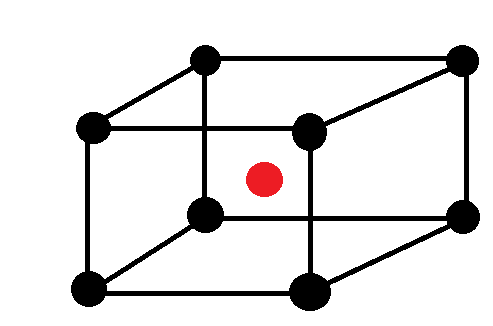
Now, Let’s calculate the number of atoms in the unit cell that is BCC.
Atoms at the corners are 8 and contribution of each corner atom is 1/8 and atom at centre is one and it is not shared with any other cell.
So,
As per question
Putting all values in equation (5) we get,
Note:
While deducing the formula
Lattice structures are topologically ordered, three-dimensional open-celled structures composed of one or more repeating unit cells [2,3]. These cells are defined by the dimensions and connectivity of their constituent strut elements, which are connected at specific nodes.This question can be solved by using the formula
Value of
Complete step by step answer:
Let’s deduce the expression
We know density of unit cell =mass of unit cell/volume of unit cell
Mass of a unit cell = number of atoms in unit cell
So,
From equation (1) & (2)
Mass of unit cell
Now we can write density of unit cell
So, equation (4) can be rewritten as

Now, Let’s calculate the number of atoms in the unit cell that is BCC.
Atoms at the corners are 8 and contribution of each corner atom is 1/8 and atom at centre is one and it is not shared with any other cell.
So,
As per question
Putting all values in equation (5) we get,
Note:
While deducing the formula
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Franz thinks Will they make them sing in German even class 12 english CBSE




