
How do ‘d’ orbitals split in an octahedral crystal field?
Answer
490.2k+ views
1 likes
Hint: All the valence orbitals of the metal cations are of the same energy(all the d orbitals have the same energy). We already know the crystal field theory called CFT. Crystal field theory states that the interaction between metal cations and ligands are electrostatic. The repulsive forces are the reason for the splitting of d orbitals.
Complete step by step answer:
We know that there are 5 lobes in the d orbital. They are
-Electrons are filled subsequently in all these lobes.
-All the orbitals have the same energy which means that they are degenerate. But when a ligand is approaching the metal, the degeneracy is lost.
-The given below is
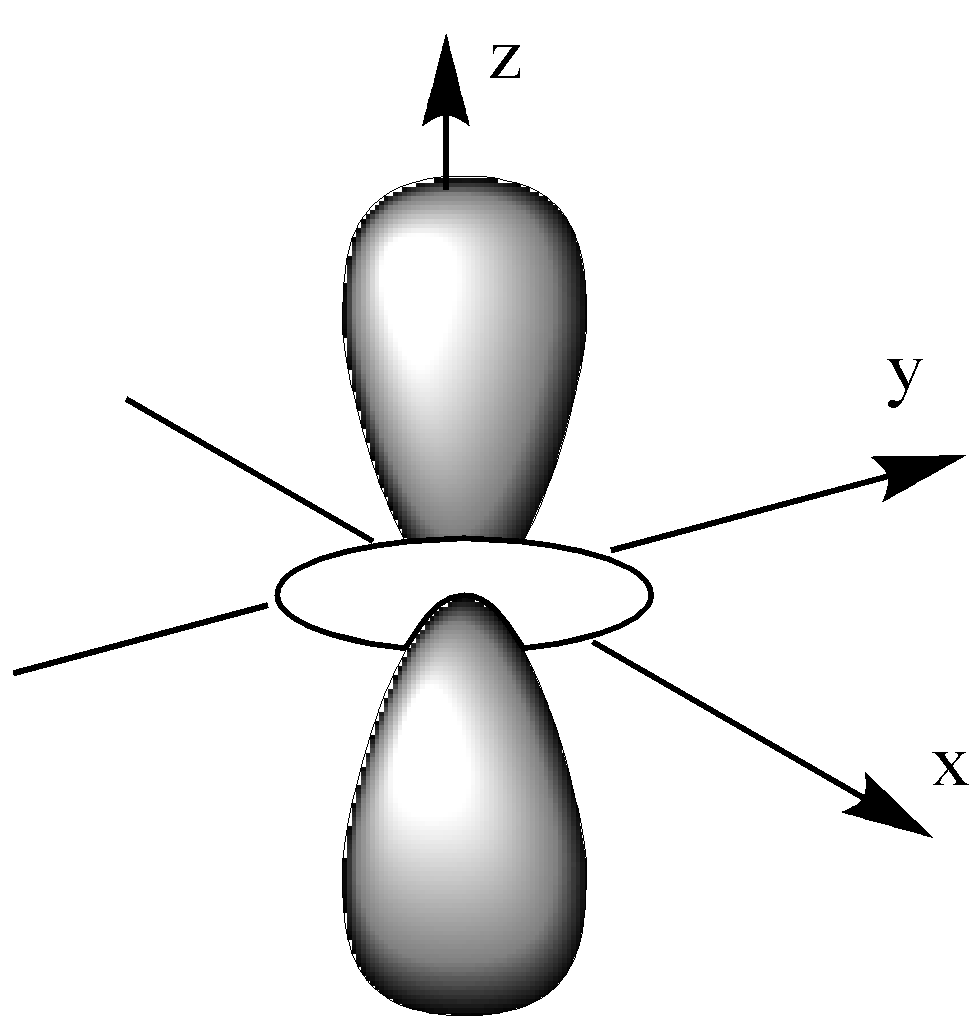
-The given below is
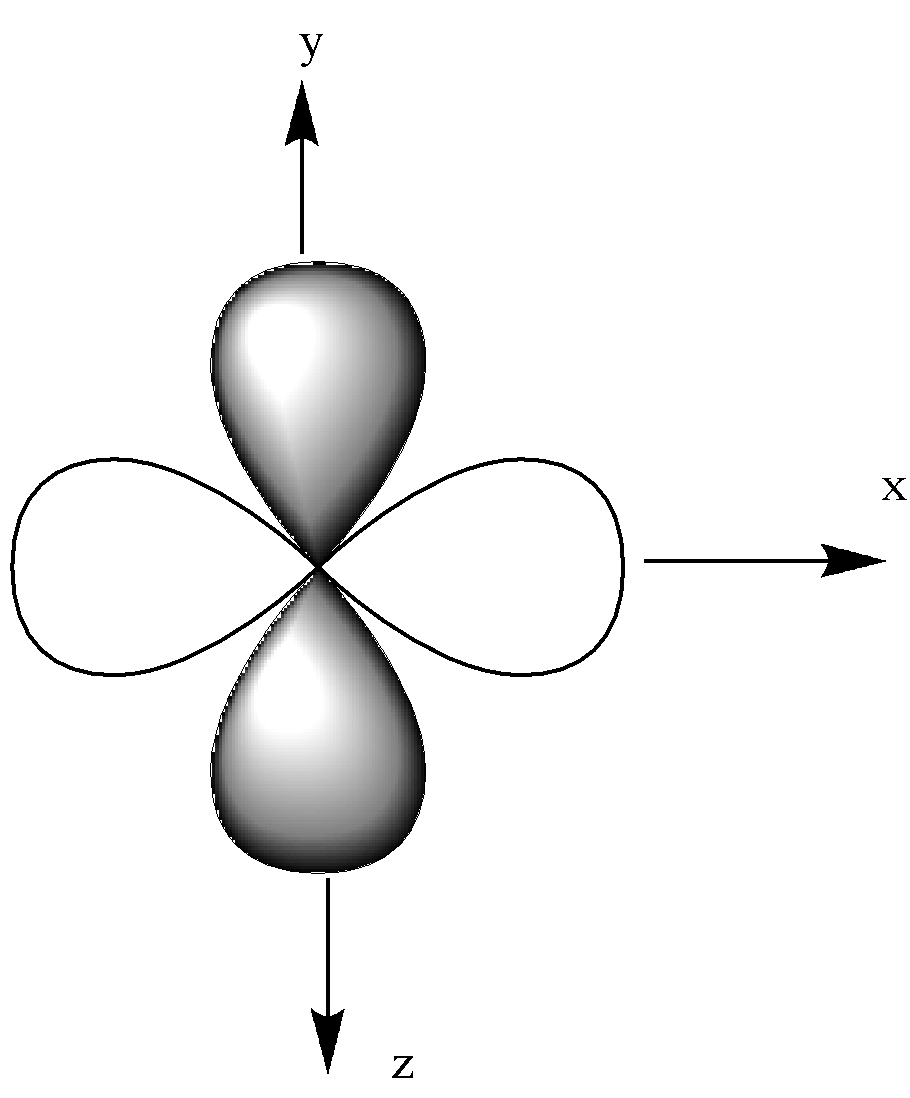
-Now, let us look at

-We already know that ligands are negatively charged and are coming to the metal cation through the axis.
-When a ligand is coming through the axis, the ligands overlap with
-As we have already learnt in CFT, this repulsive force leads to splitting of the d orbital into higher energy
-When ligands are approaching through the planes, it undergoes less repulsion and thus split into
-So, In an octahedral Crystal field, d orbitals split into higher energy
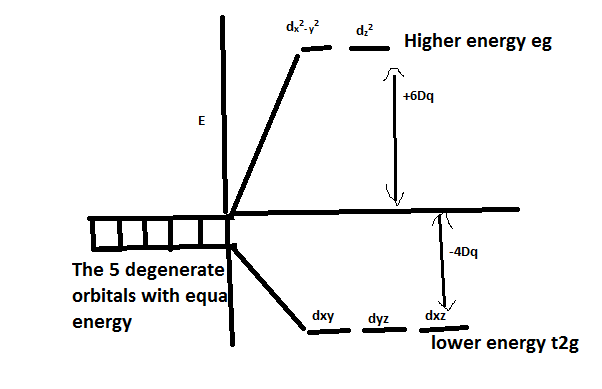
Note:The higher energy orbitals are those which undergo higher repulsion since its orbitals overlap with the approaching ligands. It is the opposite of the tetrahedral complex. In tetrahedral splitting, the higher energy is
Complete step by step answer:
We know that there are 5 lobes in the d orbital. They are
-Electrons are filled subsequently in all these lobes.
-All the orbitals have the same energy which means that they are degenerate. But when a ligand is approaching the metal, the degeneracy is lost.
-The given below is

-The given below is

It is in the x and y-axis.
-Now, let us look at

Similarly,
-We already know that ligands are negatively charged and are coming to the metal cation through the axis.
-When a ligand is coming through the axis, the ligands overlap with
-As we have already learnt in CFT, this repulsive force leads to splitting of the d orbital into higher energy
-When ligands are approaching through the planes, it undergoes less repulsion and thus split into
-So, In an octahedral Crystal field, d orbitals split into higher energy

Note:The higher energy orbitals are those which undergo higher repulsion since its orbitals overlap with the approaching ligands. It is the opposite of the tetrahedral complex. In tetrahedral splitting, the higher energy is
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹35,000 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Franz thinks Will they make them sing in German even class 12 english CBSE




