Question

Find the sum of all the angles at the five vertices of the adjoining star.

Answer 1

Hint: To solve the above question, we will first consider the regular pentagon formed inside this star. We will first calculate the sum of the interior angles in that polygon. Now, after obtaining the sum, we will divide the sum by 5 to get the measure of each interior angle of the pentagon. Now, we will consider the triangle while has base as one of the sides of the pentagon. In this triangle we will use the property that the sum of all the interior angles in a triangle is With the help of that property, we will find the angle at the vertex of the star. Now, we will multiply it by 5 to get the sum of all the angles at the vertices.

Complete step-by-step answer:
To start with, we will consider the pentagon ABCDE which is shown in the figure below.

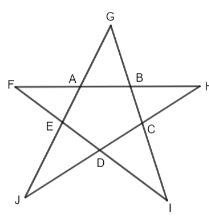
We will find the sum of the interior angles in this regular pentagon. We know that the sum of all the interior angles in a regular polygon with the number of sides ‘n’ is given by
\[\text{Sum of interior angles}=\left( n-2 \right)\times {{180}^{\circ}}\]
Thus, the sum of the interior angles in the polygon will be
\[\text{Sum of interior angles in pentagon}=\left( 5-2 \right)\times {{180}^{\circ}}\]
\[\Rightarrow \text{Sum of interior angles in pentagon}=3\times {{180}^{\circ}}\]
\[\Rightarrow \text{Sum of interior angles in pentagon}={{540}^{\circ}}\]
Now, as the pentagon is a regular pentagon, so all its interior angles are equal. Thus, each interior angle will be,
\[\text{Interior angle}=\dfrac{\text{sum of interior angles}}{5}\]
\[\Rightarrow \text{Interior angle}=\dfrac{{{540}^{\circ}}}{5}={{108}^{\circ}}\]
Thus, we can say that,
\[\angle EAB={{108}^{\circ}}\]
\[\angle CBA={{108}^{\circ}}\]
Now, we know that GAE is a straight line, so we can say that,
\[\angle GAB+\angle BAE={{180}^{\circ}}\]
Now,
\[\angle BAE=\angle EAB={{108}^{\circ}}\]
Thus, we will get,
\[\Rightarrow \angle GAB+{{108}^{\circ}}={{180}^{\circ}}\]
\[\Rightarrow \angle GAB={{180}^{\circ}}-{{108}^{\circ}}\]
\[\Rightarrow \angle GAB={{72}^{\circ}}....\left( i \right)\]
Similarly, we can say that GBC is also a straight line. So, we will have,
\[\angle GBA+\angle CBA={{180}^{\circ}}\]
\[\Rightarrow \angle GBA+{{108}^{o}}={{180}^{\circ}}\]
\[\Rightarrow \angle GBA={{180}^{\circ}}-{{108}^{\circ}}\]
\[\Rightarrow \angle GBA={{72}^{\circ}}......\left( ii \right)\]
Now, we will consider the triangle GAB. We know that in any triangle, the sum of the interior angles will be \[{{180}^{\circ}}.\] Thus, we can say that,
\[\angle GAB+\angle GBA+\angle AGB={{180}^{\circ}}\]
Now, we will put the value of \[\angle GAB\] and \[\angle GBA\] in the above equation from equations (i) and (ii). Thus, we get,
\[\Rightarrow {{72}^{\circ}}+{{72}^{\circ}}+\angle AGB={{180}^{\circ}}\]
\[\Rightarrow {{144}^{\circ}}+\angle AGB={{180}^{\circ}}\]
\[\Rightarrow \angle AGB={{180}^{\circ}}-{{144}^{\circ}}\]
\[\Rightarrow \angle AGB={{36}^{\circ}}\]
Now, all the interior angles at the vertices are equal to each other. So, the sum of all the angles at the vertices of the star will be equal to five times the angle at each vertex. Thus, we will get,
\[\text{Sum of angles}=5\times \left( \text{angle at one vertex} \right)\]
\[\text{Angle at vertex G}=\angle AGB={{36}^{\circ}}\]
Thus, we will get,
\[\text{Sum of angles}=5\times {{36}^{\circ}}\]
\[\Rightarrow \text{Sum of angles}={{180}^{\circ}}\]
Hence, the sum of all the angles at the give vertices of the adjoining star is \[{{180}^{\circ}}.\]

Note: While solving this question, we have assumed that the pentagon forming inside the star is a regular pentagon. This is because, in this question, we are not given anything about the sides of the star. Even if the pentagon becomes irregular, the sum of all the angles of the star will be \[=\left( 10-2 \right){{180}^{\circ}}={{1440}^{\circ}}\] but the angles at the vertex of the star will change.