
How do you graph
Answer
454.8k+ views
Hint: We use the given equation and assume it as a new variable. Substitute the value of ‘x’ and calculate the corresponding value of ‘y’ from the equation. Similarly, we can substitute values for ‘y’ and calculate the corresponding values for ‘x’ using the equation.
Complete step by step solution:
We have equation
Let us assume the equation as a variable say ‘y’
Then
We can divide both sides of the equation (1) by -2
Take exponential function on both sides of the equation
We will substitute the value of ‘y’ in the right hand side of equation (2) and calculate the corresponding value of ‘x’.
Put
Then
i.e.
Point becomes
Put
Then
i.e.
Point becomes
Now we plot the points
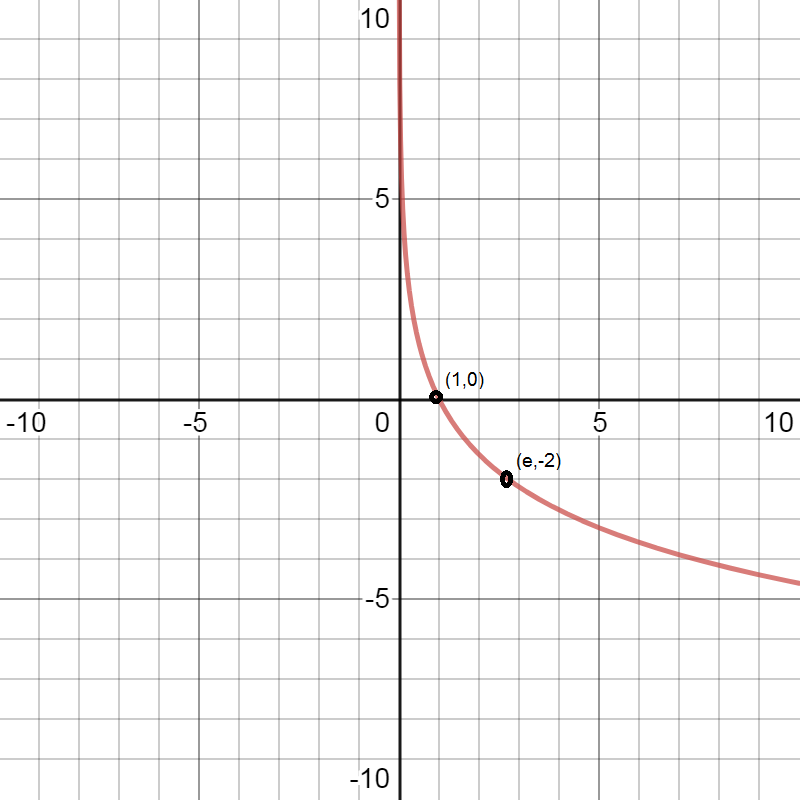
Note: Students are likely to make mistakes while shifting the values from one side of the equation to another side of the equation as they forget to change the sign of the value shifted. Keep in mind we always change the sign of the value from positive to negative and vice versa when shifting values from one side of the equation to another side of the equation. When plotting the points on the graph many students tend to mark wrong points due to the scale, keep in mind we can adjust the scale of the graph according to our need.
Alternate method:
Let us assume
Then we can write
Take exponential function on both sides
Then use the property of exponentials i.e.
Substitute the value of
Cancel exponent by log
When
So,
Point becomes
When
Then
i.e.
Point becomes
Now we plot the points
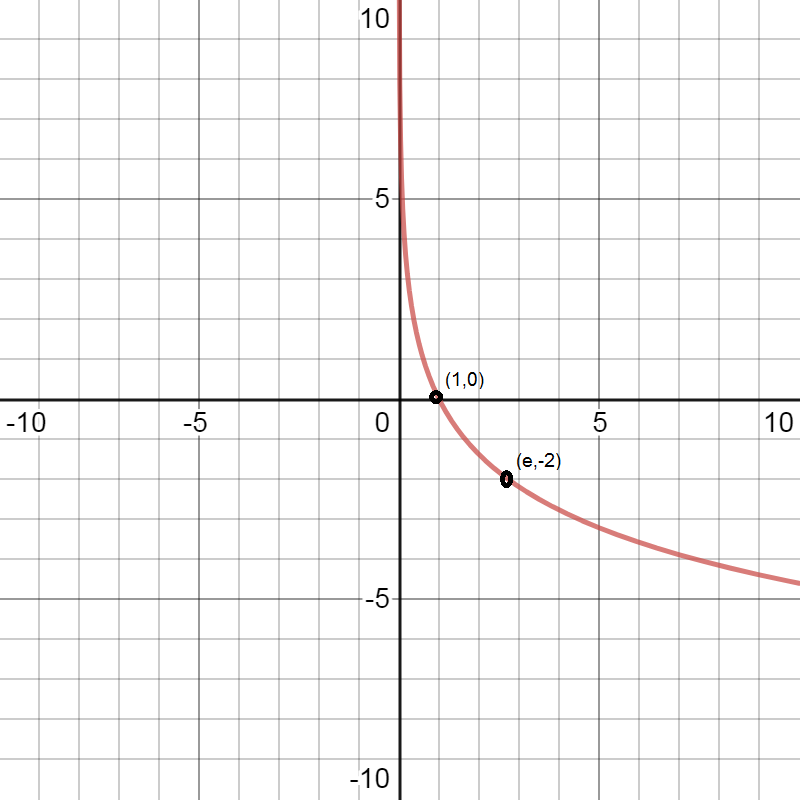
Complete step by step solution:
We have equation
Let us assume the equation as a variable say ‘y’
Then
We can divide both sides of the equation (1) by -2
Take exponential function on both sides of the equation
We will substitute the value of ‘y’ in the right hand side of equation (2) and calculate the corresponding value of ‘x’.
Put
Then
i.e.
Point becomes
Put
Then
i.e.
Point becomes
Now we plot the points

Note: Students are likely to make mistakes while shifting the values from one side of the equation to another side of the equation as they forget to change the sign of the value shifted. Keep in mind we always change the sign of the value from positive to negative and vice versa when shifting values from one side of the equation to another side of the equation. When plotting the points on the graph many students tend to mark wrong points due to the scale, keep in mind we can adjust the scale of the graph according to our need.
Alternate method:
Let us assume
Then we can write
Take exponential function on both sides
Then use the property of exponentials i.e.
Substitute the value of
Cancel exponent by log
When
So,
Point becomes
When
Then
i.e.
Point becomes
Now we plot the points

Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it





