
How do you graph y = csc(x – 45)?
Answer
461.7k+ views
Hint: Here, this problem is based on cosecant function. It is the function which is derived from basic trigonometric function i.e. sine. This equation has to be graphically represented. The angle is given in degrees i.e. 45.
Complete step-by-step solution:
Now, let’s discuss its solution.
We all are aware of the basic functions of trigonometry. They are: sine, cosine and tangent. These are the building blocks of trigonometry. When we talk about derived functions, we say secant, cosecant and cotangent. Secant is derived from cosine, cosecant is derived from sine whereas cotangent is derived from tangent. Let’s see the relationship between basic functions and derived functions.
Here,
Let’s see how we can find the value of sin
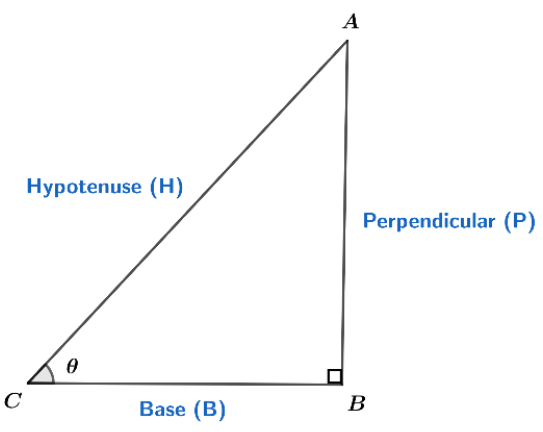
So,
As we know that cosec
Now, let’s see some even and odd functions.
Let’s draw the graph now for the equation:
y = csc(x – 45)
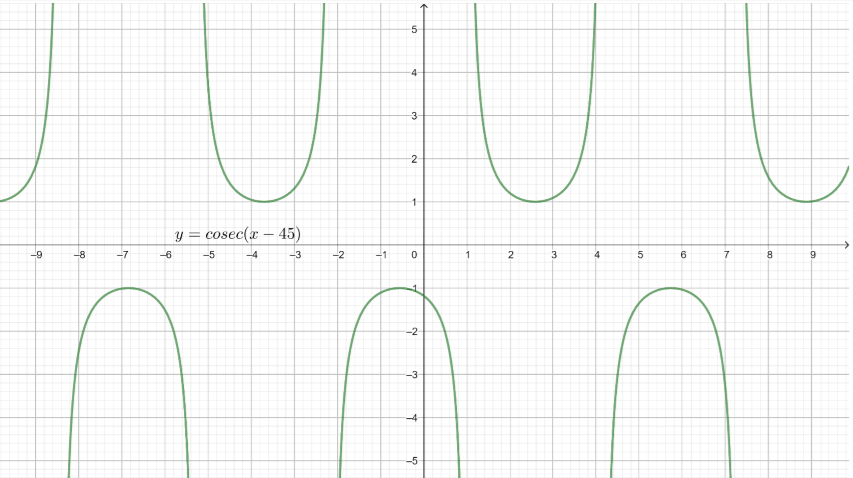
Note: Do mention the equation which you represented on the graph. And do sketch it neatly. You should know the basic trigonometric functions before solving anything related to the cosecant function.
Complete step-by-step solution:
Now, let’s discuss its solution.
We all are aware of the basic functions of trigonometry. They are: sine, cosine and tangent. These are the building blocks of trigonometry. When we talk about derived functions, we say secant, cosecant and cotangent. Secant is derived from cosine, cosecant is derived from sine whereas cotangent is derived from tangent. Let’s see the relationship between basic functions and derived functions.
Here,
Let’s see how we can find the value of sin

So,
As we know that cosec
Now, let’s see some even and odd functions.
Let’s draw the graph now for the equation:
y = csc(x – 45)

Note: Do mention the equation which you represented on the graph. And do sketch it neatly. You should know the basic trigonometric functions before solving anything related to the cosecant function.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Franz thinks Will they make them sing in German even class 12 english CBSE




