Question

If two altitudes of a triangle are equal in length, prove that it is an isosceles triangle.

Answer 1

Hint: In this question, we are given that the two altitudes of a triangle from the two different vertices are equal. Using this, we have to prove that the triangle is an isosceles triangle. We will use congruence to prove this. After the triangles have been proved congruent, using the rule of C.P.C.T. (corresponding parts of congruent triangles), the two sides can be proved equal. The student can use any pair of triangles for this.

Complete step-by-step answer:
We are given a triangle and two equal altitudes. Let us take the following.
 $\vartriangle ABC$ with $BD = CE$ (equal altitudes).

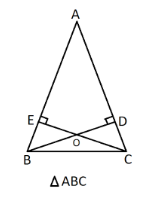
Now we will prove two triangles congruent.
Let us consider $\vartriangle BEC$ and $\vartriangle CDB$.
$BD = CE$ (given)
$BC = BC$ (common)
$\angle BEC = \angle CDB$ (each $90^\circ $)
Hence, $\vartriangle BEC \cong \vartriangle CDB$ (RHS rule)
$ \Rightarrow \angle EBC = \angle DCB$(corresponding parts of congruent triangles)
Using this,
$ \Rightarrow AB = AC$ (sides opposite to equal angles are equal)
Since two sides are equal, the triangle is an isosceles triangle.

If 2 altitudes of a triangle are equal then the triangle formed is an isosceles triangle.

Note: 1) The students can also use other methods to prove that the triangle is an isosceles triangle. For example-

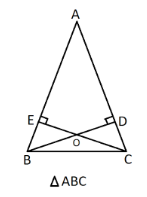
Consider $\vartriangle ABD$ and $\vartriangle ACE$.
$BD = CE$ (given)
$\angle BDA = \angle CEA$ (each $90^\circ $)
$\angle BAD = \angle CAE$(common)
Hence,$\vartriangle ABD \cong \vartriangle ACE$ (ASA rule)
$ \Rightarrow AB = AC$ (corresponding parts of congruent triangles)
Since the two sides of a triangle are equal, the triangle is an isosceles triangle.
2) While selecting a pair of triangles to prove the sides equal, it should be kept in mind that the triangles include the required side.
3) The RHS rule involves a right angle, hypotenuse and any one side.
ASA rule involves any one side and two corresponding angles.