Question

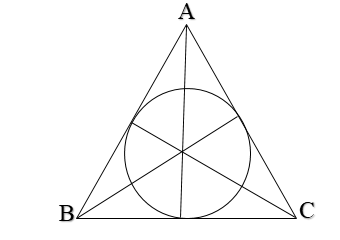
In an equilateral triangle of side 24 cm, a circle is inscribed touching its sides, find the areas of the remaining portion of the triangle.

Answer 1

Hint: Here to find the remaining area of the triangle, we consider the relation between area of triangle, semi- perimeter and radius of inner circle as the circle is inscribed in the triangle i.e.., $R = \dfrac{A}{s}$

Complete step-by-step answer:
We know the relation between area of triangle, semi-perimeter, radius of inner circle is $R = \dfrac{A}{s}$ , where A is area of triangle, s is semi perimeter, R radius of incircle.
Given, the side of an equilateral triangle is 24 cm.
Also, semi perimeter s can be given as $s = \dfrac{{24 + 24 + 24}}{2} = 36cm$.
The formula for the area A for the equilateral triangle is $\dfrac{{\sqrt 3 }}{2}{(side)^2}$ .
So, the area will be $\dfrac{{\sqrt 3 }}{2}{(24)^2} = 144\sqrt 3 c{m^2}$ .
Now, we have got the value of A and s, so we can easily calculate the value of R as follows: $R = \dfrac{{144\sqrt 3 }}{{36}} = 4\sqrt 3 cm$ .
Formula for area for the circle is $\pi {(radius)^2}$ since we have got the radius, we can move further.
Area of the circle will be $\pi {R^2} = \pi \times {4^2} \times 3 = 48\pi $ .
 Area of the remaining portion will be equal to the area of the circle subtracted from the total area of the triangle. Viz. $(144\sqrt 3 - 48)\pi c{m^2}$

Note: We can also put the value of $\pi $ as $\dfrac{{22}}{7}$ or $3.14$ for further simplification. While solving these types of problems we need to find the relations between the triangles and circles such that it makes the simplification easier.