Question

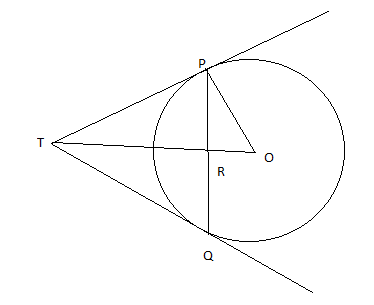
In figure, $PQ$ is a chord of length $8$ cm of a circle of radius $5$$cm$. The tangents at point $P$ and $Q$ intersect at a point $T$. The length of $TP$ is equal to $\dfrac{a}{3}$, then find the value of $a$.

Answer 1

Hint: In order to solve this question we have to apply the concept of Pythagoras theorem.
According to Pythagoras theorem, the square of the hypotenuse side is equal to the sum of the squares of the other two sides in a right-angled triangle.
By using the concept, we get the values
Finally we get the required answer.

Formula used: $Bas{e^2} + Perpendicula{r^2} = Hypotenus{e^2}$

Complete step-by-step answer:
It is stated in the question that the length of the chord $PQ$ is $8$$cm$ and the radius of the circle, that is , $OP = 5cm$
From the above diagram in $\vartriangle TPO$ and $\vartriangle PRO$ we get-
$\angle TPO = \angle PRO$ since both are right angles
$\angle OTP = \angle RPO$
So $\vartriangle TPO$ and $\vartriangle PRO$ are similar.
Therefore, $\dfrac{{TP}}{{PR}} = \dfrac{{OP}}{{OR}}$…..$(i)$
Since we have $PQ = 8cm$ therefore $PR = 4cm$ as the chord $PQ$ is bisected by $OT$ and also $OP = 5cm$
By applying Pythagoras theorem in $\vartriangle POR$ we can write-
 $P{R^2} + O{R^2} = O{P^2}$
Now, putting the values of $PR$ and $OP$ we get-
$\Rightarrow$${4^2} + O{R^2} = {5^2}$
Taking the find value as LHS and remaining as RHS, we get
$\Rightarrow$$O{R^2} = 25 - 16$
On subtracting we get,
$\Rightarrow$$O{R^2} = 9$
Taking square root on both sides we get,
So, $OR = \sqrt 9 = 3$
Now, substituting the values of $PR,OP,OR$ in equation $(i)$ we get-
$\Rightarrow$$\dfrac{{TP}}{4} = \dfrac{5}{3}$
So, $TP = \dfrac{{20}}{3}$
It is further given in the question that length of $TP$$ = \dfrac{a}{3}$
Therefore we can write that-
$\Rightarrow$$TP = \dfrac{a}{3} = \dfrac{{20}}{3}$
So,$\dfrac{a}{3} = \dfrac{{20}}{3}$
By doing cross-multiplication we get-
$\Rightarrow$$3a = 60$
On dividing $3$ on both side we get
$\Rightarrow$$a = \dfrac{{60}}{3} = 20$

Hence the value of $a$ is $20$

Note: Tangent is a straight line which touches the circle only at a particular point.
Point of tangency can be defined as the point where the tangent meets the circle.
The tangent is always perpendicular to the circle at the point of tangency.
It cannot touch the circle at two different points.
From an exterior point, the lengths of the tangents to the circle are equal.
The formula for finding out the tangent to the circle is
$\dfrac{{PR}}{{PS}} = \dfrac{{PS}}{{PQ}}$