Question

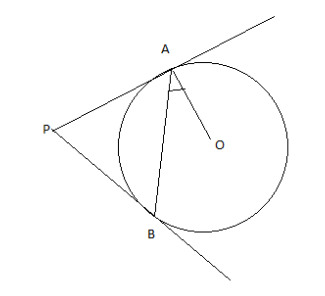
PA and PB are tangents to the circle with centre $O$ such that angle $\angle APB$ are equal to $\angle 50_{}^\circ$ write the measure of $\angle OAB$.

Answer 1

Hint: Since $PA$ and $PB$ are tangents to the circle with centre $O$ therefore $PA = PB$ and $\angle PAB = \angle PBA$.

Complete step-by-step answer:
Since we have $\angle PAB = \angle PBA$ therefore we can write that in the triangle$\Delta PAB$,
$\angle PBA + \angle PAB + \angle APB = 180_{}^\circ....\left( 1 \right)$
Since sum of all the angles of a triangle is $180_{}^\circ$
It is given that the $\angle APB = {50^ \circ }$
Now equation $\left( 1 \right)$ can be written as,
$2\angle PAB + 50_{}^\circ = 180_{}^\circ$
Since$\angle PAB = \angle PBA$
By moving $50_{}^\circ$ on the right side we get-
$2\angle PAB = 180_{}^\circ - 50_{}^\circ$
On subtracting we get,
$2\angle PAB = 130_{}^\circ$
Therefore, to find out the value of$\angle PAB$, we need to divide $130_{}^\circ$ by $2$
So, $\angle PAB = \dfrac{{130_{}^\circ}}{2}$
On dividing the terms we get,
$ = 65_{}^\circ$
Since $OA \bot PA$ so we can say that $\angle OAP$ is equal to $90_{}^\circ$
Therefore $\angle OAB = \angle OAP - \angle PAB = 90_{}^\circ - 65_{}^\circ$$ = 25_{}^\circ$ since $\angle PAB = 65_{}^\circ$

Thus the measure of $\angle OAB = 25_{}^\circ$

Note: We can define tangents as a single straight line which touches a single point in a circle. The point where tangents meet with the circle is known as the point of tangency.
The tangent is always perpendicular to the radius of the circle at the point where it meets with the circle.
Since tangent is a straight line therefore it also has an equation in slope form.
There are three conditions of the point of tangency when it lies outside the circle, when it lies inside the circle, and when the point lies on the circle.
Tangents can never intersect a circle.
Only one tangent can be drawn to a circle through a point which lies on the circle.
The lengths of two tangents which are drawn from an external point to a circle are always equal.
The basic formula which is used in case of solving of questions related to tangents is
$\dfrac{{PR}}{{PS}} = \dfrac{{PS}}{{PQ}}$