
State and explain Ampere’s circuital law.
Answer
491.4k+ views
1 likes
Hint: Ampere's circuital law states that the closed line integral of magnetic field around a current carrying conductor is equal to absolute permeability times the total current threading the conductor. We can formulate it mathematically and understand the concept well.
Complete step-by-step answer:
Amperes circulate law mathematically state that the line integral of magnetic field of induction B around any closed path in free space is equal to absolute permeability of free space
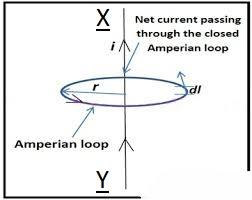
Mathematically, we can that the law as,
B is magnetic induction
I is the total current flowing through the wire
Dl is length element of path
where,
Note: Ampere's law gives us another method to calculate the magnetic field due to a given current distribution in a given fashion. Ampere's law can be derived from the Biot-Savart law and Biot-Savart law can also be derived from Ampere's law. Ampere's law is more useful under certain conditions where current distribution is symmetrical. We can calculate the magnetic field in a solenoid using this law.
Complete step-by-step answer:
Amperes circulate law mathematically state that the line integral of magnetic field of induction B around any closed path in free space is equal to absolute permeability of free space

Mathematically, we can that the law as,
B is magnetic induction
I is the total current flowing through the wire
Dl is length element of path
where,
Note: Ampere's law gives us another method to calculate the magnetic field due to a given current distribution in a given fashion. Ampere's law can be derived from the Biot-Savart law and Biot-Savart law can also be derived from Ampere's law. Ampere's law is more useful under certain conditions where current distribution is symmetrical. We can calculate the magnetic field in a solenoid using this law.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹35,000 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Franz thinks Will they make them sing in German even class 12 english CBSE




