Question

The base of an isosceles triangle is 6 cm and one of the equal sides is 12 cm. The radius of the circle through the vertices of the triangle is
A) \[\dfrac{{7\sqrt {15} }}{5}\]
B) \[4\sqrt 3 \]
C) \[3\sqrt 5 \]
D) \[6\sqrt 3 \]
E) None of these

Answer 1

Hint: Here first we will let the radius of the circle be $r$ and the altitude of the triangle to be $h$ such that it is equal to $x+r$ illustrated in the figure. Then we will apply the Pythagoras theorem to get the value of $r$.
The Pythagoras theorem states that the square of the hypotenuse is equal to the sum of squares of base and height of a right-angled triangle.
\[{\left( {hypotenuse} \right)^2} = {\left( {base} \right)^2} + {\left( {altitude} \right)^2}\]

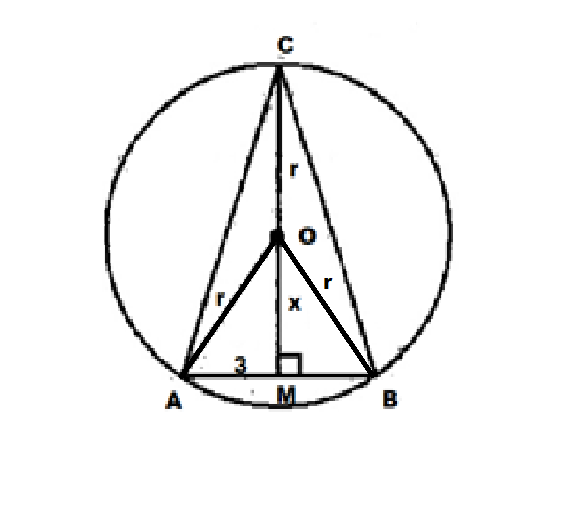
Complete step by step solution:
Let the radius of the circle
\[OA = OC = r\]
Let the height/altitude of the isosceles \[\Delta ABC\] be $h$ such that:
\[h = r + x\]……………………………..(1)
Now since it is given that the base of the triangle is 6cm
Hence,
\[AB = 6cm\]
which implies that:
\[AM = MB = 3cm\]
Also, it is given that one of the equal sides of the triangle is 12 cm
Therefore,
\[AC = 12cm\]
Now since \[\Delta AOM\] is another right angled triangle and r is the hypotenuse of the triangle
Therefore, applying Pythagoras theorem in \[\Delta AOM\]
According to Pythagoras theorem:
\[{\left( {hypotenuse} \right)^2} = {\left( {base} \right)^2} + {\left( {altitude} \right)^2}\]
Here,
$AC$ is the hypotenuse,
$AM$is the base,
$MC$ is the altitude.
Putting in the values we get:-
\[{\left( {AC} \right)^2} = {\left( {AM} \right)^2} + {\left( {MC} \right)^2} \]
${\left( {12} \right)^2} = {\left( 3 \right)^2} + {\left( h \right)^2}$
$ 144 = 9 + {h^2} $
${h^2} = 144 - 9$
$ {h^2} = 135 $
$ h = \sqrt {135} $
Now, applying Pythagoras theorem in \[\Delta AOM\]
$OA$ is hypotenuse,
$AM$ is base,
$OM$ is the altitude.
Therefore putting in the values in the formula of Pythagoras theorem we get:-
\[{r^2} = {3^2} + {x^2}\]
From equation 1 we get:-
\[x = h - r\]
Putting in this value in above equation we get:-
\[{r^2} = {3^2} + {\left( {h - r} \right)^2}\]
Now applying the following identity:
\[{\left( {a - b} \right)^2} = {a^2} + {b^2} - 2ab\]
We get:-
\[{r^2} = 9 + {h^2} + {r^2} - 2hr\]
\[2hr = 9 + {h^2} \]
Solving for $r$,
$ r = \dfrac{{9 + {h^2}}}{{2h}} $
Solving it further and putting the known values we get:-
\[\Rightarrow r = \dfrac{{9 + {{\left( {\sqrt {135} } \right)}^2}}}{{2\left( {\sqrt {135} } \right)}} \]
On simplifying the above values,
$\Rightarrow r = \dfrac{{9 + 135}}{{2\sqrt {135} }}$
On further simplifications, we get
$\Rightarrow r = \dfrac{{144}}{{2\sqrt {135} }}$
Now rationalizing the value of r by multiplying both the numerator and denominator by \[\sqrt {135} \] in order to make the denominator a whole number we get:-
\[\Rightarrow r = \dfrac{{144}}{{2\sqrt {135} }} \times \dfrac{{\sqrt {135} }}{{\sqrt {135} }}\]
Simplifying it further we get:-
\[\Rightarrow r = \dfrac{{144\sqrt {135} }}{{2 \times 135}} \]
$\Rightarrow r = \dfrac{{144\sqrt {135} }}{{270}} $
On further simplification,
$\Rightarrow r = \dfrac{{8 \times 3\sqrt {15} }}{{15}}$
$ r = \dfrac{{8\sqrt {15} }}{5} $

$\therefore$ The radius of the circle is \[\dfrac{{8\sqrt {15} }}{5}cm\]. Hence, option E is the correct option.

Note:
In an isosceles triangle, two sides are equal and the corresponding angles opposite to them are also equal.
Also, the altitude from a vertex to the opposite side bisects it into two halves.
The student can also consider $\Delta BOM$ instead of $\Delta AOM$ and then apply the Pythagoras theorem to get the value of $r$.