Answer
Verified473.4k+ views
Hint: It is given that the triangle is equilateral and perimeter is also given. So, first using perimeter we can find out the side of the triangle. The altitude bisects the base into two equal halves, hence using Pythagoras theorem we can find the height of the altitude.
Complete step by step answer:
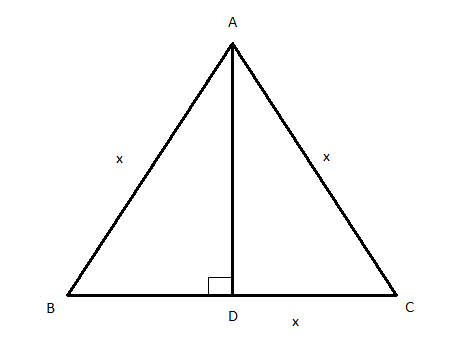
Given perimeter of \[\Delta ABC = 6...................................\left( 1 \right)\]
All sides of an equilateral triangle are equal. Let the measure of each side be \[x\].
Then \[x + x + x = 3x = 6{\text{ }}\left[ {\because {\text{equation}}\left( 1 \right)} \right]\]
Therefore, \[x = \dfrac{6}{3} = 2\]
Hence, AB = BC = AC = 2
Let AD be the altitude drawn on BC from A.
Since, D is the midpoint of BC, we have \[{\text{BD}} = {\text{CD}} = \dfrac{1}{2}{\text{BC}} = \dfrac{1}{2} \times 2 = 1..................................\left( 2 \right)\]
Now, in \[\Delta ADC\], we have \[\angle ADC = {90^0}\]
By Pythagoras theorem we have \[{\left( {{\text{Hypotenuse}}} \right)^2} = {\left( {{\text{Adjacent side}}} \right)^2} + {\left( {{\text{Opposite side}}} \right)^2}\]
So, using Pythagoras theorem in \[\Delta ADC\], we have
\[
\Rightarrow {\left( {AD} \right)^2} + {\left( {CD} \right)^2} = {\left( {AC} \right)^2} \\
\Rightarrow {\left( {AD} \right)^2} + {\left( 1 \right)^2} = {\left( 2 \right)^2}{\text{ }}\left[ {\because {\text{equation }}\left( 2 \right){\text{ and AC}} = 2} \right] \\
\Rightarrow {\left( {AD} \right)^2} + 1 = 4 \\
\Rightarrow {\left( {AD} \right)^2} = 4 - 1 = 3 \\
\therefore AD = \sqrt 3 \\
\]
Therefore, the length of the altitude is equal to \[\sqrt 3 \].
So, the correct answer is “Option D”.
Note: Equilateral triangle means the triangle has all the sides equal and all the angles are equal. Isosceles triangle is one having two equal sides and two equal angles. Pythagoras theorem says the hypotenuse square is equal to the sum of squares of its base and altitude. For solving questions like above these things are to be kept in mind.
Complete step by step answer:
Given perimeter of \[\Delta ABC = 6...................................\left( 1 \right)\]
All sides of an equilateral triangle are equal. Let the measure of each side be \[x\].
Then \[x + x + x = 3x = 6{\text{ }}\left[ {\because {\text{equation}}\left( 1 \right)} \right]\]
Therefore, \[x = \dfrac{6}{3} = 2\]
Hence, AB = BC = AC = 2
Let AD be the altitude drawn on BC from A.
Since, D is the midpoint of BC, we have \[{\text{BD}} = {\text{CD}} = \dfrac{1}{2}{\text{BC}} = \dfrac{1}{2} \times 2 = 1..................................\left( 2 \right)\]
Now, in \[\Delta ADC\], we have \[\angle ADC = {90^0}\]
By Pythagoras theorem we have \[{\left( {{\text{Hypotenuse}}} \right)^2} = {\left( {{\text{Adjacent side}}} \right)^2} + {\left( {{\text{Opposite side}}} \right)^2}\]
So, using Pythagoras theorem in \[\Delta ADC\], we have
\[
\Rightarrow {\left( {AD} \right)^2} + {\left( {CD} \right)^2} = {\left( {AC} \right)^2} \\
\Rightarrow {\left( {AD} \right)^2} + {\left( 1 \right)^2} = {\left( 2 \right)^2}{\text{ }}\left[ {\because {\text{equation }}\left( 2 \right){\text{ and AC}} = 2} \right] \\
\Rightarrow {\left( {AD} \right)^2} + 1 = 4 \\
\Rightarrow {\left( {AD} \right)^2} = 4 - 1 = 3 \\
\therefore AD = \sqrt 3 \\
\]
Therefore, the length of the altitude is equal to \[\sqrt 3 \].
So, the correct answer is “Option D”.
Note: Equilateral triangle means the triangle has all the sides equal and all the angles are equal. Isosceles triangle is one having two equal sides and two equal angles. Pythagoras theorem says the hypotenuse square is equal to the sum of squares of its base and altitude. For solving questions like above these things are to be kept in mind.